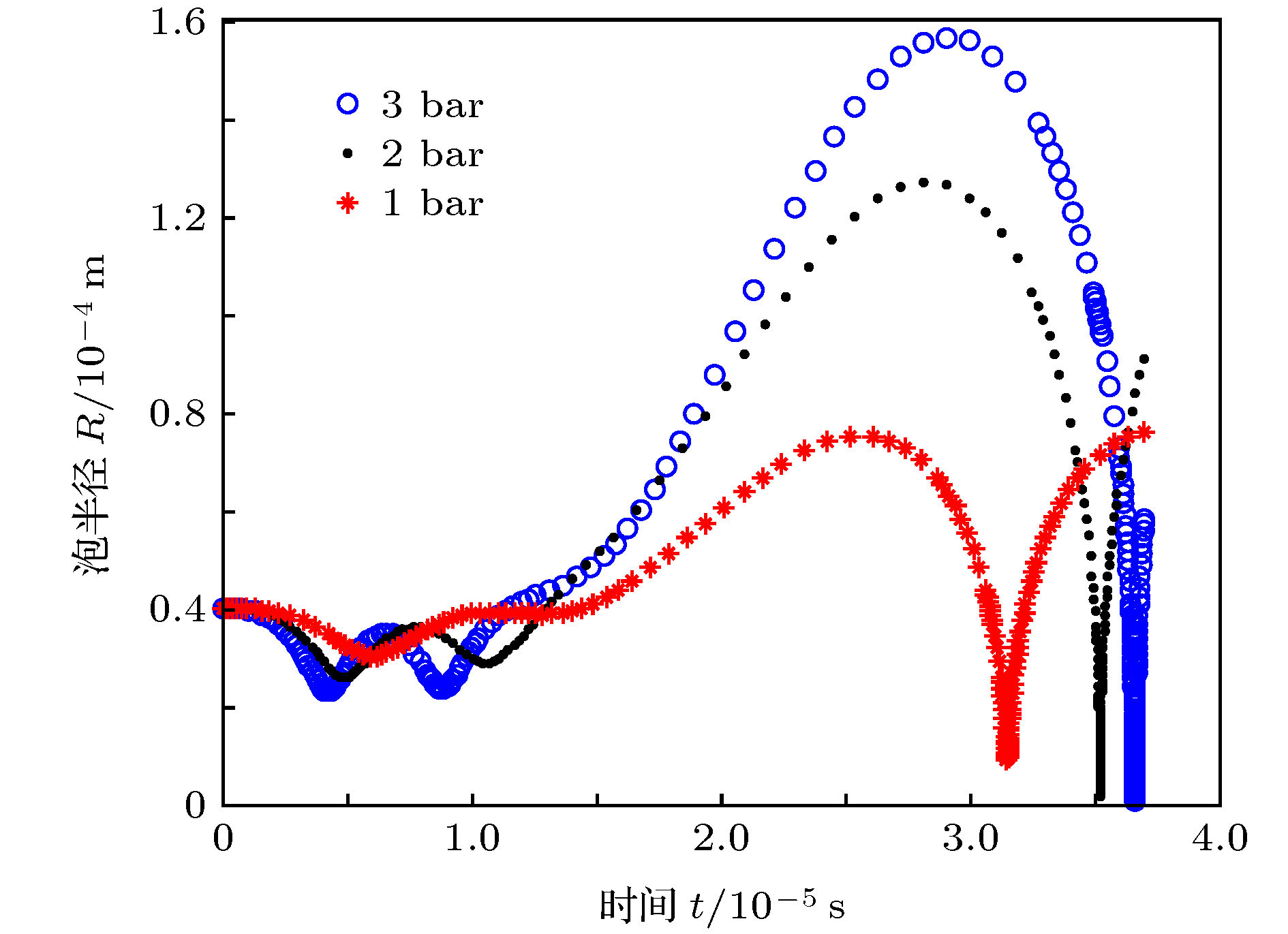
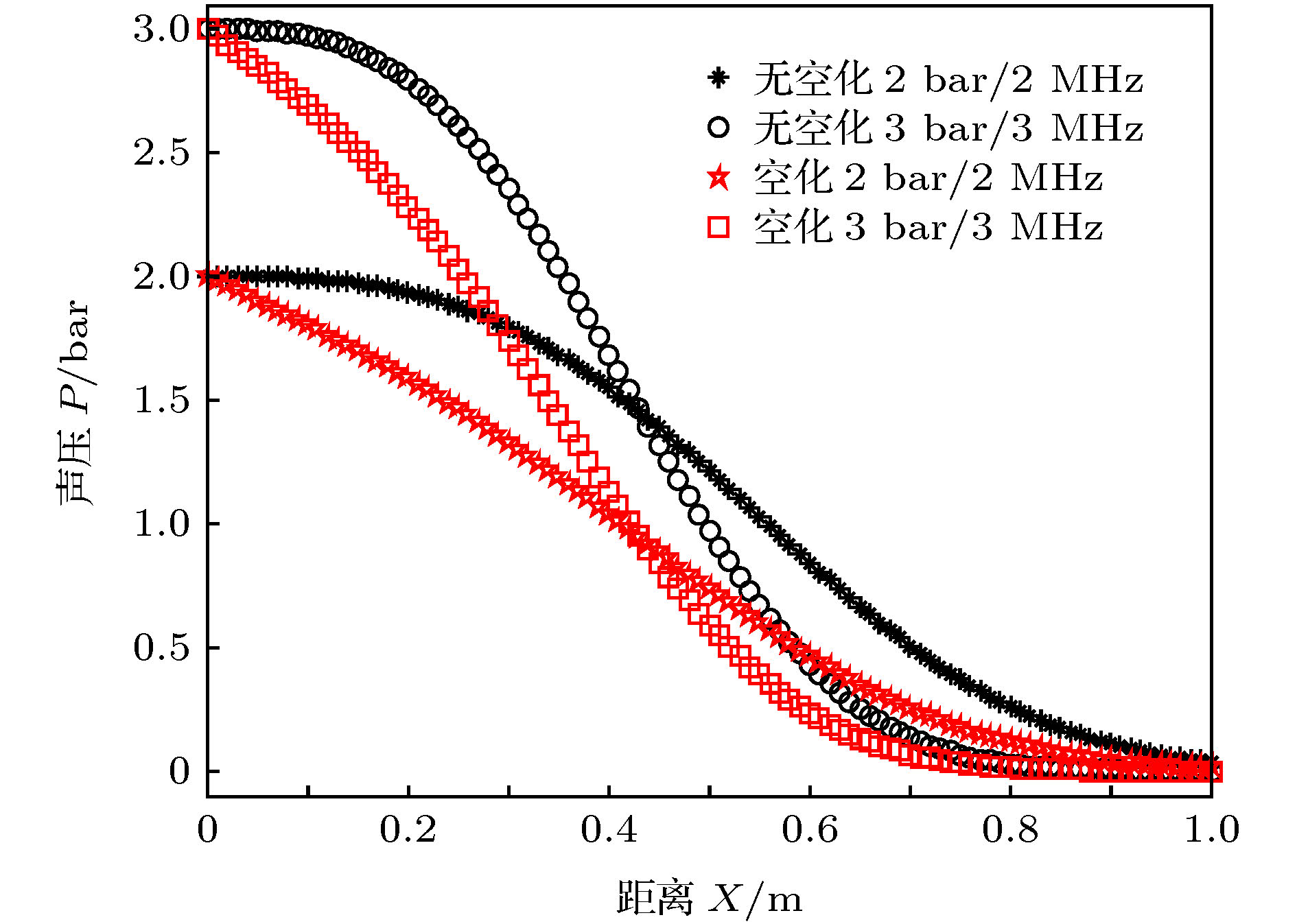
-
本文从广义的Navier-Stokes流体方程出发, 考虑到流体介质的黏滞性和存在的热传导, 导出了更接近实际流体的三维非线性声波动方程. 鉴于声传播所涉及的空间和时间尺度的复杂性和多样性, 文中针对一维情形下的非线性波动方程进行了求解和分析. 由方程的二级近似解可以看出, 声压振幅的衰减遵循几何级数规律, 而且驱动声波的频率越高声压的衰减就越快. 在满足条件
$\omega b \ll {\rho _0}c_0^2$ 时, 基波的衰减系数与驱动频率的平方及耗散系数的乘积成正比; 二次谐波的衰减规律更加复杂, 与频率的更高次幂相关. 对声衰减系数及声压的分布进行数值计算发现, 声压的分布还与初始的声压幅值及频率有关, 初始的声压与频率越高衰减得越快. 另外, 当声压高于液体的空化阈值时, 液体中就会出现大量的空化泡, 文中模拟了单个空化泡的运动, 发现随着声压的增大空化泡的振动越剧烈、空化泡所受的黏滞力变大, 随着声波作用时间的增大黏滞力的幅值迅速增大并与驱动声压值同阶, 因而空化泡的非线性径向运动引起的声衰减不容忽视. 结果表明, 驱动声压越高在空化区域附近引起的声衰减越快、输出的声压越低.-
关键词:
- 非线性超声 /
- Navier-Stokes方程 /
- 空化泡
Recent work has rendered possible the formulation for the nonlinear propagation of pressure waves in liquids by using the generalized Navier - Stokes equations and the modified equations of state, with the heat transfer and fluid viscidity taken into consideration. And the nonlinear approximation solution of the second order term is obtained. The conclusion concerns the acoustic pressure, phase speed, attenuation, and velocity distribution function. When the amplitude of driving acoustic pressure is higher than the cavitation threshold of the host liquid, the cavitation occurs. The cavitation bubbles will prevent the sound field from spreading in the liquid, and the acoustic energy accumulates near the cavitation zone. So when studying the transmission characteristics of acoustic wave in the liquid, the cavitation attenuation must be considered. Note that the particularity of cavitation bubble movement, cavitation bubble vibration and viscous force are simulated under the initial driving sound. Through the analysis, it is found that the transmission of sound is influenced by the viscosity of the fluid, heat transfer, driving sound pressure (amplitude, frequency, duration) and cavitation bubble in liquid. The physical mechanism is that the higher driving pressure causes the cavitation to turn stronger, the acoustic loss to be faster, the sound propagation distance to be smalletr and the vibration of bubbles to transfer energy from the fundamental wave to harmonics. As a result, the stronger absorption from the liquid causes abnormal phenomena, and the output sound is lower finally. It shows that the nonlinear radial motion of cavitation bubble is mainly responsible for the sound intensity attenuation.-
Keywords:
- nonlinear acoustics /
- Navier-Stokes equations /
- cavitation bubbles
[1] Louisnard O 2012 Ultrason. Sonochem. 19 56
 Google Scholar
Google Scholar
[2] Louisnard O 2012 Ultrason. Sonochem. 19 66
 Google Scholar
Google Scholar
[3] Ashokumar M 2011 Ultrason. Sonochem. 18 864
 Google Scholar
Google Scholar
[4] Wang X, Chen W Z, Liang S D, Zhao T Y, Liang J F 2017 Phys. Rev. E 95 033118
 Google Scholar
Google Scholar
[5] Wang X, Chen W Z, Y ang J, Liang S D 2018 J. Appl. Phys. 123 214904
 Google Scholar
Google Scholar
[6] 陈伟中 2018 应用声学 37 675
 Google Scholar
Google Scholar
Chen W Z 2018 J. Appl. Acoustics 37 675
 Google Scholar
Google Scholar
[7] Wijngaarden L V 1972 Ann. Rev. Fluid Mech. 4 369
 Google Scholar
Google Scholar
[8] Commander K W, Prosperetti A 1989 J. Acoust. Soc. Am. 85 732
 Google Scholar
Google Scholar
[9] Vanhille C, Cleofé C P 2011 Ultrason. Sonochem. 18 679
 Google Scholar
Google Scholar
[10] Thiessen R J, Cheviakov A F 2019 Commun. Nonliear. Sci. Numer. Simul. 73 244
 Google Scholar
Google Scholar
[11] Xu F S, Midoux N, Li H Z, Hébrard G, Dietrich N 2019 Chem. Eng. Technol. 42 2321
 Google Scholar
Google Scholar
[12] Zhang H H 2020 J. Acoust. Soc. Am. 147 399
 Google Scholar
Google Scholar
[13] Church C C 1995 J. Acoust. Soc. Am. 97 1510
 Google Scholar
Google Scholar
[14] Rayleigh L 1917 Philos. Mag. 34 94
 Google Scholar
Google Scholar
[15] Smerera P 2002 J. Fluid Mech. 454 287
 Google Scholar
Google Scholar
[16] 陈伟中 2014 声空化物理(北京: 科学出版社) 第58页
Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science Press) p58 (in Chinese)
[17] 钱祖文 2009 非线性声学 (北京: 科学出版社) 第29页
Qian Z W 2009 Nonliear Acoustics (Beijing: Science Press) p29 (in Chinese)
[18] 杜功焕, 朱哲民, 龚秀芬 2001 声学基础 (南京: 南京大学出版社) 第491页
Du G H, Zhu Z M, Gong X F 2001 Fundamentals of Sound (Nanjing: Nanjing University Press) p491 (in Chinese)
[19] 钱祖文 1981 30 442
 Google Scholar
Google Scholar
Qian Z W 1981 Acta Phys. Sin. 30 442
 Google Scholar
Google Scholar
[20] 沈壮志, 林书玉 2011 60 104302
 Google Scholar
Google Scholar
Shen Z Z, Lin S Y 2011 Acta Phys. Sin. 60 104302
 Google Scholar
Google Scholar
[21] 沈壮志, 林书玉 2011 60 084302
 Google Scholar
Google Scholar
Shen Z Z, Lin S Y 2011 Acta Phys. Sin. 60 084302
 Google Scholar
Google Scholar
[22] Tomko J, O’Malley S M, Trout C, Naddeo J J, Jimenez R, Griepenburg J C, Soliman W, Bubb D M 2017 Colloids Surf., A 522 368
 Google Scholar
Google Scholar
-
[1] Louisnard O 2012 Ultrason. Sonochem. 19 56
 Google Scholar
Google Scholar
[2] Louisnard O 2012 Ultrason. Sonochem. 19 66
 Google Scholar
Google Scholar
[3] Ashokumar M 2011 Ultrason. Sonochem. 18 864
 Google Scholar
Google Scholar
[4] Wang X, Chen W Z, Liang S D, Zhao T Y, Liang J F 2017 Phys. Rev. E 95 033118
 Google Scholar
Google Scholar
[5] Wang X, Chen W Z, Y ang J, Liang S D 2018 J. Appl. Phys. 123 214904
 Google Scholar
Google Scholar
[6] 陈伟中 2018 应用声学 37 675
 Google Scholar
Google Scholar
Chen W Z 2018 J. Appl. Acoustics 37 675
 Google Scholar
Google Scholar
[7] Wijngaarden L V 1972 Ann. Rev. Fluid Mech. 4 369
 Google Scholar
Google Scholar
[8] Commander K W, Prosperetti A 1989 J. Acoust. Soc. Am. 85 732
 Google Scholar
Google Scholar
[9] Vanhille C, Cleofé C P 2011 Ultrason. Sonochem. 18 679
 Google Scholar
Google Scholar
[10] Thiessen R J, Cheviakov A F 2019 Commun. Nonliear. Sci. Numer. Simul. 73 244
 Google Scholar
Google Scholar
[11] Xu F S, Midoux N, Li H Z, Hébrard G, Dietrich N 2019 Chem. Eng. Technol. 42 2321
 Google Scholar
Google Scholar
[12] Zhang H H 2020 J. Acoust. Soc. Am. 147 399
 Google Scholar
Google Scholar
[13] Church C C 1995 J. Acoust. Soc. Am. 97 1510
 Google Scholar
Google Scholar
[14] Rayleigh L 1917 Philos. Mag. 34 94
 Google Scholar
Google Scholar
[15] Smerera P 2002 J. Fluid Mech. 454 287
 Google Scholar
Google Scholar
[16] 陈伟中 2014 声空化物理(北京: 科学出版社) 第58页
Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science Press) p58 (in Chinese)
[17] 钱祖文 2009 非线性声学 (北京: 科学出版社) 第29页
Qian Z W 2009 Nonliear Acoustics (Beijing: Science Press) p29 (in Chinese)
[18] 杜功焕, 朱哲民, 龚秀芬 2001 声学基础 (南京: 南京大学出版社) 第491页
Du G H, Zhu Z M, Gong X F 2001 Fundamentals of Sound (Nanjing: Nanjing University Press) p491 (in Chinese)
[19] 钱祖文 1981 30 442
 Google Scholar
Google Scholar
Qian Z W 1981 Acta Phys. Sin. 30 442
 Google Scholar
Google Scholar
[20] 沈壮志, 林书玉 2011 60 104302
 Google Scholar
Google Scholar
Shen Z Z, Lin S Y 2011 Acta Phys. Sin. 60 104302
 Google Scholar
Google Scholar
[21] 沈壮志, 林书玉 2011 60 084302
 Google Scholar
Google Scholar
Shen Z Z, Lin S Y 2011 Acta Phys. Sin. 60 084302
 Google Scholar
Google Scholar
[22] Tomko J, O’Malley S M, Trout C, Naddeo J J, Jimenez R, Griepenburg J C, Soliman W, Bubb D M 2017 Colloids Surf., A 522 368
 Google Scholar
Google Scholar
计量
- 文章访问数: 14277
- PDF下载量: 268
- 被引次数: 0















 下载:
下载: