光电离过程中Fe靶和V靶特征辐射的角相关研究

柳钰; 徐忠锋; 王兴; 曾利霞; 刘婷

doi:10.7498/aps.69.20191524

摘要

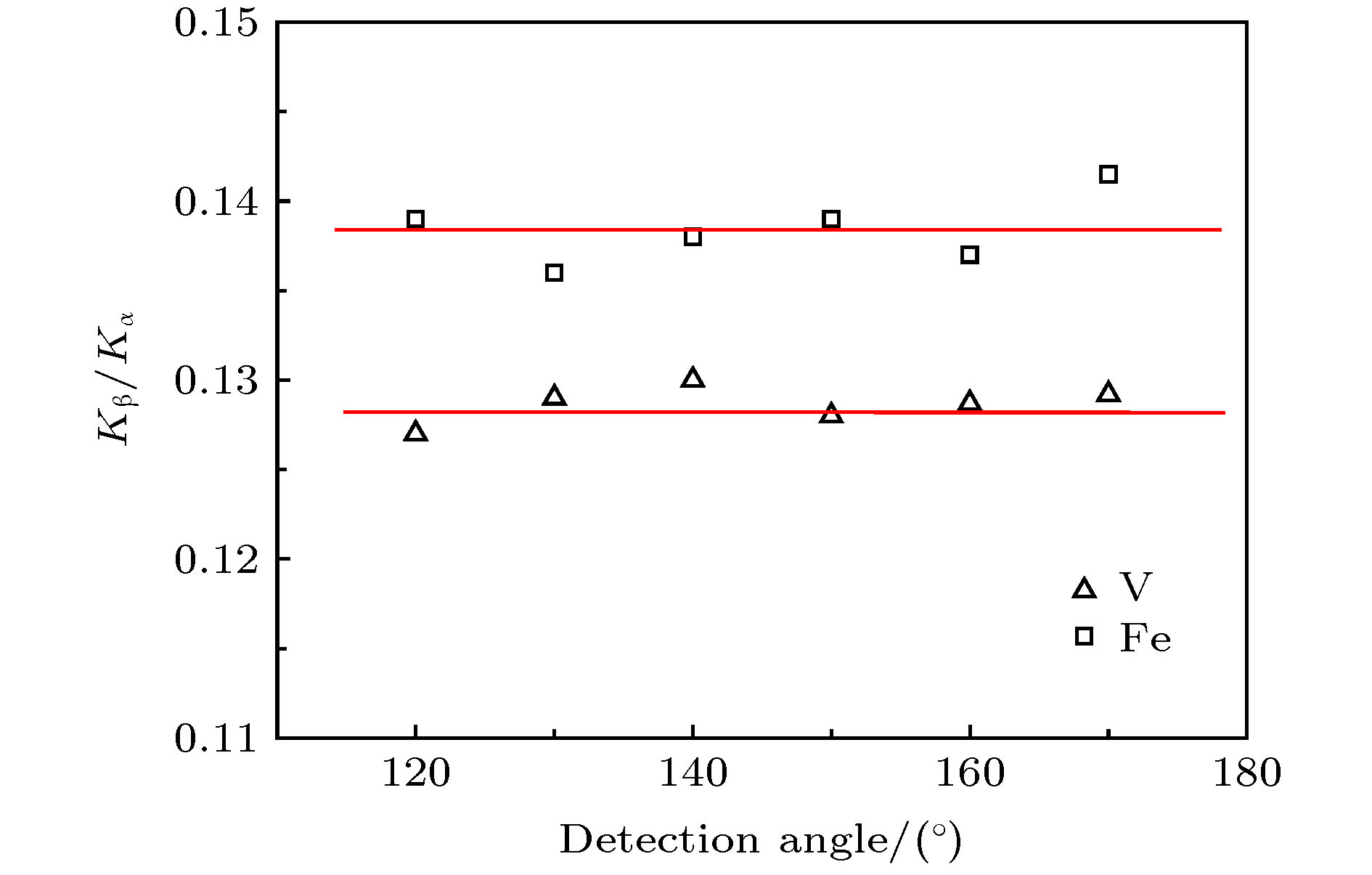
在发射角120°—170°的范围内, 应用硅漂移探测器以10°为间隔对中心能量为13.1 keV的韧致辐射诱发Fe靶和V靶发射的典型K系X射线光谱进行了测量. 得到特征X射线K_α和K_β的特征谱线, 考虑探测器对特征X射线的探测效率、靶对入射光子和出射光子吸收的校准及大气对特征X射线的吸收后, 结果显示不同探测角度下K_β与K_α的强度比为一常数. 将本次实验探测角度为150°时的K_β/K_α强度比值的实验值、理论计算值和Ertuğral的实验结果进行对比, 发现实验结果与预期相符. 对比不同探测角度下的强度比变化趋势推断特征X射线的角度依赖关系, 分析认为K_α和K_β在探测范围内是各向同性发射的.

关键词:

角分布 /
光电离 /
各向同性

Abstract

The de-excitation process of vacancy in the inner shell of the target atom caused by collision ionization produces the characteristic X-ray or Auger electrons. The precise measurement of ionization cross sections plays an important role in many basic research fields, as well as in practical fields, such as chemical analysis of Particle Induced X-ray Emission (PIXE), atomic and nuclear processes, and X-ray fluorescence (XRF) spectroscopy. As we know, when ionization cross sections are measured precisely, whether the emission of X-ray is isotropic in collision process must be considered. However, there have been few experimental results for angular dependence of K_β/K_α intensity ratios in the literature until now. Therefore, this study aims to verify that the K_α and K_β X-rays originated from filling of the K shell vacancies with total angular momentum quantum number 1/2 (J = 1/2) are isotropic. In this work, the typical K-shell X-ray spectra for Fe and V, which induced by bremsstrahlung with central energy of 13.1 keV, have been measured at emission angles varied from 120° to 170° at intervals of 10°. The characteristic X-ray spectra obtained by the detector are fitted by Gauss function, where the absorption of incident X-rays by the detector, the absorption of emitted X-rays by the atmosphere and the self-absorption correction factor of incident and emitted X-rays by the target are all taken into account. The experimental results of K_β/K_α intensity ratio in this experiment coincide with those of theoretical calculation, as well as the Ertuğral’s experimental result. The experimental results show that the intensity ratio of K_β/K_α is a constant at different detection angles. Therefore it can be concluded that the emission of K_α and K_β is isotropic in the detection range. Since the K shell has no sub-shell, there is no Coster-Kronig transition in the collision ionization process. In the process of photoionization, the vacancies in the K shell are produced by direct ionization. As a result, the cross section ratio of K shell X-ray generation is independent of the K shell photoionization cross section. In addition, the experimental results show that the K_β/K_α characteristic X-ray intensity ratio of target Fe is 8% higher than that of target V, which are consistent with the theoretical analysis results that the characteristic X-ray intensity ratio depends on the target atomic number Z.

Keywords:

作者及机构信息

1.
西安交通大学理学院, 西安　710049

2.
咸阳师范学院与中国科学院近代物理研究所联合共建离子束与光物理实验室, 咸阳　712000

通信作者: 柳钰, liuyuxianyang0625@126.com

基金项目: 省部级-陕西省科技厅自然科学基础研究计划(2019JQ-493)

Authors and contacts

1.
School of Science, Xi’an Jiaotong University, Xi’an 710049, China

2.
Ion Beam and Optical Physical Laboratory, Xianyang Normal University, Xianyang 712000, China

Corresponding author: Liu Yu, liuyuxianyang0625@126.com

文章全文 : translate this paragraph

参考文献

[1]	王兴, 赵永涛, 程锐, 周贤明, 徐戈, 孙渊博, 雷瑜, 王瑜玉, 任洁茹, 虞洋, 李永峰, 张小安, 李耀宗, 梁昌慧, 肖国青 2012 61 193201 Google Scholar Wang X, Zhao Y T, Cheng R, Zhou X M, Xu G, Sun Y B, Lei Y, Wang Y Y, Ren J R, Yu Y, Li Y F, Zhang X A, Li Y Z, Liang C H, Xiao G Q 2012 Acta Phys. Sin. 61 193201 Google Scholar
[2]	Horvat V, Watson R L, Blackadar J M 2008 Phys. Rev. A 77 032724 Google Scholar
[3]	梁昌慧, 张小安, 李耀宗, 赵永涛, 周贤明, 王兴, 梅策香, 肖国青 2018 67 243201 Google Scholar Liang C H, Zhang X A, Li Y Z, Zhao Y T, Zhou X M, Wang X, Mei C X, Xiao G Q 2018 Acta Phys. Sin. 67 243201 Google Scholar
[4]	Han I, Şahin M, Demir L 2009 Appl. Radiat. Isot. 67 1027 Google Scholar
[5]	Salem S, Stöhlker, T, Demian A B, Hagmann S, Kozhuharov C, Liesen D, Gumberidze A 2013 Phys. Rev. A 88 012701 Google Scholar
[6]	Šmit Ž 2005 Nucl. Instrum. Methods B 240 258 Google Scholar
[7]	Romo-Kröger C M 2010 Vacuum 84 1250 Google Scholar
[8]	Ma K, Dong C Z, Xie L Y, Qu Y Z 2014 Chin. Phys.Lett. 31 103201 Google Scholar
[9]	牟致栋, 魏琦瑛 2014 63 083402 Google Scholar Mu Z D, Wei Q Y 2014 Acta Phys. Sin. 63 083402 Google Scholar
[10]	Freemantle C S, Sacks N, Topic M, Pineda-vargas C A 2014 Nucl. Instrum. Methods B 318 168 Google Scholar
[11]	Kada W, Kishi A, Sueyasu M, Sato F, Kato Y, Iida T 2014 Nucl. Instrum. Methods B 318 51 Google Scholar
[12]	Fernandez J E, Scot V, Verardi L, Salvat F 2014 Radiat. Phys. Chem. 95 22 Google Scholar
[13]	Flugge S, Mehlhorn W, Schmidt V 1972 Phys. Rev. Lett. 29 7 Google Scholar
[14]	马堃, 颉录有, 张登红, 董晨钟, 屈一至 2016 65 083201 Google Scholar Ma K, Xie L Y, Zhang D H, Dong C Z, Qu Y Z 2016 Acta Phys. Sin. 65 083201 Google Scholar
[15]	Wang X, Xu Z F, Cheng L 2016 Radiat. Phys. Chem. 122 24 Google Scholar
[16]	Slivinsky V W, Ebert P J 1969 Phys. Lett. A 29 463 Google Scholar
[17]	Ertugrul M, Sogut O, Simsek O, Buyukkasap E 2001 J. Phys. B: At.Mol. Opt. Phys. 34 909 Google Scholar
[18]	Richard P, Bonner T I, Furuta T, Morgan I L, Rhodes J R 1970 Phys. Rev. A 1 1044 Google Scholar
[19]	Li T K, Watson R L 1974 Phys. Rev. A 9 1574 Google Scholar
[20]	Salem S I, Wimmer R J 1970 Phys. Rev. A 2 1121 Google Scholar
[21]	Yalçın P 2007 Nucl. Instrum. Methods B 254 182 Google Scholar
[22]	Apaydın G, Aylıkcı V, Cengiz E, Kaya N, Kobya Y, Tıraşoğlu E 2008 Radiat. Phys. Chem. 77 923 Google Scholar
[23]	Akkus T, Sahin Y, Yılmaz D, Tuzluca F N 2017 Can. J. Phys. 95 220 Google Scholar
[24]	Ertuğral B, Apaydın G, Cevika U, Ertuğrul M, Kobya A I 2007 Radiat.Phys.Chem. 76 15 Google Scholar
[25]	Scofield J H 1974 Phys. Rev. A 9 1041 Google Scholar
[26]	Scofield J H 1974 At. Data Nucl. Data Tables 14 121 Google Scholar
[27]	Manson S T, Kennedy D J 1974 At. Data Nucl. Data Tables 14 111 Google Scholar
[28]	Berezhko E G, Kabachnik N M 1977 J. Phys. B: At.Mol. Opt. Phys. 10 2467 Google Scholar
[29]	Kanaya K, Okayama S 1972 J. Phys. D: Appl. Phys. 5 43 Google Scholar
[30]	Yadav N, Bhatt P, Singh R, Llovet X, Shanker R 2011 Appl. Radiat. Isot. 69 1380 Google Scholar

施引文献

图 1 实验装置示意图

Fig. 1. Experimental setup.

下载: 全尺寸图片幻灯片

图 2 150°探测角下Fe靶和V靶的能谱分布图　(a) Fe靶; (b) V靶

Fig. 2. Characteristic K X-ray spectrum of Fe and V induced by impact with bremsstrahlung with central energy of 13.1 keV and measured at the emission angle of 150°: (a) Target Fe; (b) target V.

下载: 全尺寸图片幻灯片

图 3 探测角为120°—170°时, Fe靶和V靶特征X射线强度比K_β/K_α的角分布关系

Fig. 3. Angular distribution of characteristic X-ray intensity ratios of K_β/K_α at detection angles of 120°–170° for Fe and V.

下载: 全尺寸图片幻灯片

图 4 K_β/K_α的实验值、Ertuğral^[24]的实验结果和理论计算值^[25–27]对比图

Fig. 4. Comparison of X-ray intensity ratios K_β/K_α in the present work, literature experimental results from Ertuğrul^[24] and theoretical values^[25–27].

下载: 全尺寸图片幻灯片

map

[1]	王兴, 赵永涛, 程锐, 周贤明, 徐戈, 孙渊博, 雷瑜, 王瑜玉, 任洁茹, 虞洋, 李永峰, 张小安, 李耀宗, 梁昌慧, 肖国青 2012 61 193201 Google Scholar Wang X, Zhao Y T, Cheng R, Zhou X M, Xu G, Sun Y B, Lei Y, Wang Y Y, Ren J R, Yu Y, Li Y F, Zhang X A, Li Y Z, Liang C H, Xiao G Q 2012 Acta Phys. Sin. 61 193201 Google Scholar
[2]	Horvat V, Watson R L, Blackadar J M 2008 Phys. Rev. A 77 032724 Google Scholar
[3]	梁昌慧, 张小安, 李耀宗, 赵永涛, 周贤明, 王兴, 梅策香, 肖国青 2018 67 243201 Google Scholar Liang C H, Zhang X A, Li Y Z, Zhao Y T, Zhou X M, Wang X, Mei C X, Xiao G Q 2018 Acta Phys. Sin. 67 243201 Google Scholar
[4]	Han I, Şahin M, Demir L 2009 Appl. Radiat. Isot. 67 1027 Google Scholar
[5]	Salem S, Stöhlker, T, Demian A B, Hagmann S, Kozhuharov C, Liesen D, Gumberidze A 2013 Phys. Rev. A 88 012701 Google Scholar
[6]	Šmit Ž 2005 Nucl. Instrum. Methods B 240 258 Google Scholar
[7]	Romo-Kröger C M 2010 Vacuum 84 1250 Google Scholar
[8]	Ma K, Dong C Z, Xie L Y, Qu Y Z 2014 Chin. Phys.Lett. 31 103201 Google Scholar
[9]	牟致栋, 魏琦瑛 2014 63 083402 Google Scholar Mu Z D, Wei Q Y 2014 Acta Phys. Sin. 63 083402 Google Scholar
[10]	Freemantle C S, Sacks N, Topic M, Pineda-vargas C A 2014 Nucl. Instrum. Methods B 318 168 Google Scholar
[11]	Kada W, Kishi A, Sueyasu M, Sato F, Kato Y, Iida T 2014 Nucl. Instrum. Methods B 318 51 Google Scholar
[12]	Fernandez J E, Scot V, Verardi L, Salvat F 2014 Radiat. Phys. Chem. 95 22 Google Scholar
[13]	Flugge S, Mehlhorn W, Schmidt V 1972 Phys. Rev. Lett. 29 7 Google Scholar
[14]	马堃, 颉录有, 张登红, 董晨钟, 屈一至 2016 65 083201 Google Scholar Ma K, Xie L Y, Zhang D H, Dong C Z, Qu Y Z 2016 Acta Phys. Sin. 65 083201 Google Scholar
[15]	Wang X, Xu Z F, Cheng L 2016 Radiat. Phys. Chem. 122 24 Google Scholar
[16]	Slivinsky V W, Ebert P J 1969 Phys. Lett. A 29 463 Google Scholar
[17]	Ertugrul M, Sogut O, Simsek O, Buyukkasap E 2001 J. Phys. B: At.Mol. Opt. Phys. 34 909 Google Scholar
[18]	Richard P, Bonner T I, Furuta T, Morgan I L, Rhodes J R 1970 Phys. Rev. A 1 1044 Google Scholar
[19]	Li T K, Watson R L 1974 Phys. Rev. A 9 1574 Google Scholar
[20]	Salem S I, Wimmer R J 1970 Phys. Rev. A 2 1121 Google Scholar
[21]	Yalçın P 2007 Nucl. Instrum. Methods B 254 182 Google Scholar
[22]	Apaydın G, Aylıkcı V, Cengiz E, Kaya N, Kobya Y, Tıraşoğlu E 2008 Radiat. Phys. Chem. 77 923 Google Scholar
[23]	Akkus T, Sahin Y, Yılmaz D, Tuzluca F N 2017 Can. J. Phys. 95 220 Google Scholar
[24]	Ertuğral B, Apaydın G, Cevika U, Ertuğrul M, Kobya A I 2007 Radiat.Phys.Chem. 76 15 Google Scholar
[25]	Scofield J H 1974 Phys. Rev. A 9 1041 Google Scholar
[26]	Scofield J H 1974 At. Data Nucl. Data Tables 14 121 Google Scholar
[27]	Manson S T, Kennedy D J 1974 At. Data Nucl. Data Tables 14 111 Google Scholar
[28]	Berezhko E G, Kabachnik N M 1977 J. Phys. B: At.Mol. Opt. Phys. 10 2467 Google Scholar
[29]	Kanaya K, Okayama S 1972 J. Phys. D: Appl. Phys. 5 43 Google Scholar
[30]	Yadav N, Bhatt P, Singh R, Llovet X, Shanker R 2011 Appl. Radiat. Isot. 69 1380 Google Scholar

[1]	戈迪, 赵国鹏, 祁月盈, 陈晨, 高俊文, 侯红生. 等离子体环境中相对论效应对类氢离子光电离过程的影响. , 2024, 73(8): 083201. doi: 10.7498/aps.73.20240016
[2]	赵婷, 宫毛毛, 张松斌. 氦原子贝塞尔涡旋光电离的理论研究. , 2024, 73(24): 244201. doi: 10.7498/aps.73.20241378
[3]	柳钰, 徐忠锋, 王兴, 胡鹏飞, 张小安. 光子碰撞Au靶产生L系特征X射线角分布. , 2020, 69(12): 123201. doi: 10.7498/aps.69.20191977
[4]	涂婧怡, 陈赦, 汪沨. 光电离速率影响大气压空气正流注分支的机理研究. , 2019, 68(9): 095202. doi: 10.7498/aps.68.20190060
[5]	李琼, 沈礼, 闫俊刚, 戴长建, 杨玉娜. Eu原子4f76p1/2ns自电离过程的动力学特性. , 2016, 65(15): 153202. doi: 10.7498/aps.65.153202
[6]	陈传文, 项阳. 正交各向异性双层交换弹簧薄膜的磁矩分布. , 2016, 65(12): 127502. doi: 10.7498/aps.65.127502
[7]	马堃, 颉录有, 张登红, 董晨钟, 屈一至. 氖原子光电子角分布的理论计算. , 2016, 65(8): 083201. doi: 10.7498/aps.65.083201
[8]	戚晓秋, 汪峰, 戴长建. 碱金属原子的光激发与光电离. , 2015, 64(13): 133201. doi: 10.7498/aps.64.133201
[9]	单晓斌, 赵玉杰, 孔蕊弘, 王思胜, 盛六四, 黄明强, 王振亚. ArCO团簇光电离的实验和理论研究. , 2013, 62(5): 053602. doi: 10.7498/aps.62.053602
[10]	刘亚红, 刘辉, 赵晓鹏. 基于小型化结构的各向同性负磁导率材料与左手材料. , 2012, 61(8): 084103. doi: 10.7498/aps.61.084103
[11]	张斌, 潘雪丰, 陶卫东. 新型内反射旋光光学滤波器研究. , 2011, 60(5): 054214. doi: 10.7498/aps.60.054214
[12]	孙长平, 王国利, 周效信. F3+和Ne4+离子的光电离截面的理论计算. , 2011, 60(5): 053202. doi: 10.7498/aps.60.053202
[13]	龚伯仪, 周欣, 赵晓鹏. 光频三维各向同性左手超材料结构单元模型的仿真设计. , 2011, 60(4): 044101. doi: 10.7498/aps.60.044101
[14]	王向丽, 董晨钟, 桑萃萃. Ne原子的1s光电离及其Auger衰变过程的理论研究. , 2009, 58(8): 5297-5303. doi: 10.7498/aps.58.5297
[15]	刘凌涛, 王民盛, 韩小英, 李家明. 溴的光电离和辐射复合——平均原子模型速率系数与细致组态速率系数. , 2006, 55(5): 2322-2327. doi: 10.7498/aps.55.2322
[16]	黄超群, 卫立夏, 杨斌, 杨锐, 王思胜, 单晓斌, 齐飞, 张允武, 盛六四, 郝立庆, 周士康, 王振亚. HFC-152a的同步辐射真空紫外光电离和光解离研究. , 2006, 55(3): 1083-1088. doi: 10.7498/aps.55.1083
[17]	王思胜, 孔蕊弘, 田振玉, 单晓斌, 张允武, 盛六四, 王振亚, 郝立庆, 周士康. Ar?NO团簇的同步辐射光电离研究. , 2006, 55(7): 3433-3437. doi: 10.7498/aps.55.3433
[18]	郑志远, 李玉同, 远晓辉, 徐妙华, 梁文锡, 于全芝, 张翼, 王兆华, 魏志义, 张杰. 超热电子角分布和能谱的实验研究. , 2006, 55(10): 5349-5353. doi: 10.7498/aps.55.5349
[19]	方泉玉, 李萍, 刘勇, 邹宇, 邱玉波. Alq+(q=0—12)的光电离截面和Bethe系数. , 2001, 50(4): 655-659. doi: 10.7498/aps.50.655
[20]	张穗萌, 吴兴举. H原子(e,2e)反应中电子角分布的理论研究. , 2001, 50(11): 2137-2143. doi: 10.7498/aps.50.2137

计量

文章访问数: 8039
PDF下载量: 86
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

光电离过程中Fe靶和V靶特征辐射的角相关研究