-
针对高功率激光装置中靶面辐照均匀性的高要求, 提出了一种利用束间动态干涉改善辐照均匀性的快速匀滑方法. 基本原理是利用共轭相位板阵列对存在一定波长差的多束激光附加相位调制, 从而使各子束在远场两两相干叠加以产生动态的干涉图样, 进而引起焦斑内部散斑的动态扫动, 在ps时间内抹平不均匀性. 以典型惯性约束聚变装置中的激光集束为例, 通过建立基于束间动态干涉的快速匀滑物理模型, 定量分析了相位板类型、相位调制幅度和束间波长差等因素对焦斑动态干涉图样的影响及规律, 进而对其束匀滑特性进行了讨论. 结果表明, 基于束间动态干涉的快速匀滑方法可以有效地实现多方向、多维度的焦斑内部散斑快速扫动, 且通过与传统束匀滑技术的联用, 可以在更短的时间内达到更好的焦斑均匀性.Aiming at the high requirements for illumination uniformity on the target in laser-driven inertial confinement fusion (ICF) facilities, an ultrafast smoothing method based on dynamic interference structure between beamlets of a laser quad is proposed. The basic principle of this scheme is to use a conjugate phase plate array to add the conjugate phase modulation to the multiple beamlets of a laser quad with a certain wavelength difference. Consequently, every two beamlets are coherently superposed in the far field to generate a dynamic interference pattern, resulting in the fast redistribution of the speckles introduced by continuous phase plate inside the focal spot and further improving the illumination uniformity on the target on a picosecond timescale. The coherent beamlets with a certain wavelength difference can be generated by using a broadband seed laser. Taking the laser quad of the typical ICF facilities for example, the physical model of the ultrafast smoothing method based on dynamic interference structure of beamlets is built up. The influences of the phase-plate type, the peak-to-valley value of the phase modulation and the wavelength difference between the beamlets are analyzed quantitatively, and the smoothing characteristics of the focal spot are discussed in detail and compared with those from the traditional temporal smoothing scheme such as smoothing by spectral dispersion. The results indicate that the directions of the moving speckles in the focal spot are determined by the phase-plate type. However, the required time to achieve stable illumination uniformity, i.e, the decay time, is determined by the wavelength difference between the beamlets. Moreover, the illumination uniformity on the target becomes better with the increase of peak-to-valley value of the phase modulation at first and then remains almost the same. Thus, the ultrafast smoothing method based on dynamic interference structures with well-designed phase arrays and wavelength combinations of the beamlets can realize the multi-directional and multi-dimensional speckle sweeping inside the focal spot, and further improving the irradiation uniformity on the target within several picoseconds or sub-picoseconds. Combining with the traditional beam smoothing scheme, better illumination uniformity can be achieved on an ultrashort timescale. This novel scheme can be used as an effective supplement to the existing temporal beam smoothing techniques.
-
Keywords:
- inertial confinement fusion /
- dynamic interference /
- beam smoothing /
- conjugate phase plate
[1] Lindl J D, Amendt P, Berger R L, Glendinning S G, Glenzer S H, Haan S W, Kauffman R L, Landen O L, Suter L J 2004 Phys. Plasmas 11 339
Google Scholar
[2] Kato Y, Mima K, Miyanaga N, Arinaga S, Kitagawa Y, Nakatsuka M, Yamanaka C 1984 Phys. Rev. Lett. 53 1057
Google Scholar
[3] Dixit S N, Thomas I M, Woods B W, Morgan A J, Henesian M A, Wegner P J, Powell H T 1993 Appl. Opt. 32 2543
Google Scholar
[4] Néauport J, Ribeyre X, Daurios J, Valla D, Lavergne M, Beau V, Videau L 2003 Appl. Opt. 42 2377
Google Scholar
[5] 高妍琦, 赵晓晖, 贾果, 李福建, 崔勇, 饶大幸, 季来林, 刘栋, 冯伟, 黄秀光, 马伟新, 隋展 2019 68 075201
Google Scholar
Gao Y Q, Zhao X H, Jia G, Li F J, Cui Y, Rao D X, Ji L L, Liu D, Feng W, Huang X G, Ma W X, Sui Z 2019 Acta Phys. Sin. 68 075201
Google Scholar
[6] 李福建, 高妍琦, 赵晓晖, 季来林, 王伟, 黄秀光, 马伟新, 隋展, 裴文兵 2018 67 175201
Google Scholar
Li F J, Gao Y Q, Zhao X H, Ji L L, Wang W, Huang X G, Ma W X, Sui Z, Pei W B 2018 Acta Phys. Sin. 67 175201
Google Scholar
[7] Rothenberg J E 1997 J. Opt. Soc. Am. B 14 1664
Google Scholar
[8] 龚涛 2015 博士学位论文 (合肥: 中国科学技术大学)
Gong T 2015 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[9] Montgomery D S, Moody J D, Baldis H A, Afeyan B B, Berger R L, Estabrook K G, Lasinski B F, Williams E A 1996 Phys. Plasmas 3 1728
Google Scholar
[10] Berger R L, Lasinski B F, Langdon A B, Kaiser T B, Afeyan B B, Cohen B I, Still C H, Williams E A 1996 Phys. Rev. Lett. 76 3239
Google Scholar
[11] Jiang Y E, Li X C, Zhou S L, Fan W, Lin Z Q 2013 Chin. Opt. Lett. 11 052301
Google Scholar
[12] Regan S P, Marozas J A, Kelly J H, Boehly T R, Donaldson W R, Jaanimagi P A, Keck R L, Kessler T J, Meyerhofer D D, Seka W, Skupsky S, Smalyuk V A 2000 J. Opt. Soc. Am. B 17 1483
Google Scholar
[13] Regan S P, Marozas J A, Craxton R S, Kelly J H, Donaldson W R, Jaanimagi P A, Jacobs-Perkins D, Keck R L, Kessler T J, Meyerhofer D D, Sangster T C, Seka W, Smalyuk V A, Skupsky S, Zuegel J D 2005 J. Opt. Soc. Am. B 22 998
[14] Rothenberg J E 1995 Office of Scientific & Technical Information Technical Reports, Monterey, CA, May 30−June 2 1995 p634
[15] 钟哲强, 侯鹏程, 张彬 2016 65 094207
Google Scholar
Zhong Z Q, Hou P C, Zhang B 2016 Acta Phys. Sin. 65 094207
Google Scholar
[16] Zhong Z Q, Yi M Y, Sui Z, Zhang X, Zhang B, Yuan X 2018 Opt. Lett. 43 3285
Google Scholar
[17] Henesian M A, Haney S W, Thomas M, Trenholme J B 1997 Solid State Lasers for Application to Inertial Confinement Fusion: Second Annual International Conference Paris, France, October 22−25, 1996 p783
[18] Haynam C A, Wegner P J, Auerbach J M, Bowers M W, et al. 2017 Appl. Opt. 46 3276
[19] Skupsky S, Short R W, Kessler T, Craxton R S, Letzring S, Soures J M 1989 J. Appl. Phys. 66 3456
Google Scholar
[20] Spaeth M L, Manes K R, Bowers M, Celliers P, Nicola J M D, Nicola P D, Dixit S, Erbert G, Heebner J, Kalantar D, Landen O, MacGowan B, Wonterghem B V, Wegner P, Widmayer C, Yang S 2016 Fusion Sci. Technol. 69 366
Google Scholar
[21] Zheng W G, Wei X F, Zhu Q H, Jing F, et al. 2018 Matter and Radiation at Extremes 2 243
[22] Cui Y, Gao Y Q, Rao D X, Liu D, Li F J, Ji L L, Shi H T, Liu J N, Feng W, Xia L, Liu J, Li X L, Wang T, Ma W X, Sui Z 2019 Opt. Lett. 44 2859
Google Scholar
[23] 苟斗斗, 杨四刚, 尹飞飞, 张磊, 邢芳俭, 陈宏伟, 陈明华, 谢世钟 2013 光学学报 33 78
Gou D D, Yang S G, Yin F F, Zhang L, Xing F J, Chen H W, Chen M H, Xie S Z 2013 Acta Opt. Sin. 33 78
[24] Spaeth M L, Manes K R, Kalantar D H, Miller P E, et al. 2016 Fusion Sci. Technol. 69 25
Google Scholar
[25] 张锐 2013 博士学位论文 (合肥: 中国科学技术大学)
Zhang R 2013 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
-
图 1 基于束间动态干涉的快速匀滑方案示意图
Fig. 1. Schematic illustration of smoothing by dynamic interference structures of beamlets.
图 2 不同相位调制所得焦斑光通量对比度随时间的变化
Fig. 2. The contrast of focal spots with different phase modulation.
图 3 不同类型共轭相位调制的动态干涉图样 (a)倾斜相位; (b)柱面相位; (c)椭球面相位; (d)涡旋相位
Fig. 3. Dynamic interference structures with different kinds of phase modulation: (a) Tilted phase; (b) cylinder phase; (c) ellipsoid phase; (d) spiral phase.
图 4 不同类型相位板阵列的焦斑 (a)光通量对比度曲线和(b) FOPAI曲线
Fig. 4. (a) Variation of contrast with integral time and (b) FOAPI curves with different phase plate array.
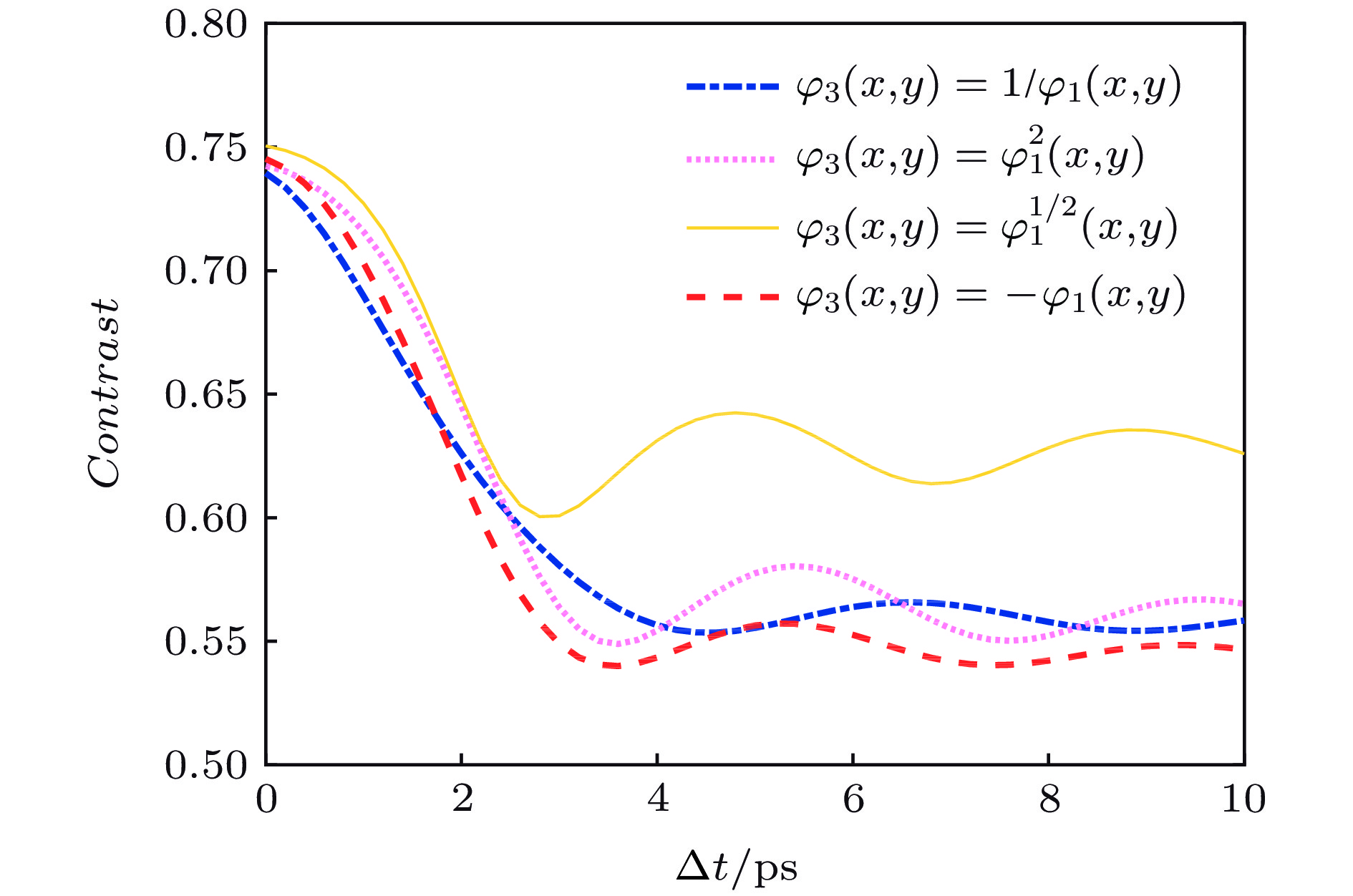
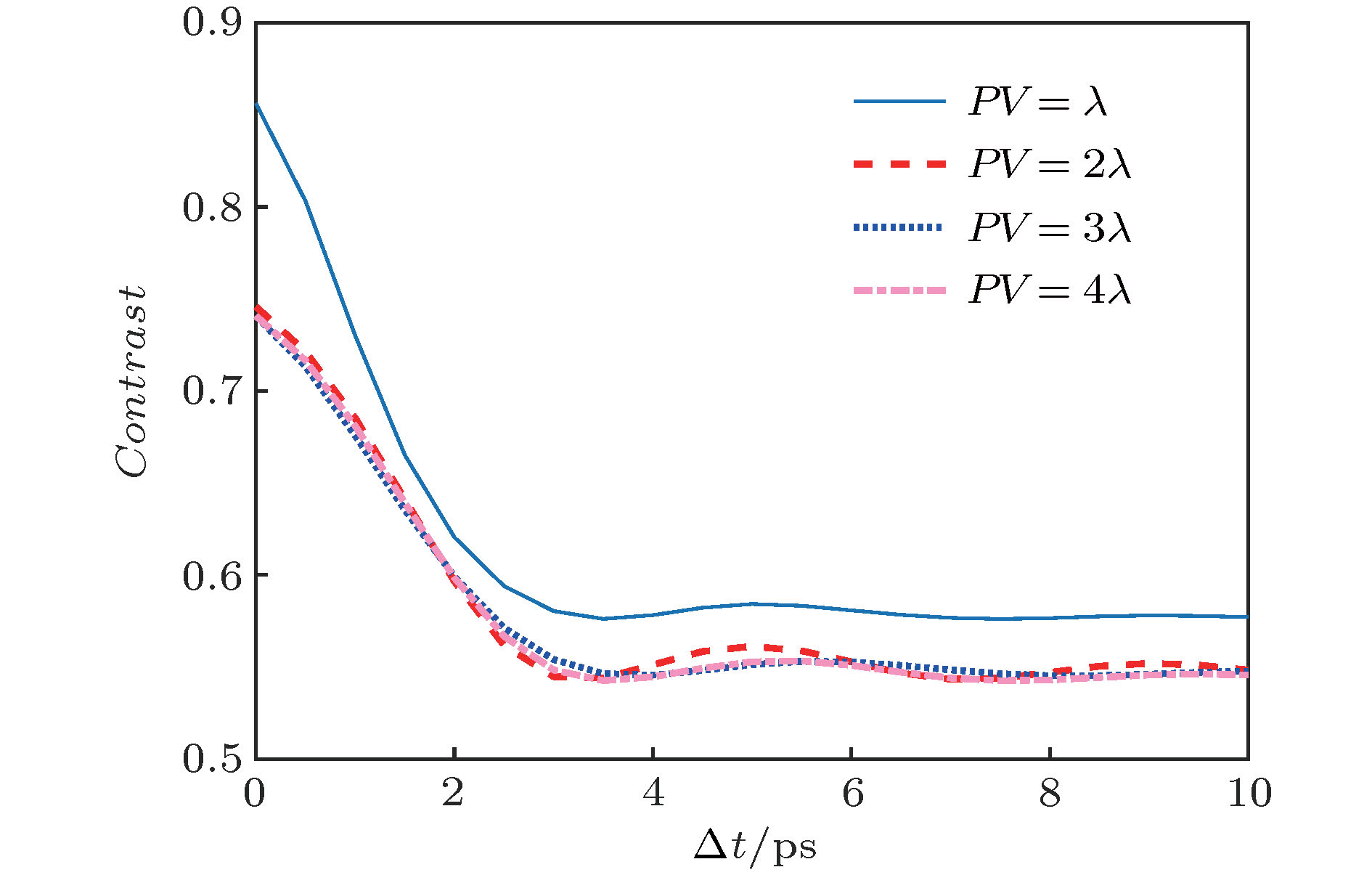
图 6 不同波长情况下, 差焦斑光通量对比度随时间的变化 (a) 600 μm × 500 μm焦斑80%环围能量; (b) 600 μm × 500 μm焦斑30%环围能量; (c) 350 μm × 350 μm焦斑80%环围能量
Fig. 6. Variation of contrast with integral time of different Δλ: (a) 80% energy of the 600 μm × 500 μm focal spot; (b) 30% energy of the 600 μm × 500 μm focal spot; (c) 80% energy of the 350 μm × 350 μm focal spot.
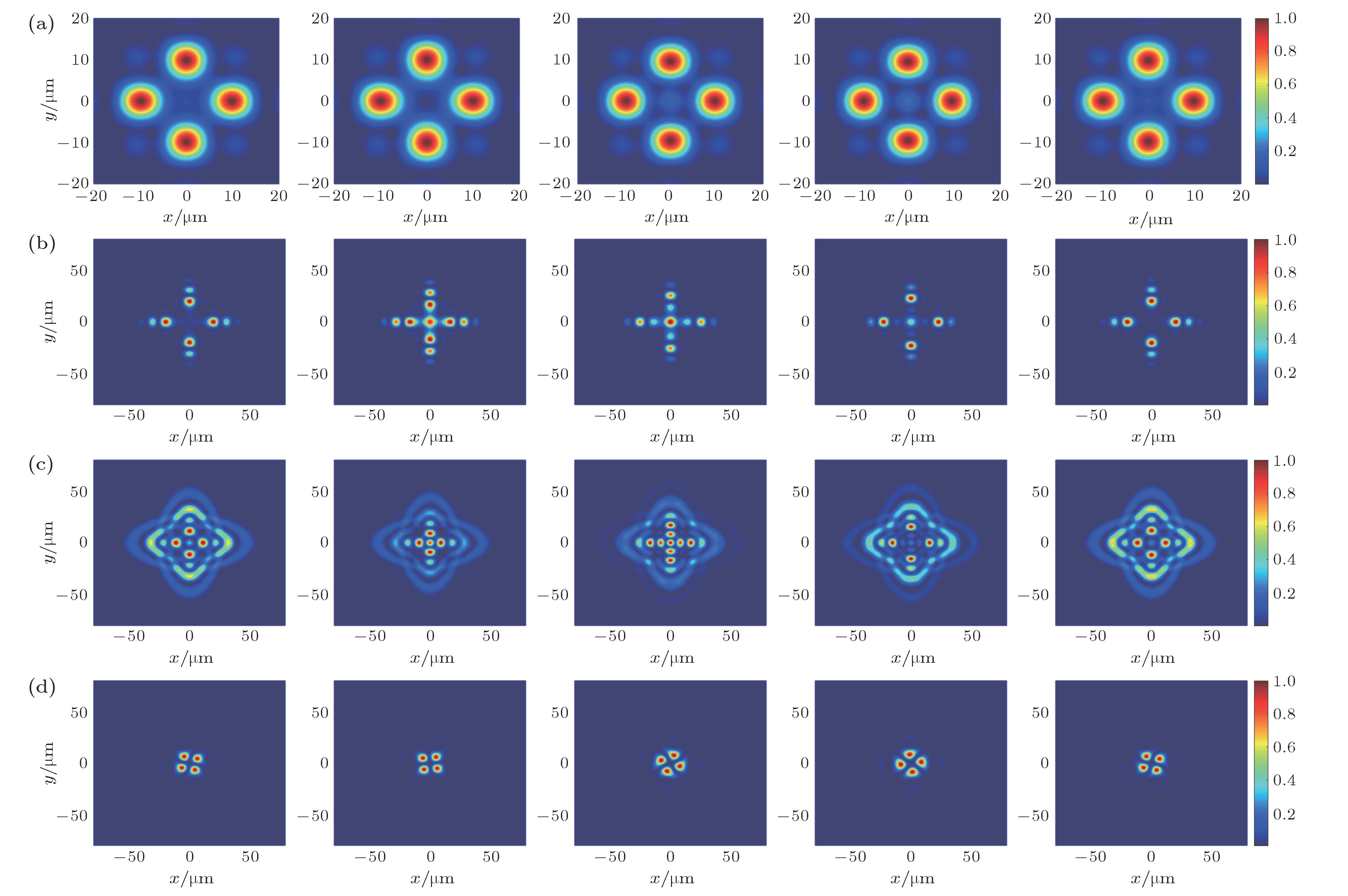
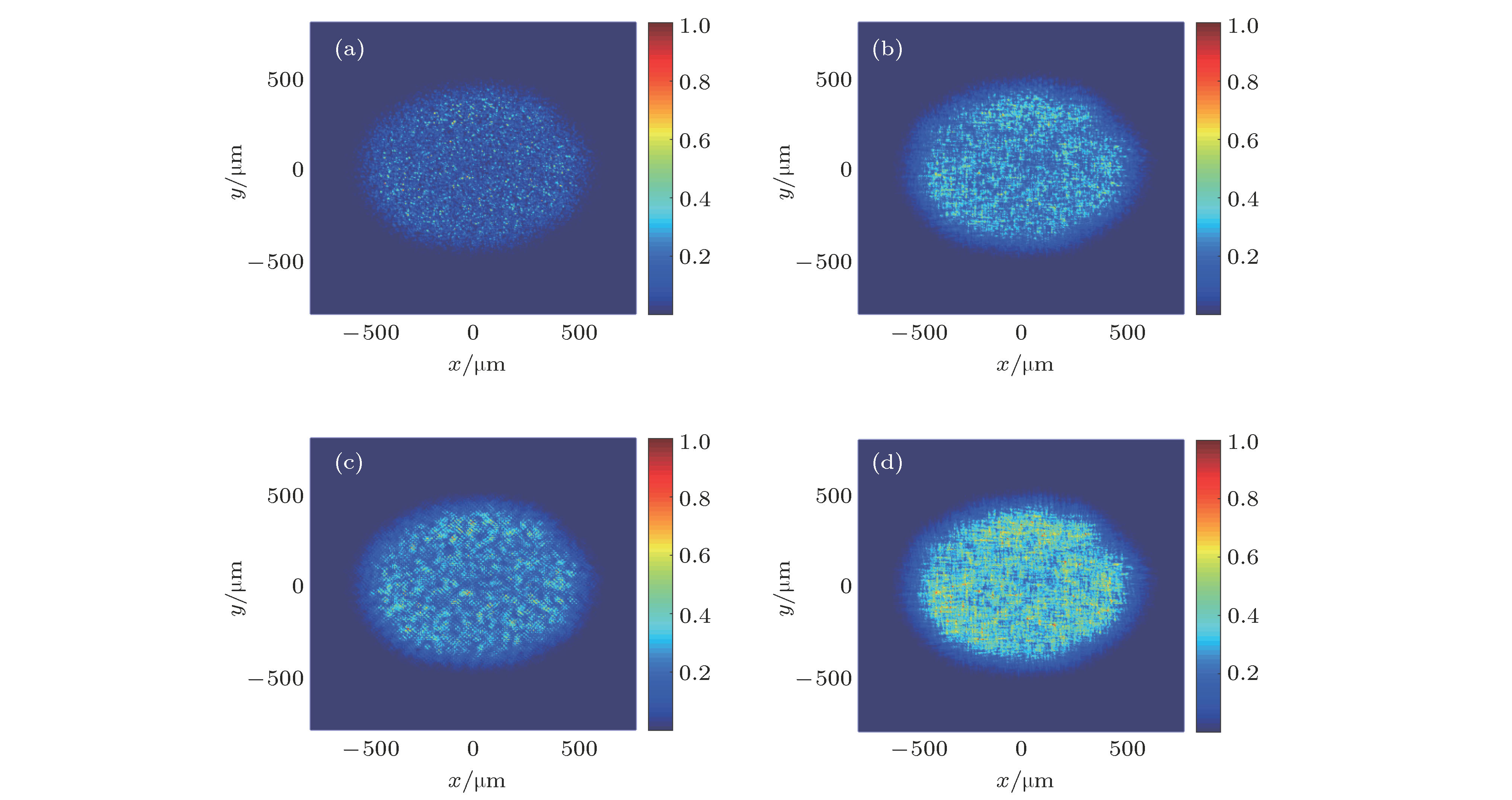
图 7 不同匀滑方案的焦斑光强分布 (a)仅CPP; (b) 2D-SSD + CPP; (c)动态干涉图样匀滑方法 + CPP; (d) 2D-SSD + 动态干涉图样匀滑方法 + CPP
Fig. 7. Focused intensity distributions with different smoothing scheme: (a) CPP only; (b) 2D-SSD + CPP; (c) smoothing by dynamic interference structures of beamlets + CPP; (d) smoothing by dynamic interference structures of beamlets+2D-SSD + CPP.
图 8 不同匀滑方案下, (a)光通量对比度随时间的变化和(b) FOPAI曲线
Fig. 8. (a) Variation of contrast with integral time and (b) FOPAI curves with different smoothing scheme.
表 1 不同相位分布的二维傅里叶变换表达式
Table 1. 2D-Fourier transform of different phase distribution.
$ {\varphi }_{1}(x_{f}, y_{f}) $ $ B_{1}(x_{f}, y_{f}) $ $ b_{1}(x_{f}, y_{f}) $ x $ {\delta }[x_{f}/({\lambda f})+1/(2\text{π} )]{\delta }[y_{f}/({\lambda f})] $ 0 –x2 $ \text{π} ^{1/2}\delta [y_{f}/(\lambda f) ]$ $ \text{π} ^{2}x_{f}^{2}/({\lambda }^{2}f^{2})-3\text{π} /4 $ $ -[h_{1}{ }(x/w)^{2}+ h_{2}(y/w)^{2}] $ $ w^{2}\text{π} (h_{1}h_{2})^{-1/2} $ $ \text{π} ^{2}x_{f}^{2}/(h_{1}{\lambda }^{2}f^{2}w^{2})+\text{π} ^{2}y_{f}^{2}/(h_{2}{\lambda }^{2}f^{2}w^{2})-3\text{π} /2 $ $ \arctan(y/x) $ $ [-{\lambda f}/(2\text{π} )]\{ {\delta }[x_{f}/({\lambda f})]{\rm d}{\delta }[y_{f}/({\lambda f})]/{\rm d}y_{f }- {\rm i}{\delta }[y_{f}/({\lambda f})]{\rm d}\delta [x_{f}/({\lambda f})]/{\rm d}x_{f}\} $ 
下载: 导出CSV
-
[1] Lindl J D, Amendt P, Berger R L, Glendinning S G, Glenzer S H, Haan S W, Kauffman R L, Landen O L, Suter L J 2004 Phys. Plasmas 11 339
Google Scholar
[2] Kato Y, Mima K, Miyanaga N, Arinaga S, Kitagawa Y, Nakatsuka M, Yamanaka C 1984 Phys. Rev. Lett. 53 1057
Google Scholar
[3] Dixit S N, Thomas I M, Woods B W, Morgan A J, Henesian M A, Wegner P J, Powell H T 1993 Appl. Opt. 32 2543
Google Scholar
[4] Néauport J, Ribeyre X, Daurios J, Valla D, Lavergne M, Beau V, Videau L 2003 Appl. Opt. 42 2377
Google Scholar
[5] 高妍琦, 赵晓晖, 贾果, 李福建, 崔勇, 饶大幸, 季来林, 刘栋, 冯伟, 黄秀光, 马伟新, 隋展 2019 68 075201
Google Scholar
Gao Y Q, Zhao X H, Jia G, Li F J, Cui Y, Rao D X, Ji L L, Liu D, Feng W, Huang X G, Ma W X, Sui Z 2019 Acta Phys. Sin. 68 075201
Google Scholar
[6] 李福建, 高妍琦, 赵晓晖, 季来林, 王伟, 黄秀光, 马伟新, 隋展, 裴文兵 2018 67 175201
Google Scholar
Li F J, Gao Y Q, Zhao X H, Ji L L, Wang W, Huang X G, Ma W X, Sui Z, Pei W B 2018 Acta Phys. Sin. 67 175201
Google Scholar
[7] Rothenberg J E 1997 J. Opt. Soc. Am. B 14 1664
Google Scholar
[8] 龚涛 2015 博士学位论文 (合肥: 中国科学技术大学)
Gong T 2015 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[9] Montgomery D S, Moody J D, Baldis H A, Afeyan B B, Berger R L, Estabrook K G, Lasinski B F, Williams E A 1996 Phys. Plasmas 3 1728
Google Scholar
[10] Berger R L, Lasinski B F, Langdon A B, Kaiser T B, Afeyan B B, Cohen B I, Still C H, Williams E A 1996 Phys. Rev. Lett. 76 3239
Google Scholar
[11] Jiang Y E, Li X C, Zhou S L, Fan W, Lin Z Q 2013 Chin. Opt. Lett. 11 052301
Google Scholar
[12] Regan S P, Marozas J A, Kelly J H, Boehly T R, Donaldson W R, Jaanimagi P A, Keck R L, Kessler T J, Meyerhofer D D, Seka W, Skupsky S, Smalyuk V A 2000 J. Opt. Soc. Am. B 17 1483
Google Scholar
[13] Regan S P, Marozas J A, Craxton R S, Kelly J H, Donaldson W R, Jaanimagi P A, Jacobs-Perkins D, Keck R L, Kessler T J, Meyerhofer D D, Sangster T C, Seka W, Smalyuk V A, Skupsky S, Zuegel J D 2005 J. Opt. Soc. Am. B 22 998
[14] Rothenberg J E 1995 Office of Scientific & Technical Information Technical Reports, Monterey, CA, May 30−June 2 1995 p634
[15] 钟哲强, 侯鹏程, 张彬 2016 65 094207
Google Scholar
Zhong Z Q, Hou P C, Zhang B 2016 Acta Phys. Sin. 65 094207
Google Scholar
[16] Zhong Z Q, Yi M Y, Sui Z, Zhang X, Zhang B, Yuan X 2018 Opt. Lett. 43 3285
Google Scholar
[17] Henesian M A, Haney S W, Thomas M, Trenholme J B 1997 Solid State Lasers for Application to Inertial Confinement Fusion: Second Annual International Conference Paris, France, October 22−25, 1996 p783
[18] Haynam C A, Wegner P J, Auerbach J M, Bowers M W, et al. 2017 Appl. Opt. 46 3276
[19] Skupsky S, Short R W, Kessler T, Craxton R S, Letzring S, Soures J M 1989 J. Appl. Phys. 66 3456
Google Scholar
[20] Spaeth M L, Manes K R, Bowers M, Celliers P, Nicola J M D, Nicola P D, Dixit S, Erbert G, Heebner J, Kalantar D, Landen O, MacGowan B, Wonterghem B V, Wegner P, Widmayer C, Yang S 2016 Fusion Sci. Technol. 69 366
Google Scholar
[21] Zheng W G, Wei X F, Zhu Q H, Jing F, et al. 2018 Matter and Radiation at Extremes 2 243
[22] Cui Y, Gao Y Q, Rao D X, Liu D, Li F J, Ji L L, Shi H T, Liu J N, Feng W, Xia L, Liu J, Li X L, Wang T, Ma W X, Sui Z 2019 Opt. Lett. 44 2859
Google Scholar
[23] 苟斗斗, 杨四刚, 尹飞飞, 张磊, 邢芳俭, 陈宏伟, 陈明华, 谢世钟 2013 光学学报 33 78
Gou D D, Yang S G, Yin F F, Zhang L, Xing F J, Chen H W, Chen M H, Xie S Z 2013 Acta Opt. Sin. 33 78
[24] Spaeth M L, Manes K R, Kalantar D H, Miller P E, et al. 2016 Fusion Sci. Technol. 69 25
Google Scholar
[25] 张锐 2013 博士学位论文 (合肥: 中国科学技术大学)
Zhang R 2013 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
下载:
计量
- 文章访问数: 6615
- PDF下载量: 62
- 被引次数: 0
