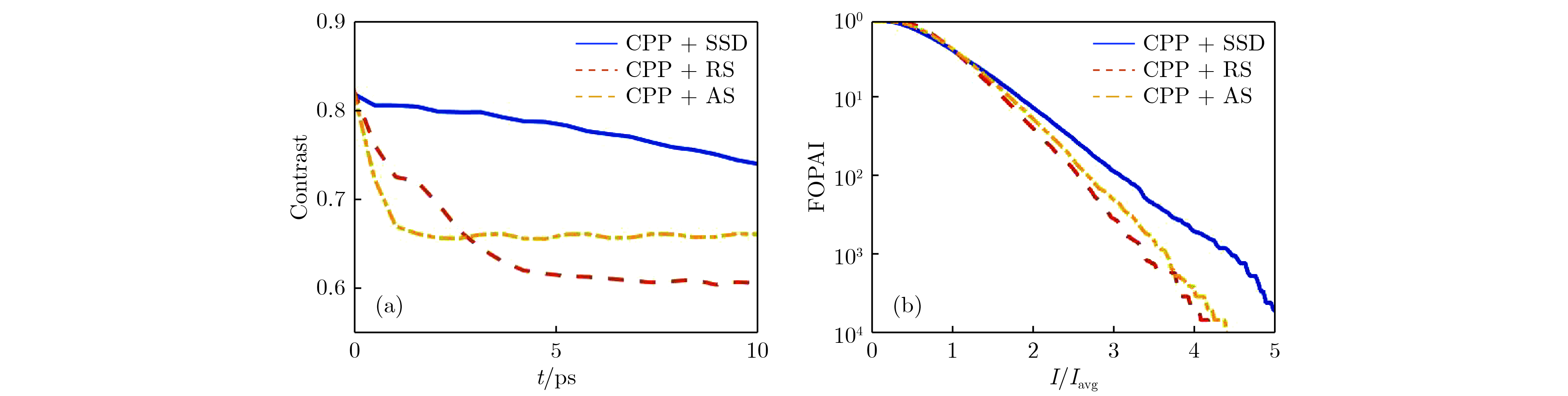
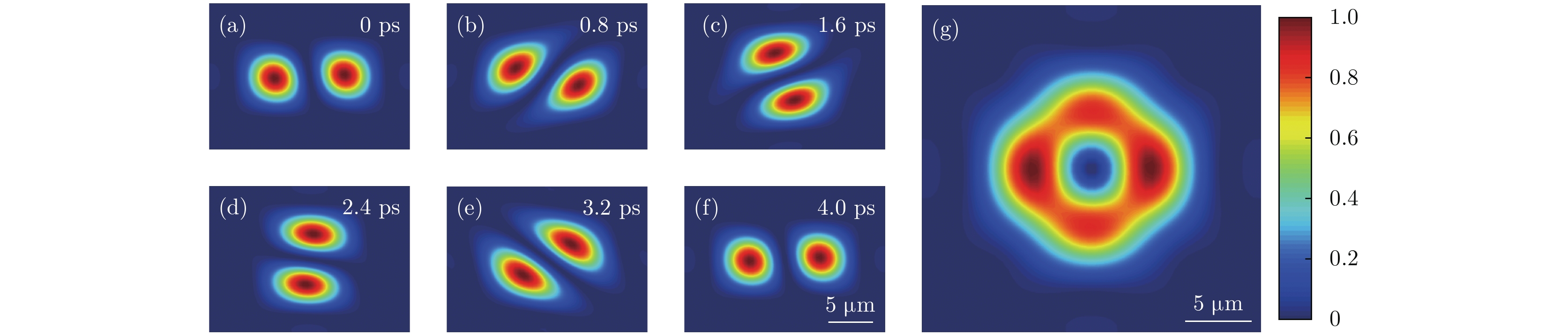
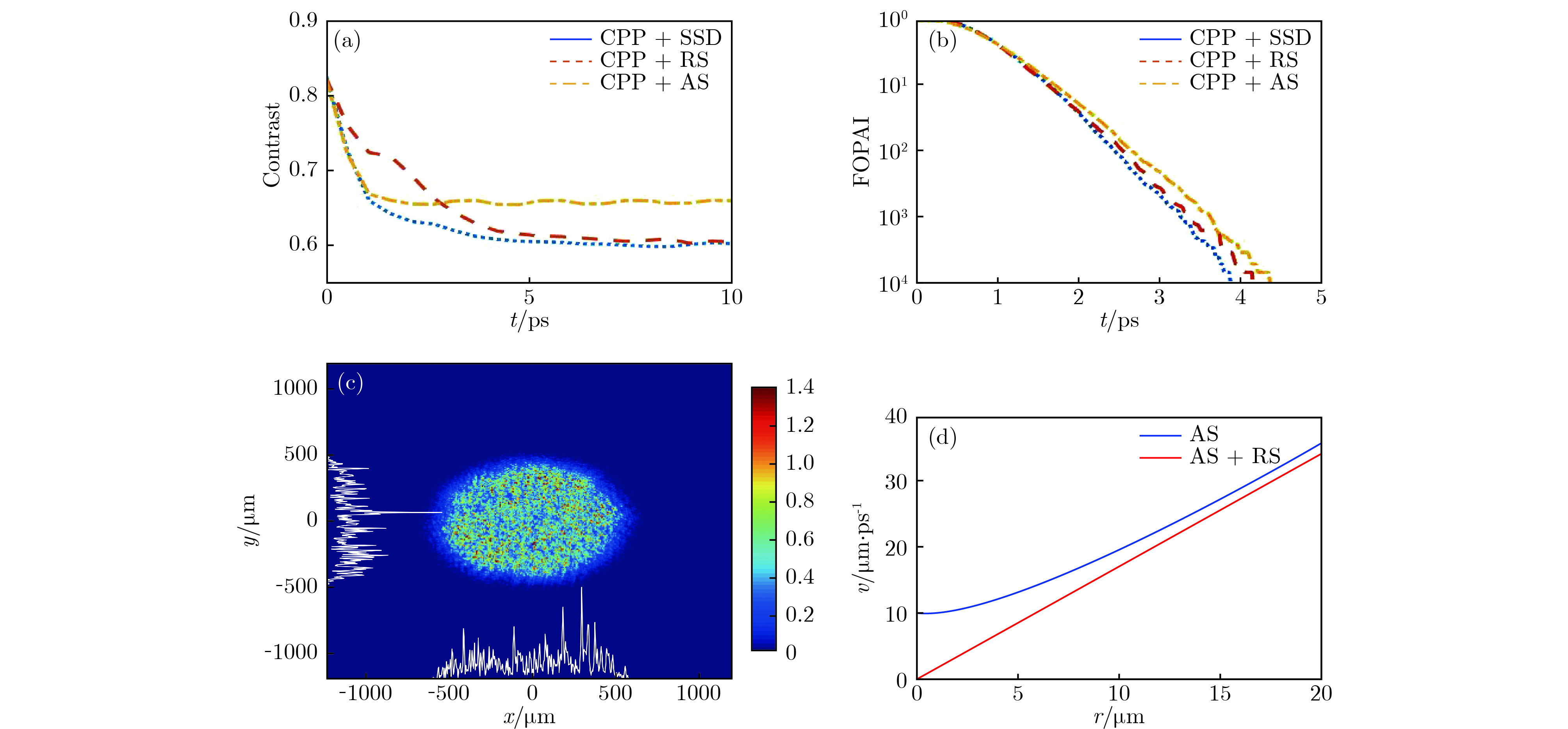
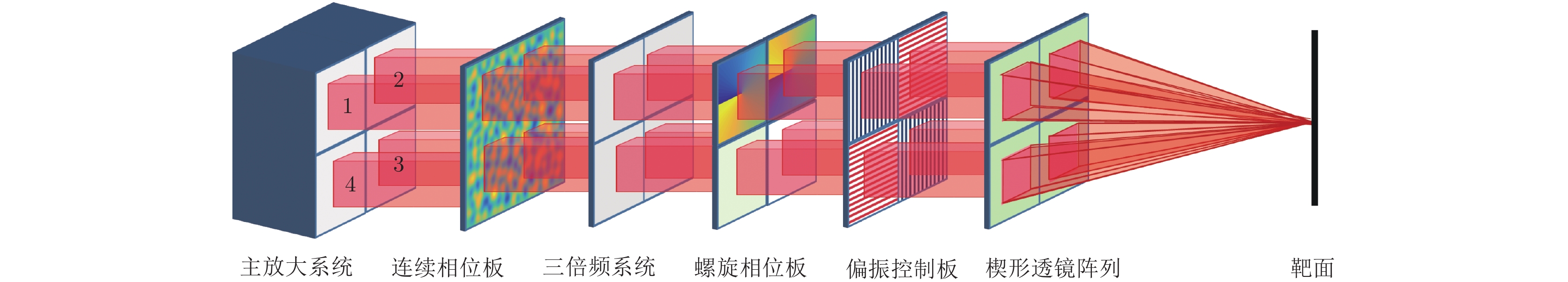
-
针对惯性约束聚变装置对激光集束辐照均匀性的需求, 提出了一种基于涡旋光束的超快速角向匀滑方案, 即利用螺旋相位板使2×2集束中的两子束由超高斯光束变换为涡旋光束, 而其余两子束不变, 进而通过对子束偏振态和中心波长的调控, 使集束中的涡旋光束和超高斯光束在靶面两两相干叠加. 相干叠加后的焦斑以皮秒量级为周期超快速旋转, 从而在极短时间内快速抹平焦斑强度调制, 改善靶面辐照均匀性. 通过建立基于螺旋相位板的激光超快速角向集束匀滑方案的物理模型, 分析了其角向匀滑特性, 并与光谱角色散技术和径向匀滑技术进行了比较分析. 结果表明, 这一新型激光集束匀滑方案能实现对焦斑的超快速角向匀滑, 且能在数皮秒时间内达到最佳辐照均匀性.The illumination uniformity of laser beams in inertial confinement fusion (ICF) facility is a key factor, which plays a crucial role in suppressing the laser plasma instabilities. However, the prevailing beam smoothing techniques cannot meet all the requirements for improving the irradiance uniformity of laser beams and mitigating the laser plasma instabilities, which are determined by the high-frequency spatial modulations and the fine-scale speckles of the focal spots. An ultrafast azimuthal beam smoothing scheme based on vortex beams is proposed in this paper. In this scheme, two of the four beams in a laser quad are transformed from super-Gaussian (SG) beams into vortex beams by inserting two spiral phase plates with opposite topological charges into the beam path, whereas the other two SG beams remain unchanged. By controlling the polarization and the center wavelength of each beam, the SG beam and the transformed vortex beam in the quad are coherently superposed on the target plane, so are the remaining two beams. Owing to the difference in central wavelength and the existence of the topological charges, two focal spots rotating in a period of a few picoseconds are generated in the target plane, which can redistribute the speckles quickly in temporal domain and thus improve the irradiance uniformity of the laser quad. By establishing the physical model of the azimuthal smoothing scheme, the smoothing characteristics including the rotation period, the illumination uniformity and the fractional-power-above-intensity of the focal spots are analyzed in detail. In order to improve the smoothing characteristics significantly, the novel smoothing scheme is further combined with another ultrafast smoothing scheme, i.e. radial smoothing scheme. The influence of the key parameters of the combined smoothing scheme on the illumination uniformity and on the smoothing velocity are discussed. Results indicate that the azimuthal smoothing scheme can achieve the ultrafast smooth of the laser quad in the azimuthal direction and the best illumination uniformity within a few picoseconds as well. Though the degree of improvement in the irradiance uniformity of the azimuthal smoothing scheme is lower than that of the radial smoothing, the combination of these two schemes can improve the uniformity effectively and rapidly. The novel smoothing scheme provides a potential smoothing approach for the high-power laser facilities.
[1] Miller G H, Moses E I, Wuest C R 2004 Nucl. Fusion 44 S228
Google Scholar
[2] Dixit S N, Thomas I M, Woods B W, Morgan A J, Henesian M A, Wegner P J, Powell H T 1993 Appl. Opt. 32 2543
Google Scholar
[3] Rushford M C, Dixit S N, Thomas I M, Martin A M, Perry M D 2000 Proc. SPIE 87 3654
[4] Néauport J, Ribeyre X, Daurios J, Valla D, Martine L, Beau V, Videau L 2003 Appl. Opt. 42 2377
Google Scholar
[5] Boehly T R, Babushkin A, Bradley D K, Craxton R S, Delettrez J A, Epstein R, Kessler T J, Knayer J P, McCrory R L, McKenty P W, Meyerhofer D D, Regan S, Seka W, Skupsky S, Smalyuk V A, Town R P J, Yaakobi B 2001 Laser Part. Beams 18 11
[6] Smalyuk V A, Boehly T R, Bradley D K, Goncharov V N, Delettrez J A, Knauer J P, Meyerhofer D D, Oron D, Shvarts D 1998 Phys. Rev. Lett. 81 5342
[7] Skupsky S, Short R W, Kessler T, Craxton R S, Letzring S, Soures J M 1989 J. Appl. Phys. 66 3456
Google Scholar
[8] Glenzer S H, Suter L J, Turner R E, Macgowan B J, Estabrook K G, Blain M A, Dixit S N, Hammel B A, Kauffman R L, Kirkwood R K, Landen O L, Monteil M C, Moody J D, Orzechowski T J, Pennington D M, Stone G F, Weiland T L 1998 Phys. Rev. Lett. 80 2845
[9] Montgomery D S, Moody J D, Baldis H A, Afeyan B B, Berger R L, Estabrook K G, Lasinski B F, Williams E A 1996 Phys. Plasmas 3 2029
Google Scholar
[10] Zhong Z, Hou P, Zhang B 2015 Opt. Lett. 40 5850
Google Scholar
[11] Chen J, Kuang D F, Gui M, Fang Z L 2009 Chin. Phys. Lett. 26 102
[12] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[13] Paisner J A, Murray J R 1997 17th IEEE/NPSS Symposium on Fusion Engineering San Diego, CA (United States), October 6−10, 1997 p57
[14] Wisoff P J, Bowers M W 2004 Proc. SPIE 5341 146
Google Scholar
[15] 刘兰琴, 张颖, 耿远超, 王文义, 朱启华, 景峰, 魏晓峰, 黄晚晴 2014 63 164201
Google Scholar
Liu L Q, Zhang Y, Geng Y C, Wang W Y, Zhu Q H, Jing F, Wei X F, Huang W Q 2014 Acta Phys. Sin. 63 164201
Google Scholar
[16] Wang Y, Wang F, Zhang Y, Huang X, Hu D, Zheng W, Zhu R, Deng X 2017 Appl. Opt. 56 8087
Google Scholar
[17] Schneider M B, Maclaren S A, Widmann K, Meezan N B, Hammer J H, Yoxall B E, Bell P M, Benedetti L R, Bradley D K, Callahan D A, Dewald E L, Doppner T, Eder D C, Edwards M J, Guymer T M, Hinkel D E, Hohenberger M, Hsing W W, Kervin M L, Kilkenny J D, Landen O L, Lindl J D, May M J, Michel P, Milovich J L, MoodyJ D, Moore A S, Ralph J E, Regan S P, Thomas C A, Wan A S 2015 Phys. Plasmas 22 122705
Google Scholar
[18] Sueda K, Miyaji G, Miyanaga N, Nakatsuka M 2004 Opt. Express 12 3548
Google Scholar
[19] Pennington D M, Henesian M A, Wilcox R B, Wilcox R B, Weiland T L, Eimerl D, Ehrlich R B, Laumann C W, Miller J L 1995 The 1st Annual International Conference on Solid-State Lasers for Application to Inertial Confinement Fusion California, American, May 30−June 2, 1995 p214
[20] Kotlyar V V, Almazov A A, Khonina S N, Soifer V A 2005 J. Opt. Soc. Am. A 22 849
Google Scholar
[21] Wang C, Liu T, Ren Y, Shao Q, Dong H 2018 Optik 171 404
[22] Guo C S, Xue D M, Han Y J, Ding J P 2006 Opt. Commun. 268 235
Google Scholar
-
图 2 靶面光强分布 (a) CPP+SSD; (b) CPP+RS; (c) CPP+AS
Fig. 2. Intensity distributions of target face: (a) CPP+SSD; (b) CPP+RS; (c) CPP+AS.
图 3 不同方案的焦斑特性 (a)光通量对比度积分时间的变化规律; (b) FOPAI
Fig. 3. Focal-spot characteristics of different schemes: (a) Change regulation of integral time of contrast; (b) FOPAI.
图 4 靶面光强分布 (a)—(f) 瞬时光强; (g) 平均光强
Fig. 4. Intensity distribution on target surface: (a)−(f) Instant intensity; (g) average intensity.
图 5 AS+RS联用的束匀滑方案 (a) 光通量对比度; (b) FOPAI; (c)焦斑光强分布; (d)散斑扫动速度径向分布
Fig. 5. Uniformity improvement of focal spot when AS is applied with RS: (a) Contrast curves; (b) FOPAI curves; (c) focal-spot intensity distribution; (d) swiping velocity distribution of speckles in radial direction.
图 6 焦斑光通量对比度随 (a)中心波长差
$ \Delta \lambda $ , (b)拓扑荷数|m|的变化Fig. 6. Contrast variations with different (a) central wavelength shift
$ \Delta \lambda $ and (b) topological charges |m|. -
[1] Miller G H, Moses E I, Wuest C R 2004 Nucl. Fusion 44 S228
Google Scholar
[2] Dixit S N, Thomas I M, Woods B W, Morgan A J, Henesian M A, Wegner P J, Powell H T 1993 Appl. Opt. 32 2543
Google Scholar
[3] Rushford M C, Dixit S N, Thomas I M, Martin A M, Perry M D 2000 Proc. SPIE 87 3654
[4] Néauport J, Ribeyre X, Daurios J, Valla D, Martine L, Beau V, Videau L 2003 Appl. Opt. 42 2377
Google Scholar
[5] Boehly T R, Babushkin A, Bradley D K, Craxton R S, Delettrez J A, Epstein R, Kessler T J, Knayer J P, McCrory R L, McKenty P W, Meyerhofer D D, Regan S, Seka W, Skupsky S, Smalyuk V A, Town R P J, Yaakobi B 2001 Laser Part. Beams 18 11
[6] Smalyuk V A, Boehly T R, Bradley D K, Goncharov V N, Delettrez J A, Knauer J P, Meyerhofer D D, Oron D, Shvarts D 1998 Phys. Rev. Lett. 81 5342
[7] Skupsky S, Short R W, Kessler T, Craxton R S, Letzring S, Soures J M 1989 J. Appl. Phys. 66 3456
Google Scholar
[8] Glenzer S H, Suter L J, Turner R E, Macgowan B J, Estabrook K G, Blain M A, Dixit S N, Hammel B A, Kauffman R L, Kirkwood R K, Landen O L, Monteil M C, Moody J D, Orzechowski T J, Pennington D M, Stone G F, Weiland T L 1998 Phys. Rev. Lett. 80 2845
[9] Montgomery D S, Moody J D, Baldis H A, Afeyan B B, Berger R L, Estabrook K G, Lasinski B F, Williams E A 1996 Phys. Plasmas 3 2029
Google Scholar
[10] Zhong Z, Hou P, Zhang B 2015 Opt. Lett. 40 5850
Google Scholar
[11] Chen J, Kuang D F, Gui M, Fang Z L 2009 Chin. Phys. Lett. 26 102
[12] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[13] Paisner J A, Murray J R 1997 17th IEEE/NPSS Symposium on Fusion Engineering San Diego, CA (United States), October 6−10, 1997 p57
[14] Wisoff P J, Bowers M W 2004 Proc. SPIE 5341 146
Google Scholar
[15] 刘兰琴, 张颖, 耿远超, 王文义, 朱启华, 景峰, 魏晓峰, 黄晚晴 2014 63 164201
Google Scholar
Liu L Q, Zhang Y, Geng Y C, Wang W Y, Zhu Q H, Jing F, Wei X F, Huang W Q 2014 Acta Phys. Sin. 63 164201
Google Scholar
[16] Wang Y, Wang F, Zhang Y, Huang X, Hu D, Zheng W, Zhu R, Deng X 2017 Appl. Opt. 56 8087
Google Scholar
[17] Schneider M B, Maclaren S A, Widmann K, Meezan N B, Hammer J H, Yoxall B E, Bell P M, Benedetti L R, Bradley D K, Callahan D A, Dewald E L, Doppner T, Eder D C, Edwards M J, Guymer T M, Hinkel D E, Hohenberger M, Hsing W W, Kervin M L, Kilkenny J D, Landen O L, Lindl J D, May M J, Michel P, Milovich J L, MoodyJ D, Moore A S, Ralph J E, Regan S P, Thomas C A, Wan A S 2015 Phys. Plasmas 22 122705
Google Scholar
[18] Sueda K, Miyaji G, Miyanaga N, Nakatsuka M 2004 Opt. Express 12 3548
Google Scholar
[19] Pennington D M, Henesian M A, Wilcox R B, Wilcox R B, Weiland T L, Eimerl D, Ehrlich R B, Laumann C W, Miller J L 1995 The 1st Annual International Conference on Solid-State Lasers for Application to Inertial Confinement Fusion California, American, May 30−June 2, 1995 p214
[20] Kotlyar V V, Almazov A A, Khonina S N, Soifer V A 2005 J. Opt. Soc. Am. A 22 849
Google Scholar
[21] Wang C, Liu T, Ren Y, Shao Q, Dong H 2018 Optik 171 404
[22] Guo C S, Xue D M, Han Y J, Ding J P 2006 Opt. Commun. 268 235
Google Scholar
下载:
计量
- 文章访问数: 7607
- PDF下载量: 59
- 被引次数: 0
