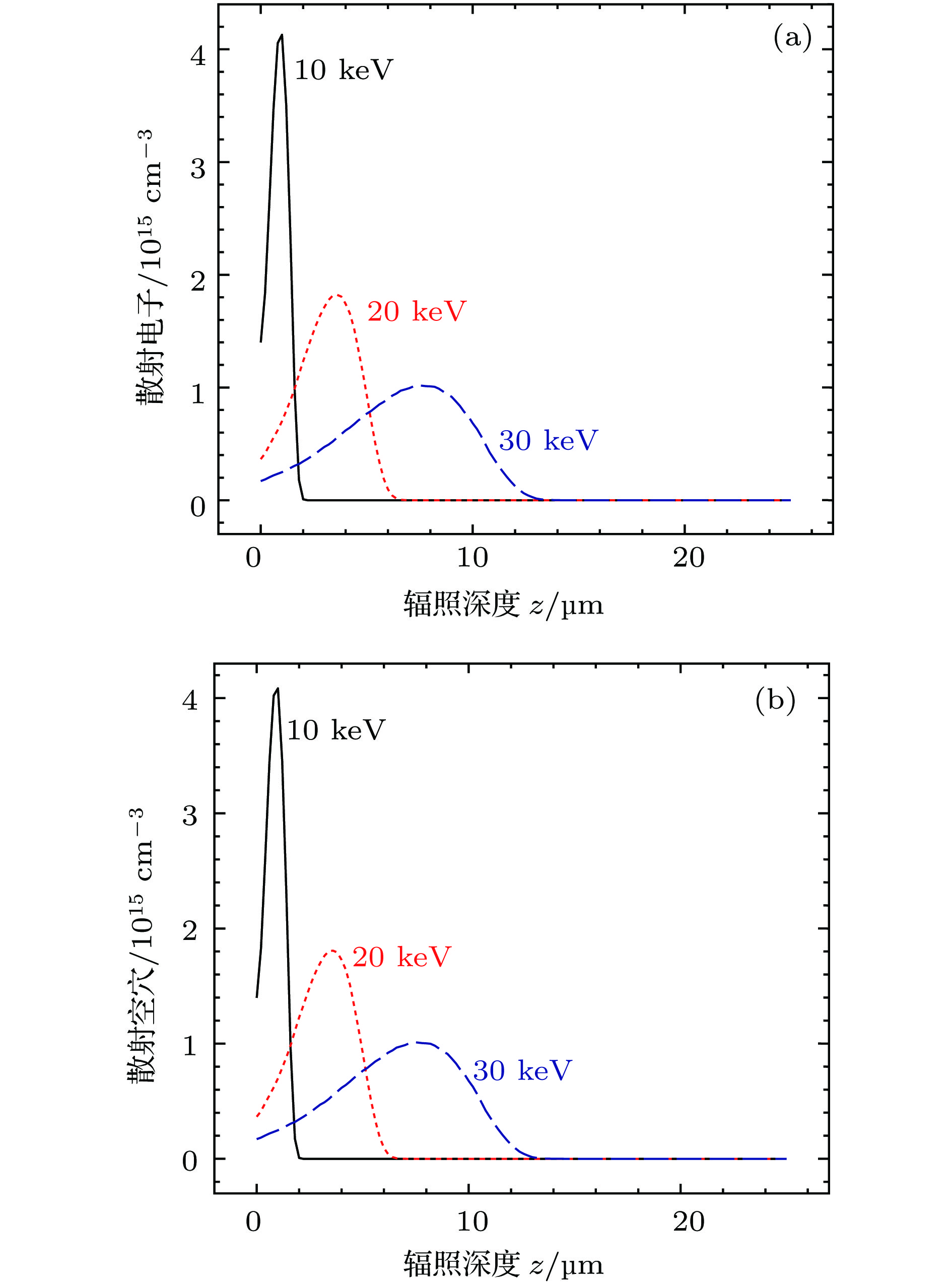
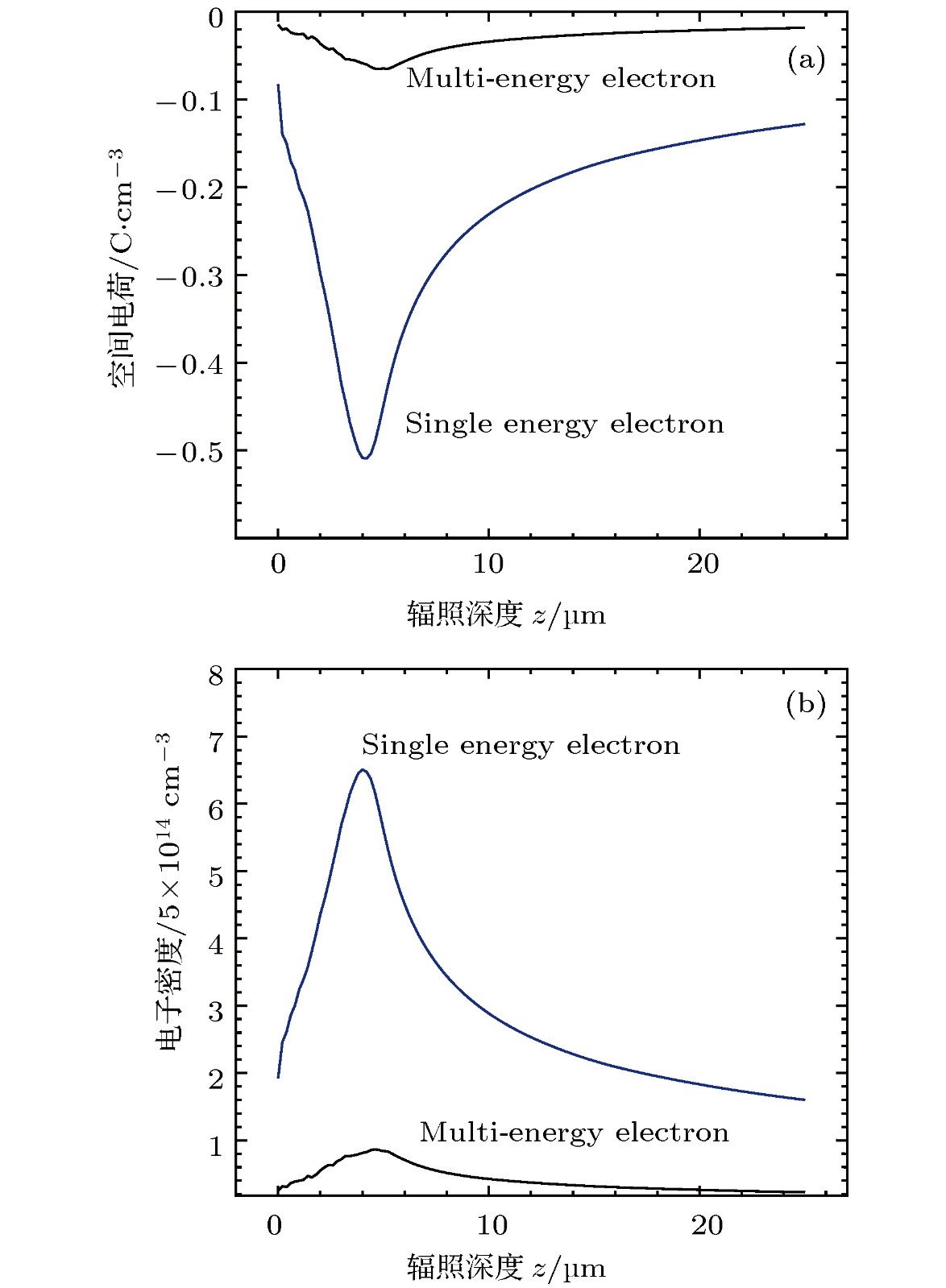
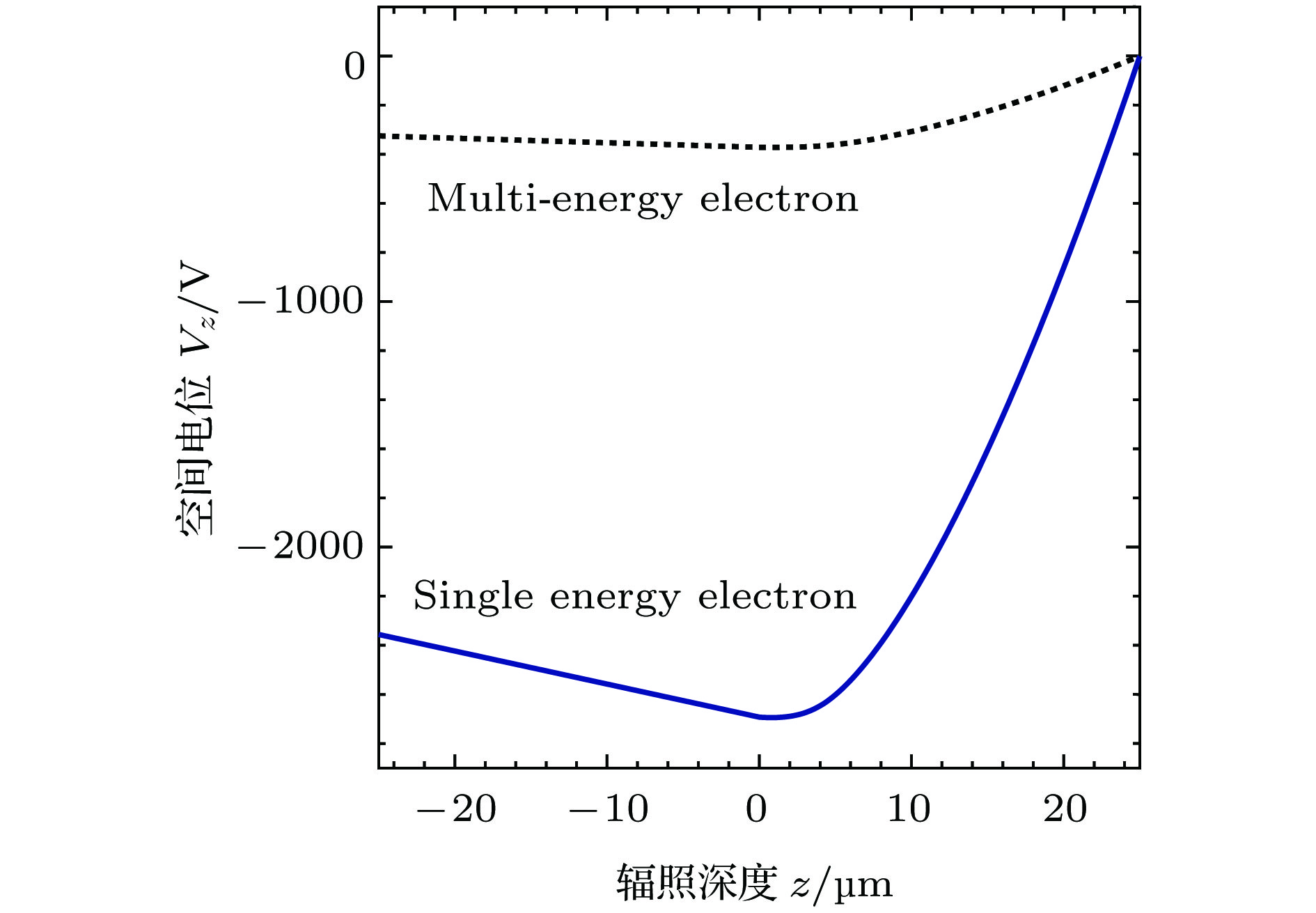
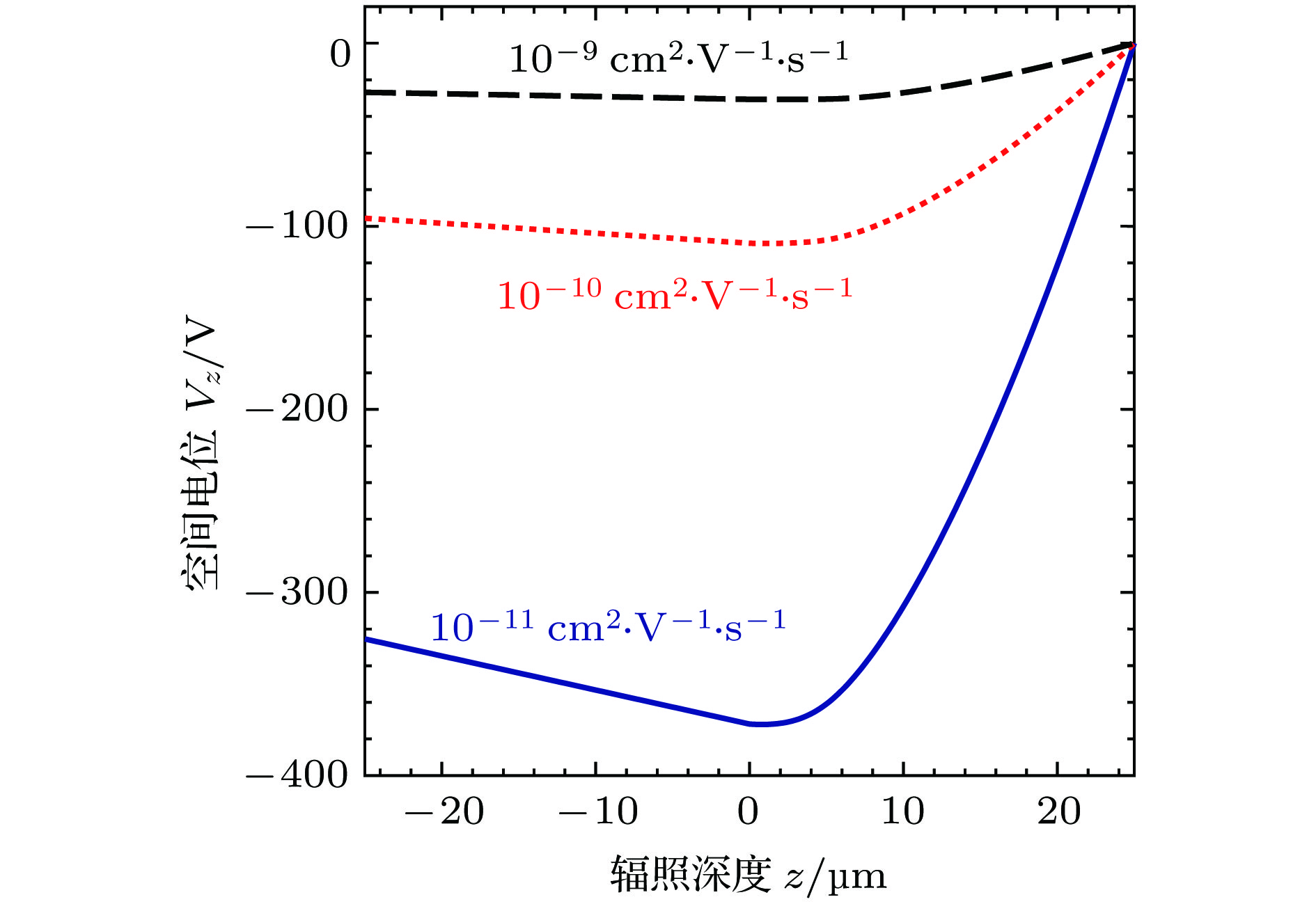
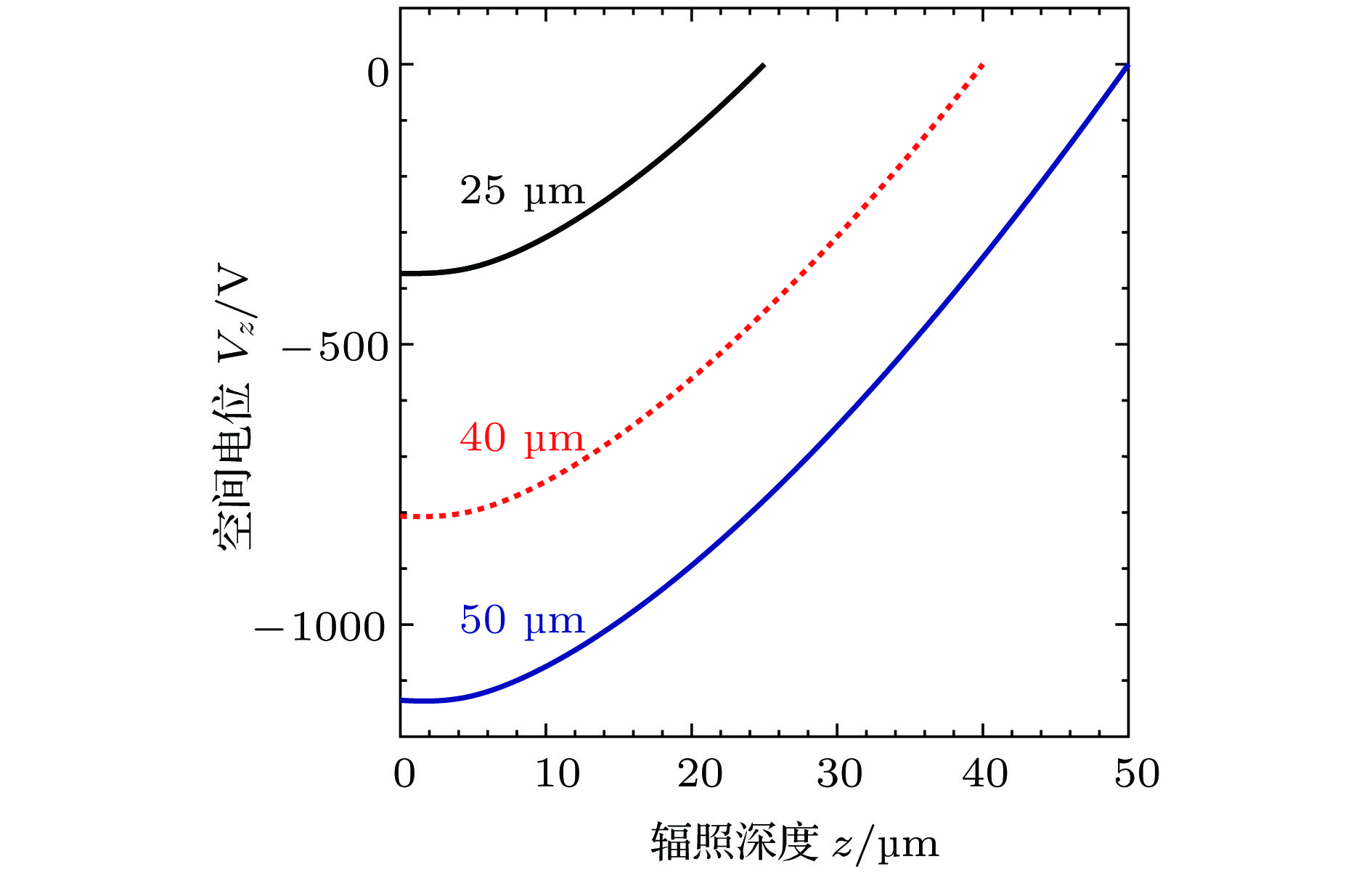
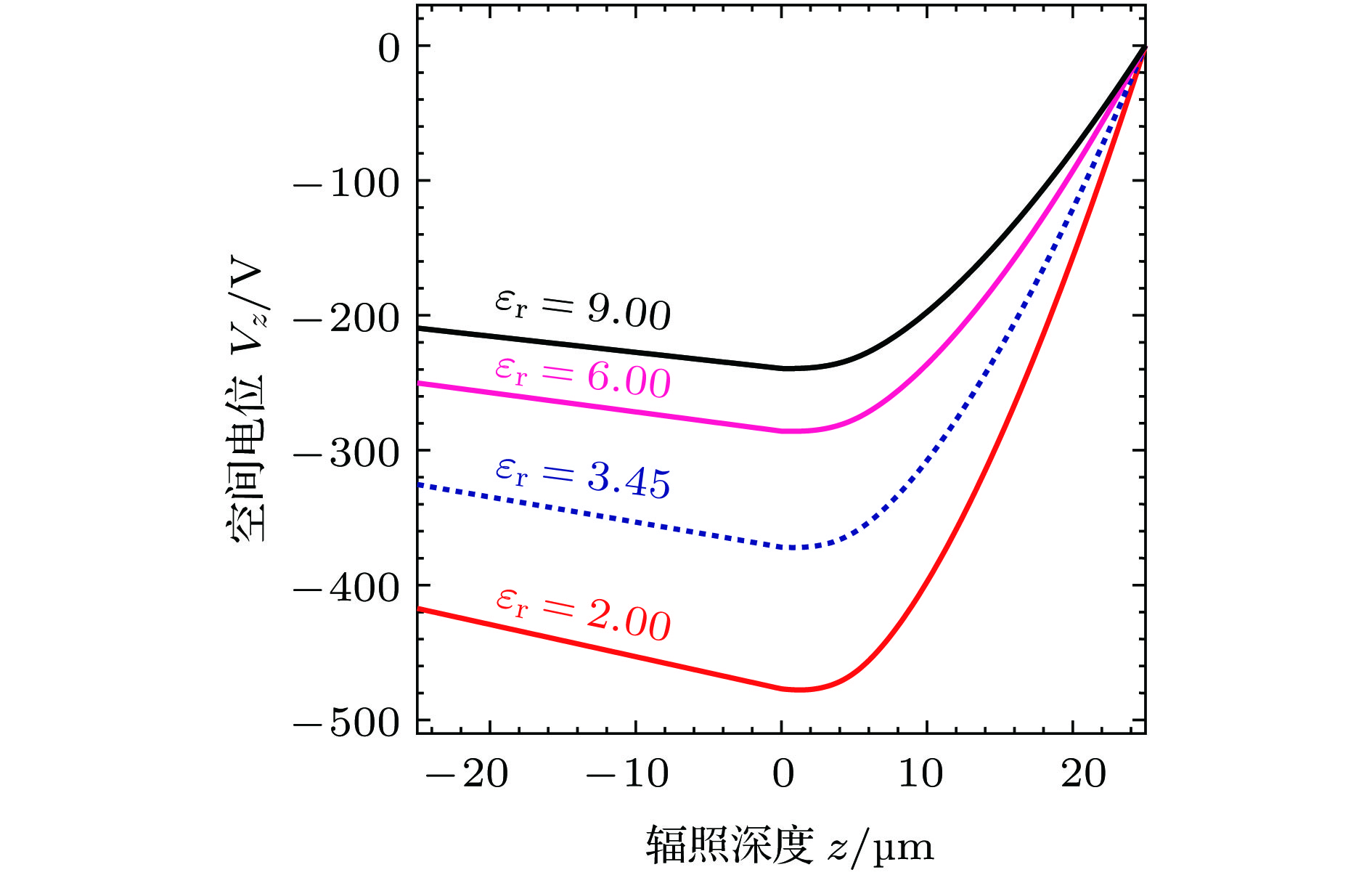
-
空间电子辐照聚合物的充电特性和微观机理是研究和防护航天器聚合物充放电特性的基础. 采用蒙特卡罗方法模拟空间电子的散射过程, 快二次电子模型模拟二次电子的产生, 有限差分法求解电荷连续性方程、电流密度方程和泊松方程的电荷输运过程, 俘获过程基于Poole-Frenkel效应来实现. 基于电子散射/输运同步模型基础, 结合法国国家航空航天科研局(ONERA)的地球同步轨道电子能谱分布理论公式和欧空局(SIRENE)机构的地面实验方法, 建立了基于地球同步轨道电子能谱分布的空间多能电子的散射模型. 通过空间电子辐照聚合物充电过程的数值模拟,获得了空间电荷密度、电位、电场和空间电位分布. 阐明了空间电子辐照聚合物的充电特性和样品微观参数与表面电位的关联性. 表面电位特性与实验结果相吻合, 单能电子的电位强度高于多能电子的电位. 充电达到稳态时, 电子迁移率较小时(小于10–11 cm2·V–1·s–1), 空间电位绝对值随电子迁移率的降低明显加强; 复合率较大时(大于10–14 cm3·s–1), 空间电位绝对值随复合率的增大而增大. 研究结果对于揭示空间电子辐照聚合物的充电特性和微观机理、提高航天器充放电故障机理研究水平 具有重要科学意义和价值.The charging characteristics and microscopic mechanism of space electrons irradiated polymers are the basis for the study and protection of spacecraft polymer charging and discharging characteristics. Monte Carlo method is used to simulate the scattering process of space electrons, and the fast secondary electron model simulates the generation of secondary electrons. The finite difference method is used to solve the charge transport process of charge continuity equation, current density equation and Poisson equation. The capture process realizes the transmission process of space electrons through the equation based on the Poole-French effect. Based on the electronic scattering/transport synchronization model and combined with the geostationary earth orbit electronic spectrum distribution theoretical formula of the French National Aeronautics and Space Research Agency (ONERA) and the ground experimental method of the agency (SIRNE), a scattering model based on the electron spectrum distribution in geosynchronous orbit is established. The numerical simulation of the charging process of space electrons irradiated polymers is carried out. The space charge density, space potential, electric field distribution and the space potential of polymer sample under the irradiation of single- and multi-energy electrons in space environment are obtained. The relationship among charging characteristics, microscopic parameters and surface potential of the sample is clarified. The surface potential characteristics of space electrons irradiated polymer are consistent with the experimental results. The single energy charge potential and strength are higher than those of multi-energy electrons. When the charging reaches a steady state, the electron mobility is smaller (less than 10–11 cm2·V–1·s–1), and the absolute value of the space potential is significantly enhanced with the decrease of the electron mobility. When the composite rate is large (greater than 10–14 cm3·s–1), the absolute value of the spatial potential increases with recombination rate increasing. The study of the charging characteristics of space electrons is not comprehensive because only the mode of single-energy electron irradiation is taken into consideration. The research results are of great scientific significance and practical value for revealing the charging characteristics and microscopic mechanism of space electrons irradiated polymer and improving the research level of spacecraft charge and discharge fault mechanism.
-
Keywords:
- space radiation /
- polyimide /
- charging characteristics /
- numerical simulation
[1] Balmain K G 1986 IEEE Trans. Electr. Insul. 21 427
[2] Garrett H B, Whittlesey A C 2000 IEEE Trans. Plasma Sci. 28 2017
Google Scholar
[3] 李盛涛, 李国倡, 闵道敏, 赵妮 2013 62 059401
Google Scholar
Li S T, Li G C, Min D M, Zhao N 2013 Acta Phys. Sin. 62 059401
Google Scholar
[4] 曹鹤飞, 刘尚合, 孙永卫, 原青云 2013 62 119401
Google Scholar
Cao H F, Liu S H, Sun Y W, Yuan Q Y 2013 Acta Phys. Sin. 62 119401
Google Scholar
[5] 黄建国, 易忠, 孟立飞, 赵华, 刘业楠 2013 62 099401
Google Scholar
Huang J G, Yi Z, Meng L F, Zhao H, Liu Y N 2013 Acta Phys. Sin. 62 099401
Google Scholar
[6] 袁端磊, 闵道敏, 黄印, 谢东日, 王海燕, 杨芳, 朱志豪, 费翔, 李盛涛 2017 66 097701
Google Scholar
Yuan D L, Min D M, Huang Y, Xie D R, Wang H Y, Yang F, Zhu Z H, Fei X, Li S T 2017 Acta Phys. Sin. 66 097701
Google Scholar
[7] 王松, 武占成, 唐小金, 孙永卫, 易忠 2016 65 025201
Google Scholar
Wang S, Wu Z C, Tang X J, Sun Y W, Yi Z 2016 Acta Phys. Sin. 65 025201
Google Scholar
[8] 刘婧, 张海波 2014 63 149401
Google Scholar
Liu J, Zhang H B 2014 Acta Phys. Sin. 63 149401
Google Scholar
[9] 杨文龙, 韩浚生, 王宇, 林家齐, 何国强, 孙洪国 2017 66 227101
Google Scholar
Yang W L, Han J S, Wang Y, Lin J Q, He G Q, Sun H G 2017 Acta Phys. Sin. 66 227101
Google Scholar
[10] 侯堃, 张占文, 黄勇, 韦建军 2016 65 035203
Hou K, Zhang Z W, Huang Y, Wei J J 2016 Acta Phys. Sin. 65 035203
[11] Garrett H B, Evans R W, Whittlesey A C, Katz I, Jun I 2008 IEEE Trans. Plasma Sci. 36 2440
Google Scholar
[12] Riffaud J, Griseri V, Berquez L 2016 Rev. Sci. Instrum. 87 073901
Google Scholar
[13] Koons H, Mazur J, Lopatin A, Pitchford D, Bogorad A, Herschitz R 2006 J. Spacer. Rockets 43 178
Google Scholar
[14] Czeremuszkin G, Latreche M, Wertheimer M R 2001 Nucl. Instrum. Meth. B 185 88
Google Scholar
[15] Okumura T, Masui H, Toyoda K, Cho M G, Nitta K, Imaizumi M 2009 J. Spacecr. Rockets 46 697
Google Scholar
[16] Toyoda K, Masui H, Muranaka T, Cho M, Urabe T, Miura T, Kawakita S, Gonohe Y, Kikuchi T 2008 IEEE Trans. Plasma Sci. 36 2413
Google Scholar
[17] Davis V A, Mandell M J, Cooke D C, Wheelock A, Matéo-Vélez J C, Roussel J F, Payan D, Cho M, Koga K 2013 IEEE Trans. Plasma Sci. 41 3303
Google Scholar
[18] Zhang P 2017 J. Comput. Theoret. Trans. 46 330
Google Scholar
[19] Donegan M M, Sample J L, Dennison J R, Hoffmann R 2010 J. Spacecr. Rockets 47 134
Google Scholar
[20] Liu J, Zhang H B, Ding Y H, Tong J S, Yuan Y, Zhao Q 2019 Micron 116 100
Google Scholar
[21] Liu J, Zhang H B 2014 Micron 67 12
[22] Sayehi M, Sahnoun R D, Fakhfakh S, Baklouti S 2018 Ceram. Int. 44 5202
Google Scholar
[23] Ammar L B, Fakhfakh S, Jbara O, Rondot S, Hadjadj A 2017 Micron 98 39
Google Scholar
[24] Fakhfakh S, Jbara O, Rondot S, Hadjadj A, Fakhfakh Z 2012 J. Non-Cryst. Solids 358 1157
Google Scholar
[25] 李维勤, 张海波 2008 57 3219
Google Scholar
Li W Q, Zhang H B 2008 Acta Phys. Sin. 57 3219
Google Scholar
[26] Li W Q, Zhang H B 2010 Appl. Surf. Sci. 256 3482
Google Scholar
[27] Li W Q, Zhang H B 2010 Micron 41 416
Google Scholar
[28] Sessler G M 1992 IEEE Trans. Electr. Insul. 27 961
Google Scholar
[29] Sessler G M, Figueiredo M T, Ferreira G F L 2004 IEEE Trans. Dielectr. Electr. Insul. 11 192
[30] Miyake H, Tanaka Y 2017 Sensor. Mater. 29 1213
[31] Hiro S, Tsuji K, Fujii H 1999 Electr. Eng. Jpn. 129 10
Google Scholar
[32] Miyake H, Honjoh M, Maruta S, Tanaka Y, Takada T, Koga K, Matsumoto H, Goka T, Dirassen B, Levy L, Payan D 2007 Annual Report Conference on Electrical Insulation and Dielectric Phenomena Vancouver, Canda, Oct. 14−17, 2007 p763
[33] Fitting H J, Touzin M 2011 J. Appl. Phys. 110 044111
Google Scholar
-
图 1 空间电子辐照聚酰亚胺(Kapton)样品的简化模型以及相关的电流密度示意图
Fig. 1. Simplified model of space electron irradiation of polyimide (Kapton) samples and diagram of associated current density.
图 3 空间电子散射 (a)散射电子密度分布; (b)散射空穴密度分布
Fig. 3. Space electron scattering: (a) Scattering electron density distribution; (b) scattering hole density distribution.
图 4 空间电子辐照聚合物空间电荷和电子密度分布 (a)空间电荷; (b)电子密度
Fig. 4. Space charge and electron density distribution of irradiated polymers: (a) Space charge; (b) electron density.
图 5 空间单能电子和多能电子辐照聚合物的空间电位分布
Fig. 5. Spatial potential distribution of polymer irradiated with single-and multi-electron energy in space.
图 6 空间单能电子和多能电子辐照聚合物的空间电场分布
Fig. 6. Distribution of space electric fields of polymer irradiated by space single-and multi-electron.
-
[1] Balmain K G 1986 IEEE Trans. Electr. Insul. 21 427
[2] Garrett H B, Whittlesey A C 2000 IEEE Trans. Plasma Sci. 28 2017
Google Scholar
[3] 李盛涛, 李国倡, 闵道敏, 赵妮 2013 62 059401
Google Scholar
Li S T, Li G C, Min D M, Zhao N 2013 Acta Phys. Sin. 62 059401
Google Scholar
[4] 曹鹤飞, 刘尚合, 孙永卫, 原青云 2013 62 119401
Google Scholar
Cao H F, Liu S H, Sun Y W, Yuan Q Y 2013 Acta Phys. Sin. 62 119401
Google Scholar
[5] 黄建国, 易忠, 孟立飞, 赵华, 刘业楠 2013 62 099401
Google Scholar
Huang J G, Yi Z, Meng L F, Zhao H, Liu Y N 2013 Acta Phys. Sin. 62 099401
Google Scholar
[6] 袁端磊, 闵道敏, 黄印, 谢东日, 王海燕, 杨芳, 朱志豪, 费翔, 李盛涛 2017 66 097701
Google Scholar
Yuan D L, Min D M, Huang Y, Xie D R, Wang H Y, Yang F, Zhu Z H, Fei X, Li S T 2017 Acta Phys. Sin. 66 097701
Google Scholar
[7] 王松, 武占成, 唐小金, 孙永卫, 易忠 2016 65 025201
Google Scholar
Wang S, Wu Z C, Tang X J, Sun Y W, Yi Z 2016 Acta Phys. Sin. 65 025201
Google Scholar
[8] 刘婧, 张海波 2014 63 149401
Google Scholar
Liu J, Zhang H B 2014 Acta Phys. Sin. 63 149401
Google Scholar
[9] 杨文龙, 韩浚生, 王宇, 林家齐, 何国强, 孙洪国 2017 66 227101
Google Scholar
Yang W L, Han J S, Wang Y, Lin J Q, He G Q, Sun H G 2017 Acta Phys. Sin. 66 227101
Google Scholar
[10] 侯堃, 张占文, 黄勇, 韦建军 2016 65 035203
Hou K, Zhang Z W, Huang Y, Wei J J 2016 Acta Phys. Sin. 65 035203
[11] Garrett H B, Evans R W, Whittlesey A C, Katz I, Jun I 2008 IEEE Trans. Plasma Sci. 36 2440
Google Scholar
[12] Riffaud J, Griseri V, Berquez L 2016 Rev. Sci. Instrum. 87 073901
Google Scholar
[13] Koons H, Mazur J, Lopatin A, Pitchford D, Bogorad A, Herschitz R 2006 J. Spacer. Rockets 43 178
Google Scholar
[14] Czeremuszkin G, Latreche M, Wertheimer M R 2001 Nucl. Instrum. Meth. B 185 88
Google Scholar
[15] Okumura T, Masui H, Toyoda K, Cho M G, Nitta K, Imaizumi M 2009 J. Spacecr. Rockets 46 697
Google Scholar
[16] Toyoda K, Masui H, Muranaka T, Cho M, Urabe T, Miura T, Kawakita S, Gonohe Y, Kikuchi T 2008 IEEE Trans. Plasma Sci. 36 2413
Google Scholar
[17] Davis V A, Mandell M J, Cooke D C, Wheelock A, Matéo-Vélez J C, Roussel J F, Payan D, Cho M, Koga K 2013 IEEE Trans. Plasma Sci. 41 3303
Google Scholar
[18] Zhang P 2017 J. Comput. Theoret. Trans. 46 330
Google Scholar
[19] Donegan M M, Sample J L, Dennison J R, Hoffmann R 2010 J. Spacecr. Rockets 47 134
Google Scholar
[20] Liu J, Zhang H B, Ding Y H, Tong J S, Yuan Y, Zhao Q 2019 Micron 116 100
Google Scholar
[21] Liu J, Zhang H B 2014 Micron 67 12
[22] Sayehi M, Sahnoun R D, Fakhfakh S, Baklouti S 2018 Ceram. Int. 44 5202
Google Scholar
[23] Ammar L B, Fakhfakh S, Jbara O, Rondot S, Hadjadj A 2017 Micron 98 39
Google Scholar
[24] Fakhfakh S, Jbara O, Rondot S, Hadjadj A, Fakhfakh Z 2012 J. Non-Cryst. Solids 358 1157
Google Scholar
[25] 李维勤, 张海波 2008 57 3219
Google Scholar
Li W Q, Zhang H B 2008 Acta Phys. Sin. 57 3219
Google Scholar
[26] Li W Q, Zhang H B 2010 Appl. Surf. Sci. 256 3482
Google Scholar
[27] Li W Q, Zhang H B 2010 Micron 41 416
Google Scholar
[28] Sessler G M 1992 IEEE Trans. Electr. Insul. 27 961
Google Scholar
[29] Sessler G M, Figueiredo M T, Ferreira G F L 2004 IEEE Trans. Dielectr. Electr. Insul. 11 192
[30] Miyake H, Tanaka Y 2017 Sensor. Mater. 29 1213
[31] Hiro S, Tsuji K, Fujii H 1999 Electr. Eng. Jpn. 129 10
Google Scholar
[32] Miyake H, Honjoh M, Maruta S, Tanaka Y, Takada T, Koga K, Matsumoto H, Goka T, Dirassen B, Levy L, Payan D 2007 Annual Report Conference on Electrical Insulation and Dielectric Phenomena Vancouver, Canda, Oct. 14−17, 2007 p763
[33] Fitting H J, Touzin M 2011 J. Appl. Phys. 110 044111
Google Scholar
下载:
计量
- 文章访问数: 6964
- PDF下载量: 35
- 被引次数: 0
