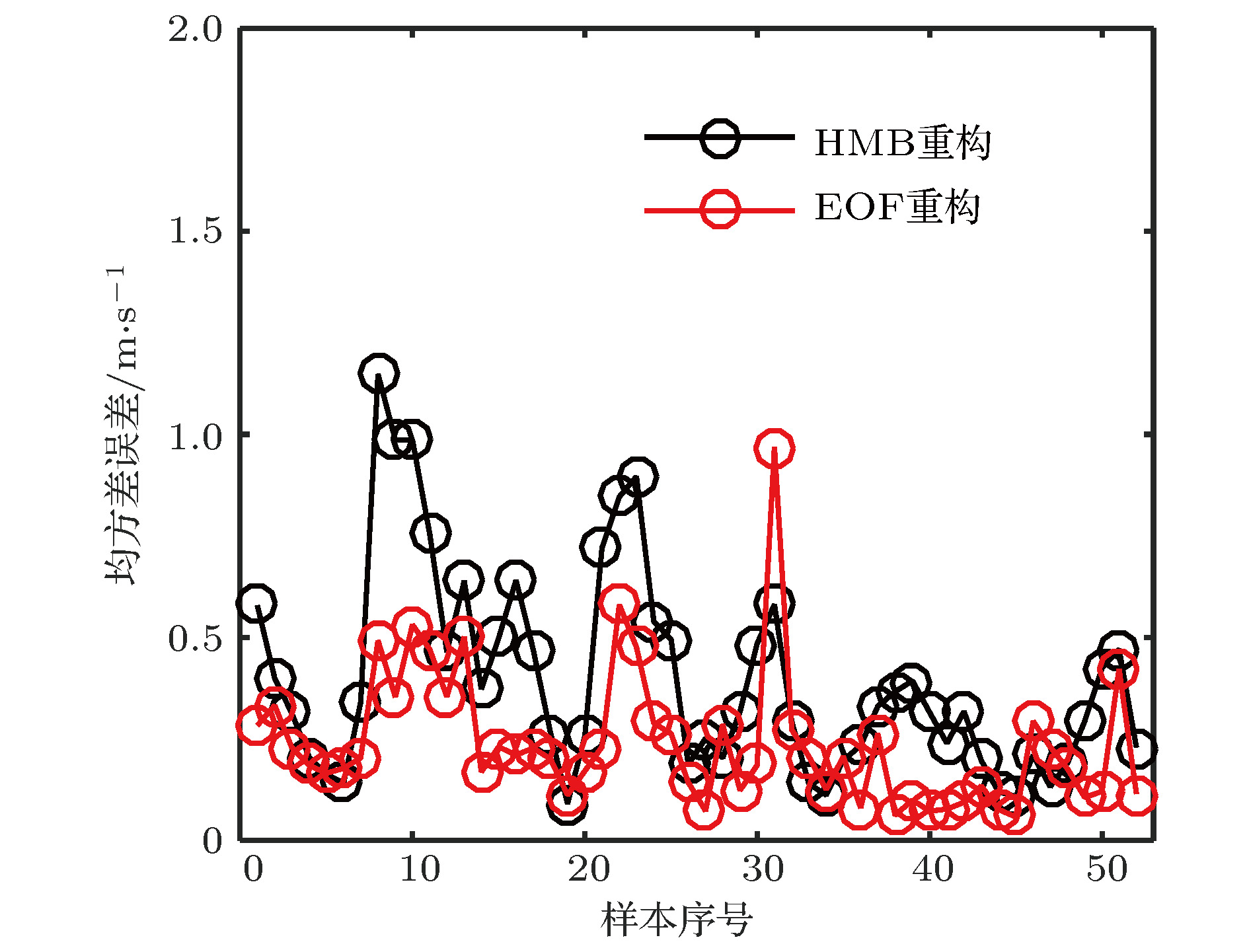
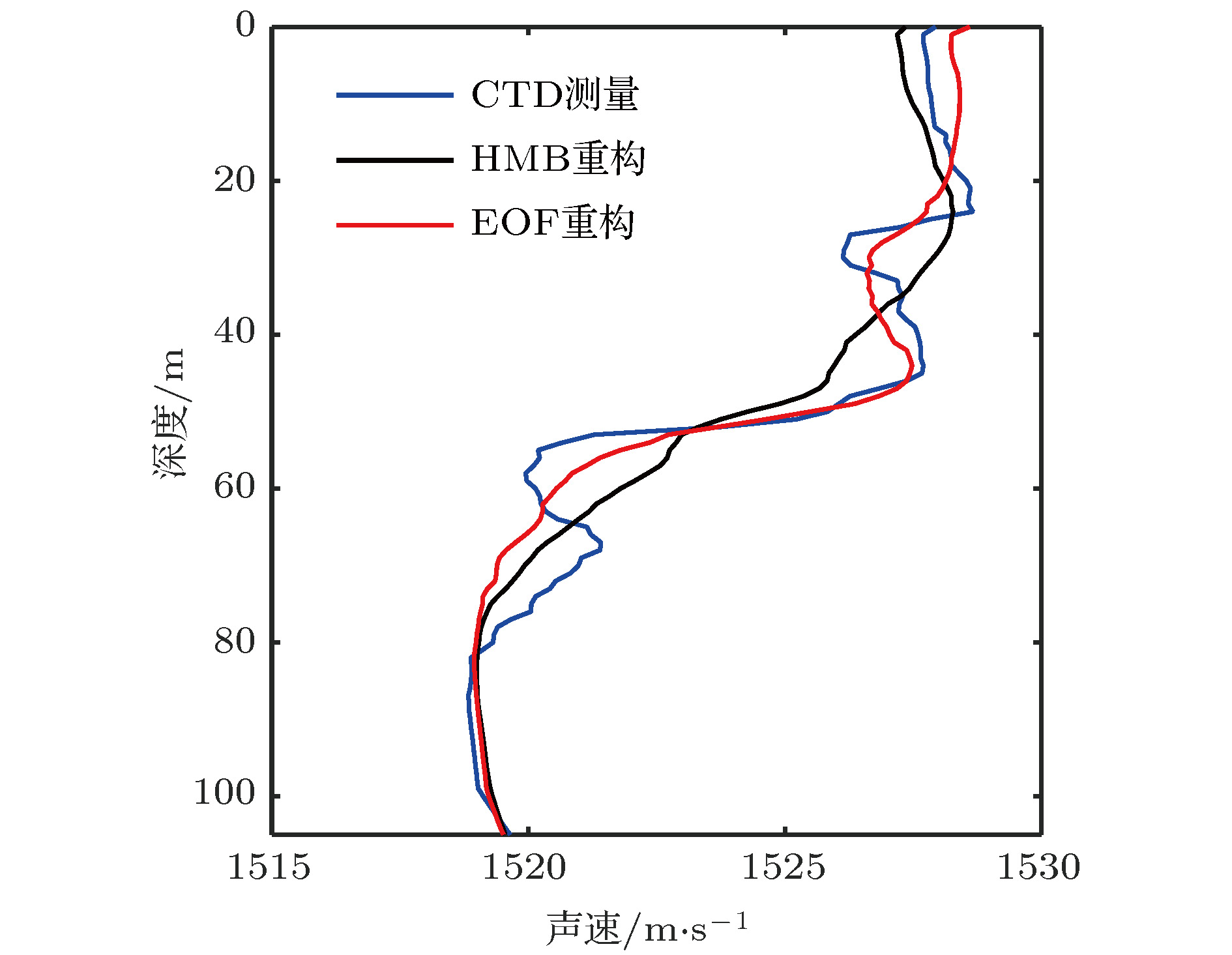
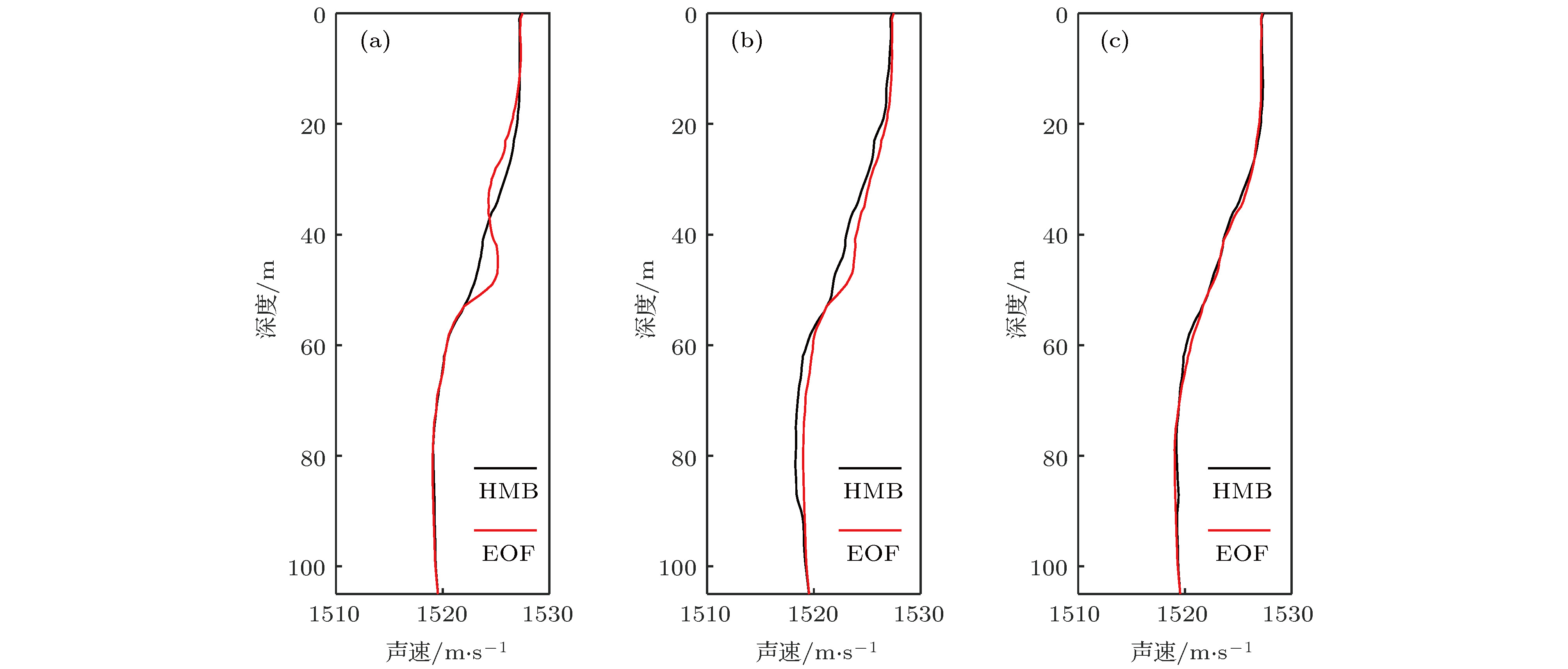
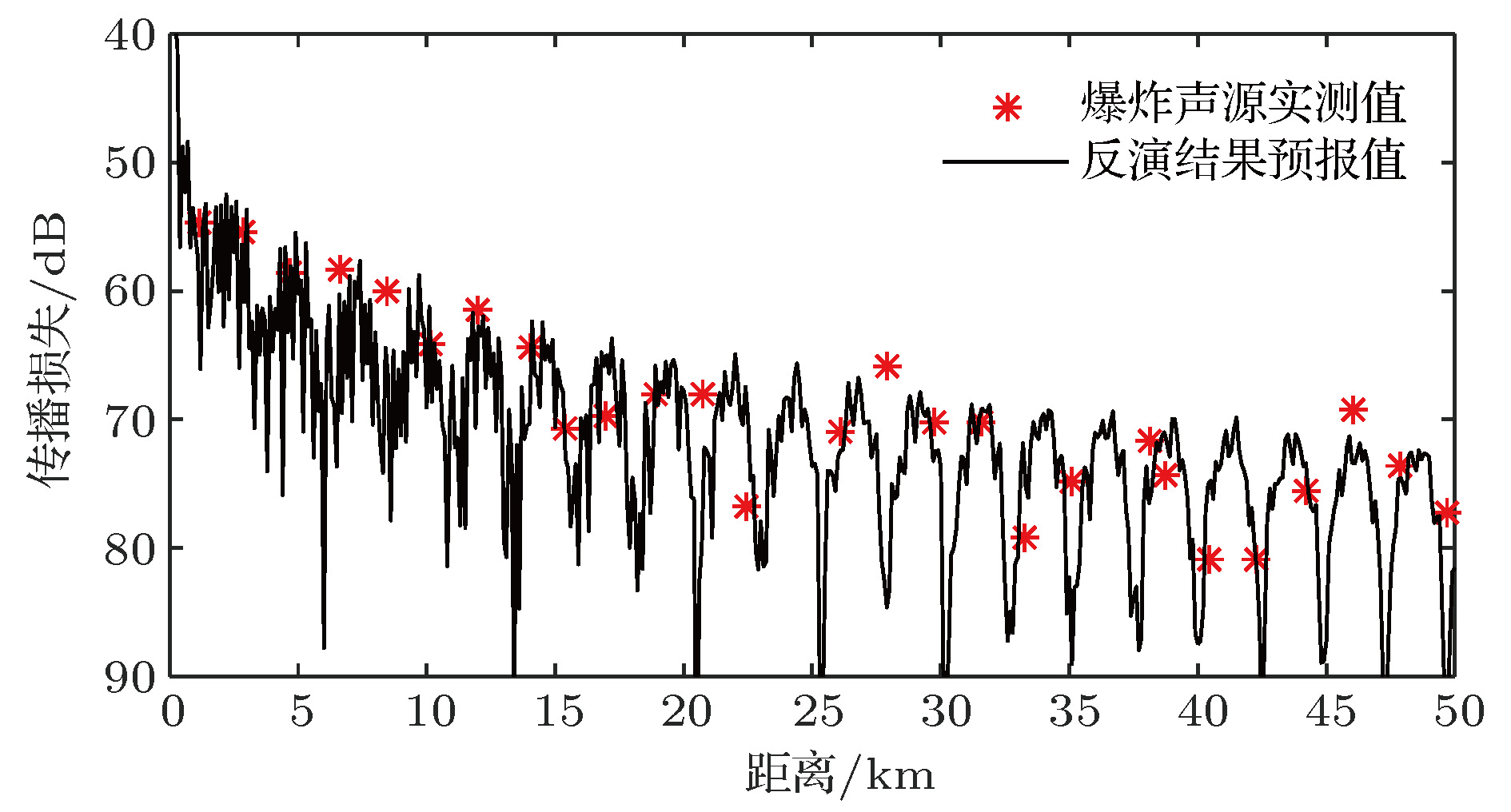
-
为了降低反演参数空间的维数, 常利用正交经验函数(EOF)来构建声速剖面. 然而, EOF方法的样本依赖性使之难以用于缺乏现场实测数据的海域. 本文提出一种全新的利用历史数据而不依靠现场实时数据即可获得的声速剖面展开基函数. 基于水质子流体静力方程和物态方程, 推导了在缺乏实时测量的情况下从历史数据获得水动力模式基函数(HMB)的办法. 利用WOA13季节平均温盐数据获得代表内潮动力特征的HMB进行分析. 较之EOF, HMB及其对应的投影系数与海洋动力特征直接相关并具有明确的物理含义. 基于东中国海实验获得的CTD (conductance-temperature-depth)及宽带爆炸声源声信号数据, 利用声速剖面重构以及匹配场声层析对HMB进行了分析, 并与EOF进行对比研究. 结果表明: HMB可以以较好的精度构建浅海声速剖面. 在对现场实时测量依赖更小的情况下, 基于HMB方法的声场预报及声层析结果与EOF方法一样好. HMB的获取更简单且直接关联海水的物理特性, 该方法可在实时测量样本不足的海域有效替代EOF进行海洋动力现象的声学监测.In order to provide constraint to the number of inversion parameters, sound speed profile is often modeled by empirical orthogonal functions (EOFs). However, the EOF method, which is dependent on the sample data, is often difficult to apply due to insufficient real-time in-situ measurements. In this paper, we present a novel basis for reconstructing the sound speed profile, which can be obtained by using historical data without real-time sample. By deducing the dynamic equations and the state function of water particle, the hydrodynamic mode bases (HMBs) can be calculated from historical data without real-time in-situ measurement, and a method of constructing the sound speed profile is established by using the dynamic characteristics of seawater. The use of the World Ocean Atlas 2013 (WOA13) can obtain the seasonal profiles of temperature and salinity, and then the HMB which represents the dynamic characteristic of internal tides is obtained and analyzed. Unlike EOF, the HMB and its projection coefficients are directly related to the sea dynamic features and have a more explicit physical meaning. According to the orthogonality analysis of hydrodynamic mode, the first-order coefficient can be used to describe the depth change of sound speed iso-lines and the second-order coefficient can be used to describe the change of sound speed gradient. Based on the conductance-temperature-depth profiles and broadband data from underwater explosion collected in the East China Sea experiment of the Asian Seas International Acoustic Experiment, the HMB is tested and compared with the EOF in the sound speed profile reconstruction and matched field tomography. The results show that the sound speed profile in shallow water area can be expressed by the HMB with proper precision. By means of the conventional matched field tomography, the valid sound speed profile can also be obtained in the form of HMB coefficients. The result of transmission loss prediction and tomography from HMB are as good as those from EOF, while the HMB has less dependent on real-time in-situ measurement. The HMB is easy to obtain and closely related to the physical characteristics of seawater, it can be used as an efficient alternative to EOF for monitoring the marine dynamic phenomena in sea areas with insufficient real-time in-situ measurement.
-
Keywords:
- sound speed profile /
- acousic tomography /
- hydrodynamic mode bases /
- inversion
[1] Yang T C, Huang C F, Huang S H, Liu J Y 2017 IEEE J. Ocean. Eng. 42 663
Google Scholar
[2] Turgut A, Mignerey P C, Goldstein D J, Schindall J A 2013 J. Acoust. Soc. Am. 133 1981
Google Scholar
[3] 刘进忠, 高大治, 王宁 2009 中国科学 G辑 39 719
Liu J Z, Gao D Z, Wang N 2009 Sci. China G 39 719
[4] Bianco M, Gerstoft P 2017 J. Acoust. Soc. Am. 141 1749
Google Scholar
[5] Huang C F, Gerstoft P, Hodgkiss W S 2008 J. Acoust. Soc. Am. 123 162
Google Scholar
[6] Taroundakis M I, Papadakis J S 1993 J. Computat. Acoust. 1 395
Google Scholar
[7] Li Z L, He L, Zhang R H, Li F H, Yu Y X, Lin P 2015 Sci. China: Phys. Mech. Astron. 58 1
[8] 张维, 杨士莪, 黄益旺, 唐俊峰, 宋扬 2012 振动与冲击 31 6
Google Scholar
Zhang W, Yang S E, Huang Y W, Tang J F, Song Y 2012 J. Vib. Shock 31 6
Google Scholar
[9] Li F H, Zhang R H 2010 Chin. Phys. Lett. 27 084303
[10] 何利, 李整林, 彭朝晖, 吴立新, 刘建军 2011 中国科学: 物理学 力学 天文学 41 49
He L, Li Z L, Peng Z H, Wu L X, Liu J J 2011 Sci. China: Phys. Mech. Astron. 41 49
[11] 李佳, 杨坤德, 雷波, 何正耀 2012 61 084301
Google Scholar
Li J, Yang K D, Lei B, He Z Y 2012 Acta Phys. Sin. 61 084301
Google Scholar
[12] 张旭, 张永刚, 张健雪, 聂邦胜, 姚忠山 2010 海洋科学进展 28 498
Google Scholar
Zhang X, Zhang Y G, Zhang J X, Nie B S, Yao Z S 2010 Adv. Marine Sci. 28 498
Google Scholar
[13] Jensen J K, Hjelmervik K T, Østenstad P 2012 IEEE J. Ocean. Eng. 37 103
Google Scholar
[14] Hjelmervik K, Hjelmervik K T 2014 Ocean Dyn. 64 655
Google Scholar
[15] 李晓曼, 张明辉, 张海刚, 朴胜春, 刘亚琴, 周建波 2017 66 094302
Google Scholar
Li X M, Zhang M H, Zhang H G, Piao S C, Liu Y Q, Zhou J B 2017 Acta Phys. Sin. 66 094302
Google Scholar
[16] 苏林, 马力, 宋文华, 郭圣明, 鹿力成 2015 64 024302
Google Scholar
Su L, Ma L, Song W H, Guo S M, Lu L C 2015 Acta Phys. Sin. 64 024302
Google Scholar
[17] Collins M D, Kuperman W A 1991 J. Acoust. Soc. Am. 90 1410
Google Scholar
[18] Munk W H 1974 J. Acoust. Soc. Am. 55 220
Google Scholar
[19] Teague W J, Carron M J, Hogan P J 1990 J. Geophys. Res. Oceans 95 7167
Google Scholar
[20] 张旭, 张永刚, 张健雪, 董楠 2011 海洋学报 33 54
Zhang X, Zhang Y G, Zhang J X, Dong N 2011 Acta Oceanol. Sin. 33 54
[21] oyer T P, Antonov J I, Baranova O K, Coleman C, Garcia H E, Grodsky A, Johnson D R, Locarnini R A, Mishonov A V, O'Brien T D, Paver C R, Reagan J R, Seidov D, Smolyar I V, Zweng M M https://repository.library.noaa.gov/view/noaa/ 1291 [2018-4-19]
[22] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) p64
[23] 蔡树群 2015 内孤立波数值模式及其在南海区域的应用 (北京: 海洋出版社) 第10页
Cai S Q 2015 Internal Solitons Numerical Model and Its Application in the South China Sea (Beijing: Ocean Press) p10 (in Chinese)
[24] 郭圣明, 胡涛 2010 哈尔滨工程大学学报 31 967
Google Scholar
Guo S M, Hu T 2010 J. Harbin Engin. Univ. 31 967
Google Scholar
[25] 崔茂常, 乔方利, 莫军, 郭炳火 2002 海洋学报 24 127
Google Scholar
Cui M C, Qiao F L, Mo J, Guo B H 2002 Acta Oceanol. Sin. 24 127
Google Scholar
[26] 宋文华, 胡涛, 郭圣明, 马力, 鹿力成 2014 声学学报 39 11
Google Scholar
Song W H, Hu T, Guo S M, Ma L, Lu L C 2014 Acta Acust. 39 11
Google Scholar
[27] Dahl P H, Zhang R, Miller J H, Bartek L R, Peng Z, Ramp S R, Zhou J X, Chiu C S, Lynch J F, Simmen J A 2004 IEEE J. Ocean. Eng. 29 920
Google Scholar
[28] 何利, 李整林, 张仁和, 李风华 2006 自然科学进展 16 351
Google Scholar
He L, Li Z L, Zhang R H, Li F H 2006 Prog. Natural Sci. 16 351
Google Scholar
[29] Gerstoft P 1994 J. Acoust. Soc. Am. 95 770
-
图 1 CTD测得的声速剖面(细线)和平均声速(粗线)
Fig. 1. Sound speed profiles (thin line) and average sound speed profile (rough line) measured by CTD.
图 3 不同方法获得的声速剖面展开基比较(黑线, HMB方法; 红线, EOF方法)
Fig. 3. Comparison of the sound speed profile bases obtained by different methods (black line, HMB; red line, EOF).
图 6 声速剖面计算传播分析(单位: dB) (a) CTD测量; (b) HMB重构; (c) EOF重构
Fig. 6. Analysis of transmission loss calculated by sound speed profiles (dB): (a) CTD measurement; (b) HMB reconstruction; (c) EOF reconstruction.
图 8 1525 m/s等声速线深度和第一阶投影系数随样本的变化
Fig. 8. Variations of the depth at a sound speed of 1525 m/s and the first-order projection coefficient with samples.
图 9 声速梯度和第二阶投影系数随样本的变化
Fig. 9. Variations of the sound speed gradient and the second-order projection coefficient with samples.
图 11 不同距离的反演结果 (a) 10.2 km; (b) 10.2 km; (c) 12 km
Fig. 11. Inversion results at different ranges: (a) 10.2 km; (b) 10.2 km; (c)12 km.
图 12 3个反演参数的边缘概率密度分布 (红线为代价函数最优值对应参数)
Fig. 12. Probability distribution for the three inversion parameters (the red line is the optimum value for the objective function).
表 1 不同方法重构效果的误差分析(单位: m/s)
Table 1. Error analysis of different reconstruction methods (m/s).
阶数 1 2 3 4 5 6 7 8 HMB方法
均方根误差1.0 0.69 0.60 0.54 0.49 0.42 0.37 0.34 EOF方法
均方根误差0.76 0.59 0.47 0.42 0.34 0.29 0.25 0.22 
下载: 导出CSV
表 2 不同方法各阶所占声速剖面变化比重
Table 2. Proportion of sound speed variety for each order in different methods.
阶数 1 2 3 4 5 6 7 8 HMB方法
所占变化比55.6 18.1 9.3 4.7 3.7 3.1 2.1 1.8 EOF方法
所占变化比71.4 15.1 5.7 4.2 1.5 0.9 0.5 0.2
下载: 导出CSV
-
[1] Yang T C, Huang C F, Huang S H, Liu J Y 2017 IEEE J. Ocean. Eng. 42 663
Google Scholar
[2] Turgut A, Mignerey P C, Goldstein D J, Schindall J A 2013 J. Acoust. Soc. Am. 133 1981
Google Scholar
[3] 刘进忠, 高大治, 王宁 2009 中国科学 G辑 39 719
Liu J Z, Gao D Z, Wang N 2009 Sci. China G 39 719
[4] Bianco M, Gerstoft P 2017 J. Acoust. Soc. Am. 141 1749
Google Scholar
[5] Huang C F, Gerstoft P, Hodgkiss W S 2008 J. Acoust. Soc. Am. 123 162
Google Scholar
[6] Taroundakis M I, Papadakis J S 1993 J. Computat. Acoust. 1 395
Google Scholar
[7] Li Z L, He L, Zhang R H, Li F H, Yu Y X, Lin P 2015 Sci. China: Phys. Mech. Astron. 58 1
[8] 张维, 杨士莪, 黄益旺, 唐俊峰, 宋扬 2012 振动与冲击 31 6
Google Scholar
Zhang W, Yang S E, Huang Y W, Tang J F, Song Y 2012 J. Vib. Shock 31 6
Google Scholar
[9] Li F H, Zhang R H 2010 Chin. Phys. Lett. 27 084303
[10] 何利, 李整林, 彭朝晖, 吴立新, 刘建军 2011 中国科学: 物理学 力学 天文学 41 49
He L, Li Z L, Peng Z H, Wu L X, Liu J J 2011 Sci. China: Phys. Mech. Astron. 41 49
[11] 李佳, 杨坤德, 雷波, 何正耀 2012 61 084301
Google Scholar
Li J, Yang K D, Lei B, He Z Y 2012 Acta Phys. Sin. 61 084301
Google Scholar
[12] 张旭, 张永刚, 张健雪, 聂邦胜, 姚忠山 2010 海洋科学进展 28 498
Google Scholar
Zhang X, Zhang Y G, Zhang J X, Nie B S, Yao Z S 2010 Adv. Marine Sci. 28 498
Google Scholar
[13] Jensen J K, Hjelmervik K T, Østenstad P 2012 IEEE J. Ocean. Eng. 37 103
Google Scholar
[14] Hjelmervik K, Hjelmervik K T 2014 Ocean Dyn. 64 655
Google Scholar
[15] 李晓曼, 张明辉, 张海刚, 朴胜春, 刘亚琴, 周建波 2017 66 094302
Google Scholar
Li X M, Zhang M H, Zhang H G, Piao S C, Liu Y Q, Zhou J B 2017 Acta Phys. Sin. 66 094302
Google Scholar
[16] 苏林, 马力, 宋文华, 郭圣明, 鹿力成 2015 64 024302
Google Scholar
Su L, Ma L, Song W H, Guo S M, Lu L C 2015 Acta Phys. Sin. 64 024302
Google Scholar
[17] Collins M D, Kuperman W A 1991 J. Acoust. Soc. Am. 90 1410
Google Scholar
[18] Munk W H 1974 J. Acoust. Soc. Am. 55 220
Google Scholar
[19] Teague W J, Carron M J, Hogan P J 1990 J. Geophys. Res. Oceans 95 7167
Google Scholar
[20] 张旭, 张永刚, 张健雪, 董楠 2011 海洋学报 33 54
Zhang X, Zhang Y G, Zhang J X, Dong N 2011 Acta Oceanol. Sin. 33 54
[21] oyer T P, Antonov J I, Baranova O K, Coleman C, Garcia H E, Grodsky A, Johnson D R, Locarnini R A, Mishonov A V, O'Brien T D, Paver C R, Reagan J R, Seidov D, Smolyar I V, Zweng M M https://repository.library.noaa.gov/view/noaa/ 1291 [2018-4-19]
[22] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) p64
[23] 蔡树群 2015 内孤立波数值模式及其在南海区域的应用 (北京: 海洋出版社) 第10页
Cai S Q 2015 Internal Solitons Numerical Model and Its Application in the South China Sea (Beijing: Ocean Press) p10 (in Chinese)
[24] 郭圣明, 胡涛 2010 哈尔滨工程大学学报 31 967
Google Scholar
Guo S M, Hu T 2010 J. Harbin Engin. Univ. 31 967
Google Scholar
[25] 崔茂常, 乔方利, 莫军, 郭炳火 2002 海洋学报 24 127
Google Scholar
Cui M C, Qiao F L, Mo J, Guo B H 2002 Acta Oceanol. Sin. 24 127
Google Scholar
[26] 宋文华, 胡涛, 郭圣明, 马力, 鹿力成 2014 声学学报 39 11
Google Scholar
Song W H, Hu T, Guo S M, Ma L, Lu L C 2014 Acta Acust. 39 11
Google Scholar
[27] Dahl P H, Zhang R, Miller J H, Bartek L R, Peng Z, Ramp S R, Zhou J X, Chiu C S, Lynch J F, Simmen J A 2004 IEEE J. Ocean. Eng. 29 920
Google Scholar
[28] 何利, 李整林, 张仁和, 李风华 2006 自然科学进展 16 351
Google Scholar
He L, Li Z L, Zhang R H, Li F H 2006 Prog. Natural Sci. 16 351
Google Scholar
[29] Gerstoft P 1994 J. Acoust. Soc. Am. 95 770
下载:
计量
- 文章访问数: 9089
- PDF下载量: 95
- 被引次数: 0
