Discrete unified gas kinetic simulation of characteristics of variable temperature wall driven thermal creep flow in cavity

LIU Zanqi; LUO Yuan; WENG Wanliang; HE Qing; TAO Shi

doi:10.7498/aps.74.20241334

Abstract

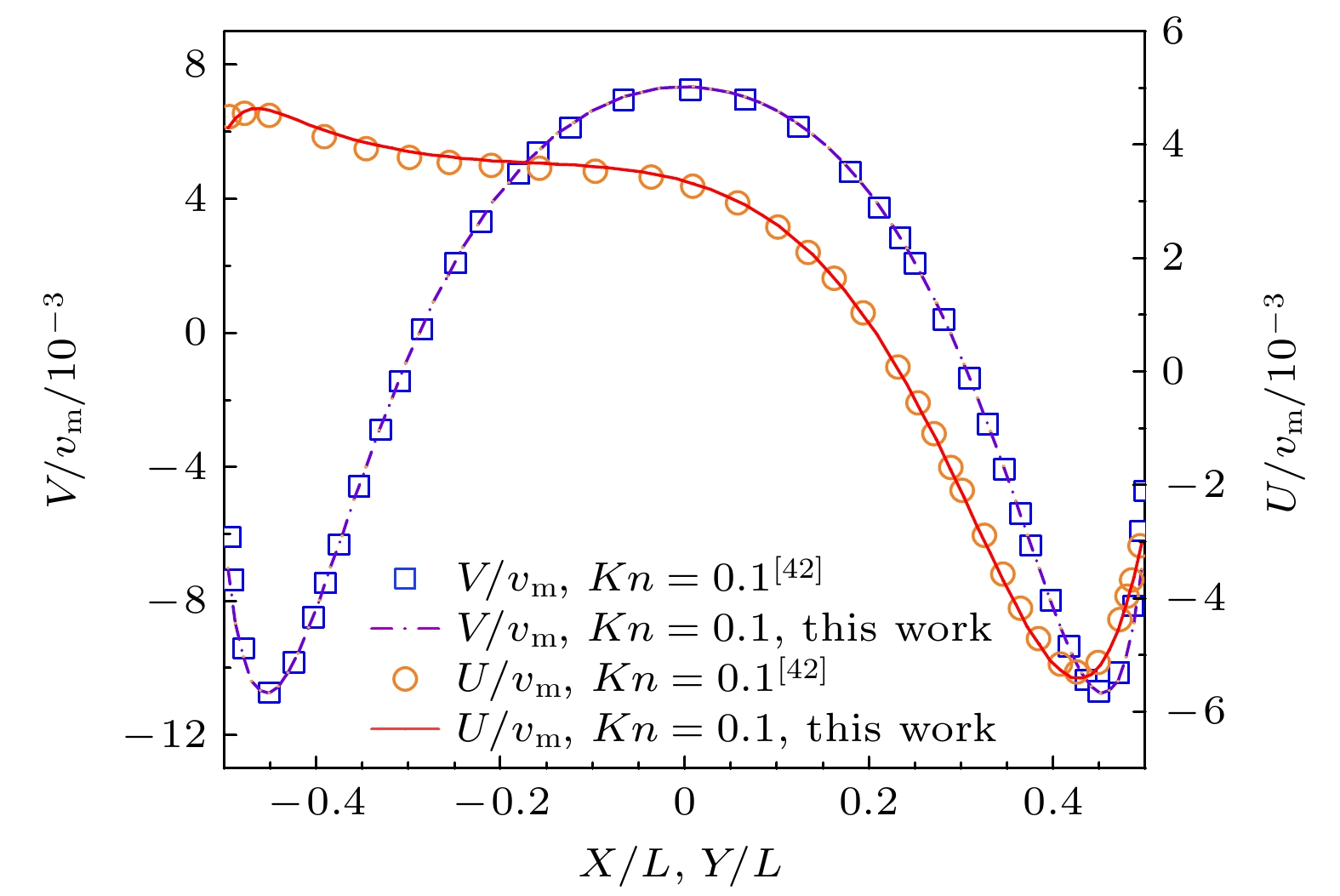
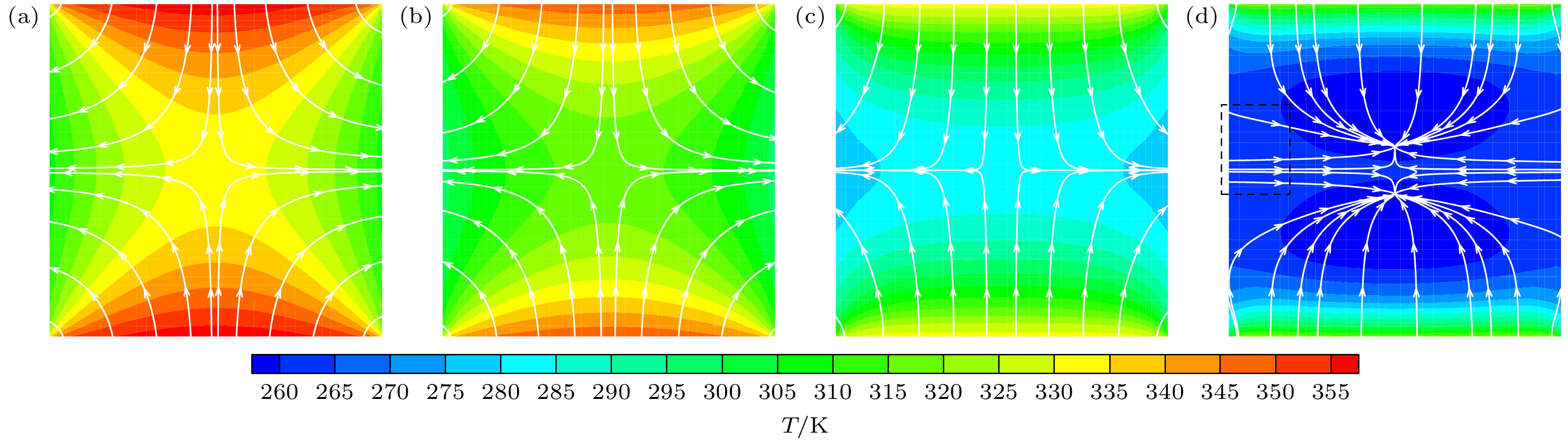
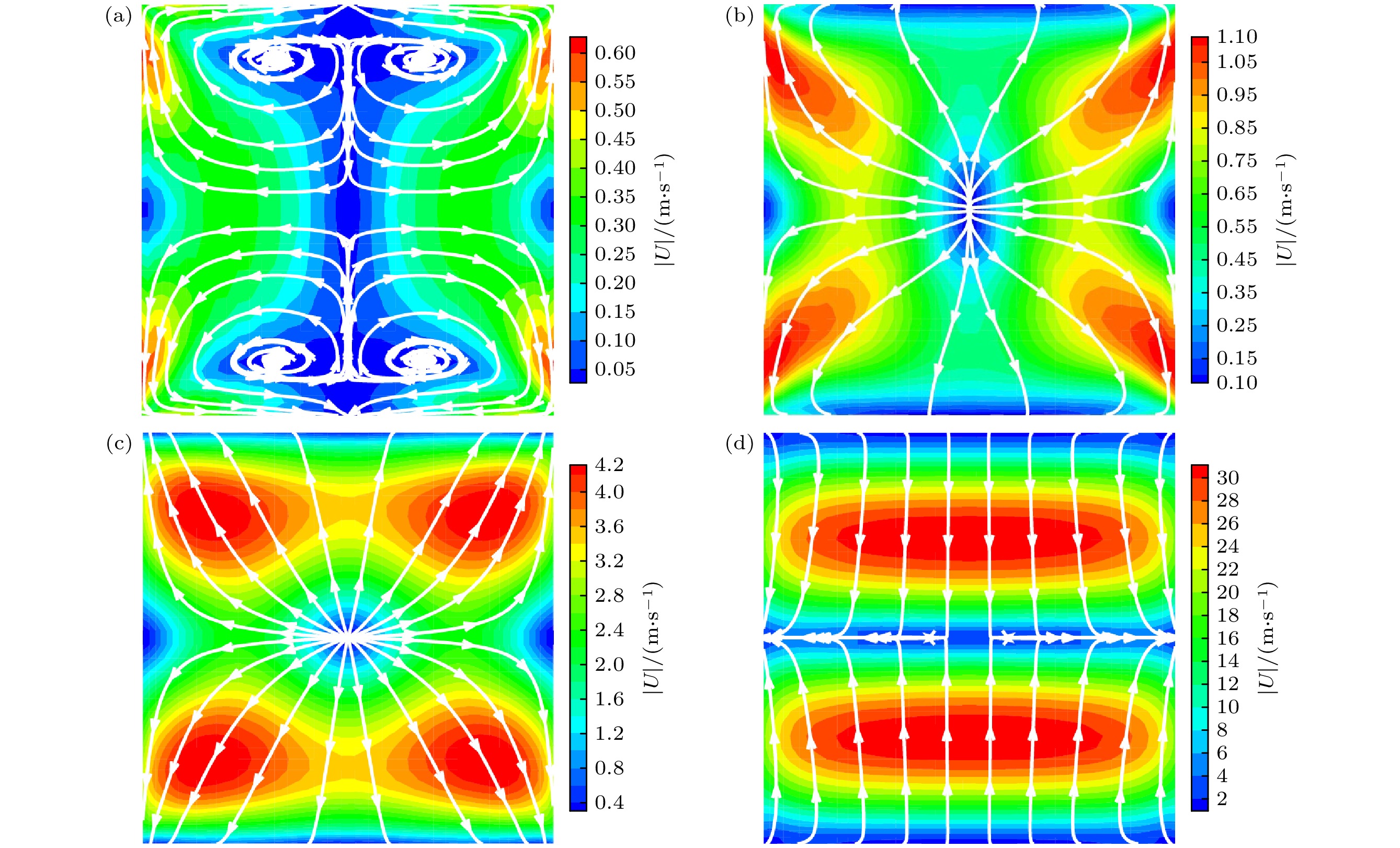
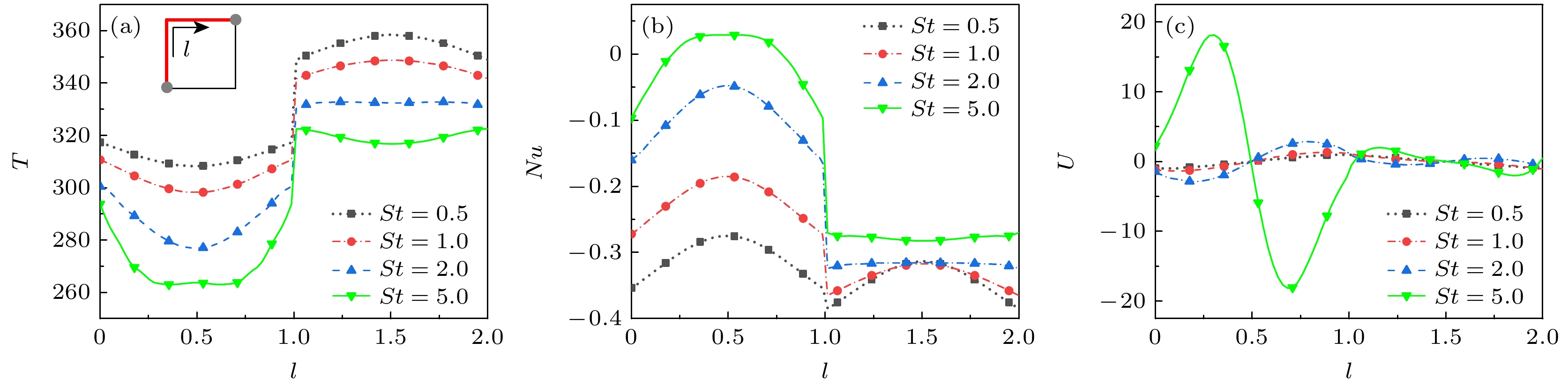
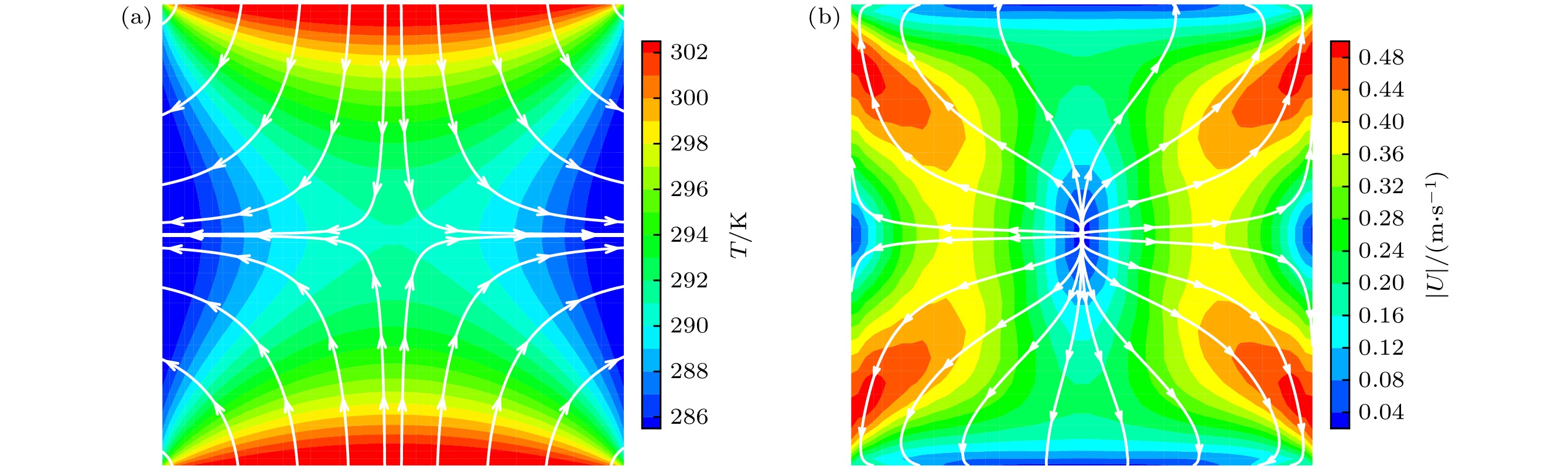
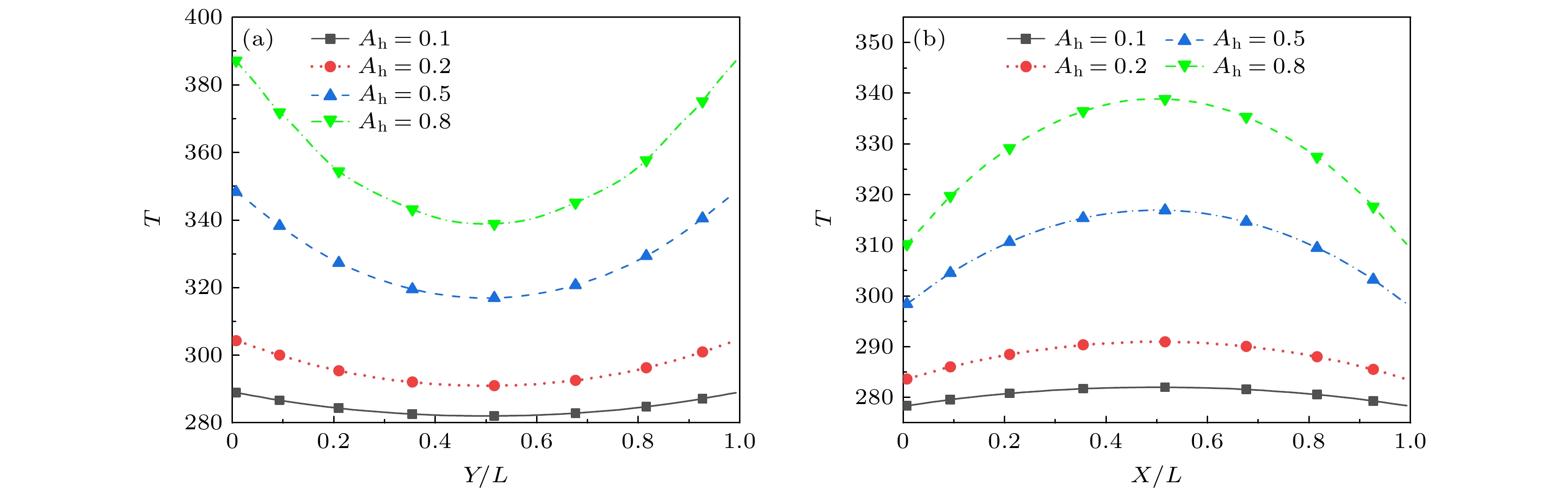
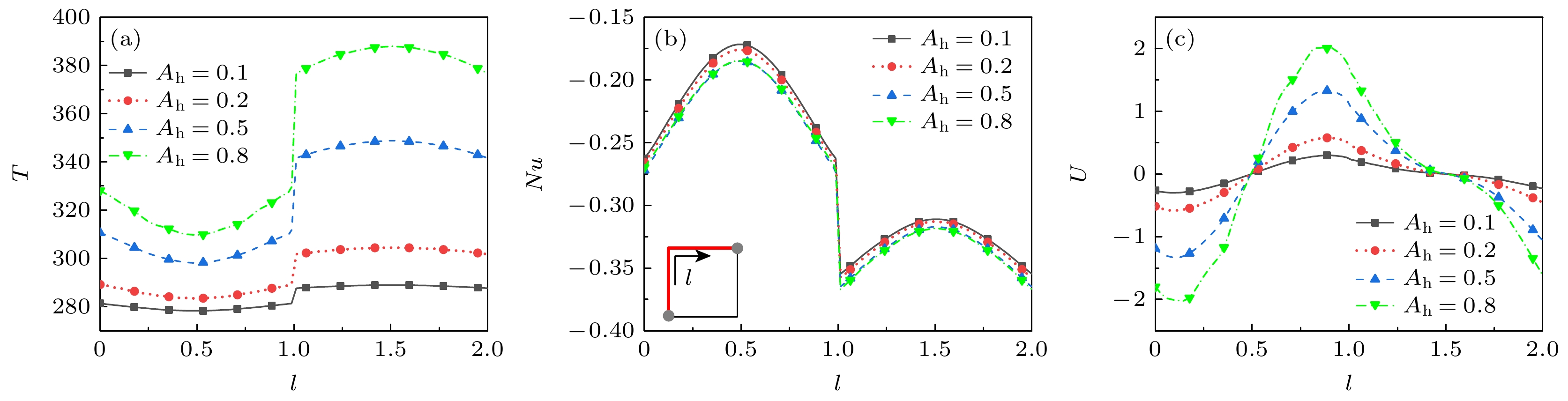
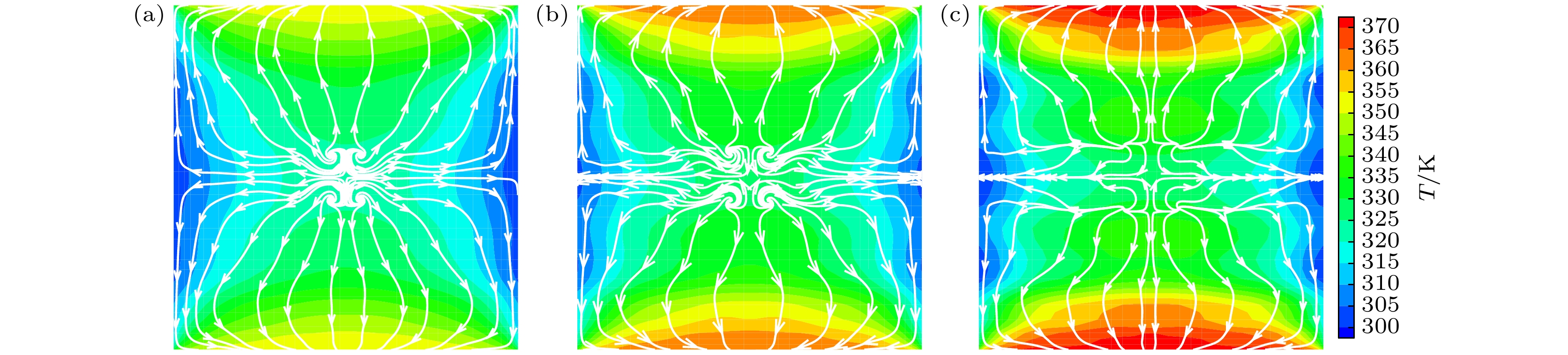
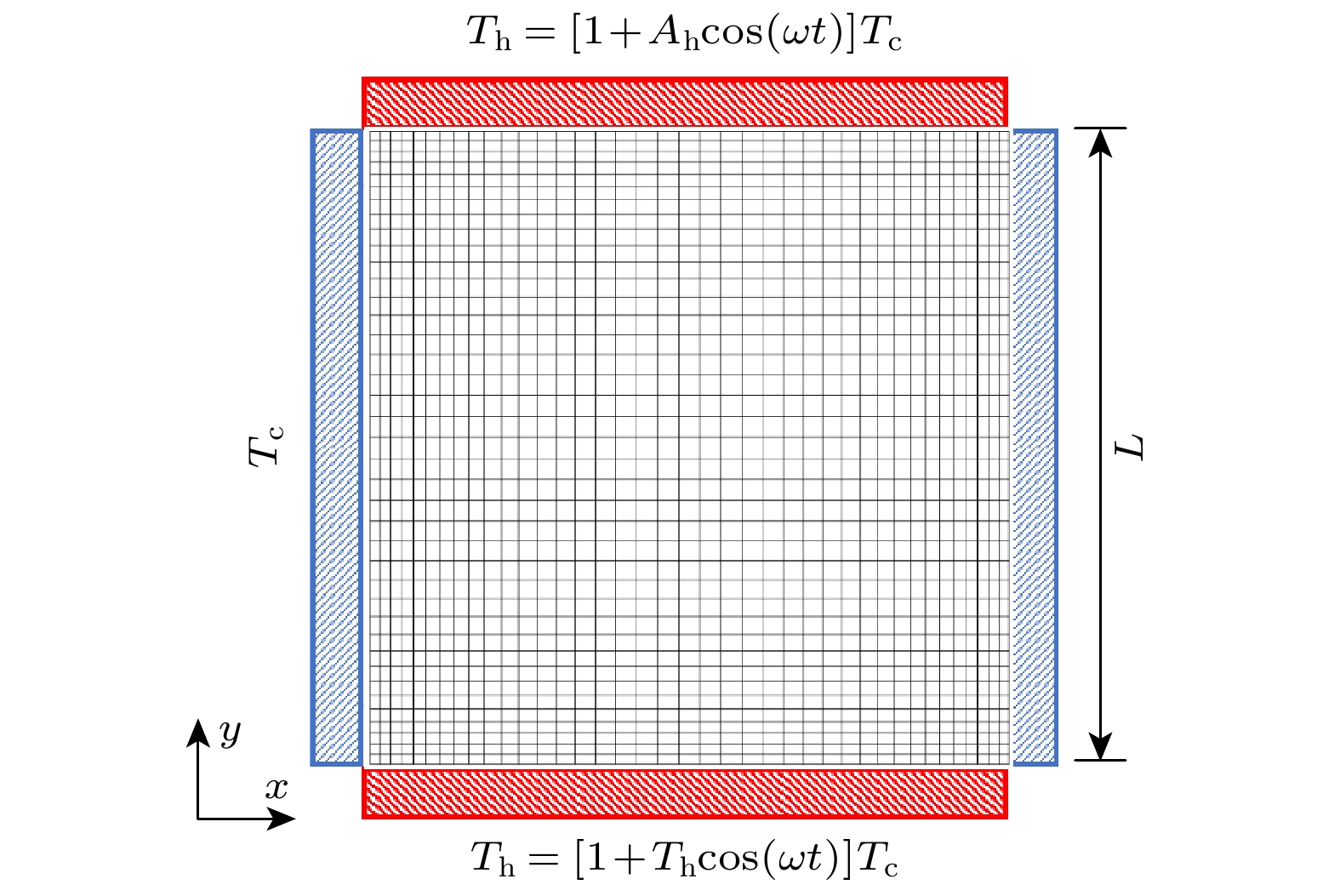
In order to examine the influence of wall temperature change on the flow and heat transfer properties of rarefied gas in restricted space, the discrete unified gas kinetic scheme (DUGKS) is used to simulate the thermal creep flows in a square cavity. All the boundaries of the cavity are stationary diffuse reflection walls. The temperature of left wall and right wall are lower, and the temperature of the upper wall and the lower wall are both subjected to periodic variation. The simulation parameters considered in the present work are set as follows: the Knudsen number Kn is in a range 0.01–10, temperature change frequency St in a range of 0.5–5, and temperature change amplitude A_hin a range of 0.1–0.8. The results indicate that the velocity field and temperature field in the cavity exhibit periodic variations. No inverse Fourier heat transfer phenomenon is observed within the parameter ranges studied. The intensity of the thermal creep flow can be increased by increasing the frequency, temperature, and the Knudsen number. This can also raise the temperature jump and velocity slip close to the temperature change walls. Heat transfer lag and a reduction in the heat transfer capability of the wall are caused by increasing St and Kn. When St is small, say, St = 0.5, a complex vortex structure is seen in the cavity. As the value of St rises to 5, the vortex disappears, the gas travels from the variable temperature wall to the horizontal centerline of cavity, and the region close to the middle of the left wall and right wall changes from an endothermic zone to an exothermic zone. Furthermore, the temperature field and velocity field inside the cavity hardly change, but the degree of heat transfer on the wall decreases with the increase of A_h. The main results are shown in the figure attached below. This work provides helpful recommendations for designing the MEMS devices by using pulsed heating.

Keywords:

Authors and contacts

Guangdong Provincial Key Laboratory of Distributed Energy Systems, Dongguan University of Technology, Dongguan 523808, China

Corresponding author: TAO Shi, taoshi@dgut.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 52476148, 51906044).

FullText HTML

References

[1]	沈青 2006 力学进展 36 142 Google Scholar Shen Q 2006 Adv. Mech. 36 142 Google Scholar
[2]	Frangi A, Frezzotti A, Lorenzani S 2007 Comput. Struct. 85 810 Google Scholar
[3]	梅涛, 陈占秀, 杨历, 朱洪漫, 苗瑞灿 2020 69 224701 Google Scholar Mei T, Chen Z X, Yang L, Zhu H M, Miao R C 2020 Acta Phys. Sin. 69 224701 Google Scholar
[4]	Ramadan K M, Qisieh O, Tlili I 2022 Proc. Inst. Mech. Eng. Part C 236 5033 Google Scholar
[5]	Mousivand M, Roohi E 2022 Phys. Fluids 34 052002 Google Scholar
[6]	Lan J, Xie J, Ye J, Peng W Z, Jiao X Y 2022 Int. J. Hydrogen Energy 47 19206 Google Scholar
[7]	韩峰, 王晓伟, 张文青, 张世伟, 张志军 2023 真空科学与技术学报 43 238 Google Scholar Han F, Wang X W, Zhang W Q, Zhang S W, Zhang Z J 2023 J. Vac. Sci. Technol. 43 238 Google Scholar
[8]	王晓伟, 张志军, 张文青, 苏天一, 张世伟 2020 真空与低温 26 73 Google Scholar Wang X W, Zhang Z J, Zhang W Q, Su T Y, Zhang S W 2020 Vac. Cryogen 26 73 Google Scholar
[9]	Wu L, Zhang Y H, Li Z H 2017 Sci. Sin. phys. Mech. As. 47 070004 Google Scholar
[10]	Tsimpoukis A, Vasileiadis N, Tatsios G, Valougeorgis D 2019 Phys. Fluids 31 067108 Google Scholar
[11]	Taassob A, Kamali R, Bordbar A 2018 Vacuum 151 197 Google Scholar
[12]	Nabapure D 2021 J. Comput. Sci. Neth. 49 101276.Google Scholar
[13]	Wu L, Reese J M, Zhang Y 2014 J. Fluid Mech. 748 350 Google Scholar
[14]	Ogata Y, Kawaguchi T 2011 J. Fluid Sci. Technol. 6 215 Google Scholar
[15]	Palharini R C, Scanlon T J, White C 2018 Comput. Fluids 165 173 Google Scholar
[16]	Yang W Q, Tang S, Yang H 2019 Appl. Sci. 9 2733 Google Scholar
[17]	单小东, 王沫然 2013 工程热 34 2159 Shan X D, Wang M R 2013 J. Eng. Thermophys. 34 2159
[18]	张帅, 方蜀州, 许阳 2021 推进技术 42 2002 Google Scholar Zhang S, Fang S Z, Xu Y 2021 J. Propul. Technol. 42 2002 Google Scholar
[19]	Zhang J, Yao S Q, Fei F, Ghalambaz M, Wen D S 2020 Phys. Fluids 32 102001 Google Scholar
[20]	Moghadam E Y, Roohi E, Esfahani J A 2014 Vacuum 109 333 Google Scholar
[21]	Yamaguchi H, Perrier P, Ho M T, Méolans J G, Niimi T, Graur I 2016 J. Fluid Mech. 795 690 Google Scholar
[22]	Barbera E, Brini F 2018 Europhys. Lett. 120 34001 Google Scholar
[23]	Akhlaghi H, Roohi E, Stefanov S 2018 Sci. Rep. 8 13533 Google Scholar
[24]	Han Y L 2010 Fluid Dyn. Res. 42 045505 Google Scholar
[25]	Zhu M B, Roohi E, Ebrahimi A 2023 Phys. Fluids 35 052012 Google Scholar
[26]	Roohi E, Shahabi V, Bagherzadeh A 2018 Int. J. Therm. Sci. 125 381 Google Scholar
[27]	Wang P, Zhu L H, Su W, Wu L, Zhang Y H 2018 Phys. Rev. E 97 043103 Google Scholar
[28]	Zhu L H, Guo Z L, Xu K 2016 Comput. Fluids 127 211 Google Scholar
[29]	Wang X W, Su T Y, Zhang W Q, Zhang Z J, Zhang S W 2020 Microsyst. Nanoeng. 6 26 Google Scholar
[30]	张贝豪, 郑林 2020 69 164401 Google Scholar Zhang B H, Zheng L 2020 Acta Phys. Sin. 69 164401 Google Scholar
[31]	Ou Y, Qu F, Wang G Y, Nie M Y, Li Z G, Ou W, Xie C Q 2016 Appl. Phys. Lett. 109 023512 Google Scholar
[32]	万启坤, 张月, 郭照立 2023 计算物理 40 653 Wan Q K, Zhang Y, Guo Z L 2023 Chinese J. Comput. Phys. 40 653
[33]	Kalempa D, Sharipov F, Silva J C 2019 Vacuum 159 82 Google Scholar
[34]	Bargatin I, Kozinsky I, Roukes M L 2007 Appl. Phys. Lett. 90 093116 Google Scholar
[35]	Ilic B, Yang Y, Aubin K, Reichenbach R, Krylov S, Craighead H G 2005 Nano Lett. 5 925 Google Scholar
[36]	Juvé V, Crut A, Maioli P, Pellarin M, Broyer M, Del Fatti N, Vallée F 2010 Nano Lett. 10 1853 Google Scholar
[37]	Guo Z L, Wang R J, Xu K 2015 Phys. Rev. E 91 033313 Google Scholar
[38]	孙喜明, 姚朝晖, 杨京龙 2002 51 1942 Google Scholar Sun X M, Yao Z H, Yang J L 2002 Acta Phys. Sin. 51 1942 Google Scholar
[39]	孙佳坤, 林传栋, 苏咸利, 谭志城, 陈亚楼, 明平剑 2024 73 110504 Google Scholar Sun J K, Lin C D, Su X L, Tan Z C, Chen Y L, Ming P J 2024 Acta Phys. Sin. 73 110504 Google Scholar
[40]	Huang J C, Xu K, Yu P 2013 Commun. Comput. Phys. 14 1147 Google Scholar
[41]	Wang Y, Zhong C W, Liu S 2019 Phys. Rev. E 100 063310 Google Scholar
[42]	Zhu L H, Chen S Z, Guo Z L 2017 Comput. Phys. Commun. 213 155 Google Scholar
[43]	Vargas M, Tatsios G, Valougeorgis D, Stefanov S 2014 Phys. Fluids 26 057101 Google Scholar

Cited By

图 1 变温壁面驱动方腔流动示意图

Figure 1. Schematic diagram of the flow of a variable temperature wall driven cavity.

DownLoad: Full-Size Img PowerPoint

图 2 Kn = 0.1时的腔内温度云图和速度场流线结果对比　(a)文献[42]结果; (b)本文结果

Figure 2. Comparison of the temperature contour and velocity field streamlines in the cavity at Kn = 0.1: (a) Results of Ref. [42]; (b) the results of this work.

DownLoad: Full-Size Img PowerPoint

图 3 通过左侧一级主涡中心的水平和竖直线上的U和V速度分布

Figure 3. U and V velocity distribution in horizontal and vertical lines through the center of the primary vortex on the left.

DownLoad: Full-Size Img PowerPoint

图 4 Kn = 1, A_h = 0.5, t = 0时, 不同St下方腔内温度场和热流线　(a) St = 0.5; (b) St = 1.0; (c) St = 2.0; (d) St = 5.0

Figure 4. Temperature field and thermal flow lines in different cavities under different St when Kn = 1, A_h = 0.5 and t = 0: (a) St = 0.5; (b) St = 1.0; (c) St = 2.0; (d) St = 5.0.

DownLoad: Full-Size Img PowerPoint

图 5 Kn = 1, A_h = 0.5, t = 0时不同频率St下中心线上的温度分布　(a)竖直中心线X/L = 0.5; (b): 水平中心线Y/L = 0.5

Figure 5. Temperature distribution on the centerline at different frequencies St when Kn = 1, A_h = 0.5 and t = 0: (a) Vertical centerline X/L = 0.5; (b) horizontal centerline Y/L = 0.5.

DownLoad: Full-Size Img PowerPoint

图 6 Kn = 1, A_h = 0.5, t = 0时不同频率St下方腔内速度云图和流线　(a) St = 0.5; (b) St = 1.0; (c) St = 2.0; (d) St = 5.0

Figure 6. Intracavity velocity contours and streamlines below St at different frequencies when Kn = 1, A_h = 0.5 and t = 0: (a) St = 0.5; (b) St = 1.0; (c) St = 2.0; (d) St = 5.0.

DownLoad: Full-Size Img PowerPoint

图 7 Kn = 1, A_h = 0.5, t = 0时不同St时左、上壁面处气体温度T、努塞尔数Nu和速度U分布

Figure 7. Distributions of gas temperature T, Nusselt number Nu and velocity U on the left and upper walls at different St when Kn = 1, A_h = 0.5 and t = 0.

DownLoad: Full-Size Img PowerPoint

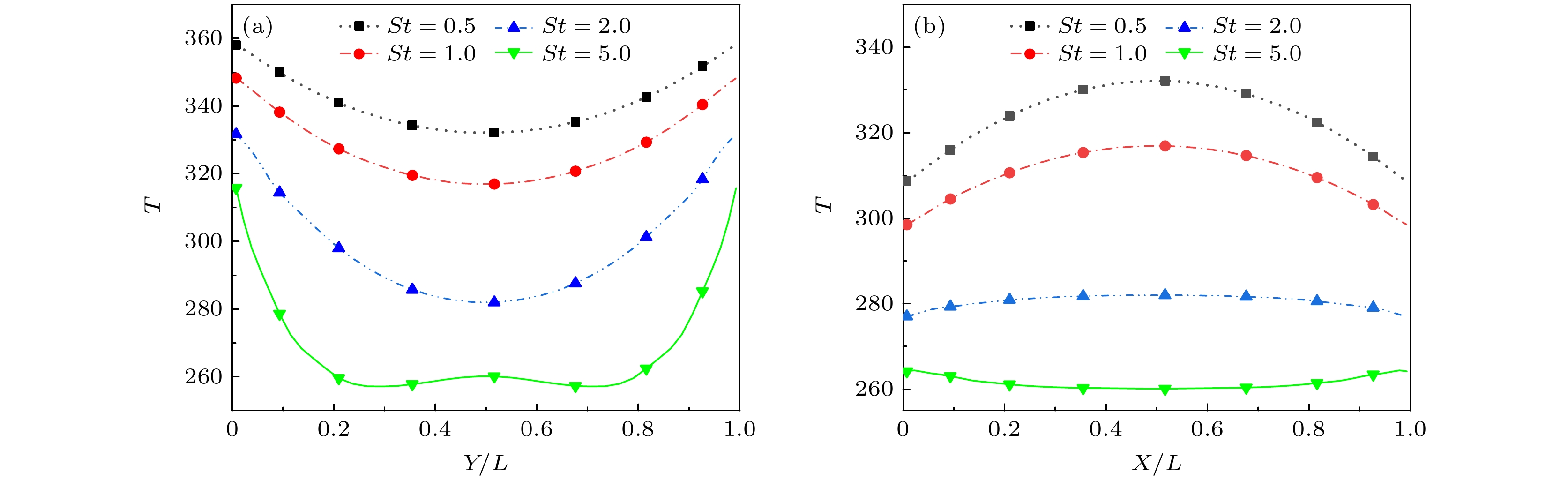
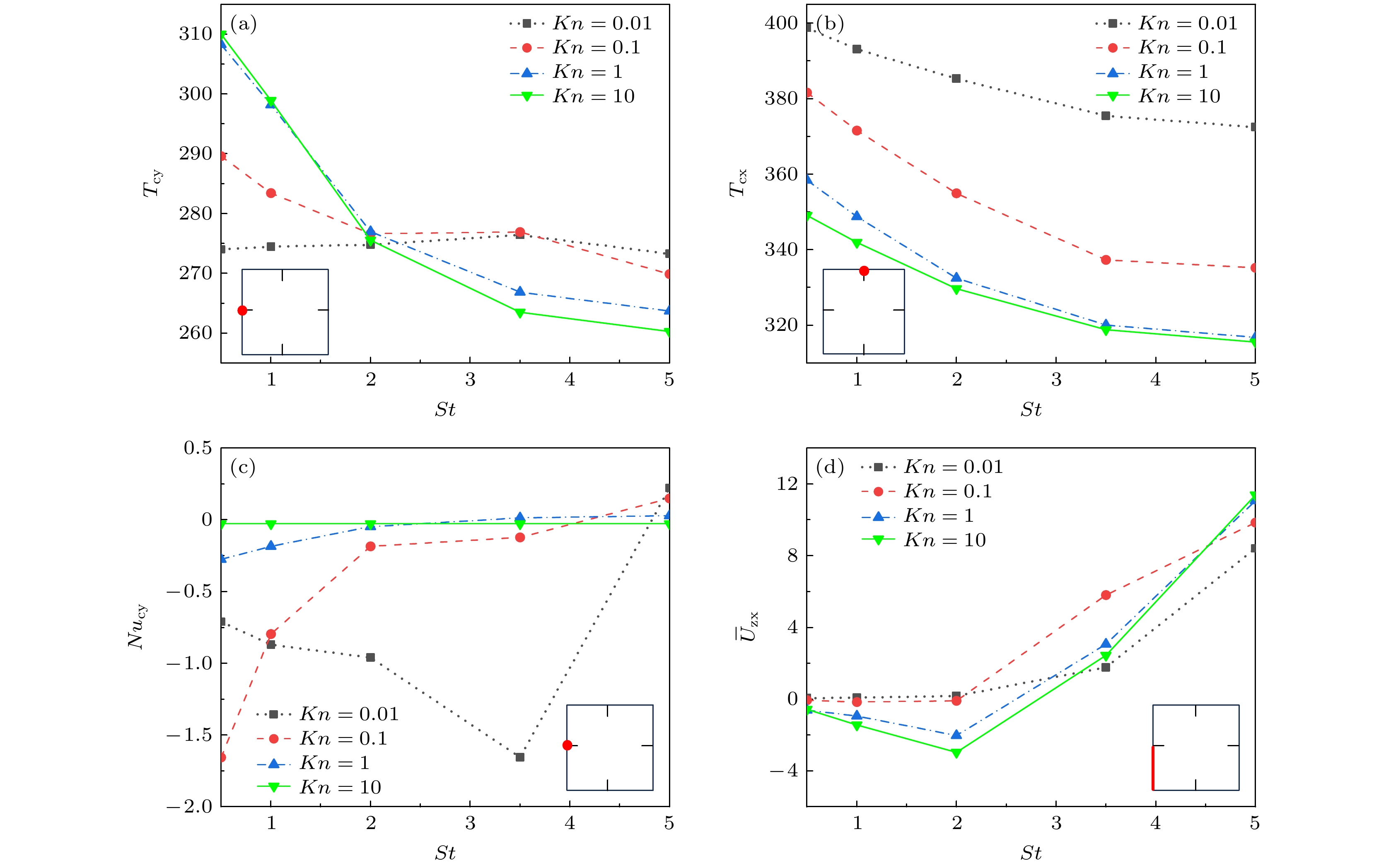
图 8 A_h = 0.5, t = 0时不同参数在不同St和Kn下的变化　(a)左壁面中点温度T_cy; (b)上壁面中点温度T_cx; (c)左壁面中点努塞尔数Nu_cy; (d)左壁面下半部分平均速度$ {\overline{U}}_{{\mathrm{z}}{\mathrm{x}}} $

Figure 8. Variations of different parameters under different St and Kn when A_h = 0.5 and t = 0: (a) Temperature of the midpoint of the left wall T_cy; (b) temperature of the midpoint of the upper wall T_cx; (c) Nussel number of the midpoint of the left wall Nu_cy; (d) the average velocity of the lower half of the left wall $ {\overline{U}}_{{\mathrm{z}}{\mathrm{x}}} $.

DownLoad: Full-Size Img PowerPoint

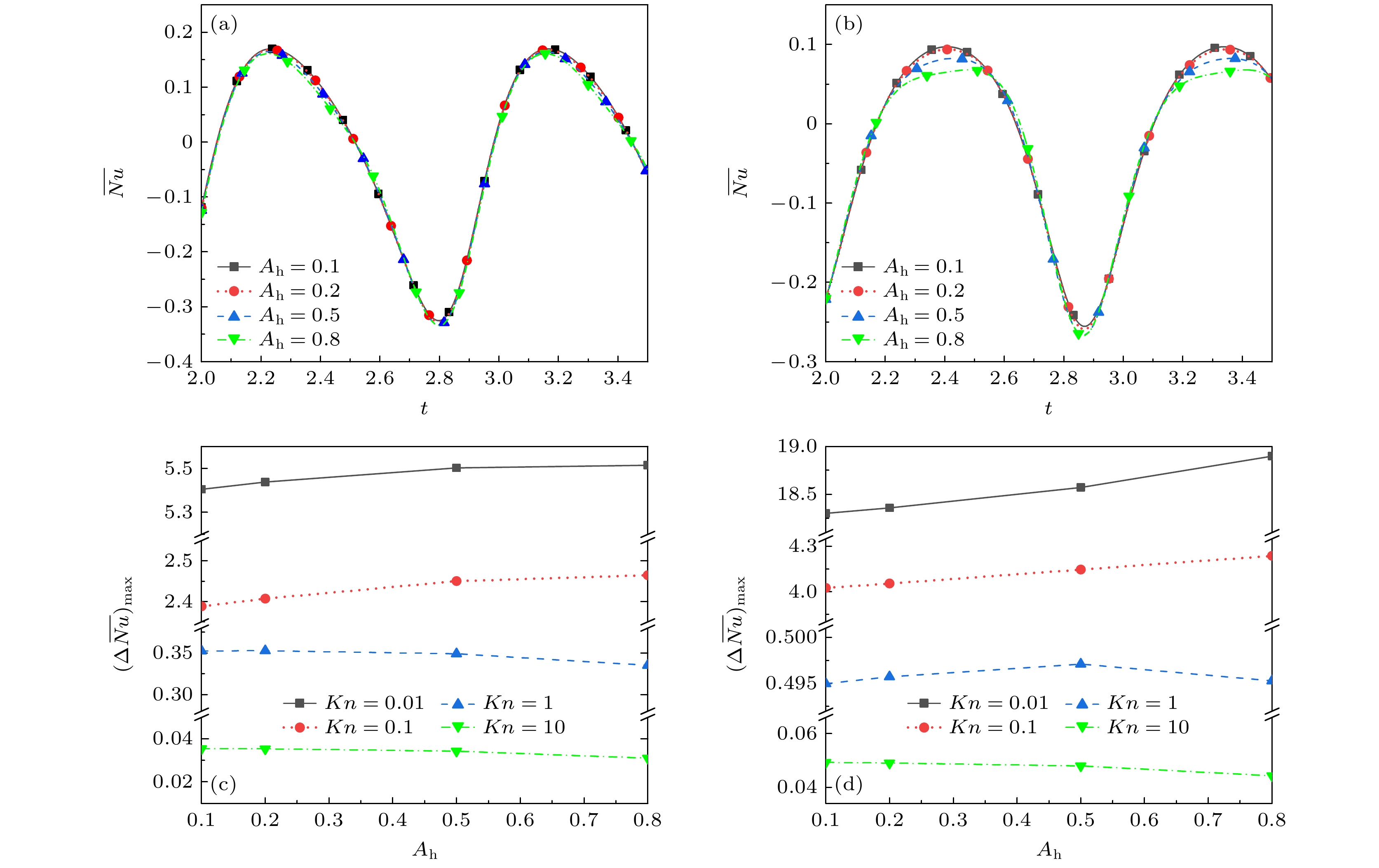
图 9 不同St和Kn下, 壁面平均努塞尔数$\overline{Nu} $的时间历程及$\overline{Nu} $的极差$ {\left(\Delta\overline{Nu}\right)}_{{\mathrm{m}}{\mathrm{a}}{\mathrm{x}}} $　(a), (c) Y = 0, 下壁面; (b), (d) X = 0, 左壁面

Figure 9. The time history of the average Nussel number $\overline{Nu} $ and the range $ {\left(\Delta\overline{Nu}\right)}_{{\mathrm{m}}{\mathrm{a}}{\mathrm{x}}} $of $\overline{Nu} $ on the wall under different St and Kn: (a), (c) Lower wall surface, Y = 0; (b), (d) left wall, X = 0.

DownLoad: Full-Size Img PowerPoint

图 10 A_h = 0.2, Kn = 1, St = 1.0, t = 0时方腔内的温度场和热流线(a)、速度场和流线(b)

Figure 10. Temperature field and thermal streamlines (a), velocity field and streamlines (b) in a square cavity at Kn = 1, St = 1.0, A_h = 0.2 and t = 0.

DownLoad: Full-Size Img PowerPoint

图 11 Kn = 1, St = 1.0, t = 0时不同振幅A_h的中心线上温度分布　(a)竖直中心线X/L = 0.5; (b)水平中心线Y/L = 0.5

Figure 11. Temperature distribution on the centerline of A_h with different amplitudes when Kn = 1, St = 1.0 and t = 0: (a) Vertical centerline X/L = 0.5; (b) horizontal centerline Y/L = 0.5.

DownLoad: Full-Size Img PowerPoint

图 12 Kn = 1, St = 1.0, t = 0时不同A_h时左、上壁面处气体温度T, 努塞尔数Nu和速度U分布

Figure 12. Distribution of gas temperature T, Nusselt number Nu and velocity U on the left and upper wall surfaces at different A_h when Kn = 1, St = 1.0 and t = 0.

DownLoad: Full-Size Img PowerPoint

图 13 St = 1.0, t = 0时, 不同参数在不同A_h和Kn下的变化　(a)左壁面中点温度T_cy; (b)上壁面中点温度T_cx; (c)左壁面中点努塞尔数Nu_cy; (d)左下半壁面平均速度$ {\overline{U}}_{{\mathrm{z}}{\mathrm{x}}} $

Figure 13. Variations of different parameters under different A_h and Kn when St = 1.0 and t = 0: (a) The midpoint temperature of the left wall T_cy; (b) temperature of the midpoint of the upper wall T_cx; (c) Nusselt number of the left wall midpoint Nu_cy; (d) the average velocity of the lower left half of the wall $ {\overline{U}}_{{\mathrm{z}}{\mathrm{x}}} $.

DownLoad: Full-Size Img PowerPoint

图 14 Kn = 10, St = 1.0, t = 0时, 不同A_h下方腔内温度云图和流线　(a) A_h = 0.6; (b) A_h = 0.7; (c) A_h = 0.8

Figure 14. Temperature cloud maps and streamlines in the cavity with different A_h values at Kn = 10, St = 1.0, and t = 0: (a) A_h = 0.6; (b) A_h = 0.7; (c) A_h = 0.8.

DownLoad: Full-Size Img PowerPoint

图 15 St = 1.0时不同A_h和Kn下, 壁面平均努塞尔数$\overline{Nu} $的时间历程及$\overline{Nu} $的极差$ {\left(\Delta\overline{Nu}\right)}_{{\mathrm{m}}{\mathrm{a}}{\mathrm{x}}} $　(a), (c) X = 0, 左壁面; (b), (d) Y= 0, 下壁面

Figure 15. The time history of the average Nussel number $\overline{Nu} $ and the range $ {\left(\Delta\overline{Nu}\right)}_{{\mathrm{m}}{\mathrm{a}}{\mathrm{x}}} $ of $\overline{Nu} $ on the wall under different A_h and Kn when St = 1.0: (a), (c) Left wall, X = 0; (b), (d) lower wall surface, Y = 0.

DownLoad: Full-Size Img PowerPoint

map

[1]	沈青 2006 力学进展 36 142 Google Scholar Shen Q 2006 Adv. Mech. 36 142 Google Scholar
[2]	Frangi A, Frezzotti A, Lorenzani S 2007 Comput. Struct. 85 810 Google Scholar
[3]	梅涛, 陈占秀, 杨历, 朱洪漫, 苗瑞灿 2020 69 224701 Google Scholar Mei T, Chen Z X, Yang L, Zhu H M, Miao R C 2020 Acta Phys. Sin. 69 224701 Google Scholar
[4]	Ramadan K M, Qisieh O, Tlili I 2022 Proc. Inst. Mech. Eng. Part C 236 5033 Google Scholar
[5]	Mousivand M, Roohi E 2022 Phys. Fluids 34 052002 Google Scholar
[6]	Lan J, Xie J, Ye J, Peng W Z, Jiao X Y 2022 Int. J. Hydrogen Energy 47 19206 Google Scholar
[7]	韩峰, 王晓伟, 张文青, 张世伟, 张志军 2023 真空科学与技术学报 43 238 Google Scholar Han F, Wang X W, Zhang W Q, Zhang S W, Zhang Z J 2023 J. Vac. Sci. Technol. 43 238 Google Scholar
[8]	王晓伟, 张志军, 张文青, 苏天一, 张世伟 2020 真空与低温 26 73 Google Scholar Wang X W, Zhang Z J, Zhang W Q, Su T Y, Zhang S W 2020 Vac. Cryogen 26 73 Google Scholar
[9]	Wu L, Zhang Y H, Li Z H 2017 Sci. Sin. phys. Mech. As. 47 070004 Google Scholar
[10]	Tsimpoukis A, Vasileiadis N, Tatsios G, Valougeorgis D 2019 Phys. Fluids 31 067108 Google Scholar
[11]	Taassob A, Kamali R, Bordbar A 2018 Vacuum 151 197 Google Scholar
[12]	Nabapure D 2021 J. Comput. Sci. Neth. 49 101276.Google Scholar
[13]	Wu L, Reese J M, Zhang Y 2014 J. Fluid Mech. 748 350 Google Scholar
[14]	Ogata Y, Kawaguchi T 2011 J. Fluid Sci. Technol. 6 215 Google Scholar
[15]	Palharini R C, Scanlon T J, White C 2018 Comput. Fluids 165 173 Google Scholar
[16]	Yang W Q, Tang S, Yang H 2019 Appl. Sci. 9 2733 Google Scholar
[17]	单小东, 王沫然 2013 工程热 34 2159 Shan X D, Wang M R 2013 J. Eng. Thermophys. 34 2159
[18]	张帅, 方蜀州, 许阳 2021 推进技术 42 2002 Google Scholar Zhang S, Fang S Z, Xu Y 2021 J. Propul. Technol. 42 2002 Google Scholar
[19]	Zhang J, Yao S Q, Fei F, Ghalambaz M, Wen D S 2020 Phys. Fluids 32 102001 Google Scholar
[20]	Moghadam E Y, Roohi E, Esfahani J A 2014 Vacuum 109 333 Google Scholar
[21]	Yamaguchi H, Perrier P, Ho M T, Méolans J G, Niimi T, Graur I 2016 J. Fluid Mech. 795 690 Google Scholar
[22]	Barbera E, Brini F 2018 Europhys. Lett. 120 34001 Google Scholar
[23]	Akhlaghi H, Roohi E, Stefanov S 2018 Sci. Rep. 8 13533 Google Scholar
[24]	Han Y L 2010 Fluid Dyn. Res. 42 045505 Google Scholar
[25]	Zhu M B, Roohi E, Ebrahimi A 2023 Phys. Fluids 35 052012 Google Scholar
[26]	Roohi E, Shahabi V, Bagherzadeh A 2018 Int. J. Therm. Sci. 125 381 Google Scholar
[27]	Wang P, Zhu L H, Su W, Wu L, Zhang Y H 2018 Phys. Rev. E 97 043103 Google Scholar
[28]	Zhu L H, Guo Z L, Xu K 2016 Comput. Fluids 127 211 Google Scholar
[29]	Wang X W, Su T Y, Zhang W Q, Zhang Z J, Zhang S W 2020 Microsyst. Nanoeng. 6 26 Google Scholar
[30]	张贝豪, 郑林 2020 69 164401 Google Scholar Zhang B H, Zheng L 2020 Acta Phys. Sin. 69 164401 Google Scholar
[31]	Ou Y, Qu F, Wang G Y, Nie M Y, Li Z G, Ou W, Xie C Q 2016 Appl. Phys. Lett. 109 023512 Google Scholar
[32]	万启坤, 张月, 郭照立 2023 计算物理 40 653 Wan Q K, Zhang Y, Guo Z L 2023 Chinese J. Comput. Phys. 40 653
[33]	Kalempa D, Sharipov F, Silva J C 2019 Vacuum 159 82 Google Scholar
[34]	Bargatin I, Kozinsky I, Roukes M L 2007 Appl. Phys. Lett. 90 093116 Google Scholar
[35]	Ilic B, Yang Y, Aubin K, Reichenbach R, Krylov S, Craighead H G 2005 Nano Lett. 5 925 Google Scholar
[36]	Juvé V, Crut A, Maioli P, Pellarin M, Broyer M, Del Fatti N, Vallée F 2010 Nano Lett. 10 1853 Google Scholar
[37]	Guo Z L, Wang R J, Xu K 2015 Phys. Rev. E 91 033313 Google Scholar
[38]	孙喜明, 姚朝晖, 杨京龙 2002 51 1942 Google Scholar Sun X M, Yao Z H, Yang J L 2002 Acta Phys. Sin. 51 1942 Google Scholar
[39]	孙佳坤, 林传栋, 苏咸利, 谭志城, 陈亚楼, 明平剑 2024 73 110504 Google Scholar Sun J K, Lin C D, Su X L, Tan Z C, Chen Y L, Ming P J 2024 Acta Phys. Sin. 73 110504 Google Scholar
[40]	Huang J C, Xu K, Yu P 2013 Commun. Comput. Phys. 14 1147 Google Scholar
[41]	Wang Y, Zhong C W, Liu S 2019 Phys. Rev. E 100 063310 Google Scholar
[42]	Zhu L H, Chen S Z, Guo Z L 2017 Comput. Phys. Commun. 213 155 Google Scholar
[43]	Vargas M, Tatsios G, Valougeorgis D, Stefanov S 2014 Phys. Fluids 26 057101 Google Scholar

[1]	LUO Shichao, WU Liyin, HU Shouchao, GONG Hongming, LYU Minglei, KONG Xiaoping. Analysis of wall catalytic effects on magnetohydrodynamic control of high-temperature non-quilibrium flow field. Acta Physica Sinica, 2025, 74(2): 024701. doi: 10.7498/aps.74.20241307
[2]	Guo Yi-Feng, Wang Zhi-Bin, Jia Li-Si, Mo Song-Ping, Chen Ying. Numerical simulation of inhibition characteristics of wall temperature rise of phase change microcapsule in liquid-cooled microchannel. Acta Physica Sinica, 2023, 72(10): 106501. doi: 10.7498/aps.72.20222400
[3]	Chen Jiang-Li, Chen Shao-Qiang, Ren Feng, Hu Hai-Bao. Artificially intelligent control of drag reduction around a circular cylinder based on wall pressure feedback. Acta Physica Sinica, 2022, 71(8): 084701. doi: 10.7498/aps.71.20212171
[4]	Lu Chang-Gen, Shen Lu-Yu, Zhu Xiao-Qing. Numerical study of effect of pressure gradient on boundary-layer receptivity under localized wall blowing/suction. Acta Physica Sinica, 2019, 68(22): 224701. doi: 10.7498/aps.68.20190684
[5]	Wang Ru-Jia, Wu Shi-Ping, Chen Wei. Propagation of thermoviscoelastic wave in inhomogeneous alloy melt with varying temperature. Acta Physica Sinica, 2019, 68(4): 048101. doi: 10.7498/aps.68.20181923
[6]	He Ju-Sheng, Zhang Meng, Pan Hua-Qing, Zou Ji-Jun, Qi Wei-Jing, Li Ping. Determination of dislocation density of a class of n-GaN based on the variable temperature Hall-effect method. Acta Physica Sinica, 2017, 66(6): 067201. doi: 10.7498/aps.66.067201
[7]	Chang Song-Tao, Tian Qi-Jie, He Feng-Yun, Yu Yi, Li Zhou. Design of varying f/number of cooled infrared detectors based on spherical reflecting warm shield. Acta Physica Sinica, 2017, 66(15): 150701. doi: 10.7498/aps.66.150701
[8]	Lu Chang-Gen, Shen Lu-Yu. Numerical study on boundary-layer receptivity with localized wall blowing/suction. Acta Physica Sinica, 2015, 64(22): 224702. doi: 10.7498/aps.64.224702
[9]	Chen Ying, Fu Shi-Xiao, Xu Yu-Wang, Zhou Qing, Fan Di-Xia. Hydrodynamic characters of a near-wall circular cylinder oscillating in cross flow direction in steady current. Acta Physica Sinica, 2013, 62(6): 064701. doi: 10.7498/aps.62.064701
[10]	Zhao Min-Jie, Si Fu-Qi, Lu Yi-Huai, Wang Shi-Mei, Jiang Yu, Zhou Hai-Jin, Liu Wen-Qing. Experimental measurement of aluminium diffuser applied to calibration system of space-borne differential optical absorption spectrometer. Acta Physica Sinica, 2013, 62(24): 249301. doi: 10.7498/aps.62.249301
[11]	Tian Hui-Juan, Niu Ping-Juan. Sensitivity of delta-P1 approximation model to the reduced scattering parameter. Acta Physica Sinica, 2013, 62(3): 034201. doi: 10.7498/aps.62.034201
[12]	Zhang Xiao-Juan, Zhou Qing-Jun, Yang Wei. Study of SP3 for spatially-resolved diffuse reflectance close to light source. Acta Physica Sinica, 2012, 61(3): 034202. doi: 10.7498/aps.61.034202
[13]	Lan Zhong, Xu Wei, Zhu Xia, Ma Xue-Hu. Reflection spectrum analysis of dropwise condensation with the clustering model. Acta Physica Sinica, 2011, 60(12): 120508. doi: 10.7498/aps.60.120508
[14]	Liu Ying, Wang Li-Jun, Guo Yun-Feng, Zhang Xiao-Juan, Gao Zong-Hui, Tian Hui-Juan. Sensitivity of spatially-resolved diffuse reflectance to high-order optical parameters. Acta Physica Sinica, 2007, 56(4): 2119-2123. doi: 10.7498/aps.56.2119
[15]	Xue Xiao-Bo, Yao Zhao-Hui, He Feng. Information preservation method for micro-scale flow with temperature variation. Acta Physica Sinica, 2006, 55(3): 1276-1282. doi: 10.7498/aps.55.1276
[16]	YOU MING-CHANG, ZHANG SHU-AN. ANALYTICAL DEVELOPMENT OF AN APPROXIMATE EXPRESSION OF THE DIFFUSE REFLECTANCE JUST BENEATH THE SEA SURFACE. Acta Physica Sinica, 1994, 43(4): 683-688. doi: 10.7498/aps.43.683
[17]	FAN TIAN-YOU, LIANG ZHEN-YA. A POSSIBLE MECHANISM OF THE GROWTH OF GRAIN-BOUNDARY CRACK AND AN ESTIMATION OF CREEP RUPTURE TIME. Acta Physica Sinica, 1978, 27(3): 269-275. doi: 10.7498/aps.27.269
[18]	CHEN CHI, DENG ZHI-SHENG, WU BAI-QUN, DING SHU-SHENG. THE CREEP AND STRESS-RUPTURE PROPERTIES OF γ′ CRYSTALS. Acta Physica Sinica, 1974, 23(1): 69-76. doi: 10.7498/aps.23.69
[19]	. . Acta Physica Sinica, 1965, 21(12): 2030-2032. doi: 10.7498/aps.21.2030
[20]	LIN HUNG-SUN. ON THE GAS FLOW AND HEAT TRANSFER IN LAMINAR BOUNDARY LAYER FLOW. Acta Physica Sinica, 1954, 10(1): 71-88. doi: 10.7498/aps.10.71

Catalog

Vol. 74, Issue 4 - 2025-02-20

Metrics

Abstract views: 471
PDF Downloads: 17
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板