-
The significant potential applications of Majorana bound state (MBS) in topological quantum computing manifest the importance and necessity of relevant in-depth research. To understand the physical properties of MBS, the most practical approach is to integrate it to a mesoscopic circuit and then investigate its quantum transport behaviors. In this work, we investigate the transport properties in the systems with MBS, and provide theoretical support for its further understanding and detection, by utilizing the nonequilibrium Green’s function method and scattering matrix theory. Specifically, we investigate theoretically the transport properties in a T-shaped double-quantum dot structure, by considering MBS to be coupled to the dot in the main channel, which shows that in the linear transmission region, when the level of side-coupled dot is tuned to the Fermi energy level, the contribution of MBS to the conductance is eliminated under weak and strong Coulomb interaction. The side-coupled dot is far away from the Fermi energy level, leading to different results. When Majorana zero mode is added, the linear conductance is independent of the level of the side-coupled quantum dot, and the conductance plateau appears. However, with coupling between the MBSs, the linear conductance is the same as that without coupling between the MBSs. The decoupling phenomenon of the MBS remains strong. Therefore, the signature of the MBS can be eliminated by adjusting the level of the side-coupled quantum dot or the inter-MBS coupling. When ferromagnetic leads are introduced, the appearance or disappearance of the conductance plateau is clearly dependent on the difference between the magnetic field direction and the lead polarization direction in the system, whereas the decoupling behavior of the MBS is still existent. This work contributes to further explaining the decoupling phenomenon of MBSs in a T-shaped double-quantum-dot system, and presents a theoretical approach to more in-depth understanding and detection of the MBS.
-
Keywords:
- Andreev reflection /
- Majorana bound state /
- quantum dot /
- decoupling
[1] Hyart T, van Heck B, Fulga I C, Burrello M, Akhmerov A R, Beenakker C W J 2013 Phys. Rev. B 88 035121
Google Scholar
[2] Reimann S M, Manninen M 2002 Rev. Mod. Phys. 74 1283
Google Scholar
[3] van der Wiel W G, De Franceschi S, Elzerman J M, Fujisawa T, Tarucha S, Kouwenhoven L P 2002 Rev. Mod. Phys. 75 1
Google Scholar
[4] Meir Y, Wingreen N S, Lee P A 1993 Phys. Rev. Lett. 70 2601
Google Scholar
[5] Zheng Y S, Lü T Q, Zhang C X, Su W H 2004 Physica E 24 290
Google Scholar
[6] Gong W J, Zheng Y S, Liu Y, Lü T Q 2006 Phys. Rev. B 73 245329
Google Scholar
[7] Sato M, Aikawa H, Kobayashi K, Katsumoto S, Iye Y 2005 Phys. Rev. Lett. 95 066801
Google Scholar
[8] Žitko R, Bonča J 2007 Phys. Rev. B 76 241305
Google Scholar
[9] Miroshnichenko A E, Flach S, Kivshar Y S 2010 Rev. Mod. Phys. 82 2257
Google Scholar
[10] Lee W R, Kimand J U, Sim H S 2008 Phys. Rev. B 77 033305
Google Scholar
[11] Michalek G, Bulka B R 2022 J. Magn. Magn. Mater. 544 168700
Google Scholar
[12] Ding G H, Kim C K, Nahm K 2005 Phys. Rev. B 71 205313
Google Scholar
[13] Eto M, Sakano R 2020 Phys. Rev. B 102 245402
Google Scholar
[14] Akera H 1993 Phys. Rev. B 47 6835
Google Scholar
[15] Brandes T, Kramer B 1999 Phys. Rev. Lett. 83 3021
Google Scholar
[16] Głodzik S, Wójcik K P, Weymann I, Domański T 2017 Phys. Rev. B 95 125419
Google Scholar
[17] Monteros A L, Uppal G S, McMillan S R, Crisan M, Tifrea I 2014 Eur. Phys. J. B 87 302
Google Scholar
[18] Piotr M, Wójcik K P, Weymann I 2022 Phys. Rev. B 105 075418
Google Scholar
[19] Wójcik K P, Weymann I 2014 Phys. Rev. B 90 115308
Google Scholar
[20] Wójcik K P, Weymann I 2015 Phys. Rev. B 91 134422
Google Scholar
[21] Mourik V, Zuo Z, Frolov S M, Plissard S R, Bakkers E P A M, Kouwenhoven L P 2012 Science 336 1003
Google Scholar
[22] Wang D F, Kong L Y, Fan P, Chen H, Zhu S Y, Liu W Y, Cao L, Sun Y J, Du S X, Schneeloch J, Zhong R D, Gu G, Fu L, Ding H, Gao H J 2018 Science 362 333
Google Scholar
[23] Stern A 2010 Nature 464 187
Google Scholar
[24] Chiu C K, Machida T, Huang Y, Hanaguri T, Zhang F C 2020 Sci. Adv. 6 eaay0443
Google Scholar
[25] Žitko R 2011 Phys. Rev. B 83 195137
Google Scholar
[26] Smirnov S 2022 Phys. Rev. B 105 205430
Google Scholar
[27] Máthé L, Sticlet D, Zârbo L P 2022 Phys. Rev. B 105 155409
Google Scholar
[28] Liu D E, Baranger H U 2011 Phys. Rev. B 84 201308
Google Scholar
[29] Gong W J, Zhang S F, Li Z C, Yi G Y, Zheng Y S 2014 Phys. Rev. B 89 245413
Google Scholar
[30] Gong W J, Zhao Y, Gao Z 2015 Curr. Appl. Phys. 15 520
Google Scholar
[31] Liu J, Wang J, Zhang F C 2014 Phys. Rev. B 90 035307
Google Scholar
[32] Zocher B, Rosenow B 2013 Phys. Rev. Lett. 111 036802
Google Scholar
[33] Gong T, Zhang L L, Dai X F, Jiang C, Gong W J 2022 Eur. Phys. J. Plus 137 122
Google Scholar
[34] Gao Z, Gong W J 2016 Phys. Rev. B 94 104506
Google Scholar
[35] Cheng M, Becker M, Bauer B, Bauer B, Lutchyn R M 2014 Phys. Rev. X 4 031051
[36] Weymann I, Wójcik K P, Majek P 2020 Phys. Rev. B 101 235404
Google Scholar
[37] Calle A M, Pacheco M, Orellana P A, Otálora J A 2020 Ann. Phys. 532 1900409
Google Scholar
[38] Wang X Q, Zhang S F, Han Y, Gong W J 2019 Phys. Rev. B 100 115405
Google Scholar
[39] Ramos-Andrade J P, Peña F J, González A, Ávalos-Ovando O, Orellana P A 2017 Phys. Rev. B 96 165413
Google Scholar
[40] Žitko R, Bonča J 2008 Phys. Rev. B 77 245112
Google Scholar
[41] Žitko R, 2011 Comput. Phys. Commun. 182 2259
Google Scholar
[42] Liu Y, Zheng Y S, Gong W J, Lü T Q 2007 Phys. Rev. B 75 195316
Google Scholar
-
图 1 (a) T形双量子点结构示意图. 主通道中量子点侧耦合马约拉纳束缚态(标为$ \eta_1 $), 马约拉纳束缚态出现在受纵向磁场和超导邻近效应影响且具有强自旋-轨道相互作用的纳米线的末端. (b) 普通电极条件下, Nambu表象中T形双量子点结构自旋向上示意图. 量子点的电子、空穴以及马约拉纳束缚态部分用不同的颜色表示
Figure 1. (a) Schematic of a T-shaped double-quantum-dot (TDQD) structure with the quantum dot (QD) in the main channel coupling to the first Majorana bound state (MBS) which is labeled as $ \eta_1 $. The MBSs are assumed to generate at the ends of the one-dimensional nanowire with strong spin-orbit interaction which suffers from longitudinal magnetic field and superconducting proximity effect. (b) Spin-up illustration of our considered TDQDs in the Nambu representation. The electron and hole parts of the QDs and the MBS part are colored differently for comparison.
图 2 不同条件下的线性电导曲线 (a)下自旋的电导; (b)—(d) $ \varepsilon_{\rm m}=0 $, 0.1, 0.5时上自旋的电导
Figure 2. Linear-conductance curves in different cases: (a) Spin-down result of the linear conductance; (b)–(d) spin-up conductance in the cases of $ \varepsilon_{\rm m}=0 $, 0.1, 0.5.
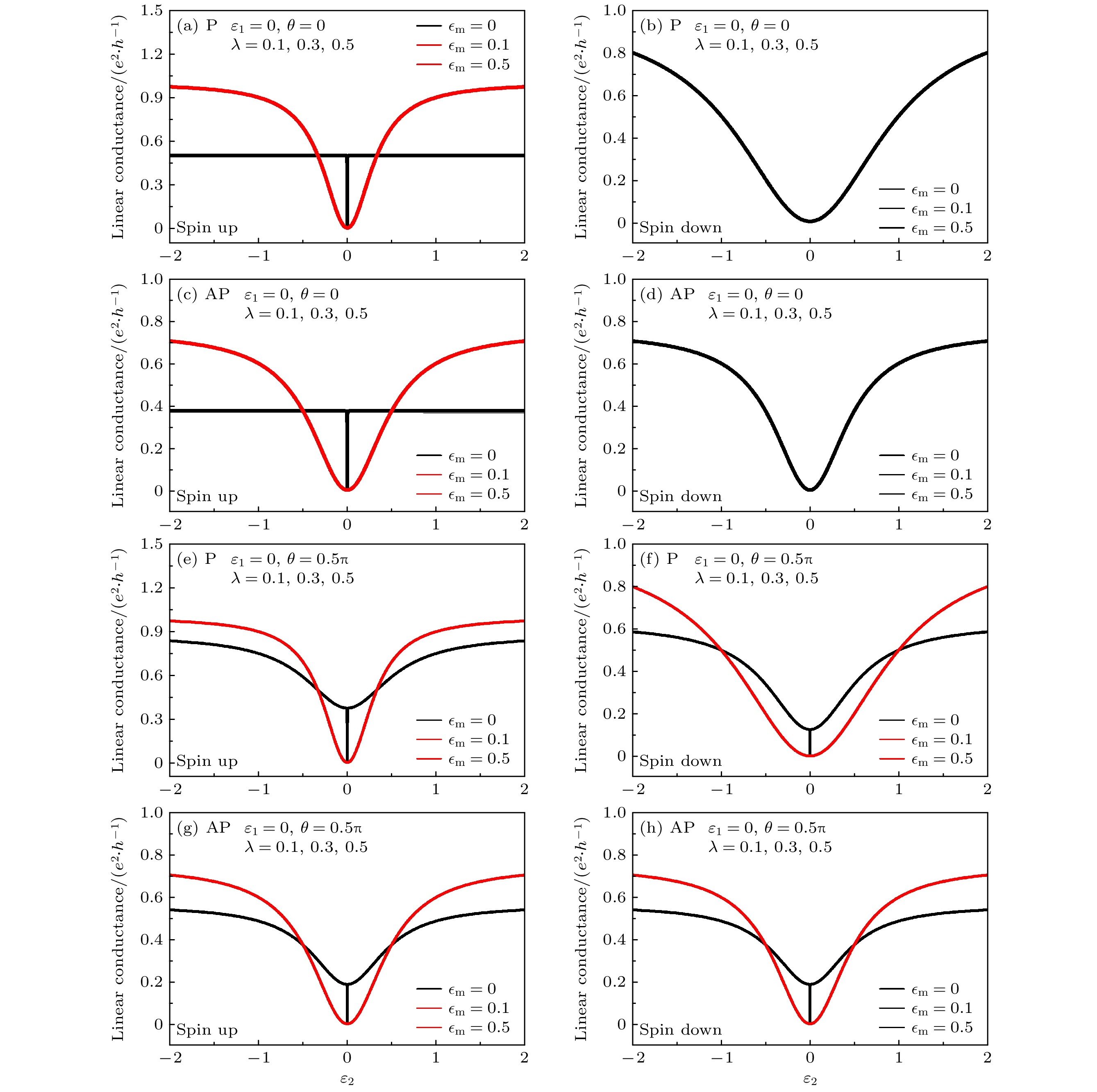
图 3 $ \varepsilon_1=0 $时不同参数取值下的铁磁性电极体系的线性电导谱 (a), (b) $ \theta=0 $时的自旋平行结构线性电导谱; (c), (d) $ \theta=0 $时的自旋反平行结构线性电导谱; (e), (f) $ \theta=0.5\pi $时的自旋平行结构线性电导谱; (g), (h) $ \theta=0.5\pi $时的自旋反平行结构线性电导谱
Figure 3. Linear conductance spectra of the TDQD system with ferromagnetic leads when $ \varepsilon_1=0 $: (a), (b) Linear conductances in the P spin configuration with $ \theta=0 $; (c), (d) linear conductances in the AP spin configuration with $ \theta=0 $; (e), (f) linear conductances in the P spin configuration with $ \theta=0.5\pi $; (g), (h) linear conductance in the AP spin configurations with $ \theta=0.5\pi $.
图 4 $ \varepsilon_1=0 $, $ \lambda=0.1 $时铁磁性电极条件下T 型双量子点体系的磁电阻 (a) $ \varepsilon_{\rm m}=0 $时的磁电阻; (b) $ \varepsilon_{\rm m}=0.1 $时的磁电阻
Figure 4. Magnetoresistance (MR) of the TDQD system with ferromagnetic leads when $ \varepsilon_1=0 $, $ \lambda=0.1 $: (a) MR in the case of $ \varepsilon_{\rm m}=0 $; (b) MR in the case of $ \varepsilon_{\rm m}=0.1 $.
图 5 Nambu表象中耦合铁磁性电极的T形双量子点结构示意图. 量子点的电子、空穴以及马约拉纳束缚态部分用不同的颜色表示
Figure 5. Illustration of our considered TDQDs with ferromagnetic leads in the Nambu representation. The electron and hole parts of the QDs and the MBS part are colored differently for comparison.
图 6 $ \varepsilon_1=0 $, $ \varepsilon_{\rm m}=0 $, $ U_2=0.1 $, 0.2, 0.5时铁磁性电极体系中存在库仑相互作用时的线性电导谱线 (a), (b) $ \theta=0 $ 时自旋平行结构线性电导谱; (c), (d) $ \theta=0 $时自旋反平行结构线性电导谱; (e), (f) $ \theta=0.5\pi $时自旋平行结构线性电导谱; (g), (h) $ \theta=0.5\pi $时自旋反平行结构线性电导谱
Figure 6. Linear conductance spectra of the system with ferromagnetic leads when $ \varepsilon_1=0 $, $ \varepsilon_{\rm m}=0 $, $ U_2=0.1, 0.2, 0.5 $: (a), (b) Linear conductances in the P spin configuration with $ \theta=0 $; (c), (d) linear conductances in the AP spin configuration with $ \theta=0 $; (e), (f) linear conductances in the P spin configuration with $ \theta=0.5\pi $; (g), (h) linear conductances in the AP spin configuration with $ \theta=0.5\pi $.
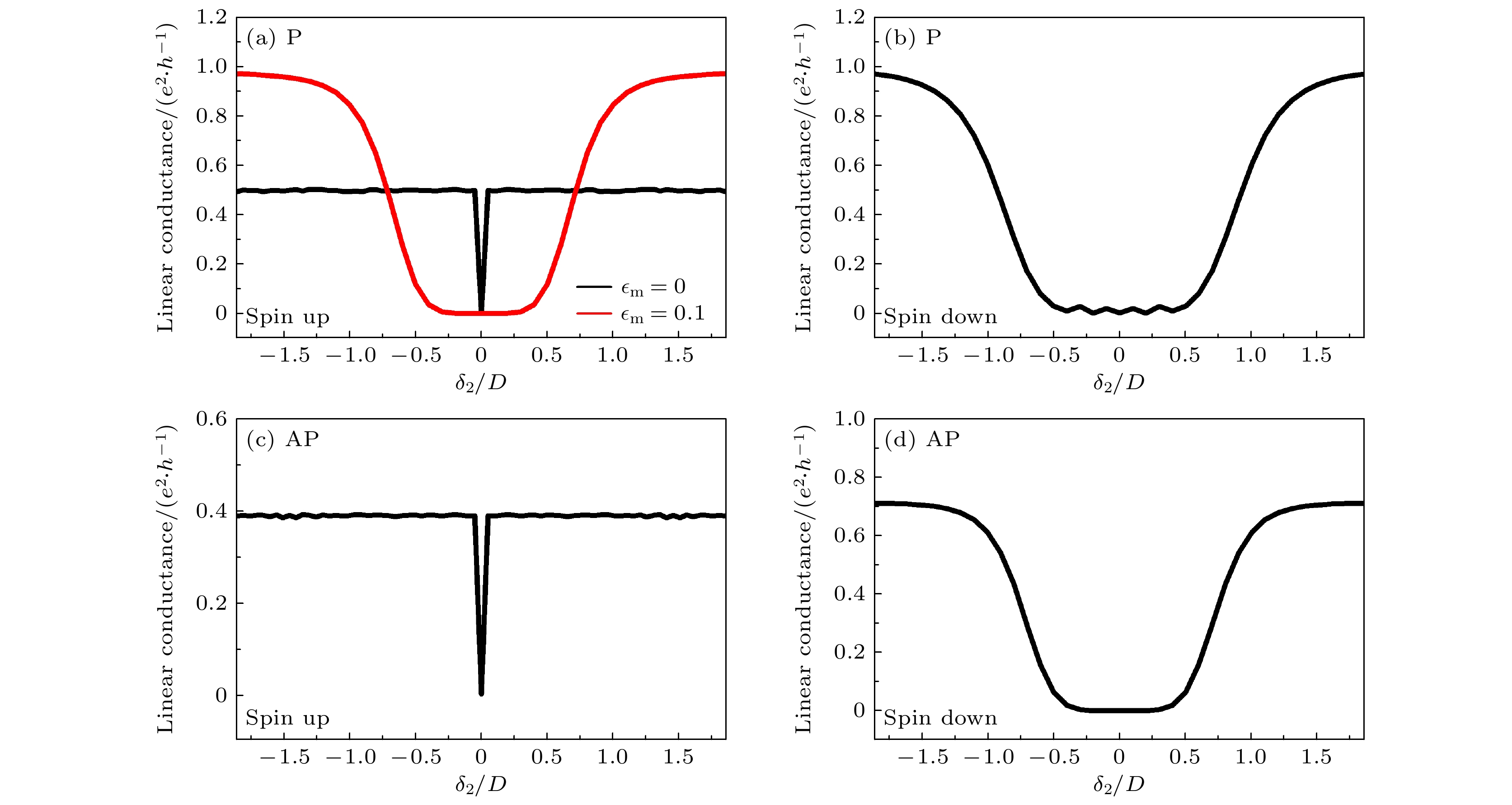
图 7 $ \lambda=0.1 D $时铁磁性电极耦合体系中Kondo区内量子点侧耦合马约拉纳束缚态的线性电导谱 (a), (b)自旋平行结构线性电导谱; (c), (d)自旋反平行结构线性电导谱
Figure 7. When $ \lambda=0.1 D $, linear conductances in the case of ferromagnetic leads, due to the side-coupled QD in the Kondo regime: (a), (b) Linear conductances in the P spin configuration; (c), (d) linear conductances in the AP spin configuration.
-
[1] Hyart T, van Heck B, Fulga I C, Burrello M, Akhmerov A R, Beenakker C W J 2013 Phys. Rev. B 88 035121
Google Scholar
[2] Reimann S M, Manninen M 2002 Rev. Mod. Phys. 74 1283
Google Scholar
[3] van der Wiel W G, De Franceschi S, Elzerman J M, Fujisawa T, Tarucha S, Kouwenhoven L P 2002 Rev. Mod. Phys. 75 1
Google Scholar
[4] Meir Y, Wingreen N S, Lee P A 1993 Phys. Rev. Lett. 70 2601
Google Scholar
[5] Zheng Y S, Lü T Q, Zhang C X, Su W H 2004 Physica E 24 290
Google Scholar
[6] Gong W J, Zheng Y S, Liu Y, Lü T Q 2006 Phys. Rev. B 73 245329
Google Scholar
[7] Sato M, Aikawa H, Kobayashi K, Katsumoto S, Iye Y 2005 Phys. Rev. Lett. 95 066801
Google Scholar
[8] Žitko R, Bonča J 2007 Phys. Rev. B 76 241305
Google Scholar
[9] Miroshnichenko A E, Flach S, Kivshar Y S 2010 Rev. Mod. Phys. 82 2257
Google Scholar
[10] Lee W R, Kimand J U, Sim H S 2008 Phys. Rev. B 77 033305
Google Scholar
[11] Michalek G, Bulka B R 2022 J. Magn. Magn. Mater. 544 168700
Google Scholar
[12] Ding G H, Kim C K, Nahm K 2005 Phys. Rev. B 71 205313
Google Scholar
[13] Eto M, Sakano R 2020 Phys. Rev. B 102 245402
Google Scholar
[14] Akera H 1993 Phys. Rev. B 47 6835
Google Scholar
[15] Brandes T, Kramer B 1999 Phys. Rev. Lett. 83 3021
Google Scholar
[16] Głodzik S, Wójcik K P, Weymann I, Domański T 2017 Phys. Rev. B 95 125419
Google Scholar
[17] Monteros A L, Uppal G S, McMillan S R, Crisan M, Tifrea I 2014 Eur. Phys. J. B 87 302
Google Scholar
[18] Piotr M, Wójcik K P, Weymann I 2022 Phys. Rev. B 105 075418
Google Scholar
[19] Wójcik K P, Weymann I 2014 Phys. Rev. B 90 115308
Google Scholar
[20] Wójcik K P, Weymann I 2015 Phys. Rev. B 91 134422
Google Scholar
[21] Mourik V, Zuo Z, Frolov S M, Plissard S R, Bakkers E P A M, Kouwenhoven L P 2012 Science 336 1003
Google Scholar
[22] Wang D F, Kong L Y, Fan P, Chen H, Zhu S Y, Liu W Y, Cao L, Sun Y J, Du S X, Schneeloch J, Zhong R D, Gu G, Fu L, Ding H, Gao H J 2018 Science 362 333
Google Scholar
[23] Stern A 2010 Nature 464 187
Google Scholar
[24] Chiu C K, Machida T, Huang Y, Hanaguri T, Zhang F C 2020 Sci. Adv. 6 eaay0443
Google Scholar
[25] Žitko R 2011 Phys. Rev. B 83 195137
Google Scholar
[26] Smirnov S 2022 Phys. Rev. B 105 205430
Google Scholar
[27] Máthé L, Sticlet D, Zârbo L P 2022 Phys. Rev. B 105 155409
Google Scholar
[28] Liu D E, Baranger H U 2011 Phys. Rev. B 84 201308
Google Scholar
[29] Gong W J, Zhang S F, Li Z C, Yi G Y, Zheng Y S 2014 Phys. Rev. B 89 245413
Google Scholar
[30] Gong W J, Zhao Y, Gao Z 2015 Curr. Appl. Phys. 15 520
Google Scholar
[31] Liu J, Wang J, Zhang F C 2014 Phys. Rev. B 90 035307
Google Scholar
[32] Zocher B, Rosenow B 2013 Phys. Rev. Lett. 111 036802
Google Scholar
[33] Gong T, Zhang L L, Dai X F, Jiang C, Gong W J 2022 Eur. Phys. J. Plus 137 122
Google Scholar
[34] Gao Z, Gong W J 2016 Phys. Rev. B 94 104506
Google Scholar
[35] Cheng M, Becker M, Bauer B, Bauer B, Lutchyn R M 2014 Phys. Rev. X 4 031051
[36] Weymann I, Wójcik K P, Majek P 2020 Phys. Rev. B 101 235404
Google Scholar
[37] Calle A M, Pacheco M, Orellana P A, Otálora J A 2020 Ann. Phys. 532 1900409
Google Scholar
[38] Wang X Q, Zhang S F, Han Y, Gong W J 2019 Phys. Rev. B 100 115405
Google Scholar
[39] Ramos-Andrade J P, Peña F J, González A, Ávalos-Ovando O, Orellana P A 2017 Phys. Rev. B 96 165413
Google Scholar
[40] Žitko R, Bonča J 2008 Phys. Rev. B 77 245112
Google Scholar
[41] Žitko R, 2011 Comput. Phys. Commun. 182 2259
Google Scholar
[42] Liu Y, Zheng Y S, Gong W J, Lü T Q 2007 Phys. Rev. B 75 195316
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1827
- PDF Downloads: 63
- Cited By: 0
