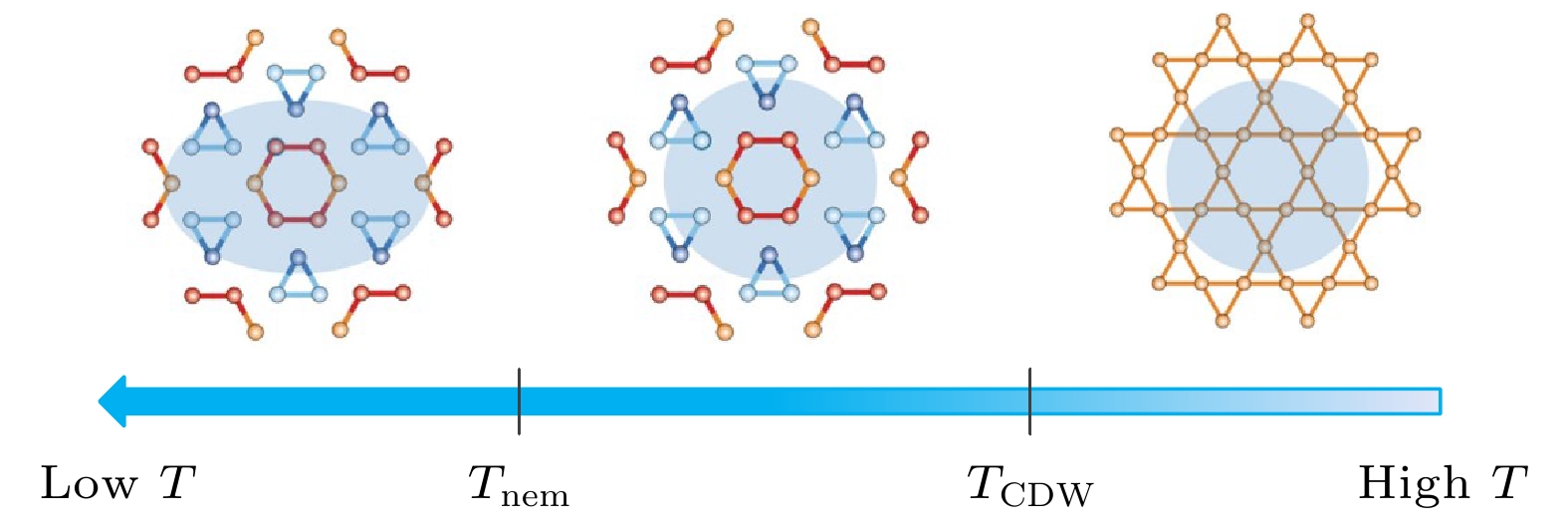
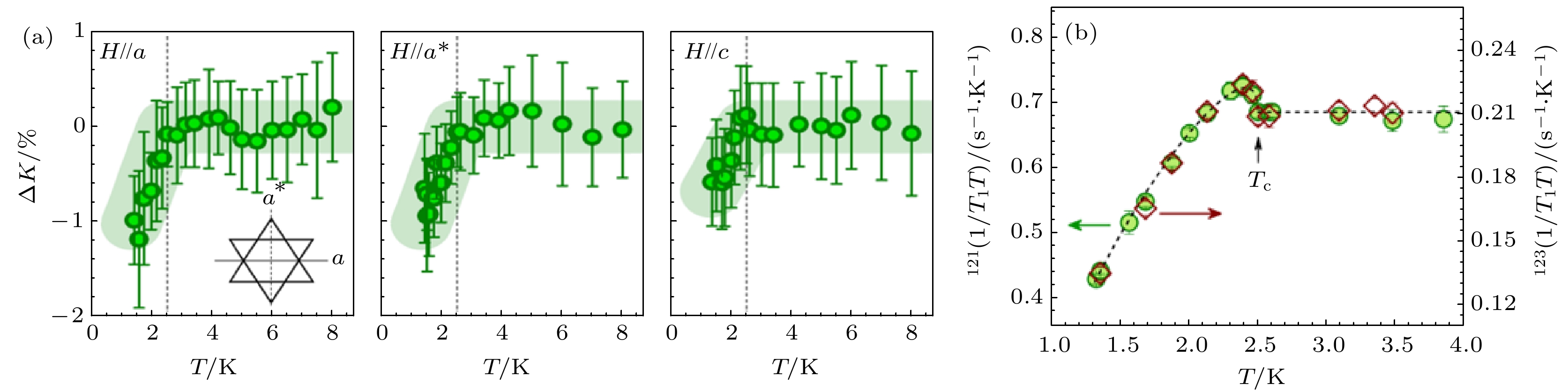
-
The newly discovered Kagome superconductors $ A{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5}(A=\mathrm{K},\mathrm{R}\mathrm{b},\mathrm{C}\mathrm{s}) $ provide a platform to investigate the interplay of the topological property, superconductivity and geometrical frustration. Since their discovery, many research groups, especially many groups in China, have made tremendous progress in this field, including time-reversal-symmetry-breaking (TRSB), charge density wave (CDW), electronic nematicity, superconductivity properties and pair density wave (PDW). In this paper, we introduce the$ A{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5} $ properties, discuss the recent research progress and highlight the future focus of this Kagome superconductor.The paper is organized as follows. We start from the exotic normal states of $ A{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5} $ , where a CDW emerges at the temperature around 70–100 K depending on$ A $ . This CDW enlarges the unit cell size to 2×2 with additional c-direction modulation as observed by scanning tunneling microscope (STM) and X-ray scattering experiments. Interestingly, this CDW behaves differently under opposite magnetic fields. Namely, this CDW may break the time reversal symmetry. To confirm this property, the zero field muon spin relaxation (ZFμSR) experiment is performed with increasing relaxation rates after the CDW transition. Additionally, the intrinsic anomalous Hall effect is also observed, which may relate to this time reversal symmetry breaking (TRSB). Since there are no long-range magnetic orders observed in the elastic neutron scattering experiment and μSR, the TRSB is not related to the electron spin degree of freedom. To explain the TRSB, the chiral flux phase (CFP) with orbital magnetism is theoretically proposed. Moreover, the electronic nematicity is also observed at about 30–50 K below the CDW transition temperature. This phase breaks the$ {C}_{6} $ rotation symmetry of the Kagome lattice as confirmed by STM and nuclear magnetic resonance (NMR). What is the microscopic origin of this nematicity is still under investigation.Then, we move to the superconducting properties of $ A{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5} $ . Combining the inversion symmetry property found in optical measurement and decreasing of the spin susceptibility found in NMR, the$ A{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5} $ superconductor is proven to be a spin-singlet superconductor. Experiments in NMR, angle-resolved photoemission, superfluid density and specific heat further confirm the superconductivity in Kagome superconductors is a conventional s-wave superconductor. Although this superconductor is conventional,$ A{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5} $ also contains the unconventional property. Importantly, a PDW is observed in$ \mathrm{C}\mathrm{s}{\mathrm{V}}_{3}{\mathrm{S}\mathrm{b}}_{5} $ by high-resolution STM. What is the PDW origin or microscopic mechanism is still an open question. These new progress reveal the intriguing physical properties behind the Kagome superconductors and also bring many unsolved questions, which calls for further investigations.-
Keywords:
- superconductivity /
- charge density waves /
- time-reversal symmetry breaking /
- electron nematic phases /
- paired density waves
[1] Onsager L 1944 Phys. Rev. 65 117
Google Scholar
[2] Syozi I 1951 Prog. Theor. Phys. 6 306
Google Scholar
[3] Ortiz B R, Gomes L C, Morey J R, et al. 2019 Phys. Rev. Mater. 3 094407
Google Scholar
[4] Ortiz B R, Teicher S M L, Hu Y, et al. 2020 Phys. Rev. Lett. 125 247002
Google Scholar
[5] Jiang Y X, Yin J X, Denner M M, et al. 2021 Nat. Mater. 20 1353
Google Scholar
[6] Yu L, Wang C, Zhang Y, et al. arXiv: 2107.10714
[7] Mielke III C, Das D, Yin J X, et al. 2022 Nature 602 245
Google Scholar
[8] Guguchia Z, Mielke III C, Das D, et al. arXiv: 2202.07713
[9] Yu F H, Wu T, Wang Z Y, Lei B, Zhuo W Z, Ying J J, Chen X H 2021 Phys. Rev. B 104 L041103
Google Scholar
[10] Feng X, Jiang K, Wang Z, Hu J 2021 Sci. Bull. 66 1384
Google Scholar
[11] Feng X, Zhang Y, Jiang K, Hu J 2021 Phys. Rev. B 104 165136
Google Scholar
[12] Nie L, Sun K, Ma W, et al. 2022 Nature 604 59
Google Scholar
[13] Mu C, Yin Q, Tu Z, Gong C, Lei H, Li Z, Luo J 2021 Chin. Phys. Lett. 38 077402
Google Scholar
[14] Duan W, Nie Z, Luo S, et al. 2021 Sci. China Phys. Mech. Astron. 64 107462
Google Scholar
[15] Luo H, Gao Q, Liu H, et al. 2022 Nat Commun 13 273
Google Scholar
[16] Chen H, Yang H, Hu B, et al. 2021 Nature 599 222
Google Scholar
[17] Hamidian M H, Edkins S D, Joo S H, Kostin A, Eisaki H, Uchida S, Lawler M J, Kim E A, Mackenzie A P, Fujita K, Lee J, Davis J C S 2016 Nature 532 343
Google Scholar
[18] Edkins S D, Kostin A, Fujita K, Mackenzie A P, Eisaki H, Uchida S, Sachdev S, Lawler M J, Kim E A, Davis J C S, Hamidian M H 2019 Science 364 976
Google Scholar
-
图 1 (a) 笼目结构示意图; (b) CsV3Sb5元胞结构示意图[4]; (c) 仅考虑最近邻原子间电子跃迁时, 笼目晶格的电子能谱; (d) 常压下笼目超导体随温度变化的相图
Figure 1. (a) Schematic diagram of the Kagome lattice structure; (b) schematic diagram of the primitive unit cell structure of CsV3Sb5[4]; (c) the electronic energy spectrum of the Kagome lattice when electrons hop between the nearest neighbor atoms; (d) the Kagome superconductor’s phase diagram as a function of temperature under ambient pressure.
图 2 (a) CsV3Sb5比热随温度的变化[4], 其中ZFC代表零场条件, FC代表有场条件; (b) STM下KV3Sb5的电荷密度分布图, 内嵌图为其傅里叶变换的结果表现出2×2有序的特性[5]
Figure 2. (a) The specific heat of CsV3Sb5 varies with temperature[4], ZFC means zero field case, and FC means finite field case; (b) the charge density distribution of KV3Sb5 observed by STM, the inset image is the result of Fourier transform which shows the characteristic of 2×2 order[5].
图 7 (a) BCS超导电子配对示意图(左)与FFLO态配对示意图(右); (b) CsV3Sb5的零场STM结果快速傅里叶变换图, 绿色圈中的峰对应于2×2的CDW相, 粉色圈中的峰对应于PDW相[16]
Figure 7. (a) Cooper pair for BCS superconductor (left) and for FFLO state (right); (b) Fourier transform of the STM diagram of CsV3Sb5 under zero field, the green cycle shows the 2×2 CDW phase, the pink cycle shows the PDW phase[16].
-
[1] Onsager L 1944 Phys. Rev. 65 117
Google Scholar
[2] Syozi I 1951 Prog. Theor. Phys. 6 306
Google Scholar
[3] Ortiz B R, Gomes L C, Morey J R, et al. 2019 Phys. Rev. Mater. 3 094407
Google Scholar
[4] Ortiz B R, Teicher S M L, Hu Y, et al. 2020 Phys. Rev. Lett. 125 247002
Google Scholar
[5] Jiang Y X, Yin J X, Denner M M, et al. 2021 Nat. Mater. 20 1353
Google Scholar
[6] Yu L, Wang C, Zhang Y, et al. arXiv: 2107.10714
[7] Mielke III C, Das D, Yin J X, et al. 2022 Nature 602 245
Google Scholar
[8] Guguchia Z, Mielke III C, Das D, et al. arXiv: 2202.07713
[9] Yu F H, Wu T, Wang Z Y, Lei B, Zhuo W Z, Ying J J, Chen X H 2021 Phys. Rev. B 104 L041103
Google Scholar
[10] Feng X, Jiang K, Wang Z, Hu J 2021 Sci. Bull. 66 1384
Google Scholar
[11] Feng X, Zhang Y, Jiang K, Hu J 2021 Phys. Rev. B 104 165136
Google Scholar
[12] Nie L, Sun K, Ma W, et al. 2022 Nature 604 59
Google Scholar
[13] Mu C, Yin Q, Tu Z, Gong C, Lei H, Li Z, Luo J 2021 Chin. Phys. Lett. 38 077402
Google Scholar
[14] Duan W, Nie Z, Luo S, et al. 2021 Sci. China Phys. Mech. Astron. 64 107462
Google Scholar
[15] Luo H, Gao Q, Liu H, et al. 2022 Nat Commun 13 273
Google Scholar
[16] Chen H, Yang H, Hu B, et al. 2021 Nature 599 222
Google Scholar
[17] Hamidian M H, Edkins S D, Joo S H, Kostin A, Eisaki H, Uchida S, Lawler M J, Kim E A, Mackenzie A P, Fujita K, Lee J, Davis J C S 2016 Nature 532 343
Google Scholar
[18] Edkins S D, Kostin A, Fujita K, Mackenzie A P, Eisaki H, Uchida S, Sachdev S, Lawler M J, Kim E A, Davis J C S, Hamidian M H 2019 Science 364 976
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7505
- PDF Downloads: 664
- Cited By: 0
