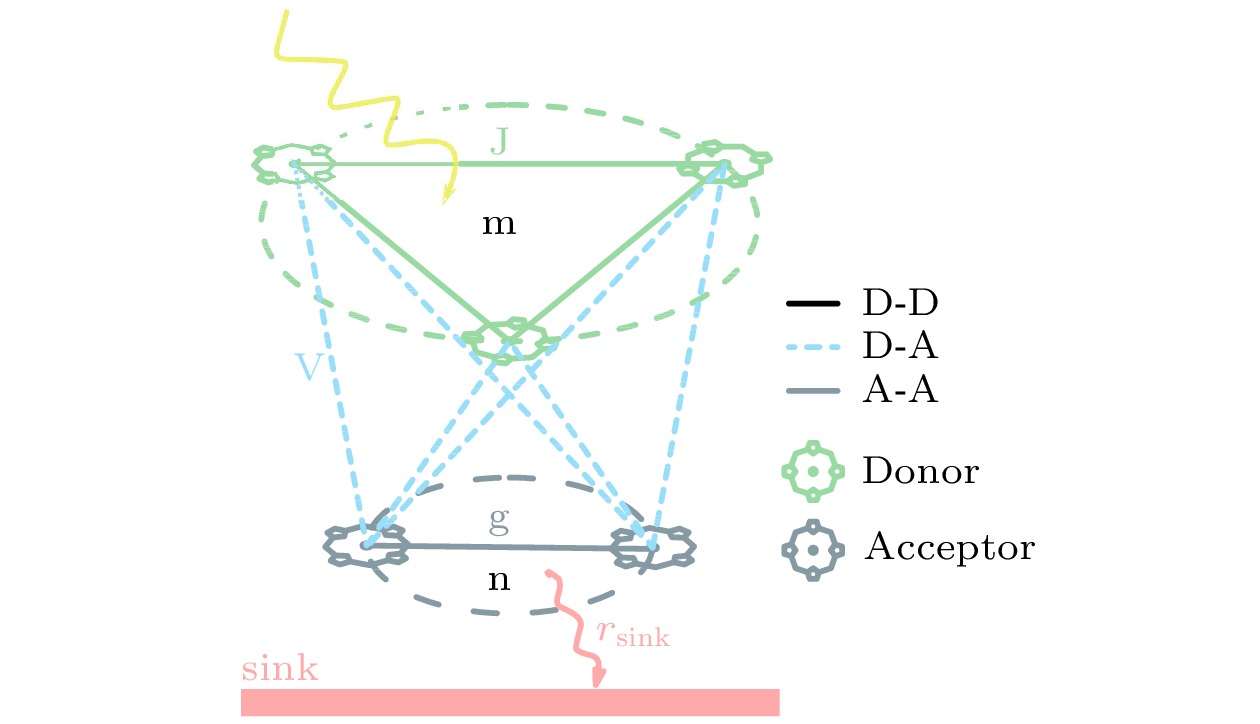
-
Most studies on quantum effects in the process of excitation energy transfer in photosynthesis system are based on the single-excitation initial state hypothesis, which can well describe the initial state of some photosynthesis systems that people are concerned about. But for natural and artificial photosynthesis systems that do not meet the above hypothesis, the excitation process has a non-negligible impact on the system dynamics. Based on a multi-pigments model excited by Gaussian pulse, the effects of the excitation pulse width and the excitation interval on system dynamics and excitation energy transfer efficiency are studied. First, the kinetic equations for the overall evolution of the donor system and the acceptor system that can theoretically contain any number of pigments are derived. Afterwards, the relationship between the excitation energy transfer efficiency and the related parameters of the system, as well as the optimal range of the corresponding parameters are demonstrated by numerical simulation. It is found that under the condition of donor pigments being excited by a single Gaussian pulse, there exists optimal pulse width, and the optimal range of the pigment molecule numbers, the coupling strength as well as the dephasing rate can be modulated by the pulse width. The mechanism of the above modulation is also analyzed and presented. Under the condition of donor pigments being excited by two Gaussian pulses sequentially, there exists an optimal combination of pulse width and pulse interval. The kinetic equations obtained in this paper can be extended to other forms of excitation pulses. The numerical results and the related optimal design principles obtained have reference significance for the optimal design of artificial photosynthesis systems under different light conditions.
[1] Cardona T 2018 Heliyon 4 e00548
Google Scholar
[2] Van A H, Valkunas L, Van G R 2000 Photosynthetic Excitons (Singapore: World Scientific) pp1-45, 56
[3] May V, Kühn O 2011 Charge and Energy Transfer Dynamics in Molecular Systems (Vol. 2) (Weinheim: Wiley-vch Verlag GmbH & Co. KGaA) pp1-7
[4] Ishizaki A, Calhoun T R, Schlau-Cohen G S, Fleming G R 2010 Phys. Chem. Chem. Phys. 12 7319
Google Scholar
[5] Scholes G D, Fleming G R, Olaya-Castro A, Van G R 2011 Nat. Chem. 3 763
Google Scholar
[6] Olaya-Castro A, Scholes G D 2011 Int. Rev. Phys. Chem. 30 49
Google Scholar
[7] Collini E 2013 Chem. Soc. Rev. 42 4932
Google Scholar
[8] Levi F, Mostarda S, Rao F, Mintert F 2015 Rep. Prog. Phys. 78 082001
Google Scholar
[9] Olaya-Castro A, Lee C F, Olsen F F, Johnson N F 2008 Phys. Rev. B 78 085115
Google Scholar
[10] Fassioli F, Nazir A, Olaya-Castro A 2010 J. Phys. Chem. Lett. 1 2139
Google Scholar
[11] Zhang Y P, Li H R, Fang A P, Chen H, Li F L 2013 Chin. Phys. B 22 057104
Google Scholar
[12] Plenio M B, Huelga S F 2008 New J. Phys. 10 113019
Google Scholar
[13] Mohseni M, Rebentrost P, Lloyd S, Aspuru-Guzik A 2008 J. Chem. Phys. 129 174106
Google Scholar
[14] Chen H, Wang X, Han C M, Li H R 2019 J. Phys. B: At. Mol. Opt. Phys. 52 075501
Google Scholar
[15] Chan H C, Gamel O E, Fleming G R, Whaley K B 2018 J. Phys. B: At. Mol. Opt. Phys. 51 054002
Google Scholar
[16] Barbatti M 2020 J. Chem. Theory Comput. 16 4849
Google Scholar
[17] Li H R, Zhang P, Liu Y J, Li F L, Zhu S Y 2013 Phys. Rev. A 87 053831
Google Scholar
[18] Zong X L, Song W, Zhou J, Yang M, Yu L B, Cao Z L 2018 Quantum Inf. Process. 17 158
[19] Golubev N V, Kuleff A I 2014 Phys. Rev. A 90 035401
Google Scholar
[20] Medina I, Semiao F L 2019 Phys. Rev. A 100 012103
Google Scholar
[21] Scully M O, Zubairy M S 1997 Quantum Optics (Cambridge: Cambridge University Press) pp248-268
[22] Yang S, Xu D Z, Song Z, Sun C P 2010 J. Chem. Phys. 132 234501
Google Scholar
[23] Caruso F, Chin A W, Datta A, Huelga S F, Plenio M B 2009 J. Chem. Phys. 131 105106
Google Scholar
-
图 1 理论模型示意图
Figure 1. Schematic diagram of the theoretical model.
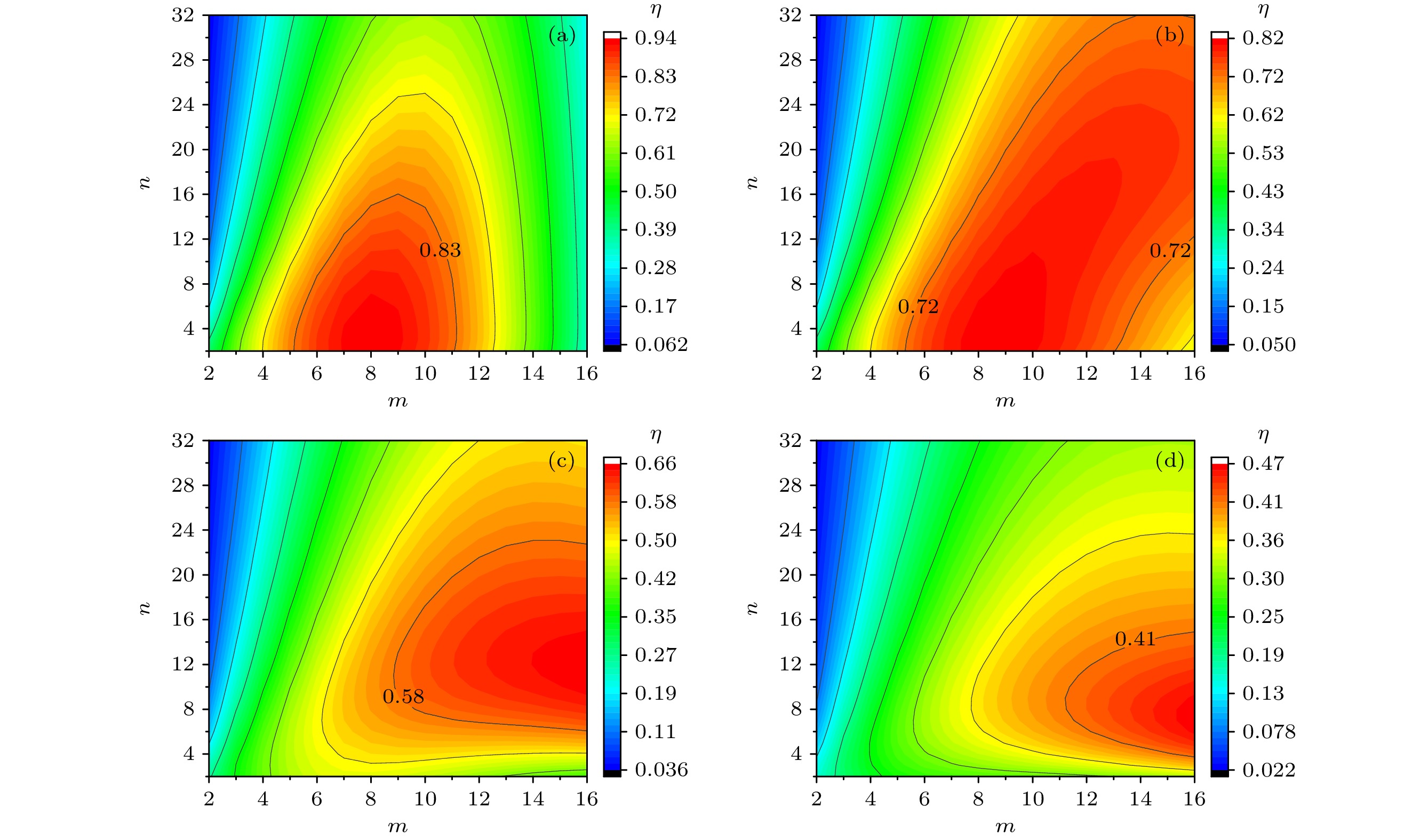
图 2 脉冲宽度
$ \tau_{\rm{p}} $ 不同时激发能传输效率$ \eta $ 与供体数目$ m $ 和受体数目$ n $ 之间的关系 (a)$ \tau_{{\rm{p}}} = 0.01 V_{0}^{-1} $ ; (b)$ \tau_{{\rm{p}}} = 0.05 V_{0}^{-1} $ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1} $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1} $ Figure 2. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the number of donors ($ m $ ) and acceptors ($ n $ ) with different pulse width$ \tau_{\rm{p}} $ : (a)$ \tau_{{\rm{p}}} = 0.01 V_{0}^{-1} $ ; (b)$ \tau_{{\rm{p}}} = 0.05 V_{0}^{-1} $ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1} $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1} $ .
图 3 脉冲宽度
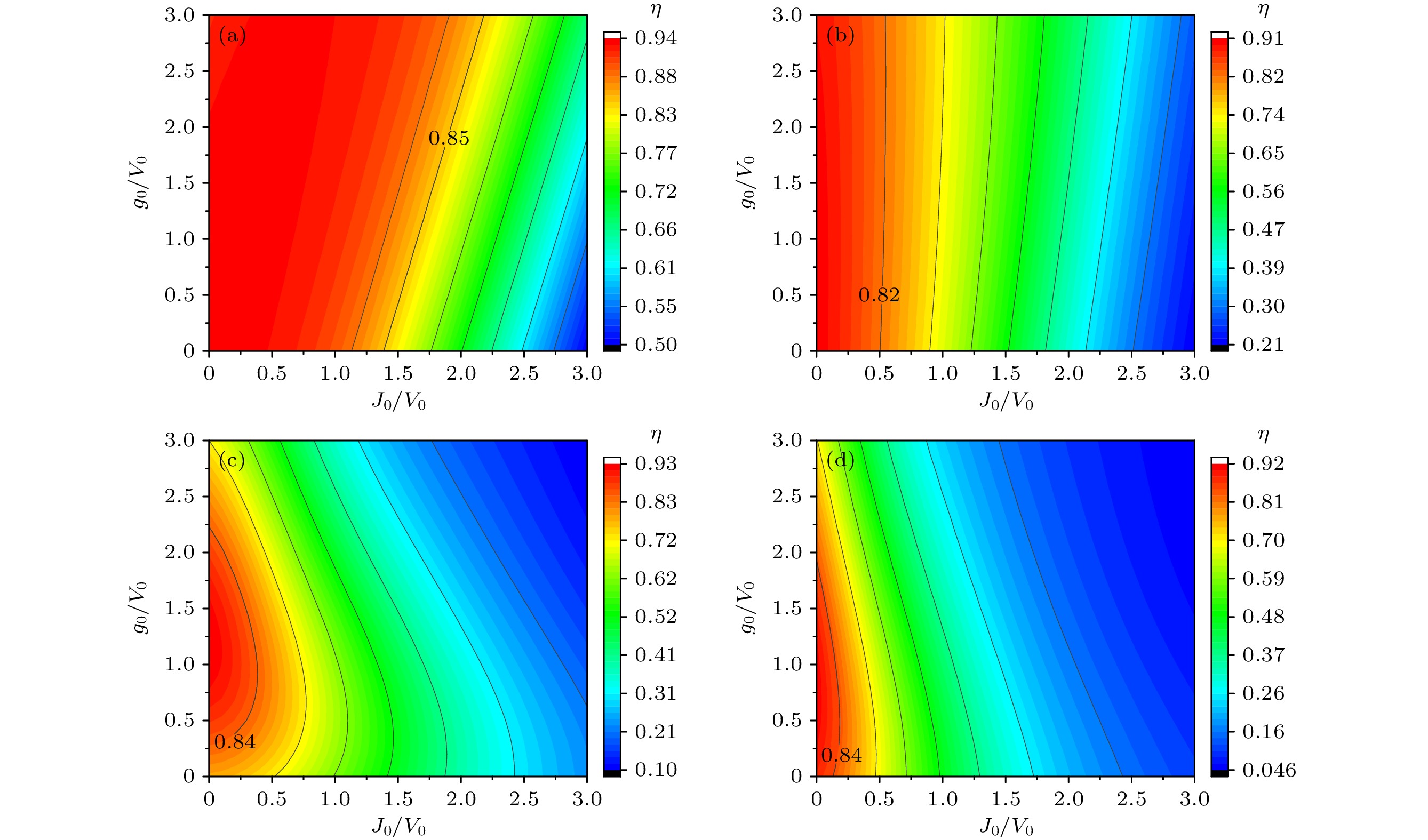
$ \tau_{\rm{p}} $ 不同时激发能传输效率$ \eta $ 与供体色素分子间耦合强度$ J_{0} $ 以及受体色素分子间耦合强度$ g_{0} $ 之间的关系 (a)$\tau_{{\rm{p}}}= $ $ 0.01 V_{0}^{-1}$ ; (b)$ \tau_{{\rm{p}}} = 0.05 V_{0}^{-1} $ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1} $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1} $ Figure 3. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the coupling strength of the donor pigments$ J_{0} $ as well as the coupling strength of the acceptor pigments$ g_{0} $ with different pulse width$ \tau_{\rm{p}} $ : (a)$ \tau_{{\rm{p}}} = 0.01 V_{0}^{-1} $ ; (b)$\tau_{{\rm{p}}} = $ $ 0.05 V_{0}^{-1}$ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1} $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1} $ 
图 4 脉冲宽度
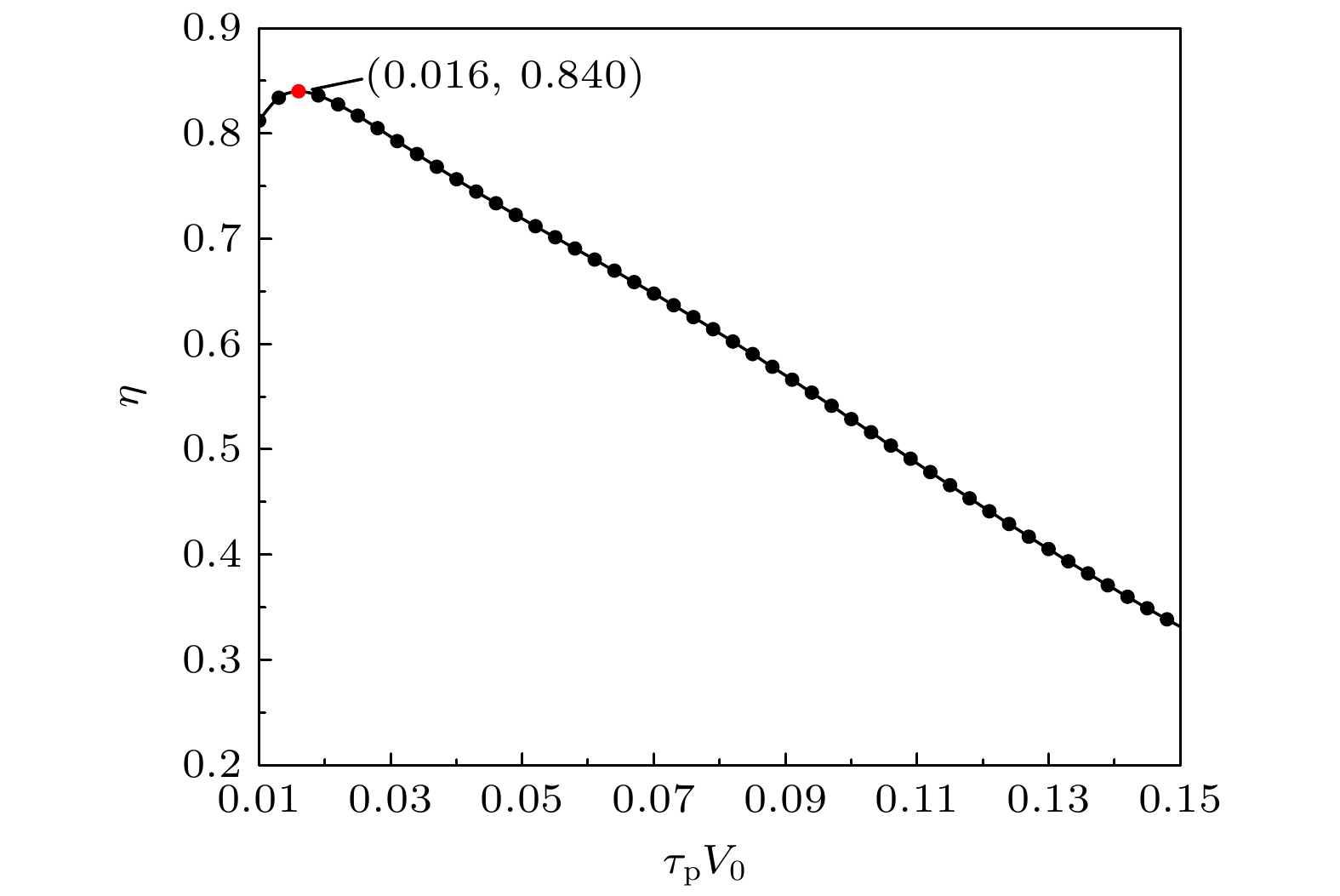
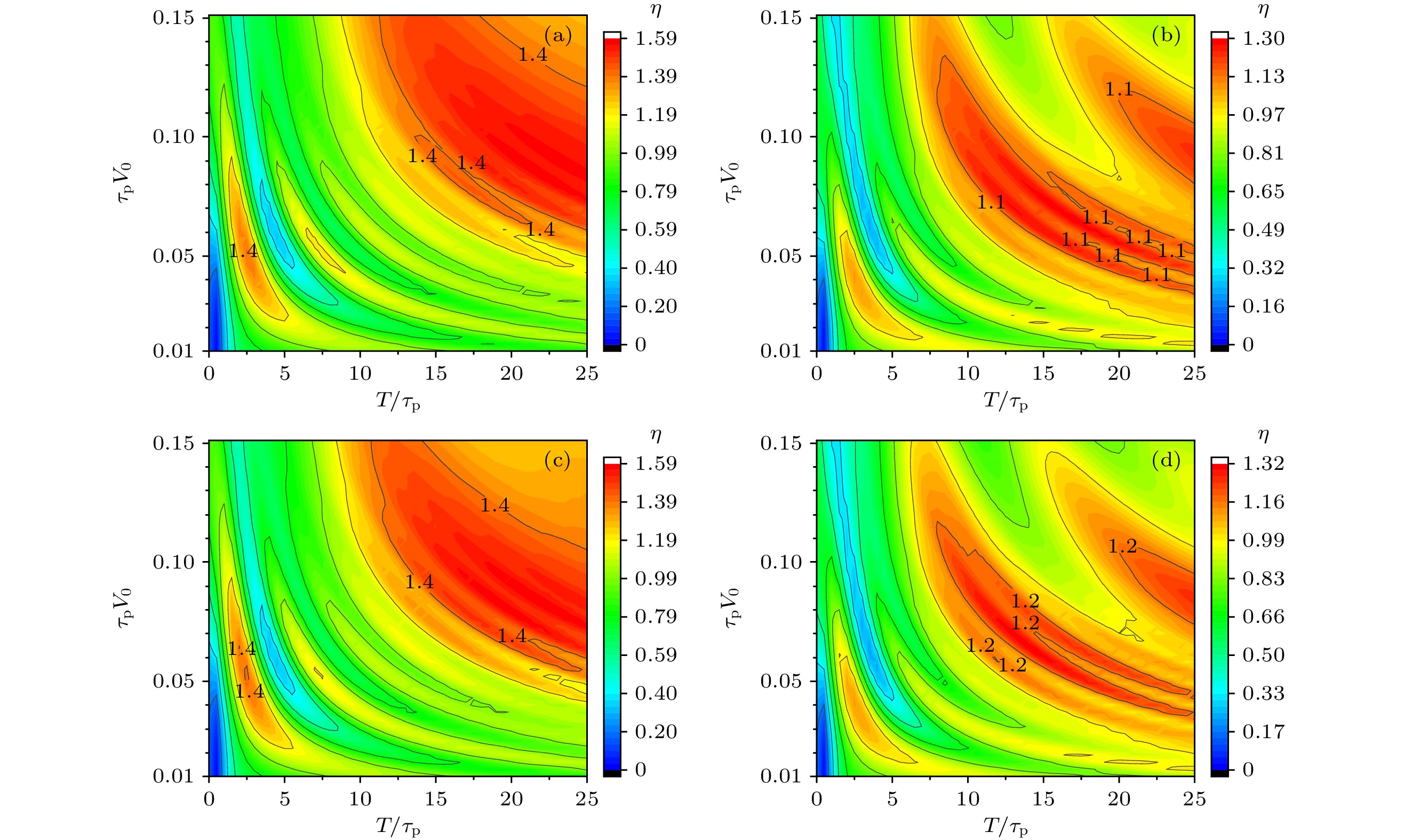
$ \tau_{\rm{p}} $ 及供体数目$ m $ 和受体数目$ n $ 不同时, 激发能传输效率$ \eta $ 与供体色素分子间耦合强度$ J_{0} $ 和受体色素分子间耦合强度$ g_{0} $ 之间的关系 (a)$ \tau_{{\rm{p}}} = 0.01 V_{0}^{-1}, \; m = 8, \; n = 3 $ ; (b)$ \tau_{{\rm{p}}} = 0.05 V_{0}^{-1}, \; m = 9, \; n = 3 $ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1}, \; m = 16, \; n = 7 $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1}, \; m = 16, \; n = 12 $ Figure 4. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the coupling strength of the donor pigments$ J_{0} $ as well as the coupling strength of the acceptor pigments$ g_{0} $ with different pulse width$ \tau_p $ , donor pigments number$ m $ and acceptor pigments number$ n $ : (a) Pulse width$ \tau_{{\rm{p}}}=0.01 V_{0}^{-1}, \; m = 8, \; n = 3 $ ; (b) pulse width$ \tau_{{\rm{p}}}=0.05 V_{0}^{-1}, \; m = 9, \; n = 3 $ ; (c) pulse width$ \tau_{{\rm{p}}}=0.1 V_{0}^{-1}, \; m = 16, \; n = 7 $ ; (d) pulse width$ \tau_{{\rm{p}}}=0.15 V_{0}^{-1}, \; m = 16, \; n = 12 $ 
图 5 激发能传输效率
$ \eta $ 与脉冲宽度$ \tau_{{\rm{p}}} $ 之间的关系Figure 5. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the pulse width$ \tau_{\rm{p}} $ 
图 6 脉冲宽度
$ \tau_{\rm{p}} $ 不同时激发能传输效率$ \eta $ 与供体色素分子的退相位速率$ \varGamma^{\prime} $ 以及受体分子的退相位速率$ \kappa^{\prime} $ 之间的关系 (a)$\tau_{{\rm{p}}} = $ $ 0.01 V_{0}^{-1}$ ; (b)$ \tau_{{\rm{p}}} = 0.05 V_{0}^{-1} $ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1} $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1} $ Figure 6. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the dephasing rate of the donor pigments$ \varGamma^{\prime} $ as well as the dephasing rate of the acceptor pigments$ \kappa^{\prime} $ with different pulse width$ \tau_{\rm{p}} $ : (a)$ \tau_{{\rm{p}}} = 0.01 V_{0}^{-1} $ ; (b)$ \tau_{{\rm{p}}} = 0.05 V_{0}^{-1} $ ; (c)$ \tau_{{\rm{p}}} = 0.1 V_{0}^{-1} $ ; (d)$ \tau_{{\rm{p}}} = 0.15 V_{0}^{-1} $ 
图 7 双脉冲激发时, 激发能传输效率与脉冲间隔
$ T $ 之间的关系Figure 7. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the pulse interval$ T $ with double-pulse excitation
图 8 双脉冲激发时, 激发能传输效率
$ \eta $ 与脉冲间隔$ T $ 以及脉冲宽度$\tau_{{\rm{p}}}$ 之间的关系Figure 8. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the pulse interval$ T $ as well as the pulse width$\tau_{\rm{p}}$ with double-pulse excitation
图 9 供体色素分子间耦合强度
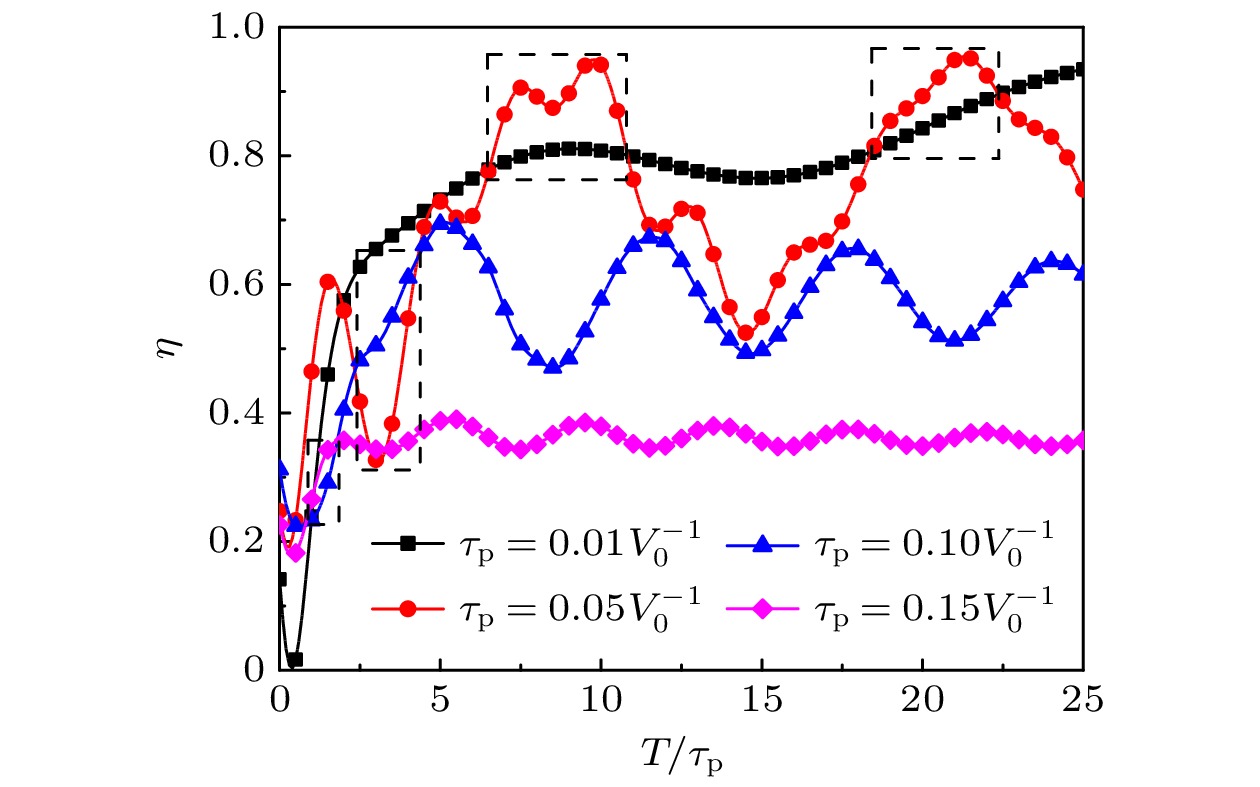
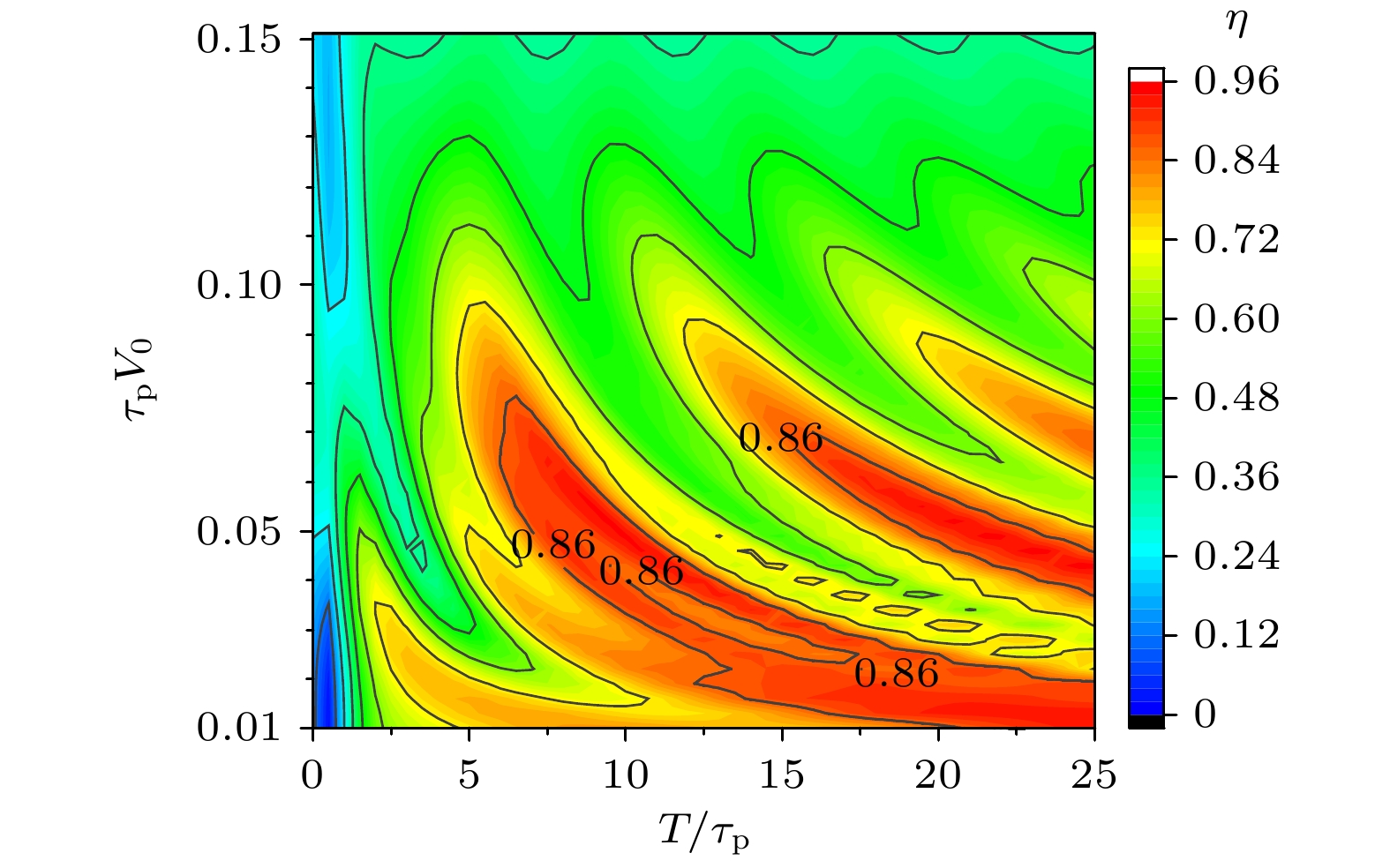
$ J_{0} $ 以及受体色素分子间耦合强度$ g_{0} $ 不同时激发能传输效率$ \eta $ 与脉冲间隔$ T $ 以及脉冲宽度$ \tau_{{\rm{p}}} $ 之间的关系 (a)$ J_{0} = 0.1 V_{0}, \; g_{0}=0.5 V_{0} $ ; (b)$ J_{0} = 0.1 V_{0}, \; g_{0}=1.0 V_{0} $ ; (c)$ J_{0} = 0.2 V_{0}, \; g_{0}=0.5 V_{0} $ ; (d)$ J_{0} = 0.2 V_{0}, \; g_{0}=1.0 V_{0} $ Figure 9. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the pulse interval$ T $ as well as the pulse width$ \tau_{\rm{p}} $ with different coupling strength of the donor pigments$ J_{0} $ and coupling strength of the acceptor pigments$ g_{0} $ : (a)$J_{0} = $ $ 0.1 V_{0}, \; g_{0}=0.5 V_{0}$ ; (b)$ J_{0} = 0.1 V_{0}, \; g_{0}=1.0 V_{0} $ ; (c)$ J_{0} = 0.2 V_{0}, \; g_{0}=0.5 V_{0} $ ; (d)$ J_{0} = 0.2 V_{0}, \; g_{0}=1.0 V_{0} $ 
图 10 供体色素分子数目
$ m $ 及受体色素分子数目$ n $ 不同时激发能传输效率$ \eta $ 与脉冲间隔$ T $ 以及脉冲宽度$\tau_{{\rm{p}}}$ 之间的关系 (a) 色素分子数目$ m = 8, \; n = 3 $ ; (b)色素分子数目$ m = 9, \; n = 3 $ ; (c)色素分子数目$ m = 16, \; n = 7 $ ; (d)色素分子数目$m = 16, \; $ $ n = 12$ Figure 10. The relationship between the efficiency of the excitation energy transfer
$ \eta $ and the pulse interval$ T $ as well as the pulse width$\tau_{\rm{p}}$ with different donor pigments number$ m $ and acceptor pigments number$ n $ : (a)$ m = 8, \; n = 3 $ ; (b)$ m = 9, \; n = 3 $ ; (c)$ m = $ $ 16, \; n = 7 $ ; (d)$ m = 16, \; n = 12 $ -
[1] Cardona T 2018 Heliyon 4 e00548
Google Scholar
[2] Van A H, Valkunas L, Van G R 2000 Photosynthetic Excitons (Singapore: World Scientific) pp1-45, 56
[3] May V, Kühn O 2011 Charge and Energy Transfer Dynamics in Molecular Systems (Vol. 2) (Weinheim: Wiley-vch Verlag GmbH & Co. KGaA) pp1-7
[4] Ishizaki A, Calhoun T R, Schlau-Cohen G S, Fleming G R 2010 Phys. Chem. Chem. Phys. 12 7319
Google Scholar
[5] Scholes G D, Fleming G R, Olaya-Castro A, Van G R 2011 Nat. Chem. 3 763
Google Scholar
[6] Olaya-Castro A, Scholes G D 2011 Int. Rev. Phys. Chem. 30 49
Google Scholar
[7] Collini E 2013 Chem. Soc. Rev. 42 4932
Google Scholar
[8] Levi F, Mostarda S, Rao F, Mintert F 2015 Rep. Prog. Phys. 78 082001
Google Scholar
[9] Olaya-Castro A, Lee C F, Olsen F F, Johnson N F 2008 Phys. Rev. B 78 085115
Google Scholar
[10] Fassioli F, Nazir A, Olaya-Castro A 2010 J. Phys. Chem. Lett. 1 2139
Google Scholar
[11] Zhang Y P, Li H R, Fang A P, Chen H, Li F L 2013 Chin. Phys. B 22 057104
Google Scholar
[12] Plenio M B, Huelga S F 2008 New J. Phys. 10 113019
Google Scholar
[13] Mohseni M, Rebentrost P, Lloyd S, Aspuru-Guzik A 2008 J. Chem. Phys. 129 174106
Google Scholar
[14] Chen H, Wang X, Han C M, Li H R 2019 J. Phys. B: At. Mol. Opt. Phys. 52 075501
Google Scholar
[15] Chan H C, Gamel O E, Fleming G R, Whaley K B 2018 J. Phys. B: At. Mol. Opt. Phys. 51 054002
Google Scholar
[16] Barbatti M 2020 J. Chem. Theory Comput. 16 4849
Google Scholar
[17] Li H R, Zhang P, Liu Y J, Li F L, Zhu S Y 2013 Phys. Rev. A 87 053831
Google Scholar
[18] Zong X L, Song W, Zhou J, Yang M, Yu L B, Cao Z L 2018 Quantum Inf. Process. 17 158
[19] Golubev N V, Kuleff A I 2014 Phys. Rev. A 90 035401
Google Scholar
[20] Medina I, Semiao F L 2019 Phys. Rev. A 100 012103
Google Scholar
[21] Scully M O, Zubairy M S 1997 Quantum Optics (Cambridge: Cambridge University Press) pp248-268
[22] Yang S, Xu D Z, Song Z, Sun C P 2010 J. Chem. Phys. 132 234501
Google Scholar
[23] Caruso F, Chin A W, Datta A, Huelga S F, Plenio M B 2009 J. Chem. Phys. 131 105106
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4039
- PDF Downloads: 60
- Cited By: 0
