Optimal resonance response of  nonlinear system excited by nonlinear frequency modulation signal

Gong Tao; Yang Jian-Hua; Shan Zhen; Wang Zhi-Le; Liu Hou-Guang

doi:10.7498/aps.71.20211959

Abstract

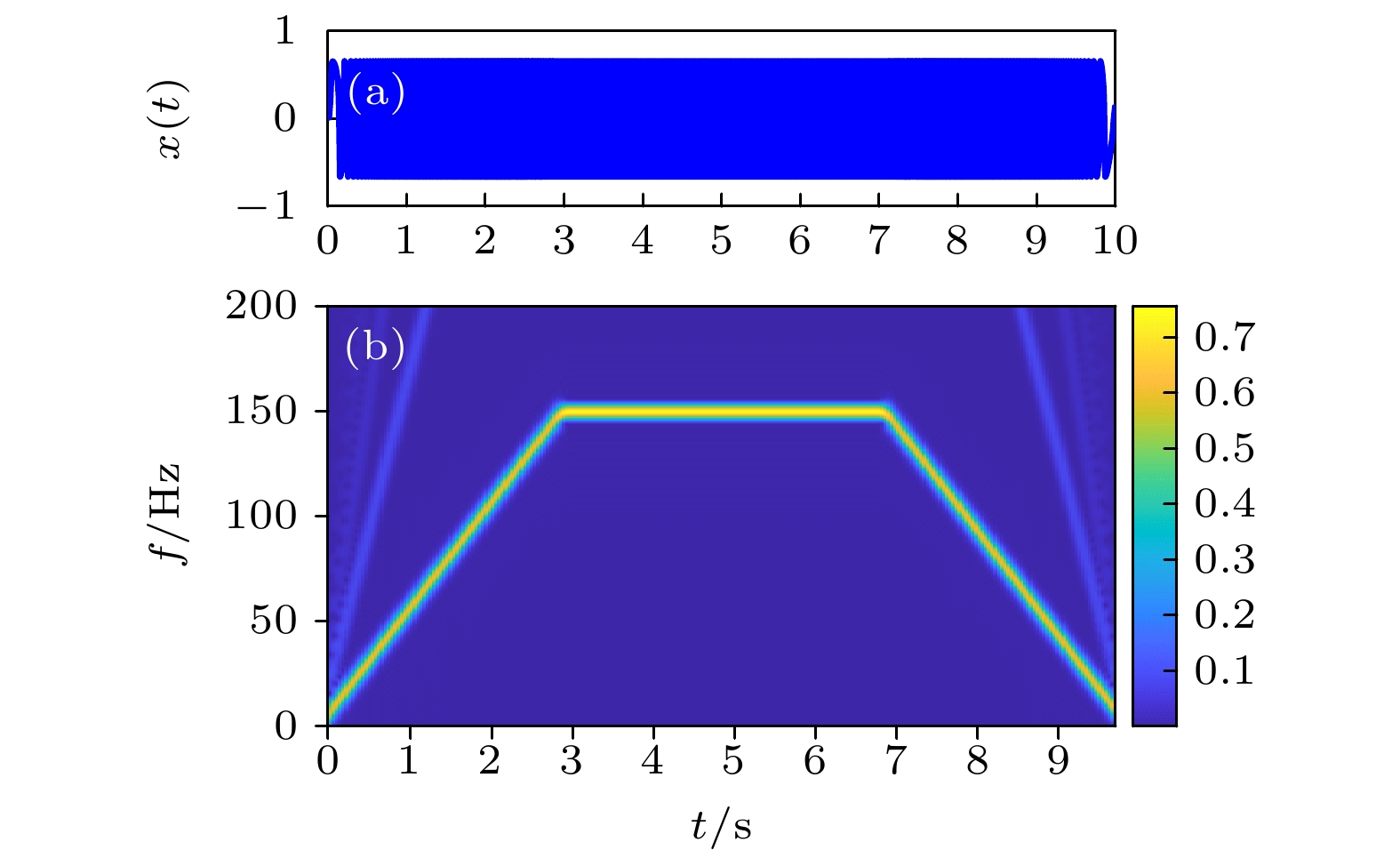
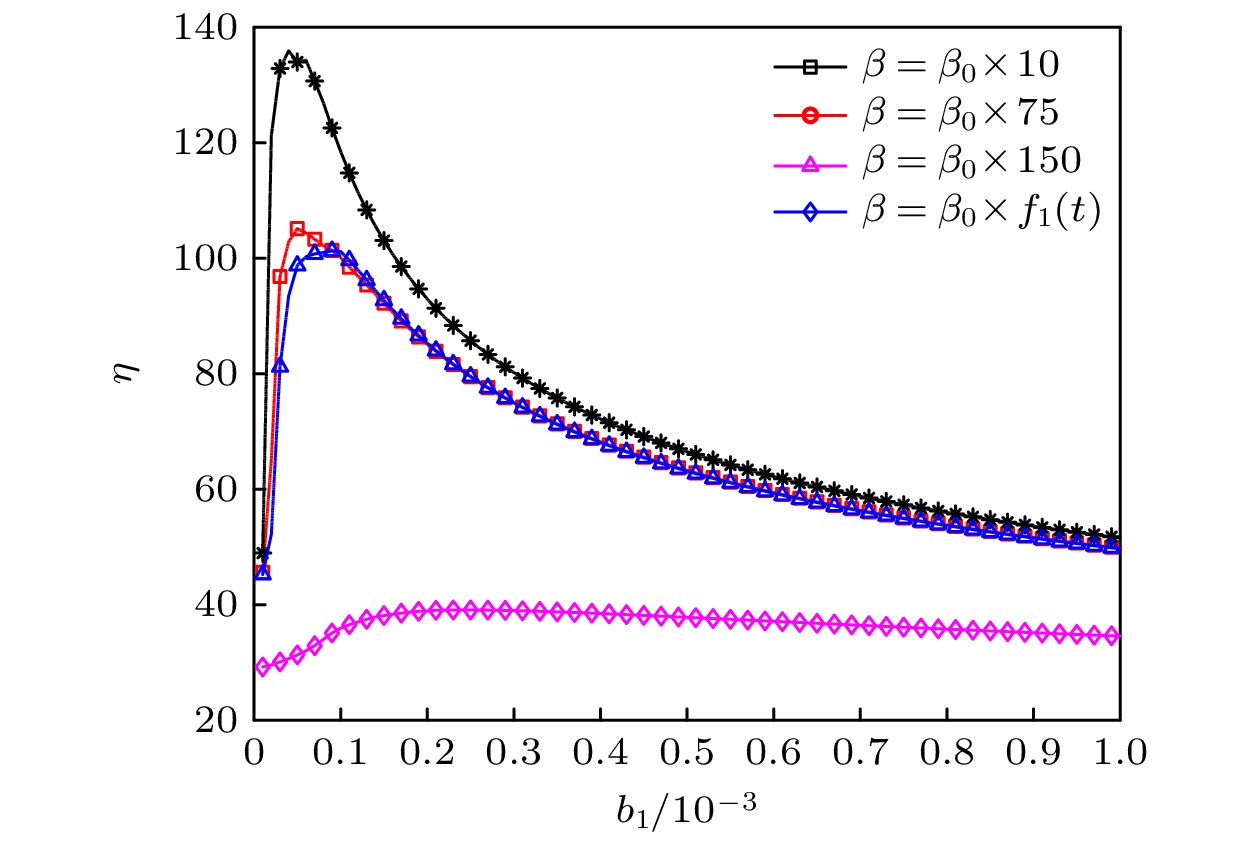
Nonlinear frequency modulation (NLFM) signal is widely used in radar, communication and signal processing. The response of nonlinear system excited by this kind of signal has rich information. At the same time, enhancing different types of signals by resonance phenomenon has unique advantages in the field of signal processing. Compared with other signal processing methods, such as empirical mode decomposition, variational mode decomposition, wavelet transform, signal filtering, etc., this kind of method can not only enhance the signal, but also effectively suppress the interference noise. Therefore, it has certain significance to study the nonlinear system optimal response excited by different NLFM signals and enhance the NLFM signal through resonance phenomenon. In this paper, what is mainly studied is the nonlinear system resonance phenomenon excited by different NLFM signals, which is different from in previous studies. Firstly, a real-time scale transformation method is proposed to process the NLFM signals, and its basic principle is to match different NLFM signals by real-time scale coefficients and system parameters. The signal frequency at each time corresponds to the coefficients with different scales and system parameters, thereby realizing the optimal resonance response at each time. In order to describe the optimal resonance response excited by the NLFM signal more accurately, unlike the traditional spectral amplification factor, the real-time spectral amplification factor is introduced as an evaluation index. Then, the influence of system parameters on the optimal system resonance response is discussed, and the optimal resonance region is obtained, which means that the optimal resonance response can be achieved by selecting the parameters in a reasonable range. This method not only greatly enhances the signal characteristics, but also maintains the continuity of signal time-frequency characteristics. Finally, the real-time scale transformation method is compared with the general scale transformation method, showing the superiority of the proposed method in processing NLFM signal. The method and the results of this paper show some potential in dealing with complex NLFM, which provides a reference for NLFM signal enhancement and detection, and has a certain practical significance in signal enhancement. Furthermore, the relevant influence law of the system optimal response excited by the NLFM signal is given, which has a certain reference value for studying the system dynamic behavior under different signal excitations.

Keywords:

Authors and contacts

Jiangsu Key Laboratory of Mine Mechanical and Electrical Equipment, School of Mechatronic Engineering, China University of Mining and Technology, Xuzhou 221116, China

Corresponding author: Yang Jian-Hua, jianhuayang@cumt.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12072362) and the Priority Academic Program Development of Jiangsu Higher Education Institutions, China.

FullText HTML

References

[1]	Gammaitoni L, Hänggi P, Jung P, Marchesoni F 1998 Rev. Mod. Phys. 70 223 Google Scholar
[2]	Landa P S, McClintock P V E 2000 J. Phys. A Math. Theor. 33 L433
[3]	Benzi R, Sutera A, Vulpiani A 1981 J. Phys. A Math. Theor. 14 L453
[4]	王珊, 王辅忠 2018 67 160502 Google Scholar Wang S, Wang F Z 2018 Acta Phys. Sin. 67 160502 Google Scholar
[5]	Qiao Z, Lei Y, Li N 2019 Mech. Syst. Signal. Process. 122 502 Google Scholar
[6]	Lu S, He Q, Wang J 2019 Mech. Syst. Signal. Process. 116 230 Google Scholar
[7]	冷永刚, 王太勇 2003 52 2432 Google Scholar Leng Y G, Wang T Y 2003 Acta Phys. Sin. 52 2432 Google Scholar
[8]	Tan J, Chen X, Wang J, Chen H, Cao H, Zi Y, He Z 2009 Mech. Syst. Signal. Process. 23 811 Google Scholar
[9]	Hu N, Chen M, Qin G, Xia L, Pan Z, Feng Z 2009 Front. Mech. Eng. 4 450 Google Scholar
[10]	Huang D, Yang J, Zhang J, Liu H 2018 Int. J. Mod. Phys. B 32 1850185 Google Scholar
[11]	Wu C, Yang J, Huang D, Liu H, Hu E 2019 Meas. Sci. Technol. 30 035004 Google Scholar
[12]	Alsalah A, Holloway D, Mousavi M, Lavroff J 2021 Mech. Syst. Signal. Process. 151 107385 Google Scholar
[13]	Kumar A, Zhou Y, Xiang J 2021 Measurement 168 108402 Google Scholar
[14]	Sakar C O, Serbes G, Gunduz A, Tunc H C, Nizam H, Sakar B E, Apaydin H 2019 Appl. Soft Comput. 74 255 Google Scholar
[15]	Wang G, Peng B, Feng Z, Yang X, Deng J, Wang N 2021 Signal Process. 179 107836 Google Scholar
[16]	Li Z, Chen B, Sun H, Liu G, Zhu S 2021 Chin. Phys. B 30 080502 Google Scholar
[17]	Chizhevsky V N, Giacomelli G 2008 Phys. Rev. E 77 051126 Google Scholar
[18]	Zeng L, Li J, Shi J 2012 Chaos Solitons Fract. 45 378 Google Scholar
[19]	彭皓, 钟苏川, 屠浙, 马洪 2013 62 080501 Google Scholar Peng H, Zhong S C, Tu Z, Ma H 2013 Acta Phys. Sin. 62 080501 Google Scholar
[20]	Yang J, Zhang S, Sanjuán M A F, Liu H 2020 Commun. Nonlinear Sci. Numer. Simul. 85 105258 Google Scholar
[21]	屈奎, 张荣福, 肖鹏程 2021 70 198402 Google Scholar Qu K, Zhang R F, Xiao P C 2021 Acta Phys. Sin. 70 198402 Google Scholar
[22]	Daskalakis S N, Kimionis J, Collado A, Goussetis G, Tentzeris M M, Georgiadis A 2017 IEEE Trans. Microw. Theory Tech. 65 5251 Google Scholar
[23]	Zhu D, Gao Q, Lu Y, Sun D 2020 Digit. Signal Process. 107 102860 Google Scholar
[24]	Alphonse S, Williamson G A 2021 IEEE T. Aero. Elec. Sys. 57 1793 Google Scholar
[25]	Song J, Gao Y, Gao D 2015 J. Commun. 10 976
[26]	Vizitiu I C 2014 Prog. Electromagn. Res. C 47 119
[27]	Kim Y, Park J, Na K, Yuan H, Youn B D, Kang C S 2020 Mech. Syst. Signal Process. 138 106544 Google Scholar
[28]	Li Y, Xu F 2021 Struct. Health. Monit. 14759217211033627
[29]	Iatsenko D, McClintock P V E, Stefanovska A 2016 Signal Process. 125 290 Google Scholar

Cited By

图 1 输入的NLFM信号　(a)时域波形图; (b) STFT频谱图 (仿真参数A = 0.1, f₀ = 0, γ = 50, $\phi $= 0, t₁ = 3, t₂ = 10)

Figure 1. The input NLFM signal: (a) The time domain waveform; (b) the STFT spectrum. The simulation parameters are A = 0.1, f₀ = 0, γ = 50, $\phi $= 0, t₁ = 3, t₂ = 10.

DownLoad: Full-Size Img PowerPoint

图 2 不同尺度系数β = β₀ × 10, β₀ × 75, β₀ × 150, β = β₀ ×f₁(t)下的系统参数a₁与实时谱放大因子η的关系图 (仿真参数为b₁ = 1, β₀ = 100, m = 2)

Figure 2. Curves of η-a₁ are obtained under different scale coefficient β = β₀ × 10, β₀ × 75, β₀ × 150, β = β₀ ×f₁(t). The simulation parameters are b₁ = 1, β₀ = 100, m = 2.

DownLoad: Full-Size Img PowerPoint

图 3 实时谱放大因子η与系统参数a₁, b₁的关系图 (仿真参数为m = 2, β₀ = 100)

Figure 3. Contour plot of the real time spectral amplification factor η in the a₁ - b₁ plane. The simulation parameters are m = 2, β₀ = 100.

DownLoad: Full-Size Img PowerPoint

图 4 b₁ = 1时系统的共振输出结果　(a) 时域波形图; (b) STFT频谱图(仿真参数为m = 2, β₀ = 100)

Figure 4. The output response of system resonance when b₁ = 1: (a) The time domain waveform; (b) the STFT spectrum. The simulation parameters are m = 2, β₀ = 100.

DownLoad: Full-Size Img PowerPoint

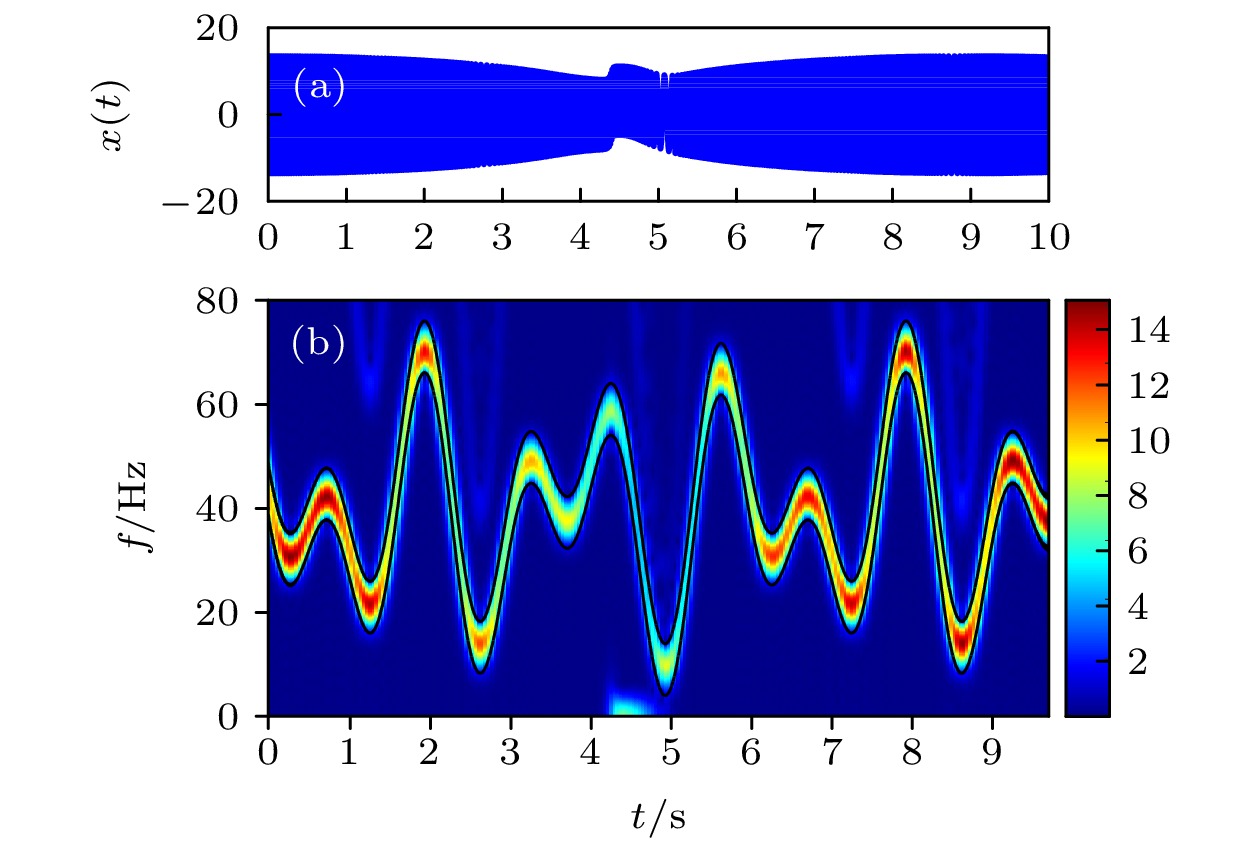
图 5 不同的固定尺度系数β下的系统共振输出结果　(a) β = β₀ × 10下系统输出STFT谱图; (b) β = β₀ ×10下系统输出时域波形图; (c) β = β₀ × 75下系统输出STFT谱图; (d) β = β₀ × 75下时域波形图; (e) β = β₀ ×150下系统输出STFT谱图; (f) β = β₀ ×150下时域波形图 (仿真参数为β₀ = 100)

Figure 5. The output response of system resonance under different fixed scale coefficients β: (a) The output STFT spectrum under β = β₀ × 10; (b) the output time domain waveform under β = β₀ × 10; (c) the output STFT spectrum under β = β₀ × 75; (d) the output time domain waveform under β = β₀ × 75; (e) the output STFT spectrum under β = β₀ × 150; (f) the output time domain waveform under β = β₀ × 150. The simulation parameters are β₀= 100

DownLoad: Full-Size Img PowerPoint

图 6 输入的NLFM信号　(a) 时域波形图; (b) STFT时频图

Figure 6. The input NLFM signal: (a) The time domain waveform; (b) the STFT spectrum.

DownLoad: Full-Size Img PowerPoint

图 7 不同尺度系数下的实时谱放大因子η与系统参数b₁关系图 (仿真参数为a₁ = 0.01, m = 5, β₀ = 1000)

Figure 7. Curves of η-b₁ are obtained under different scale coefficients. The simulation parameters are a₁ = 0.01, m = 5, β₀ = 1000.

DownLoad: Full-Size Img PowerPoint

图 8 时变尺度系数下最优共振输出结果　(a) 时域波形图; (b) STFT频谱图 (仿真参数为m = 5, β₀ = 1000)

Figure 8. The output response of optimal resonance: (a) The time domain waveform; (b) the STFT spectrum. The simulation parameters are m = 5, β₀ = 1000.

DownLoad: Full-Size Img PowerPoint

图 9 不同固定尺度系数β下共振响应　(a) β = β₀ × 10下输出STFT谱图; (b) β = β₀ × 10下输出时域波形图; (c) β = β₀ × 40下输出STFT谱图; (d) β = β₀ × 40下输出时域波形图; (e) β = β₀ × 75下输出STFT谱图; (f) β = β₀ × 75下输出时域波形图

Figure 9. The resonance response under different fixed scale coefficient β: (a) The output STFT spectrum under β = β₀ × 10; (b) the output time domain waveform under β = β₀ × 10; (c) the output STFT spectrum under β = β₀ × 40; (d) the output time domain waveform under β = β₀ × 40; (e) the output STFT spectrum under β = β₀ × 75; (f) the output time domain waveform under β = β₀ × 75.

DownLoad: Full-Size Img PowerPoint

map

[1]	Gammaitoni L, Hänggi P, Jung P, Marchesoni F 1998 Rev. Mod. Phys. 70 223 Google Scholar
[2]	Landa P S, McClintock P V E 2000 J. Phys. A Math. Theor. 33 L433
[3]	Benzi R, Sutera A, Vulpiani A 1981 J. Phys. A Math. Theor. 14 L453
[4]	王珊, 王辅忠 2018 67 160502 Google Scholar Wang S, Wang F Z 2018 Acta Phys. Sin. 67 160502 Google Scholar
[5]	Qiao Z, Lei Y, Li N 2019 Mech. Syst. Signal. Process. 122 502 Google Scholar
[6]	Lu S, He Q, Wang J 2019 Mech. Syst. Signal. Process. 116 230 Google Scholar
[7]	冷永刚, 王太勇 2003 52 2432 Google Scholar Leng Y G, Wang T Y 2003 Acta Phys. Sin. 52 2432 Google Scholar
[8]	Tan J, Chen X, Wang J, Chen H, Cao H, Zi Y, He Z 2009 Mech. Syst. Signal. Process. 23 811 Google Scholar
[9]	Hu N, Chen M, Qin G, Xia L, Pan Z, Feng Z 2009 Front. Mech. Eng. 4 450 Google Scholar
[10]	Huang D, Yang J, Zhang J, Liu H 2018 Int. J. Mod. Phys. B 32 1850185 Google Scholar
[11]	Wu C, Yang J, Huang D, Liu H, Hu E 2019 Meas. Sci. Technol. 30 035004 Google Scholar
[12]	Alsalah A, Holloway D, Mousavi M, Lavroff J 2021 Mech. Syst. Signal. Process. 151 107385 Google Scholar
[13]	Kumar A, Zhou Y, Xiang J 2021 Measurement 168 108402 Google Scholar
[14]	Sakar C O, Serbes G, Gunduz A, Tunc H C, Nizam H, Sakar B E, Apaydin H 2019 Appl. Soft Comput. 74 255 Google Scholar
[15]	Wang G, Peng B, Feng Z, Yang X, Deng J, Wang N 2021 Signal Process. 179 107836 Google Scholar
[16]	Li Z, Chen B, Sun H, Liu G, Zhu S 2021 Chin. Phys. B 30 080502 Google Scholar
[17]	Chizhevsky V N, Giacomelli G 2008 Phys. Rev. E 77 051126 Google Scholar
[18]	Zeng L, Li J, Shi J 2012 Chaos Solitons Fract. 45 378 Google Scholar
[19]	彭皓, 钟苏川, 屠浙, 马洪 2013 62 080501 Google Scholar Peng H, Zhong S C, Tu Z, Ma H 2013 Acta Phys. Sin. 62 080501 Google Scholar
[20]	Yang J, Zhang S, Sanjuán M A F, Liu H 2020 Commun. Nonlinear Sci. Numer. Simul. 85 105258 Google Scholar
[21]	屈奎, 张荣福, 肖鹏程 2021 70 198402 Google Scholar Qu K, Zhang R F, Xiao P C 2021 Acta Phys. Sin. 70 198402 Google Scholar
[22]	Daskalakis S N, Kimionis J, Collado A, Goussetis G, Tentzeris M M, Georgiadis A 2017 IEEE Trans. Microw. Theory Tech. 65 5251 Google Scholar
[23]	Zhu D, Gao Q, Lu Y, Sun D 2020 Digit. Signal Process. 107 102860 Google Scholar
[24]	Alphonse S, Williamson G A 2021 IEEE T. Aero. Elec. Sys. 57 1793 Google Scholar
[25]	Song J, Gao Y, Gao D 2015 J. Commun. 10 976
[26]	Vizitiu I C 2014 Prog. Electromagn. Res. C 47 119
[27]	Kim Y, Park J, Na K, Yuan H, Youn B D, Kang C S 2020 Mech. Syst. Signal Process. 138 106544 Google Scholar
[28]	Li Y, Xu F 2021 Struct. Health. Monit. 14759217211033627
[29]	Iatsenko D, McClintock P V E, Stefanovska A 2016 Signal Process. 125 290 Google Scholar

[1]	Li Hao, Pang Yong-Qiang, Qu Bing-Yue, Zheng Jiang-Shan, Xu Zhuo. Optical transparent metasurface lenses and their wireless communication efficiency enhancement. Acta Physica Sinica, 2024, 73(14): 144104. doi: 10.7498/aps.73.20240464
[2]	Wang Zhong-Qiu, Yang Jian-Hua. Aperiodic resonance of a nonlinear system excited by aperiodic binary signal or M-ary signal. Acta Physica Sinica, 2023, 72(22): 222501. doi: 10.7498/aps.72.20231154
[3]	Zhou Pei, Zhang Ren-Heng, Zhu Jian, Li Nian-Qiang. High-performance linear frequency-modulated signal generation based on optically injected semiconductor laser with dual-loop optoelectronic feedback. Acta Physica Sinica, 2022, 71(21): 214204. doi: 10.7498/aps.71.20221308
[4]	Zhang Mei-Mei, Gao Fan, Tu Juan, Wu Yi-Yun, Zhang Dong. Classification of benign and malignant breast masses using entropy from nonlinear ultrasound radiofrequency signal. Acta Physica Sinica, 2021, 70(8): 084302. doi: 10.7498/aps.70.20201919
[5]	. Study the optimal resonance response of nonlinear system excited by NLFM signal. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211959
[6]	Liu Guang-Kai, Quan Hou-De, Kang Yan-Mei, Sun Hui-Xian, Cui Pei-Zhang, Han Yue-Ming. A quadratic polynomial receiving scheme for sine signals enhanced by stochastic resonance. Acta Physica Sinica, 2019, 68(21): 210501. doi: 10.7498/aps.68.20190952
[7]	Cheng Jian, Feng Jin-Xia, Li Yuan-Ji, Zhang Kuan-Shou. Measurement of low-frequency signal based on quantum-enhanced fiber Mach-Zehnder interferometer. Acta Physica Sinica, 2018, 67(24): 244202. doi: 10.7498/aps.67.20181335
[8]	Lu Wen-Long, Xie Jun-Wei, Wang He-Ming, Sheng Chuan. Signal component extraction method based on polynomial chirp Fourier transform. Acta Physica Sinica, 2016, 65(8): 080202. doi: 10.7498/aps.65.080202
[9]	Zhang Lu, Xie Tian-Ting, Luo Mao-Kang. Vibrational resonance in a Duffing system with fractional-order external and intrinsic dampings driven by the two-frequency signals. Acta Physica Sinica, 2014, 63(1): 010506. doi: 10.7498/aps.63.010506
[10]	Peng Hao, Zhong Su-Chuan, Tu Zhe, Ma Hong. Stochastic resonance of over-damped bistable system driven by chirp signal and Gaussian white noise. Acta Physica Sinica, 2013, 62(8): 080501. doi: 10.7498/aps.62.080501
[11]	Jiang Fei, Liu Zhong, Hu Wen, Bao Bo-Cheng. Dynamical design and analysis of continuous chaos frequency modulation signal. Acta Physica Sinica, 2010, 59(1): 116-122. doi: 10.7498/aps.59.116
[12]	Ning Li-Juan, Xu Wei. Stochastic resonance under modulated noise in linear systems driven by dichotomous noise. Acta Physica Sinica, 2009, 58(5): 2889-2894. doi: 10.7498/aps.58.2889
[13]	Lin Min, Fang Li-Min, Zhu Ruo-Gu. The dual-resonance characteristic of coupled bistable system affected by two-frequency signal. Acta Physica Sinica, 2008, 57(5): 2638-2642. doi: 10.7498/aps.57.2638
[14]	Zhang Liang-Ying, Jin Guo-Xiang, Cao Li. Stochastic resonance of frequency modulated signals in a linear model of single-mode laser. Acta Physica Sinica, 2008, 57(8): 4706-4711. doi: 10.7498/aps.57.4706
[15]	Du Dan, Hu Xiang-Ming. Enhancement of nonlinear-optical signals in a cascade three-level system. Acta Physica Sinica, 2006, 55(10): 5232-5236. doi: 10.7498/aps.55.5232
[16]	Xu Wei, Jin Yan-Fei, Xu Meng, Li Wei. Stochastic resonance for bias-signal-modulated noise in a linear system. Acta Physica Sinica, 2005, 54(11): 5027-5033. doi: 10.7498/aps.54.5027
[17]	Zhang Jia-Shu, Li Heng-Chao, Xiao Xian-Ci. A DCT domain quadratic predictor for real-time prediction of continuous chaotic signals. Acta Physica Sinica, 2004, 53(3): 710-716. doi: 10.7498/aps.53.710
[18]	Zhou Xin, Luo Jun, Sun Xian-Ping, Zeng Xi-Zhi, Liu Mai-Li, Liu Wu-Yang. . Acta Physica Sinica, 2002, 51(10): 2221-2224. doi: 10.7498/aps.51.2221
[19]	Peng Jian-Hua, Tian Xiao-Jian, Wang Pei-Jin, Chen Xiao-Bing. . Acta Physica Sinica, 1995, 44(2): 177-183. doi: 10.7498/aps.44.177
[20]	MIAO RUN-CAI, PAN DUO-HAI, CHEN FA-TANG, ZHANG XIN-HUI. INFLUENCE OF HALOGEN FAMILY ANIONS ON SERS SIGNAL IN Ag SURFACE-MOLECULE SYSTEM. Acta Physica Sinica, 1992, 41(9): 1542-1546. doi: 10.7498/aps.41.1542

Catalog

Vol. 71, Issue 5 - 2022-03-05

Metrics

Abstract views: 5649
PDF Downloads: 112
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板