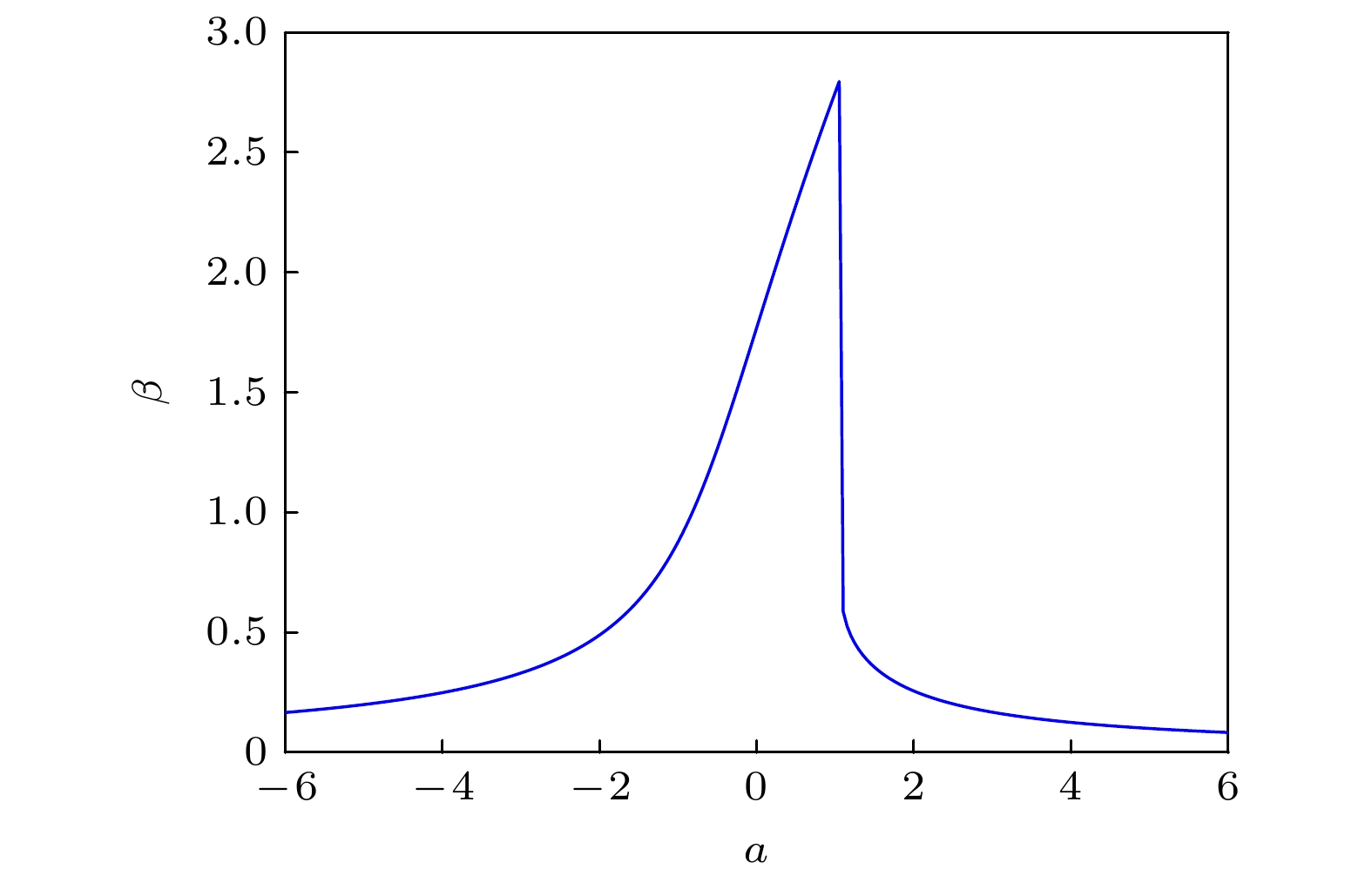
-
研究单一非周期二进制或M进制信号激励下一类非线性系统的非周期共振现象及其度量方法, 重点探讨了系统参数引起的非周期共振. 提出了适用于非周期共振度量的响应幅值增益指标, 并结合互相关系数和误码率展开研究. 结果发现, 互相关系数能够较好地描述系统输出和输入信号之间的同步性及波形相似性但无法刻画信号通过系统后被放大的程度. 响应幅值增益能够较好地描述信号通过系统后幅值被放大的程度, 但无法反映系统输出和输入信号之间的同步性及波形相似性. 非周期共振发生在互相关系数取谷值和响应幅值增益取峰值处, 且两种指标曲线反映的共振点相同. 误码率在合适的阈值下可以描述系统输出和输入信号之间的同步性以及非周期信号通过系统后被放大的程度, 误码率曲线可以直接给出非周期共振的共振区. 单一非周期二进制或M进制信号激励下的非线性系统可以发生非周期共振, 其共振效果需要综合互相关系数、响应幅值增益、误码率等指标进行度量.The aperiodic resonance of a typical nonlinear system that excited by a single aperiodic binary or M-ary signal and its measuring method are studied. The focus is on exploring aperiodic resonance caused by the system parameter. A response amplitude gain index suitable for aperiodic excitation is proposed to measure the effect of aperiodic resonance, and the research is carried out by combining the cross-correlation coefficient index and bit error rate index. The results show that the cross-correlation coefficient can better describe the synchronization and waveform similarity between the system output and the input aperiodic signal, but cannot describe the situation whether the signal is amplified after passing through the nonlinear system. The response amplitude gain can better describe the amplification of signal amplitude after passing through the nonlinear system, but cannot reflect the synchronization and waveform similarity between the system output and the input aperiodic signal. The aperiodic resonance occurs at the valley corresponding to the cross-correlation coefficient and the peak corresponding the response amplitude gain. The aperiodic resonance locations reflected on both the cross-correlation coefficient and the response amplitude gain curves are the same. The bit error rate can describe the synchronization between the system output and the input signal at appropriate thresholds, as well as the degree to which the aperiodic signal is amplified after passing through the nonlinear system. The bit error rate curve can directly indicate the resonance region of the aperiodic resonance. The aperiodic resonance can occur in a nonlinear system excited by a single aperiodic binary or M-ary signal, and its aperiodic resonance effect needs to be measured by combining the cross-correlation coefficient, response amplitude gain, bit error rate and other indices together.
-
Keywords:
- aperiodic excitation /
- binary signal /
- M-ary signal /
- aperiodic resonance /
- nonlinear system
[1] Lee H, Lee I, Quek T Q, Lee S H 2018 Opt. Express 26 18131
Google Scholar
[2] Chizhevsky V N, Smeu E, Giacomelli G 2003 Phys. Rev. Lett. 91 220602
Google Scholar
[3] Chizhevsky V N, Giacomelli G 2008 Phys. Rev. E 77 051126
Google Scholar
[4] Liu J, Li Z 2015 IET Image Process. 9 1033
Google Scholar
[5] Chen W, Chen X 2015 Europhys. Lett. 110 44002
Google Scholar
[6] Gammaitoni L, Hänggi P, Jung P, Marchesoni F 1998 Rev. Mod. Phys. 70 223
Google Scholar
[7] Xu W, Jin Y F, Li W, Ma S J 2005 Chin. Phys. 14 1077
Google Scholar
[8] Jin Y F 2018 Chin. Phys. B 27 050501
Google Scholar
[9] 靳艳飞, 许鹏飞, 李永歌, 马晋忠, 许勇 2023 力学进展 53 357
Google Scholar
Jin Y F, Xu P F, Li Y G, Ma J Z, Xu Y 2023 Advances in Mechanics 53 357
Google Scholar
[10] 杨建华, 周登极 2020 变尺度共振理论及在故障诊断中的应用 (北京: 科学出版社)
Yang J H, Zhou D J 2020 Re-scaled Resonance Theory and Applications in Fault Diagnosis (Beijing: Science Press
[11] Landa P S, McClintock P V E 2000 J. Phys. A: Math. Gen. 33 L433
Google Scholar
[12] Rajasekar S, Miguel A F, Sanjuán 2016 Nonlinear Resonances (Switzerland: Springer International Publishing
[13] Vincent U E, McClintock P V E, Khovanov I A, Rajasekar S 2021 Philos. T. R. Soc. A 379 20200226
Google Scholar
[14] Collins J J, Chow C C, Capela A C, Imhoff T T 1996 Phys. Rev. E 54 5575
Google Scholar
[15] Collins J J, Chow C C, Imhoff T T 1995 Phys. Rev. E 52 R3321
Google Scholar
[16] Heneghan C, Chow C C, Collins J J, Imhoff T T, Lowen S B, Teich M C 1996 Phys. Rev. E 54 R2228
Google Scholar
[17] Jia P X, Wu C J, Yang J H, Sanjuán M A F, Liu G X 2018 Nonlinear Dynam. 91 2699
Google Scholar
[18] 杨建华, 马强, 吴呈锦, 刘后广 2018 67 054501
Google Scholar
Yang J H, Ma Q, Wu C J, Liu H G 2018 Acta Phys. Sin. 67 054501
Google Scholar
[19] Duan L, Duan F, Chapeau-Blondeau F, Abbott D 2020 Phys. Lett. A 384 126143
Google Scholar
[20] Kang Y, Liu R, Mao X 2021 Cogn. Neurodynamics 15 517
Google Scholar
[21] Zhao J, Ma Y, Pan Z, Zhang H 2022 J. Syst. Sci. Complex. 35 179
Google Scholar
[22] Huang W, Zhang G, Jiao S, Wang J 2022 Appl. Sci. 12 12084
Google Scholar
[23] Zeng L, Li J, Shi J 2012 Chaos, Soliton. Fract. 45 378
Google Scholar
[24] Liang L, Zhang N, Huang H, Li Z 2019 China Commun. 16 196
Google Scholar
[25] McDonnell M D, Gao X 2015 Europhys. Lett. 108 60003
Google Scholar
[26] Cheng C, Zhou B, Gao X, McDonnell M D 2017 Physica A 479 48
Google Scholar
[27] Yang C, Yang J, Zhou D, Zhang S, Litak G 2021 Philos. T. R. Soc. A 379 20200239
Google Scholar
[28] Qin T, Zhou L, Chen S, Chen Z 2022 IEEE Sens. J. 22 17043
Google Scholar
[29] Xu Z, Wang Z, Yang J, Sanjuán M A F, Sun B, Huang S 2023 Eur. Phys. J. Plus 138 386
Google Scholar
[30] Chizhevsky V N 2014 Phys. Rev. E 89 062914
Google Scholar
[31] Morfu S, Usama B I, Marquié P 2019 Electron. Lett. 55 650
Google Scholar
[32] Morfu S, Usama B I, Marquié P 2021 Philos. T. R. Soc. A 379 20200240
Google Scholar
[33] Zhang S, Yang J, Zhang J, Liu H, Hu E 2019 Nonlinear Dynam. 98 2035
Google Scholar
[34] Zhai Y, Yang J, Zhang S, Liu H 2020 Phys. Scripta 95 065213
Google Scholar
[35] Yang J, Zhang S, Sanjuán M A F, Liu H 2020 Commun. Nonlinear Sci. Numer. Simulat. 85 105258
Google Scholar
[36] Jeevarathinam C, Rajasekar S, Sanjuán M A F 2011 Phys. Rev. E 83 066205
Google Scholar
[37] Li J L, Zeng L Z 2011 Chin. Phys. B 20 010503
Google Scholar
-
图 1 A = 0.3, T = 100时, 非周期二进制信号的不同时域波形. 自上而下信号分别标记为Signal 1, Signal 2, Signal 3
Fig. 1. Different waveforms of the aperiodic binary signal at A = 0.3, T = 100. The signals are labeled as Signal 1, Signal 2, Signal 3 from top to bottom.
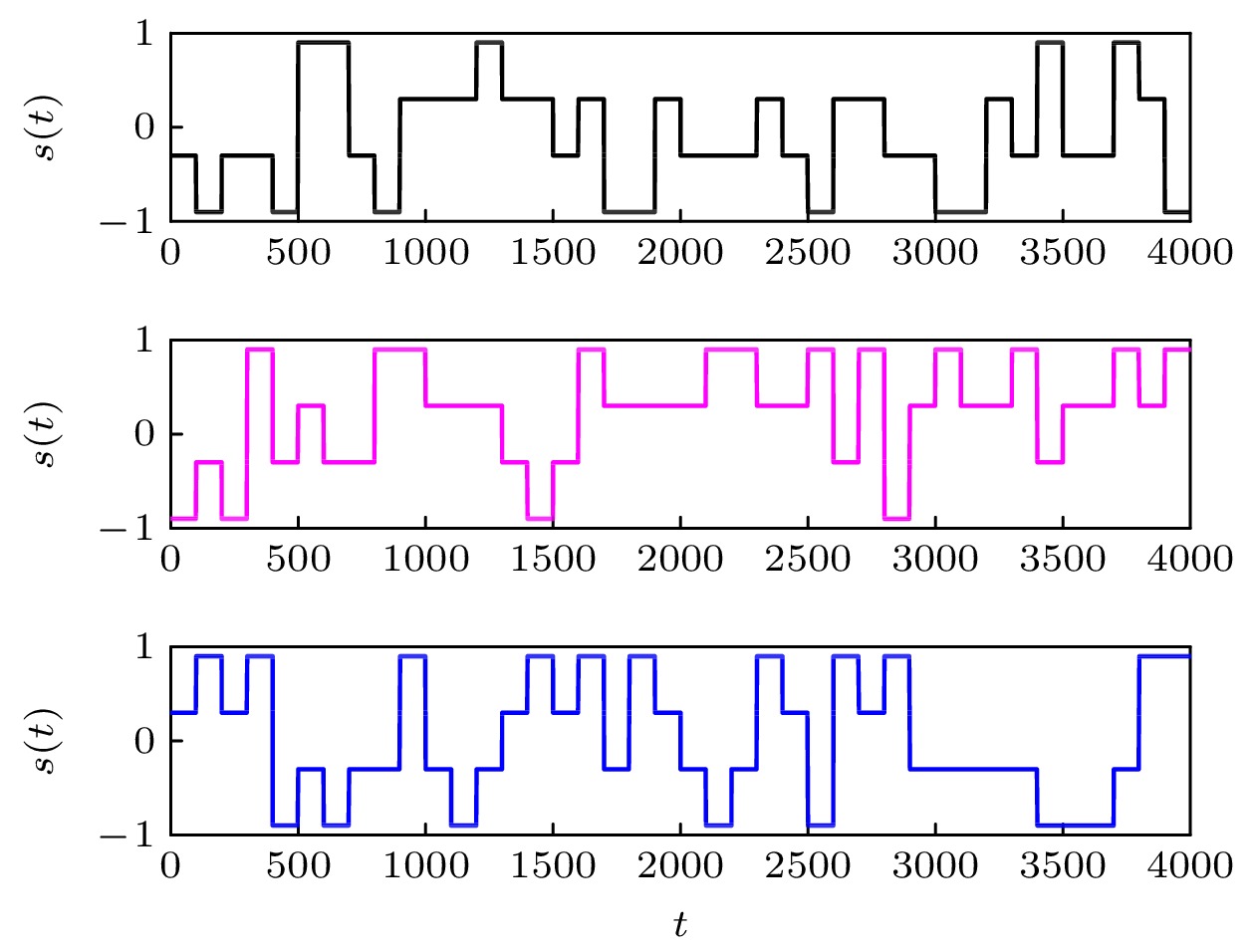
图 2 b = 2, A = 0.3, T = 100时, 不同波形的非周期二进制信号激励下, 系统输出与输入信号之间的互相关系数
$ C_{sx} $ 与系统参数a之间的关系Fig. 2. Cross-correlation coefficient
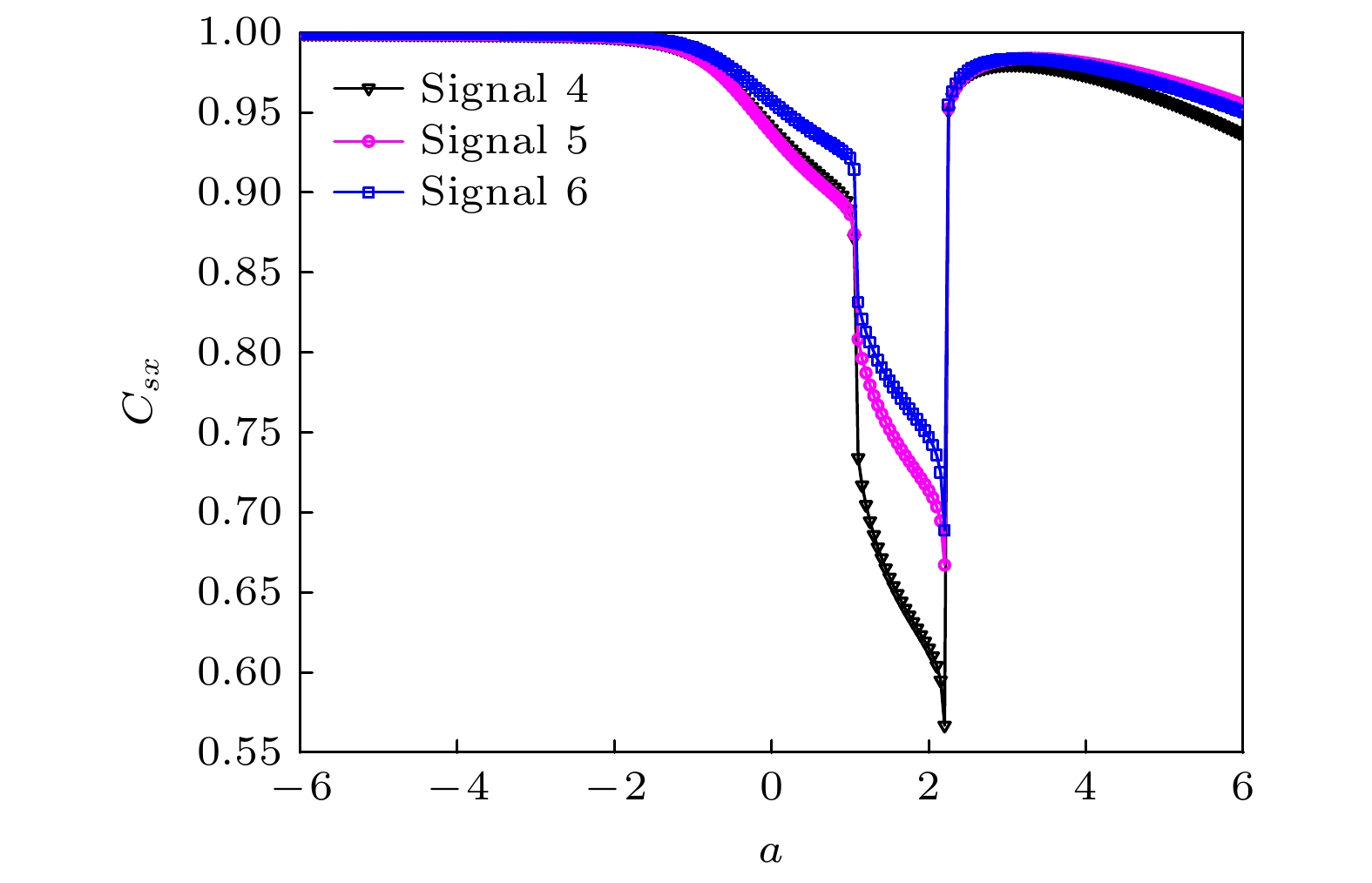
$ C_{sx} $ between the system output and input signal versus the system parameter a under the excitation of aperiodic binary signal with different waveforms at b = 2, A = 0.3, T = 100.
图 3 b = 2, A = 0.3, T = 100时, 系统参数a取值不同时输出的不同波形 (a)
$ a=0 $ ; (b)$ a=1.05 $ ; (c)$ a=1.1 $ ; (d)$ a=2 $ Fig. 3. Different waveforms of the output under different values of the system parameter a with b = 2, A = 0.3, T = 100: (a)
$ a=0 $ ; (b)$ a=1.05 $ ; (c)$ a=1.1 $ ; (d)$ a=2 $ 
图 4 b = 2, A = 0.3, T = 100时, 响应幅值增益β与系统参数a之间的函数关系
Fig. 4. Response amplitude gain β versus the system parameter a with b = 2, A = 0.3, T = 100.
图 5 b = 2, A = 0.3, T = 100时, 误码率BER与系统参数a之间的函数关系
Fig. 5. Bit error rate (BER) versus the system parameter a with b = 2, A = 0.3, T = 100
图 6 M = 4, b = 2, A = 0.3, T = 100时, 非周期M进制信号的不同时域波形. 自上而下信号分别标记为Signal 4, Signal 5, Signal 6
Fig. 6. Different waveforms of the aperiodic M-ary signal with M = 4, b = 2, A = 0.3, T = 100. The signals are labeled as Signal 4, Signal 5, Signal 6 from top to bottom
图 7 M = 4, b = 2, A = 0.3, T = 100时, 不同波形的非周期M进制信号激励下, 系统输出与输入信号之间的互相关系数
$ C_{sx} $ 与系统参数a之间的关系Fig. 7. Cross-correlation coefficient
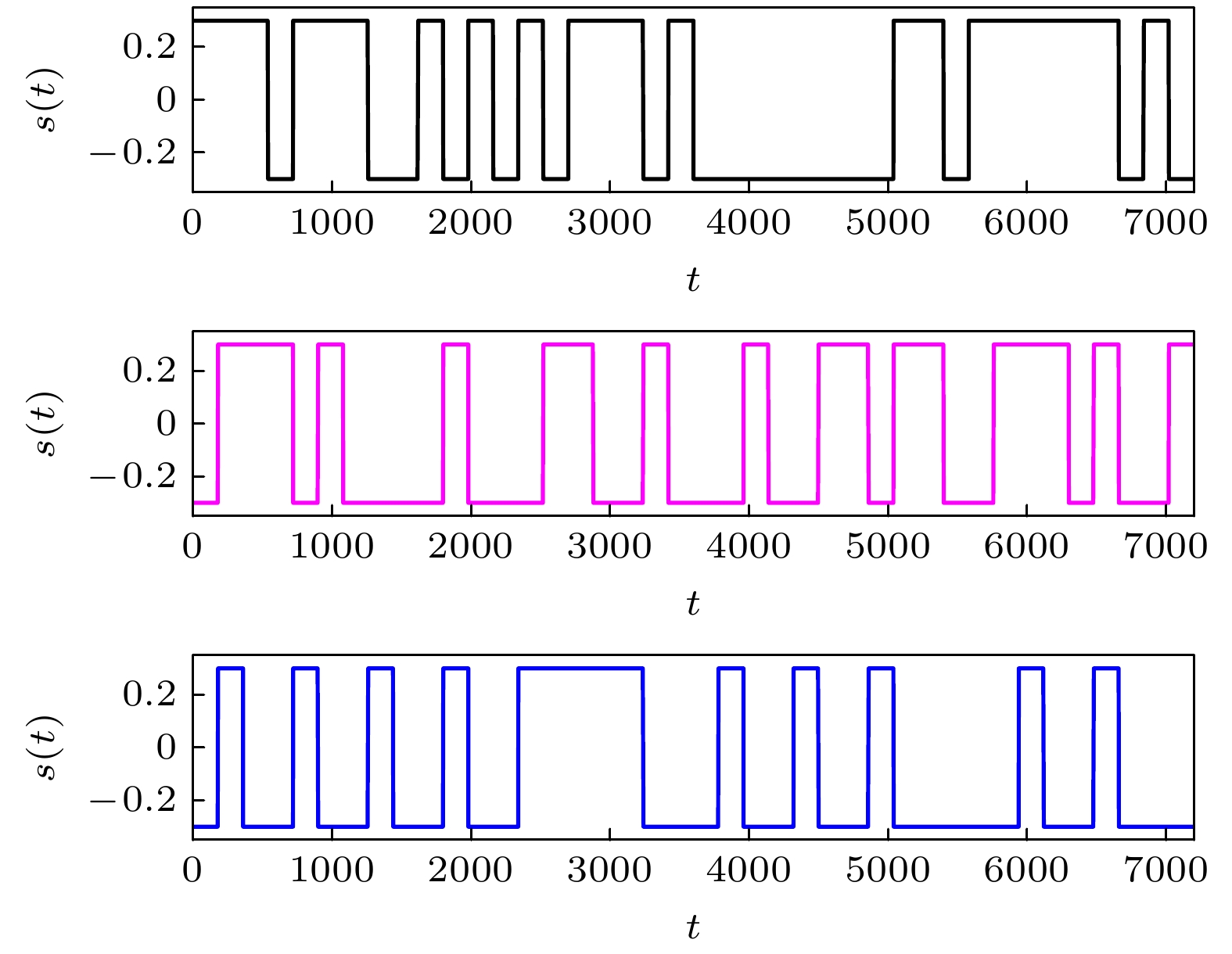
$ C_{sx} $ between the system output and input signal versus the system parameter a under the excitation of aperiodic M-ary signal with different waveforms at M = 4, b = 2, A = 0.3, T = 100.
图 8 b = 2, A = 0.3, T = 100时, 系统参数a取值不同时输出的不同波形 (a)
$ a=0 $ ; (b)$ a=2.2 $ ; (c)$ a=3 $ ; (d)$ a=5 $ Fig. 8. Different waveforms of the output under different values of the system parameter a with b = 2, A = 0.3, T = 100: (a)
$ a=0 $ ; (b)$ a=2.2 $ ; (c)$ a=3 $ ; (d)$ a=5 $ 
图 9 M = 4, b = 2, A = 0.3, T = 100时, 响应幅值增益β与系统参数a之间的函数关系
Fig. 9. Response amplitude gain β versus the system parameter a with M = 4, b = 2, A = 0.3, T = 100.
图 10 M = 4, b = 2, A = 0.3, T = 100时, 误码率BER与系统参数a之间的函数关系
Fig. 10. Bit error rate BER versus the system parameter a with M = 4, b = 2, A = 0.3, T = 100
图 11 M = 4, b = 2, A = 0.3, T = 100时, 系统参数a处于共振区时输出的波形 (a)
$ a=0.65 $ ; (b)$ a=0.75 $ ; (c)$ a= 0.85 $ ; (d)$ a=0.95 $ Fig. 11. Different waveforms of the output when the system parameter a lies in the resonance region with M = 4, b = 2, A = 0.3, T = 100: (a)
$ a=0.65 $ ; (b)$ a=0.75 $ ; (c)$ a=0.85 $ ; (d)$ a=0.95 $ -
[1] Lee H, Lee I, Quek T Q, Lee S H 2018 Opt. Express 26 18131
Google Scholar
[2] Chizhevsky V N, Smeu E, Giacomelli G 2003 Phys. Rev. Lett. 91 220602
Google Scholar
[3] Chizhevsky V N, Giacomelli G 2008 Phys. Rev. E 77 051126
Google Scholar
[4] Liu J, Li Z 2015 IET Image Process. 9 1033
Google Scholar
[5] Chen W, Chen X 2015 Europhys. Lett. 110 44002
Google Scholar
[6] Gammaitoni L, Hänggi P, Jung P, Marchesoni F 1998 Rev. Mod. Phys. 70 223
Google Scholar
[7] Xu W, Jin Y F, Li W, Ma S J 2005 Chin. Phys. 14 1077
Google Scholar
[8] Jin Y F 2018 Chin. Phys. B 27 050501
Google Scholar
[9] 靳艳飞, 许鹏飞, 李永歌, 马晋忠, 许勇 2023 力学进展 53 357
Google Scholar
Jin Y F, Xu P F, Li Y G, Ma J Z, Xu Y 2023 Advances in Mechanics 53 357
Google Scholar
[10] 杨建华, 周登极 2020 变尺度共振理论及在故障诊断中的应用 (北京: 科学出版社)
Yang J H, Zhou D J 2020 Re-scaled Resonance Theory and Applications in Fault Diagnosis (Beijing: Science Press
[11] Landa P S, McClintock P V E 2000 J. Phys. A: Math. Gen. 33 L433
Google Scholar
[12] Rajasekar S, Miguel A F, Sanjuán 2016 Nonlinear Resonances (Switzerland: Springer International Publishing
[13] Vincent U E, McClintock P V E, Khovanov I A, Rajasekar S 2021 Philos. T. R. Soc. A 379 20200226
Google Scholar
[14] Collins J J, Chow C C, Capela A C, Imhoff T T 1996 Phys. Rev. E 54 5575
Google Scholar
[15] Collins J J, Chow C C, Imhoff T T 1995 Phys. Rev. E 52 R3321
Google Scholar
[16] Heneghan C, Chow C C, Collins J J, Imhoff T T, Lowen S B, Teich M C 1996 Phys. Rev. E 54 R2228
Google Scholar
[17] Jia P X, Wu C J, Yang J H, Sanjuán M A F, Liu G X 2018 Nonlinear Dynam. 91 2699
Google Scholar
[18] 杨建华, 马强, 吴呈锦, 刘后广 2018 67 054501
Google Scholar
Yang J H, Ma Q, Wu C J, Liu H G 2018 Acta Phys. Sin. 67 054501
Google Scholar
[19] Duan L, Duan F, Chapeau-Blondeau F, Abbott D 2020 Phys. Lett. A 384 126143
Google Scholar
[20] Kang Y, Liu R, Mao X 2021 Cogn. Neurodynamics 15 517
Google Scholar
[21] Zhao J, Ma Y, Pan Z, Zhang H 2022 J. Syst. Sci. Complex. 35 179
Google Scholar
[22] Huang W, Zhang G, Jiao S, Wang J 2022 Appl. Sci. 12 12084
Google Scholar
[23] Zeng L, Li J, Shi J 2012 Chaos, Soliton. Fract. 45 378
Google Scholar
[24] Liang L, Zhang N, Huang H, Li Z 2019 China Commun. 16 196
Google Scholar
[25] McDonnell M D, Gao X 2015 Europhys. Lett. 108 60003
Google Scholar
[26] Cheng C, Zhou B, Gao X, McDonnell M D 2017 Physica A 479 48
Google Scholar
[27] Yang C, Yang J, Zhou D, Zhang S, Litak G 2021 Philos. T. R. Soc. A 379 20200239
Google Scholar
[28] Qin T, Zhou L, Chen S, Chen Z 2022 IEEE Sens. J. 22 17043
Google Scholar
[29] Xu Z, Wang Z, Yang J, Sanjuán M A F, Sun B, Huang S 2023 Eur. Phys. J. Plus 138 386
Google Scholar
[30] Chizhevsky V N 2014 Phys. Rev. E 89 062914
Google Scholar
[31] Morfu S, Usama B I, Marquié P 2019 Electron. Lett. 55 650
Google Scholar
[32] Morfu S, Usama B I, Marquié P 2021 Philos. T. R. Soc. A 379 20200240
Google Scholar
[33] Zhang S, Yang J, Zhang J, Liu H, Hu E 2019 Nonlinear Dynam. 98 2035
Google Scholar
[34] Zhai Y, Yang J, Zhang S, Liu H 2020 Phys. Scripta 95 065213
Google Scholar
[35] Yang J, Zhang S, Sanjuán M A F, Liu H 2020 Commun. Nonlinear Sci. Numer. Simulat. 85 105258
Google Scholar
[36] Jeevarathinam C, Rajasekar S, Sanjuán M A F 2011 Phys. Rev. E 83 066205
Google Scholar
[37] Li J L, Zeng L Z 2011 Chin. Phys. B 20 010503
Google Scholar
下载:
计量
- 文章访问数: 1906
- PDF下载量: 49
- 被引次数: 0
