-
The ballistic target uses a two-stage light gas gun to launch the model into a hypersonic state, and the model forms a plasma wake when flying at a hypersonic speed in the target chamber. In order to obtain the radial two-dimensional distribution of transient plasma electron density in the wake of hypervelocity model, a seven-channel Ka-band microwave interferometer measuring system is developed. In the transceiver system, a transmitting antenna achieves the plane wave irradiation plasma, and a seven-port array antenna is used to receive plane waves which are passing through the plasma: the antenna beam can completely cover the radial range of the wake. The shortest response time of measuring system is 1 s, and electron density measurement range of the interferometer measuring system is 1011–1013/cm3 . The plasma is often treated as layered medium in data processing of multichannel microwave interferometer. Taking into consideration the effect of refraction on the stratified interface in this work, the ray tracing (RT) method is used to establish the electromagnetic propagation model. Combined with the measurement data to construct the objective function, the genetic algorithm (GA) is used to invert the radial two-dimensional distribution of the electron density under different test conditions. The result shows good agreement with the numerical simulation under the same test state, which proves the the data processing method reliable. Then, the influence of the layered model on the calculation result is analyzed, which shows that the seven-layer model is suitable for the wake modeling under given experimental conditions, and thus maximizing the number of receiving channels and ensuring the accuracy. The RT method is used for the first time to achieve the two-dimensional distribution of electron density in the wake of hypervelocity projectile, and some rules of two-dimensional electron density distribution of the hypersonic model under given experimental conditions are realized.
-
Keywords:
- wake of hypervelocity model /
- seven channels microwave interferometer /
- ray tracing /
- two-dimensional distribution
[1] 于哲峰, 孙良奎, 马平, 杨益兼, 张志成, 黄洁 2017 红外 38 039
Google Scholar
Yu Z F, Sun L K, Ma P, Yang Y J, Zhang Z C, Huang J 2017 Infrared 38 039
Google Scholar
[2] 池凌飞, 林揆训, 姚若河, 林璇英, 余楚迎, 余云鹏 2001 50 1313
Google Scholar
Chi L F, Lin K X, Yao R H, Lin X Y, Yu C Y, Yu Y P 2001 Acta Phys. Sin. 50 1313
Google Scholar
[3] 吴莹, 白顺波, 王俊彦, 陈建平, 倪晓武 2007 光电子技术 27 49
Google Scholar
Wu Y, Bai S B, Wang J Y, Chen J P, Ni X W 2007 Optoelectron. Technol. 27 49
Google Scholar
[4] 吴蓉, 李燕, 朱顺官, 冯红艳, 张琳, 王俊德 2008 光谱学与光谱分析 28 731
Google Scholar
Wu R, Li Y, Zhu S G, Feng H Y, Zhang L, Wang J D 2008 Spectrosc. Spectral Anal. 28 731
Google Scholar
[5] 王彦飞, 朱悉铭, 张明志, 孟圣峰, 贾军伟, 柴昊, 王旸, 宁中喜 2021 70 095211
Google Scholar
Wang Y F, Zhu X M, Zhang M Z, Meng S F, Jia J W, Chai H, Wang Y, Ning Z X 2021 Acta Phys. Sin. 70 095211
Google Scholar
[6] 武晋泽, 唐晋娥, 董有尔, 张国峰, 王彦华 2012 61 195208
Google Scholar
Wu J Z, Tang J E, Dong Y E, Zhang G F, Wang Y H 2012 Acta Phys. Sin 61 195208
Google Scholar
[7] Rishabhkumar M N, Nandurbarkar A B, Buch J U 2017 International Conference on Inventive Computing and Informatics Coimbatore, India, November 23–24, 2017 pp267–272
[8] Jobes F C, Mansfield D K 1992 Rev. Sci. Instrum. 63 5154
Google Scholar
[9] Ohler G S, Gilchrist E B, Gallimore D A 1995 IEEE Trans. Plasma Sci. 23 428
Google Scholar
[10] Ghaderi M, Moradi G, Mousavi P 2018 IEEE Trans. Plasma Sci. 47 451
Google Scholar
[11] Atrey P, Pujara D, Mukherjee S, Rakesh L 2019 IEEE Trans. Plasma. Sci. 47 1316
Google Scholar
[12] Yoshikawa M, Matsumoto T, Shima Y, Negishi S, Imai T 2008 Rev. Sci. Instrum. 79 2281
Google Scholar
[13] Eiichirou K, Lin Y H, Atsushi M, Yasushi N, Cheng C Z 2014 Rev. Sci. Instrum. 85 023507
Google Scholar
[14] 易臻 2006 制造业自动化 28 091
Google Scholar
Yi Z 2006 Manuf. Autom. 28 091
Google Scholar
[15] 马平, 石安华, 杨益兼, 于哲峰, 梁世昌, 黄洁 2017 66 102401
Google Scholar
Ma P, Shi A H, Yang Y J, Yu Z F, Liang S C, Huang J 2017 Acta Phys. Sin. 66 102401
Google Scholar
[16] 马昊军, 王国林, 罗杰, 刘丽萍, 潘德贤, 张军, 刑英丽, 唐飞 2018 67 025201
Google Scholar
Ma H J, Wang G L, Luo J, Liu L P, Pan D X, Zhang J, Xing Y L, Tang F 2018 Acta Phys. Sin. 67 025201
Google Scholar
[17] 肖礼康, 唐璞, 陈波, 万莉莉, 何子远, 马平 2017 兵器装备工程学报 38 44
Google Scholar
Xiao L K, Tang P, Chen B, Wang L L, He Z Y, Ma P 2017 J. Ordnance Equip. Eng. 38 44
Google Scholar
[18] Shi P W, Shi Z B, Chen W, Zhong W L, Yang Z C, Jiang M, Zhang B Y, Li Y G, Yu L M, Liu Z T, Ding X T 2016 Plasma Sci. Technol. 18 708
Google Scholar
[19] 叶民友, 郭文康, 范叔平 1991 核聚变与等离子体物理 11 185
Google Scholar
Ye M Y, Guo W K, Fan S P 1991 Nucl. Fusion Plasma Phys. 11 185
Google Scholar
[20] 何璐, 董晓龙, 张祥坤 2016 空间科学学报 36 358
Google Scholar
He L, Dong X L, Zhang X K 2016 Chin. J. Space Sci. 36 358
Google Scholar
[21] 江少恩, 刘忠礼, 唐道源, 郑志坚 1999 光学学报 19 660
Google Scholar
Jiang S E, Liu Z L, Tang D Y, Zheng Z J 1999 Acta Opt. Sin. 19 660
Google Scholar
-
图 1 模型尾迹示意图
Figure 1. The schematic diagram of wake.
图 2
$P = 40000{\text{ }}{\rm{Pa}}, V = 5.83{\text{ km}}/{\text{s}}$ 钢球模型尾迹电子密度分布 (a) 电子密度流场; (b) 不同轴向位置电子密度径向二维分布Figure 2. The wake of ball electron density distribution (
$P = 40000{\text{ }}{\rm{Pa}}, V = 5.83{\text{ km}}/{\text{s}}$ ): (a) The flow field of electron density; (b) radial two-dimensional distribution of electron density at different axial positions.
图 3 Ka波段七通道微波干涉仪测量系统工作原理
Figure 3. Operating principle of seven channels Ka band microwave interferometer measurement system working in Ka band.
图 4 接收天线端口分布
Figure 4. The port distribution of the receiving antenna.
图 5 射线追踪示意图 (a) 传播路径; (b) 分界面折射
Figure 5. The schematic diagram of ray tracing: (a) Tracking path; (b) refraction at the interface.
图 6 径向不同位置电子密度随轴向距离的分布 (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P = 40000 Pa, V = 4.72 km/s
Figure 6. The electron density at different radial positions distribution with axial distance: (a) Steel ball of ϕ12 mm, P = 40000 Pa, V = 5.83 km/s; (b) steel ball of ϕ12 mm, P = 20000 Pa, V = 5.80 km/s; (c) Al2O3 ball of ϕ15 mm, P = 40000 Pa, V = 4.72 km/s.
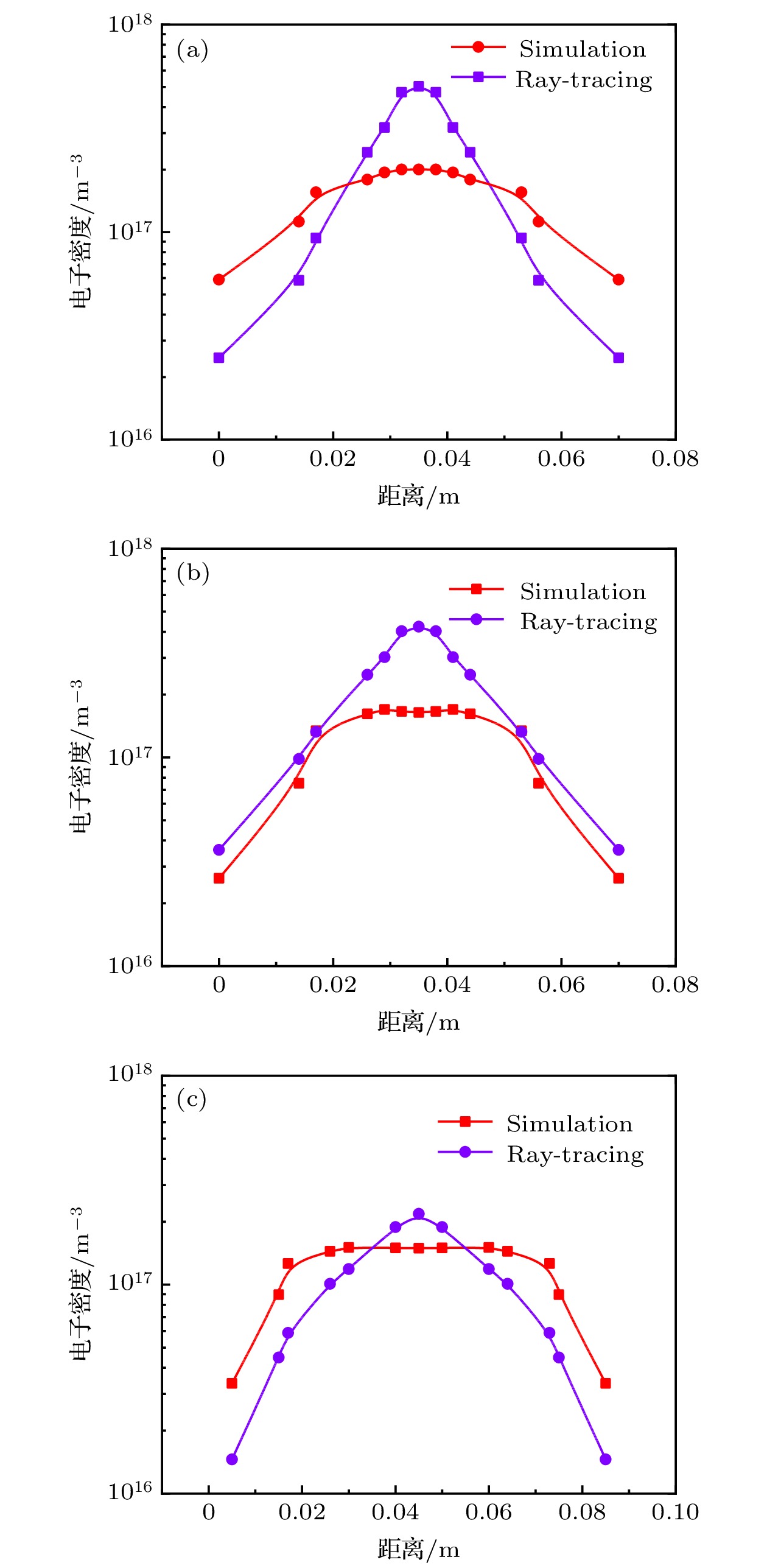
图 7 x = 10
$ 0\phi $ 电子密度径向二维分布 (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P = 40000 Pa, V = 4.72 km/sFigure 7. The radial two-dimensional distribution of plasma electron density (x = 100
$ \phi $ ): (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P = 40000 Pa, V = 4.72 km/s.
图 8 x = 50
$ \phi $ 电子密度径向二维分布 (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P = 40000 Pa, V = 4.72 km/sFigure 8. The radial two-dimensional distribution of plasma electron density (x = 50
$ \phi $ ): (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P 40000 Pa, V = 4.72 km/s.
图 9 x = 10
$ \phi $ 电子密度径向二维分布 (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P = 40000 Pa, V = 4.72 km/sFigure 9. The radial two-dimensional distribution of plasma electron density (x = 10
$ \phi $ ): (a) ϕ12 mm 钢球, P = 40000 Pa, V = 5.83 km/s; (b) ϕ12 mm 钢球, P = 20000 Pa, V = 5.80 km/s; (c) ϕ15 mm Al2O3球, P = 40000 Pa, V = 4.72 km/s.
图 10 不同分层数计算结果对比图 (a) x = 50
$ \phi $ ; (b) x = 10$ \phi $ Figure 10. Comparison diagram of results of different stratification numbers: (a) x = 50
$ \phi $ ; (b) x = 10$ \phi $ . -
[1] 于哲峰, 孙良奎, 马平, 杨益兼, 张志成, 黄洁 2017 红外 38 039
Google Scholar
Yu Z F, Sun L K, Ma P, Yang Y J, Zhang Z C, Huang J 2017 Infrared 38 039
Google Scholar
[2] 池凌飞, 林揆训, 姚若河, 林璇英, 余楚迎, 余云鹏 2001 50 1313
Google Scholar
Chi L F, Lin K X, Yao R H, Lin X Y, Yu C Y, Yu Y P 2001 Acta Phys. Sin. 50 1313
Google Scholar
[3] 吴莹, 白顺波, 王俊彦, 陈建平, 倪晓武 2007 光电子技术 27 49
Google Scholar
Wu Y, Bai S B, Wang J Y, Chen J P, Ni X W 2007 Optoelectron. Technol. 27 49
Google Scholar
[4] 吴蓉, 李燕, 朱顺官, 冯红艳, 张琳, 王俊德 2008 光谱学与光谱分析 28 731
Google Scholar
Wu R, Li Y, Zhu S G, Feng H Y, Zhang L, Wang J D 2008 Spectrosc. Spectral Anal. 28 731
Google Scholar
[5] 王彦飞, 朱悉铭, 张明志, 孟圣峰, 贾军伟, 柴昊, 王旸, 宁中喜 2021 70 095211
Google Scholar
Wang Y F, Zhu X M, Zhang M Z, Meng S F, Jia J W, Chai H, Wang Y, Ning Z X 2021 Acta Phys. Sin. 70 095211
Google Scholar
[6] 武晋泽, 唐晋娥, 董有尔, 张国峰, 王彦华 2012 61 195208
Google Scholar
Wu J Z, Tang J E, Dong Y E, Zhang G F, Wang Y H 2012 Acta Phys. Sin 61 195208
Google Scholar
[7] Rishabhkumar M N, Nandurbarkar A B, Buch J U 2017 International Conference on Inventive Computing and Informatics Coimbatore, India, November 23–24, 2017 pp267–272
[8] Jobes F C, Mansfield D K 1992 Rev. Sci. Instrum. 63 5154
Google Scholar
[9] Ohler G S, Gilchrist E B, Gallimore D A 1995 IEEE Trans. Plasma Sci. 23 428
Google Scholar
[10] Ghaderi M, Moradi G, Mousavi P 2018 IEEE Trans. Plasma Sci. 47 451
Google Scholar
[11] Atrey P, Pujara D, Mukherjee S, Rakesh L 2019 IEEE Trans. Plasma. Sci. 47 1316
Google Scholar
[12] Yoshikawa M, Matsumoto T, Shima Y, Negishi S, Imai T 2008 Rev. Sci. Instrum. 79 2281
Google Scholar
[13] Eiichirou K, Lin Y H, Atsushi M, Yasushi N, Cheng C Z 2014 Rev. Sci. Instrum. 85 023507
Google Scholar
[14] 易臻 2006 制造业自动化 28 091
Google Scholar
Yi Z 2006 Manuf. Autom. 28 091
Google Scholar
[15] 马平, 石安华, 杨益兼, 于哲峰, 梁世昌, 黄洁 2017 66 102401
Google Scholar
Ma P, Shi A H, Yang Y J, Yu Z F, Liang S C, Huang J 2017 Acta Phys. Sin. 66 102401
Google Scholar
[16] 马昊军, 王国林, 罗杰, 刘丽萍, 潘德贤, 张军, 刑英丽, 唐飞 2018 67 025201
Google Scholar
Ma H J, Wang G L, Luo J, Liu L P, Pan D X, Zhang J, Xing Y L, Tang F 2018 Acta Phys. Sin. 67 025201
Google Scholar
[17] 肖礼康, 唐璞, 陈波, 万莉莉, 何子远, 马平 2017 兵器装备工程学报 38 44
Google Scholar
Xiao L K, Tang P, Chen B, Wang L L, He Z Y, Ma P 2017 J. Ordnance Equip. Eng. 38 44
Google Scholar
[18] Shi P W, Shi Z B, Chen W, Zhong W L, Yang Z C, Jiang M, Zhang B Y, Li Y G, Yu L M, Liu Z T, Ding X T 2016 Plasma Sci. Technol. 18 708
Google Scholar
[19] 叶民友, 郭文康, 范叔平 1991 核聚变与等离子体物理 11 185
Google Scholar
Ye M Y, Guo W K, Fan S P 1991 Nucl. Fusion Plasma Phys. 11 185
Google Scholar
[20] 何璐, 董晓龙, 张祥坤 2016 空间科学学报 36 358
Google Scholar
He L, Dong X L, Zhang X K 2016 Chin. J. Space Sci. 36 358
Google Scholar
[21] 江少恩, 刘忠礼, 唐道源, 郑志坚 1999 光学学报 19 660
Google Scholar
Jiang S E, Liu Z L, Tang D Y, Zheng Z J 1999 Acta Opt. Sin. 19 660
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3479
- PDF Downloads: 55
- Cited By: 0
