-
The macroscopic fundamental diagram (MFD), which can describe the macroscopic state of a regional network intuitively, describes a unimodal, statistical and reproducible relationship between accumulation and the trip completion flow of a region. Existing researches have proved that using MFD characteristics can ‘metering’ the boundary flow and relieve the traffic congestion problem effectively. As the foundation of traffic control, existing studies on the characteristic of MFD have proved that external origin to destination demand does not influence the MFD distribution. However, the traffic generation and attraction sources in the regional road networkwill changes the distribution of traffic density of the road network, thus affecting the characteristic of MFD. However, to date, no related analysis explored the influence of the traffic generation and attraction sourcesin the regional road network. To solve this problem, according to the spatial and temporal distribution of traffic generation and attraction sources in the regional road network, this paper puts forward an aggregation degree index analysis model of traffic generation and attraction sources, based on the dynamic parameters of traffic generation and attraction sources, and section traffic impedance. Taking a square-format road network as the target, nine groups of schemes for different traffic generation and attraction sources are designed. Two conclusions can be drawn after comparing the MFD curve of the road network under different aggregation degree index of traffic generation and attraction sources: (1) when the traffic flow of the road network is in the state of free flow or critical flow, the aggregation effect of traffic generation and attraction sources has no effect on MFD distribution; (2) when the traffic flow of the road network is in the congestion flow state, the aggregation effect of traffic generation and attraction sources influences MFD distribution. Moreover, under the same road network flow conditions, the aggregation degree of traffic generation and attraction sources is lower in the road network (the distribution of traffic generation and attraction volume is more balanced), the trip completion flow of road network will be higher. Otherwise, the aggregation degree of traffic generation and attraction sources is higher in the road network (the distribution of traffic generation and attraction volume is more unbalanced), the trip completion flow of road network will be lower.
-
Keywords:
- macroscopic fundamental diagram /
- traffic generation and attraction source /
- aggregation degree /
- traffic simulation
[1] Geroliminis N, Daganzo C F 2008 Transp. Res. Pt. B-Methodol. 42 759
Google Scholar
[2] Gonzales E J, Chavis C, Li Y, Daganzo C F 2009 Multimodal transport modeling for Nairobi, Kenya: Insights and recommendations with an evidencebased mode (Berkeley: UC Berkeley Center for Future Urban Transport) pp1—36
[3] Haddad J 2017 Control Eng. Practice 61 134
Google Scholar
[4] Yang K D, Zheng N, Menendez M 2018 Transp. Res. Pt. C-Emerg. Technol. 94 32
Google Scholar
[5] Ding H, Zhang Y, Zheng X Y, Yuan H Y, Zhang W H 2018 IEEE Trans. Control Syst. Technol. 26 2049
Google Scholar
[6] Kim S, Tak S, Yeo H 2018 Transp. Res. Pt. C-Emerg. Technol. 93 79
Google Scholar
[7] Haddad J, Mirkin B 2017 Transp. Res. Pt. C-Emerg. Technol. 77 495
Google Scholar
[8] 丁恒, 朱良元, 蒋程镔, 袁含雨 2017 交通运输系统工程与信息 17 104
Ding H, Zhu L Y, Jiang C B, Yuan H Y 2017 J. Transport. Syst. Engineer. Inform. Technol. 17 104
[9] Ding H, Guo F, Zheng X Y, Zhang W H 2017 Transp. Res. Pt. C-Emerg. Technol. 81 300
Google Scholar
[10] Godfrey J W 1969 TrafficEngineer. Control. 11 323
[11] Gao F 2011 Ph. D. Dissertation (Stockholm: School of Architecture and the Built Environment)
[12] Leclercq L, Geroliminis N 2013 Transp. Res. Pt. B-Methodol. 57 468
Google Scholar
[13] Daganzo C F, Geroliminis N 2008 Transp. Res. Pt. B-Methodol. 42 771
Google Scholar
[14] Courbon T, Leclercq L 2011 Procedia Soc. Behav Sci 20 417
Google Scholar
[15] Geroliminis N, Danes J, Estrada M A 2013 Transportation Research Board 92 nd Annual Meeting Washington DC, United States, January 13–17, 2013 p1013
[16] Zheng N, Geroliminis N 2013 Transp. Res. Pt. B-Methodol. 57 326
Google Scholar
[17] Wang Y, Duan Z Y 2012 The 7 th Annual Conference of ITS China Beijig, China, September 26, 2012 p112
[18] Gayah V V, Daganzo C F 2011 Transp. Res. Pt. B-Methodol. 45 643
Google Scholar
[19] 许菲菲, 何兆成, 沙志仁 2013 交通运输系统工程与信息 13 185
Google Scholar
Xu F F, He Z C, Sha Z R 2013 J. Transport. Syst. Engineer. Inform. Technol. 13 185
Google Scholar
[20] 朱琳, 于雷, 宋国华 2012 华南理工大学学报(自然科学版) 40 138
Zhu L, Yu L, Song G H 2012 J. South Chin Univ. Technol. (Nat. Sci.) 40 138
[21] Geroliminis N, Sun J 2011 Transp. Res. Pt. B-Methodol. 45 605
Google Scholar
[22] Geroliminis N, Boyaci B 2012 Transp. Res. Pt. B-Methodol. 46 1607
Google Scholar
[23] Buisson C, Ladier C 2009 Transp. Res. Record 2124 127
Google Scholar
[24] Jin W L, Gan Q J, Gayah V V 2013 Transp. Res. Pt. B-Methodol. 57 114
Google Scholar
[25] Alonso B, Ibeas A, Musolino G, Rindone C, Vitetta A 2019 Transp. Res. Pt. A-Policy Pract. 126 136
Google Scholar
[26] Leclercq L, Geroliminis N 2013 Procedia Soc. Behav Sci 80 960
Google Scholar
[27] Mazloumian A, Geroliminis N, Helbing D 2010 Philos. Trans. R. Soc. A-Math. Phys. Eng. Sci. 368 4627
Google Scholar
[28] 丁恒, 朱良元, 蒋程镔, 郑小燕 2018 重庆交通大学学报(自然科学版) 37 77
Ding H, Zhu L Y, Jiang C B, Zheng X Y 2018 J. Chongqing Jiaotong Univ. (Nat. Sci.) 37 77
[29] 马寒月 2016 硕士学位论文 (合肥: 合肥工业大学)
Ma H Y 2016 M. S. Thesis (Hefei: Hefei University of Technology) (in Chinese)
[30] Elilsion G, Glaeser E 1997 J. Polit. Econ. 105 889
Google Scholar
[31] 四兵锋, 钟鸣, 高自友 2008 交通运输系统工程与信息 8 68
Google Scholar
Si B F, Zhong M, Gao Z Y 2008 J. Transport. Syst. Engineer. Inform. Technol. 8 68
Google Scholar
[32] Branston D 1976 Transp. Res. 10 223
Google Scholar
[33] Bates D M, Watts D G 1988 Nonlinear Regression Analysis and Its Applications (New York: Wiley) pp1−372
[34] Gonzales E J, Chavis C, Li Y, Daganzo C F 2011 Transportation Research Board 90th Annual Meeting Washington DC, United States, January 23−27, 2011 p11
-
图 1 MFD (a) MFD的基本特征; (b) 路网状态划分
Figure 1. MFD: (a) Basic characteristics of MFD; (b) state classification of MFD curve.
图 2 仿真路网
Figure 2. Simulation road network.
图 3 不同交通发生吸引源集聚影响下交通密度分布
Figure 3. Traffic density distribution under different traffic generation and attraction sources.
图 4 不同交通发生吸引源规模下路网MFDs (a) 800 pcu·h–1; (b) 1200 pcu·h–1; (c) 2000 pcu·h–1
Figure 4. MFDs under different traffic generation and attraction source scale: (a) 800 pcu·h–1; (b) 1200 pcu·h–1; (c) 2000 pcu·h–1.
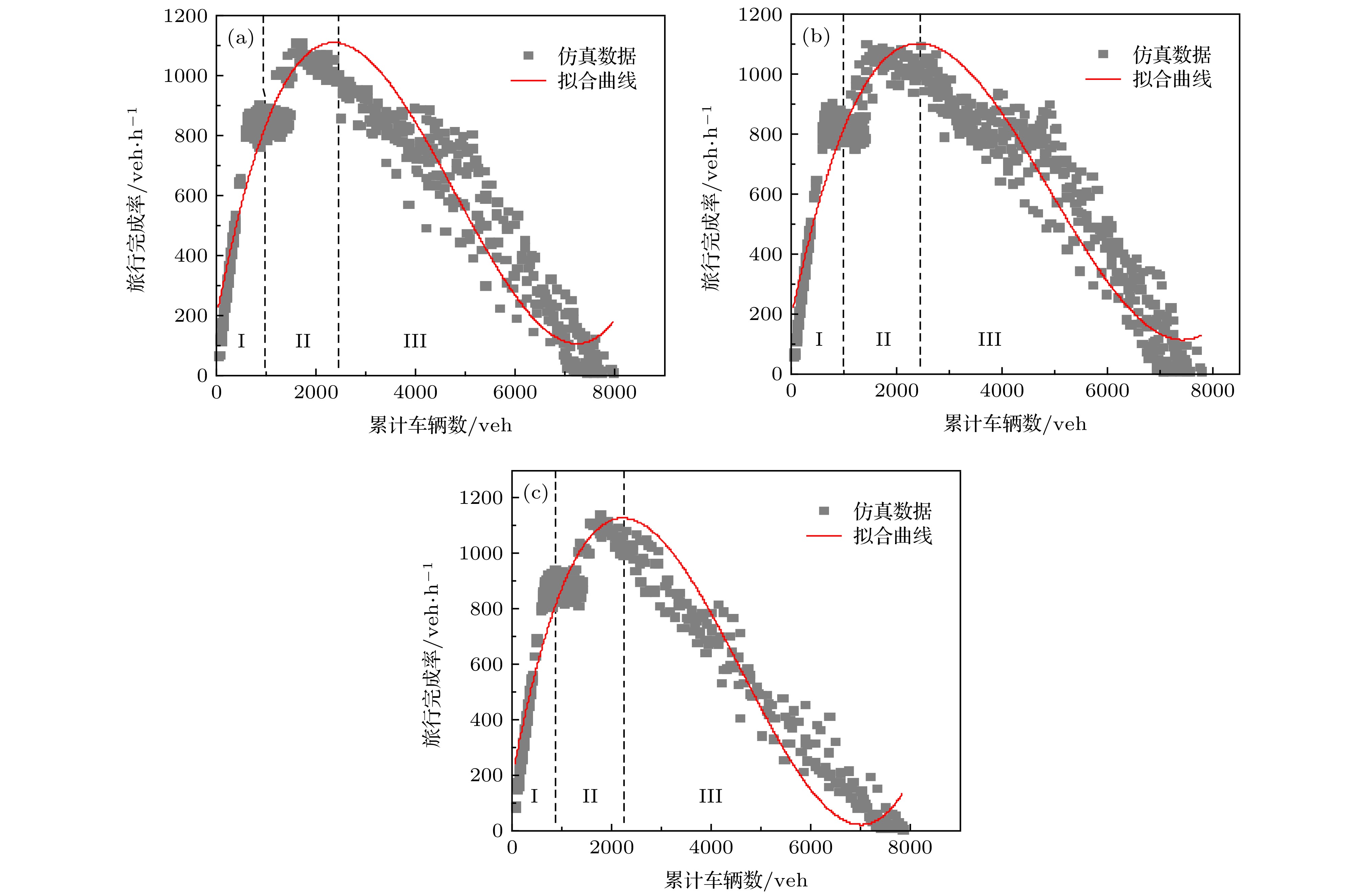
图 5 不同交通发生吸引源配置条件下MFDs和聚集度曲线 (a1) A组仿真实验MFD; (a2) A组仿真实验聚集度曲线; (b1) B组仿真实验MFD; (b2) B组仿真实验聚集度曲线; (c1) C组仿真实验MFD; (c2) C组仿真实验聚集度曲线
Figure 5. MFDs and aggregation degree curves under different traffic generation and attraction source configuration: (a1) MFDof group A simulation scheme; (a2) aggregation degree curve of group A simulation scheme; (b1) MFDof group B simulation scheme; (b2) aggregation degree curve of group B simulation scheme; (b1) MFDof group C simulation scheme; (b2) aggregation degree curve of group C simulation scheme.
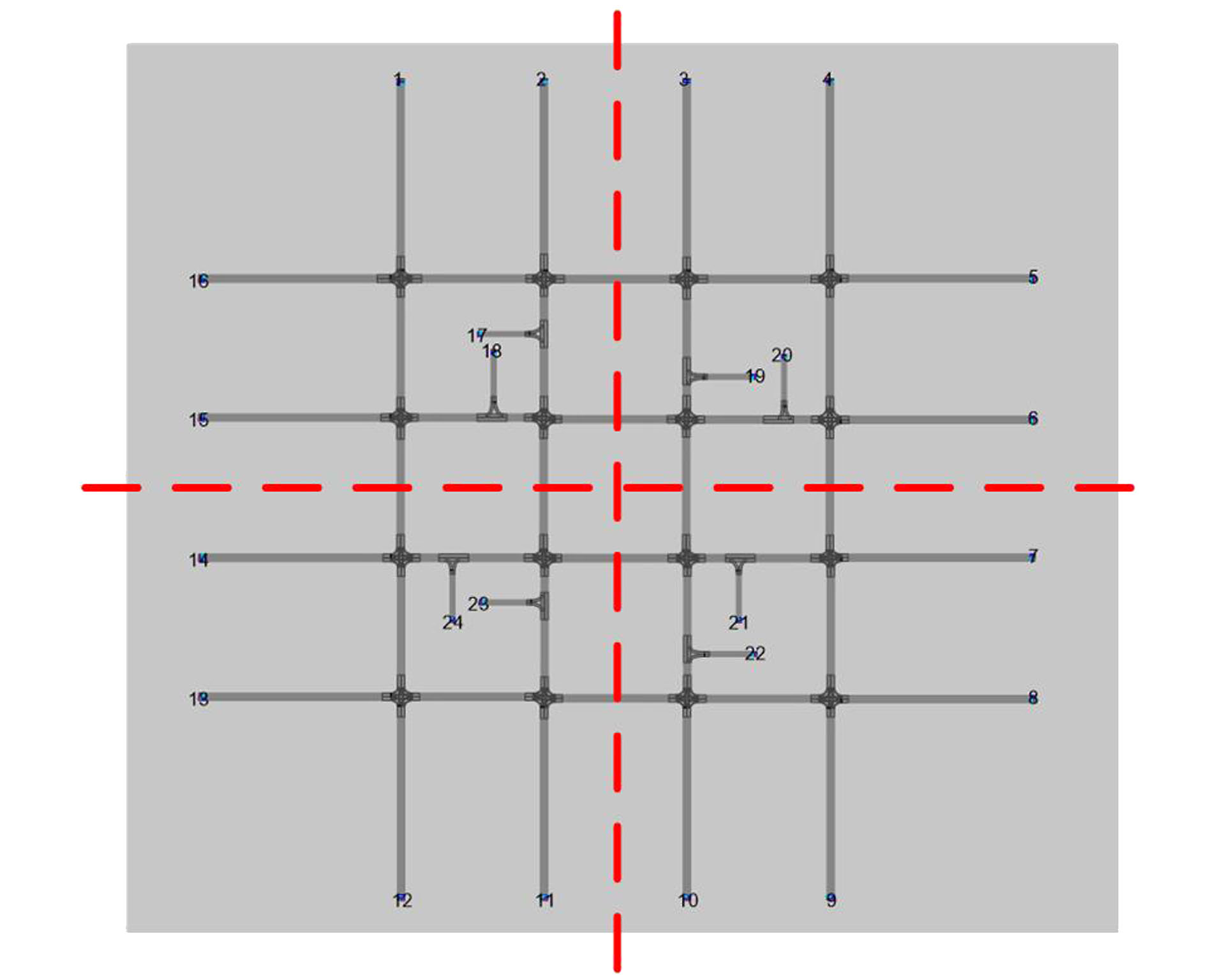
表 1 交通发生吸引源配置参数
Table 1. Traffic generation and attraction source configuration parameters.
交通发生吸引源配置方案 1 2 3 4 5 初始聚集度 –0.142 –0.146 –0.182 –0.082 –0.009 停车场规模配置情况/pcu·h–1 20 38 93 272 38 40 72 58 203 71 60 279 121 50 50 80 123 74 49 86 100 46 80 322 408 120 38 132 150 530 140 108 147 49 389 240 96 95 105 428 
DownLoad: CSV
表 2 不同交通发生吸引源规模下MFD参数
Table 2. MFD parameters under different traffic generation and attraction sourcescale.
吸引源规模/pcu·h–1 MFD拟合参数 ${R^2}$ ${a_i}$ ${b_i}$ ${c_i}$ ${d_i}$ 800 2 × 10–8 –0.0002 0.8434 192.34 0.876 1200 2 × 10–8 –0.0002 0.8742 190.75 0.888 2000 2 × 10–8 –0.0003 0.9424 188.86 0.901
DownLoad: CSV
表 3 仿真实验基本参数
Table 3. Basic parameters of simulation experiment.
实验编号 A B C 停车场总规模/pcu·h–1 800 1200 2000 实验组数 1 2 3 1 2 3 1 2 3 初始聚集度 –0.142 –0.146 –0.182 –0.082 –0.144 –0.171 –0.009 –0.104 –0.151 停车场规模/pcu·h–1 20 38 93 272 138 70 38 100 268 40 72 58 203 245 300 71 170 189 60 279 121 50 367 210 50 140 99 80 123 74 49 103 65 86 200 304 100 46 80 322 179 70 408 260 341 120 38 132 150 48 130 530 290 107 140 108 147 49 37 220 389 350 402 240 96 95 105 83 135 428 490 290
DownLoad: CSV
表 4 不同交通发生吸引源配置条件下MFD参数
Table 4. MFD parameters under differenttraffic generation and attraction sourceconfiguration.
实验组 MFD拟合参数 ${R^2}$ ${a_i}$ ${b_i}$ ${c_i}$ ${d_i}$ A1组 3 × 10–8 –0.0003 1.0743 111.66 0.951 A2组 3 × 10–8 –0.0004 1.0819 111.44 0.960 A3组 3 × 10–8 –0.0003 1.0588 116.9 0.953 B1组 5 × 10–8 –0.0005 1.3327 61.38 0.982 B2组 3 × 10–8 –0.0004 1.0518 125.81 0.967 B3组 2 × 10–8 –0.0003 0.9445 152.79 0.934 C1组 5 × 10–8 –0.0005 1.3081 84.82 0.942 C2组 4 × 10–8 –0.0005 1.2132 110 0.929 C3组 4 × 10–8 –0.0005 1.2579 100.65 0.927
DownLoad: CSV
表 5 各组仿真实验平均旅行完成率评估参数
Table 5. Evaluation parameters of the average trip completionflow of simulation experiment.
实验编号 平均旅行完成率
变化率/%交通流状态 Ⅰ Ⅱ Ⅲ A (A3–A1)/A1 0.77 3.13 30.96 (A3–A2)/A2 0.61 1.14 3.29 (A2–A1)/A1 0.16 1.96 26.79 B (B3–B1)/B1 –0.11 –0.51 34.36 (B3–B2)/B2 –0.02 0.24 7.45 (B2–B1)/B1 –0.09 –0.74 25.05 C (C3–C1)/C1 0.10 1.52 14.87 (C3–C2)/C2 0.41 1.17 6.01 (C2–C1)/C1 –0.31 0.34 8.36
DownLoad: CSV
-
[1] Geroliminis N, Daganzo C F 2008 Transp. Res. Pt. B-Methodol. 42 759
Google Scholar
[2] Gonzales E J, Chavis C, Li Y, Daganzo C F 2009 Multimodal transport modeling for Nairobi, Kenya: Insights and recommendations with an evidencebased mode (Berkeley: UC Berkeley Center for Future Urban Transport) pp1—36
[3] Haddad J 2017 Control Eng. Practice 61 134
Google Scholar
[4] Yang K D, Zheng N, Menendez M 2018 Transp. Res. Pt. C-Emerg. Technol. 94 32
Google Scholar
[5] Ding H, Zhang Y, Zheng X Y, Yuan H Y, Zhang W H 2018 IEEE Trans. Control Syst. Technol. 26 2049
Google Scholar
[6] Kim S, Tak S, Yeo H 2018 Transp. Res. Pt. C-Emerg. Technol. 93 79
Google Scholar
[7] Haddad J, Mirkin B 2017 Transp. Res. Pt. C-Emerg. Technol. 77 495
Google Scholar
[8] 丁恒, 朱良元, 蒋程镔, 袁含雨 2017 交通运输系统工程与信息 17 104
Ding H, Zhu L Y, Jiang C B, Yuan H Y 2017 J. Transport. Syst. Engineer. Inform. Technol. 17 104
[9] Ding H, Guo F, Zheng X Y, Zhang W H 2017 Transp. Res. Pt. C-Emerg. Technol. 81 300
Google Scholar
[10] Godfrey J W 1969 TrafficEngineer. Control. 11 323
[11] Gao F 2011 Ph. D. Dissertation (Stockholm: School of Architecture and the Built Environment)
[12] Leclercq L, Geroliminis N 2013 Transp. Res. Pt. B-Methodol. 57 468
Google Scholar
[13] Daganzo C F, Geroliminis N 2008 Transp. Res. Pt. B-Methodol. 42 771
Google Scholar
[14] Courbon T, Leclercq L 2011 Procedia Soc. Behav Sci 20 417
Google Scholar
[15] Geroliminis N, Danes J, Estrada M A 2013 Transportation Research Board 92 nd Annual Meeting Washington DC, United States, January 13–17, 2013 p1013
[16] Zheng N, Geroliminis N 2013 Transp. Res. Pt. B-Methodol. 57 326
Google Scholar
[17] Wang Y, Duan Z Y 2012 The 7 th Annual Conference of ITS China Beijig, China, September 26, 2012 p112
[18] Gayah V V, Daganzo C F 2011 Transp. Res. Pt. B-Methodol. 45 643
Google Scholar
[19] 许菲菲, 何兆成, 沙志仁 2013 交通运输系统工程与信息 13 185
Google Scholar
Xu F F, He Z C, Sha Z R 2013 J. Transport. Syst. Engineer. Inform. Technol. 13 185
Google Scholar
[20] 朱琳, 于雷, 宋国华 2012 华南理工大学学报(自然科学版) 40 138
Zhu L, Yu L, Song G H 2012 J. South Chin Univ. Technol. (Nat. Sci.) 40 138
[21] Geroliminis N, Sun J 2011 Transp. Res. Pt. B-Methodol. 45 605
Google Scholar
[22] Geroliminis N, Boyaci B 2012 Transp. Res. Pt. B-Methodol. 46 1607
Google Scholar
[23] Buisson C, Ladier C 2009 Transp. Res. Record 2124 127
Google Scholar
[24] Jin W L, Gan Q J, Gayah V V 2013 Transp. Res. Pt. B-Methodol. 57 114
Google Scholar
[25] Alonso B, Ibeas A, Musolino G, Rindone C, Vitetta A 2019 Transp. Res. Pt. A-Policy Pract. 126 136
Google Scholar
[26] Leclercq L, Geroliminis N 2013 Procedia Soc. Behav Sci 80 960
Google Scholar
[27] Mazloumian A, Geroliminis N, Helbing D 2010 Philos. Trans. R. Soc. A-Math. Phys. Eng. Sci. 368 4627
Google Scholar
[28] 丁恒, 朱良元, 蒋程镔, 郑小燕 2018 重庆交通大学学报(自然科学版) 37 77
Ding H, Zhu L Y, Jiang C B, Zheng X Y 2018 J. Chongqing Jiaotong Univ. (Nat. Sci.) 37 77
[29] 马寒月 2016 硕士学位论文 (合肥: 合肥工业大学)
Ma H Y 2016 M. S. Thesis (Hefei: Hefei University of Technology) (in Chinese)
[30] Elilsion G, Glaeser E 1997 J. Polit. Econ. 105 889
Google Scholar
[31] 四兵锋, 钟鸣, 高自友 2008 交通运输系统工程与信息 8 68
Google Scholar
Si B F, Zhong M, Gao Z Y 2008 J. Transport. Syst. Engineer. Inform. Technol. 8 68
Google Scholar
[32] Branston D 1976 Transp. Res. 10 223
Google Scholar
[33] Bates D M, Watts D G 1988 Nonlinear Regression Analysis and Its Applications (New York: Wiley) pp1−372
[34] Gonzales E J, Chavis C, Li Y, Daganzo C F 2011 Transportation Research Board 90th Annual Meeting Washington DC, United States, January 23−27, 2011 p11
DownLoad:
Catalog
Metrics
- Abstract views: 6975
- PDF Downloads: 95
- Cited By: 0
