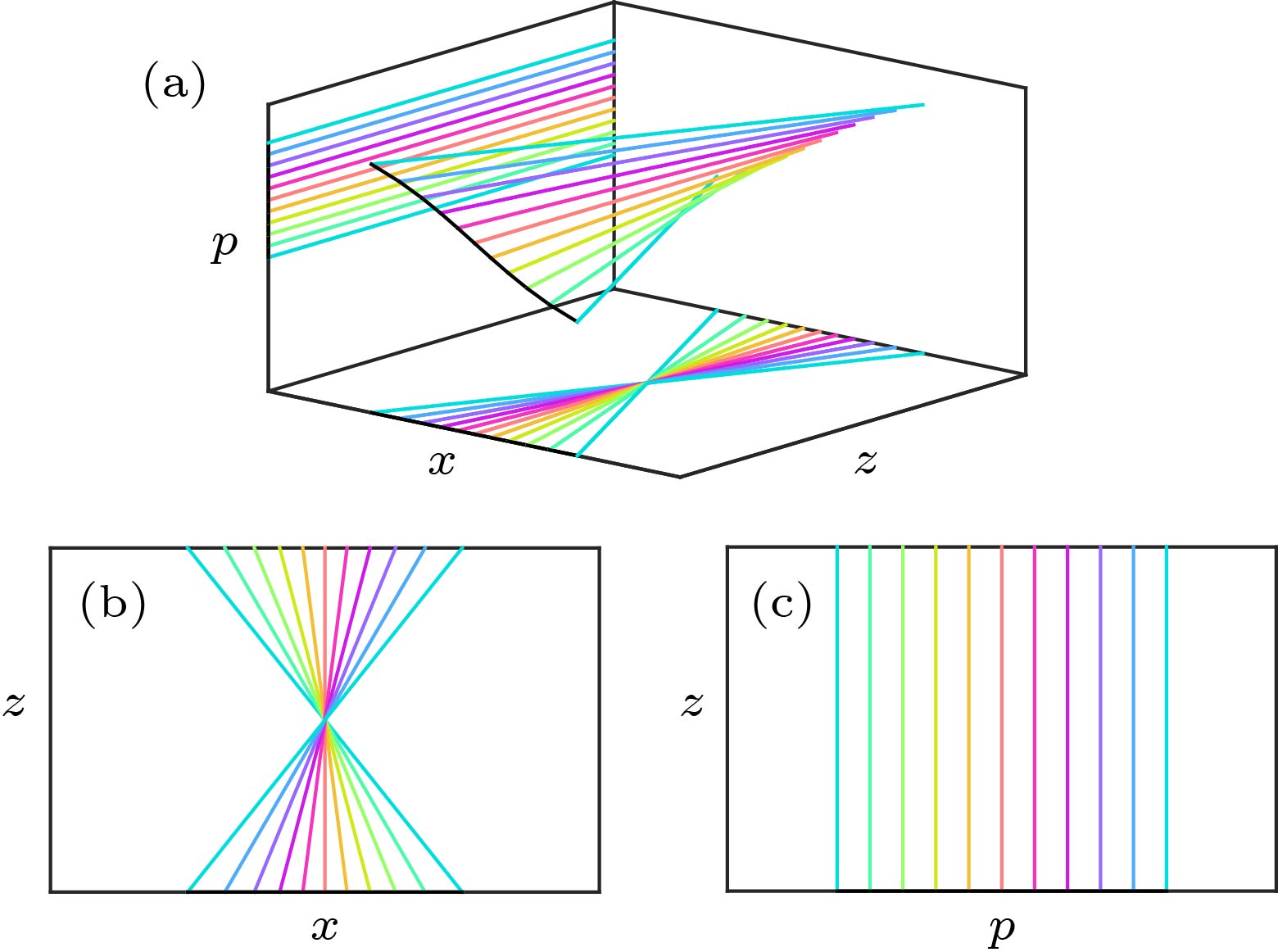
-
Establishing a universal model to characterize the relationship between light rays and optical waves is of great significance in optics. The ray model provides us with an intuitive way to study the propagation of beams as well as their interaction between objects. Traditional ray model is based on the normal of a beam wave front. The normal vector is defined as the direction of ray. However, it fails to describe the relationship between light ray and optical wave in the neighborhood of focus or caustic lines/surface since light ray in those regions are no longer perpendicular to the wavefront. In this work, the ray model of a light beam is built according to its Fourier angular spectrum, where the positions of rays can be determined by the gradient of the phase of the Fourier angular spectrum. On the other hand, the Fourier angular spectrum of a light beam can be reconstructed through the ray model. Using Fourier angular spectra, we construct the ray model of two typical beams including the Airy beam and the Cusp beam. It is hard to construct ray model directly from the optical field of these beams. In this ray model, the information about ray including direction and position involves the propagation properties of light beams such as self-accelerating. In addition, we demonstrate that the optical field of the focused plane wave can be reconstructed by the ray model in Fourier regime, and the optical field in spatial domain can be obtained by inverse Fourier transform. Simulation results are consistent with the results from Debye’s method. Finally, the high-dimensional ray model of light beams is elaborated in both spatial and spectral regime. Combined with focused plane wave, Airy beam and rays in quadratic gradient-index waveguide, our results show that the ray model actually carries the information about optical field in both spatial and Fourier domain. Actually, the traditional ray model is just a spatial projection of the high-dimensional ray model. Hence, when traditional ray model fails at the focus or caustic lines/surface, it is able to obtain the spectrum of the corresponding optical field from the Fourier domain, and then obtain the field distribution in spatial domain by inverse Fourier transform.
-
Keywords:
- geometrical optics /
- light ray /
- high dimension light ray /
- Fourier angular spectrum
[1] 萧泽新, 安连生 2014 工程光学设计 (北京: 电子工业出版社) 第4−7页
Xiao Z X, An L S 2014 Engineering Optical Design (Beijing: Publishing House of Electronics Industry) (in Chinese) pp4−7
[2] Wikipedia contributor, " Ray tracing (graphics)” from Wikipedia—The Free Encyclopedia. https://en.wikipedia.org/w/index.php?title=Ray_tracing_(graphics)&oldid=888247514 [2019-5-27]
[3] Zhang Z, Levoy M 2009 IEEE International Conferenceon the Computational Photography San Francisco, CA, USA April 16−17, 2009 pp1−10
[4] 张春萍, 王庆 2016 中国激光 43 0609004
Zhang C P, Wang Q 2016 Chin. J. Lasers 43 0609004
[5] Goodman J W 1968 Introduction to Fourier Optics (New York: McGraw-Hill)
[6] 玻恩 M, 沃耳夫 E 著 (杨薛荪 译) 2005 光学原理 (北京: 电子工业出版社) 第403页
Born M, Wolf E (translated by Yang X S) 2005 Principle of Optics (Beijing: Publishing House of Electronics Industry) p 403 (in Chinese)
[7] McNamara D A, Pistorius C W I, Malherbe J A G 1990 Introduction to the Uniform Geometrical Theory of Diffraction (London: Artech House) pp17−27
[8] Keller J B 1962 J. Opt. Soc. Am. 52 116
Google Scholar
[9] Kaganovsky Y, Heyman E 2010 Opt. Express 18 8440
[10] 马亮, 吴逢铁, 黄启禄 2010 光学学报 30 2417
Ma L, Wu F T, Huang Q L 2010 Acta Opt. Sin. 30 2417
[11] Alonso M A, Dennis M R 2017 Optica 4 476
Google Scholar
[12] Bouchard F, Harris J, Mand H, Boyd R W, Karimi E 2016 Optica 3 351
Google Scholar
[13] 左超, 陈钱, 孙佳嵩, Asundi A 2016 中国激光 43 0609002
Zuo C, Chen Q, Sun J S, Asundi A 2016 Chin. J. Lasers 43 0609002
[14] 吕乃光, 金国藩, 苏显渝 2016 傅立叶光学 (北京: 机械工业出版社) 第73页
Lü N G, Jin G P, Su X Y 2016 Fourier Optics (Beijing: China Machine Press) p73 (in Chinese)
[15] Wolf E 1959 Proc. R. Soc. Lond. A 253 349
Google Scholar
[16] Siviloglou G A, Christodoulides D N 2007 Opt. Lett. 32 979
Google Scholar
[17] Barwick S 2010 Opt. Lett. 35 4118
[18] Gong L, Liu W W, Ren Y X, Lu Y, Li Y M 2015 Appl. Phys. Lett. 107 231110
Google Scholar
[19] Forbes G W, Alonso M A 1998 Proc. SPIE 3482 22
[20] Berry M V, Balazs N L 1979 Am. J. Phys. 47 264
Google Scholar
[21] Alonso M A, Forbes G W 2002 Opt. Express 10 728
Google Scholar
-
图 1 平行光经透镜聚焦后产生锥形光线 (a)光线追踪示意图; (b)光锥的简化光线模型
Figure 1. Ray cone that produced by convergent parallel rays through a lens: (a) Sketch of ray-tracing; (b) simplified ray model of ray cone.
图 2 不同横截面处Airy光束的光线分布, 其中(a)
$z = - 180\;{\text{μm}}$ , (b)$z = - 100\;{\text{μm}}$ , (c)$z = 0\;{\text{μm}}$ , (d)$z = 100\;{\text{μm}}$ , (e)$z = 180\;{\text{μm}}$ ; 背景色为归一化的光强分布; 灰色点为光线起点, 红色箭头为光线在xoy面投影矢量, 长度正比于光线与z轴的夹角大小; (f) Airy光束的光线模型; 不同颜色用以区分不同位置的光线Figure 2. Ray model of Airy beam at (a)
$z = - 180\;{\text{μm}}$ , (b)$z = - 100\;{\text{μm}}$ , (c)$z = 0\;{\text{μm}}$ , (d)$z = 100\;{\text{μm}}$ , and (e)$z = 180\;{\text{μm}}$ . Backgrounds is the normalized intensity distribution. The transverse directions of rays are represented by red arrows, the length of arrow is proportional to the sine of the angle between the ray and the z axis. (f) Ray model of Airy beam. Different colors are used to distinguish the rays at different positions.
图 3 不同横截面处Cusp光束的光线分布, 其中(a)
$z = - 180\;{\text{μm}}$ , (b)$z = - 100\;{\text{μm}}$ , (c)$z = 0\;{\text{μm}}$ , (d)$z = 100\;{\text{μm}}$ , (e)$z = 180\;{\text{μm}}$ ; 背景色为归一化的光强分布; 灰色点为光线起点, 红色箭头为光线在xoy面投影矢量, 长度正比于光线与z轴的夹角大小; (f) Cusp光束的光线模型; 不同的颜色用以区分不同位置的光线Figure 3. Ray model of Cusp beam at (a)
$z = - 180\;{\text{μm}}$ , (b)$z = - 100\;{\text{μm}}$ , (c)$z = 0\;{\text{μm}}$ , (d)$z = 100\;{\text{μm}}$ , and (e)$z = 180\;{\text{μm}}$ . Backgrounds is the normalized intensity distribution. The transverse directions of rays are represented by red arrows, the length of arrow is proportional to the sine of the angle between the ray and the z axis. (f) Ray model of Cusp beam. Different colors are used to distinguish the rays at different positions.
图 4 光锥模型及其焦面的傅里叶角谱 (a)经过物镜聚焦后的平行光的光线追踪示意图; (b)使用光线重构得到的焦面上光场的傅里叶角谱
Figure 4. Ray-cone and its Fourier angular spectrum: (a) Ray tracing model of convergent parallel rays; (b) reconstructed Fourier angular spectrum according to the ray model.
图 5 一维聚焦光束的高维光线模型 (a)聚焦光束的三维光线模型; (b)三维光线模型在xoz平面内的投影; (c)三维光线模型在poz平面内的投影
Figure 5. High-dimensional ray model of convergent beam: (a) 3D ray model of convergent beam; (b) projection of 3D ray model in xoz plane; (c) projection of 3D ray model in poz plane.
图 6 二维Airy光束的高维光线模型 (a) Airy光束的三维光线模型; (b)三维光线模型在xoz平面内的投影; (c)三维光线模型在poz平面内的投影
Figure 6. High-dimensional ray model of (1 + 1)D Airy beam: (a) 3D ray model of (1 + 1)D Airy beam; (b) projection of 3D ray model in xoz plane; (c) projection of 3D ray model in poz plane.
图 7 二维抛物线型波导中的厄米-高斯光束的高维光线模型 (a)三维光线模型; (b)三维光线模型在xoz平面内的投影; (c)三维光线模型在poz平面内的投影
Figure 7. High-dimensional ray model of Hermit-Gaussian beam in quadratic gradient-index waveguide: (a) 3D ray model; (b) projection of 3D ray model in xoz plane; (c) projection of 3D ray model in poz plane.
-
[1] 萧泽新, 安连生 2014 工程光学设计 (北京: 电子工业出版社) 第4−7页
Xiao Z X, An L S 2014 Engineering Optical Design (Beijing: Publishing House of Electronics Industry) (in Chinese) pp4−7
[2] Wikipedia contributor, " Ray tracing (graphics)” from Wikipedia—The Free Encyclopedia. https://en.wikipedia.org/w/index.php?title=Ray_tracing_(graphics)&oldid=888247514 [2019-5-27]
[3] Zhang Z, Levoy M 2009 IEEE International Conferenceon the Computational Photography San Francisco, CA, USA April 16−17, 2009 pp1−10
[4] 张春萍, 王庆 2016 中国激光 43 0609004
Zhang C P, Wang Q 2016 Chin. J. Lasers 43 0609004
[5] Goodman J W 1968 Introduction to Fourier Optics (New York: McGraw-Hill)
[6] 玻恩 M, 沃耳夫 E 著 (杨薛荪 译) 2005 光学原理 (北京: 电子工业出版社) 第403页
Born M, Wolf E (translated by Yang X S) 2005 Principle of Optics (Beijing: Publishing House of Electronics Industry) p 403 (in Chinese)
[7] McNamara D A, Pistorius C W I, Malherbe J A G 1990 Introduction to the Uniform Geometrical Theory of Diffraction (London: Artech House) pp17−27
[8] Keller J B 1962 J. Opt. Soc. Am. 52 116
Google Scholar
[9] Kaganovsky Y, Heyman E 2010 Opt. Express 18 8440
[10] 马亮, 吴逢铁, 黄启禄 2010 光学学报 30 2417
Ma L, Wu F T, Huang Q L 2010 Acta Opt. Sin. 30 2417
[11] Alonso M A, Dennis M R 2017 Optica 4 476
Google Scholar
[12] Bouchard F, Harris J, Mand H, Boyd R W, Karimi E 2016 Optica 3 351
Google Scholar
[13] 左超, 陈钱, 孙佳嵩, Asundi A 2016 中国激光 43 0609002
Zuo C, Chen Q, Sun J S, Asundi A 2016 Chin. J. Lasers 43 0609002
[14] 吕乃光, 金国藩, 苏显渝 2016 傅立叶光学 (北京: 机械工业出版社) 第73页
Lü N G, Jin G P, Su X Y 2016 Fourier Optics (Beijing: China Machine Press) p73 (in Chinese)
[15] Wolf E 1959 Proc. R. Soc. Lond. A 253 349
Google Scholar
[16] Siviloglou G A, Christodoulides D N 2007 Opt. Lett. 32 979
Google Scholar
[17] Barwick S 2010 Opt. Lett. 35 4118
[18] Gong L, Liu W W, Ren Y X, Lu Y, Li Y M 2015 Appl. Phys. Lett. 107 231110
Google Scholar
[19] Forbes G W, Alonso M A 1998 Proc. SPIE 3482 22
[20] Berry M V, Balazs N L 1979 Am. J. Phys. 47 264
Google Scholar
[21] Alonso M A, Forbes G W 2002 Opt. Express 10 728
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 8056
- PDF Downloads: 83
- Cited By: 0
