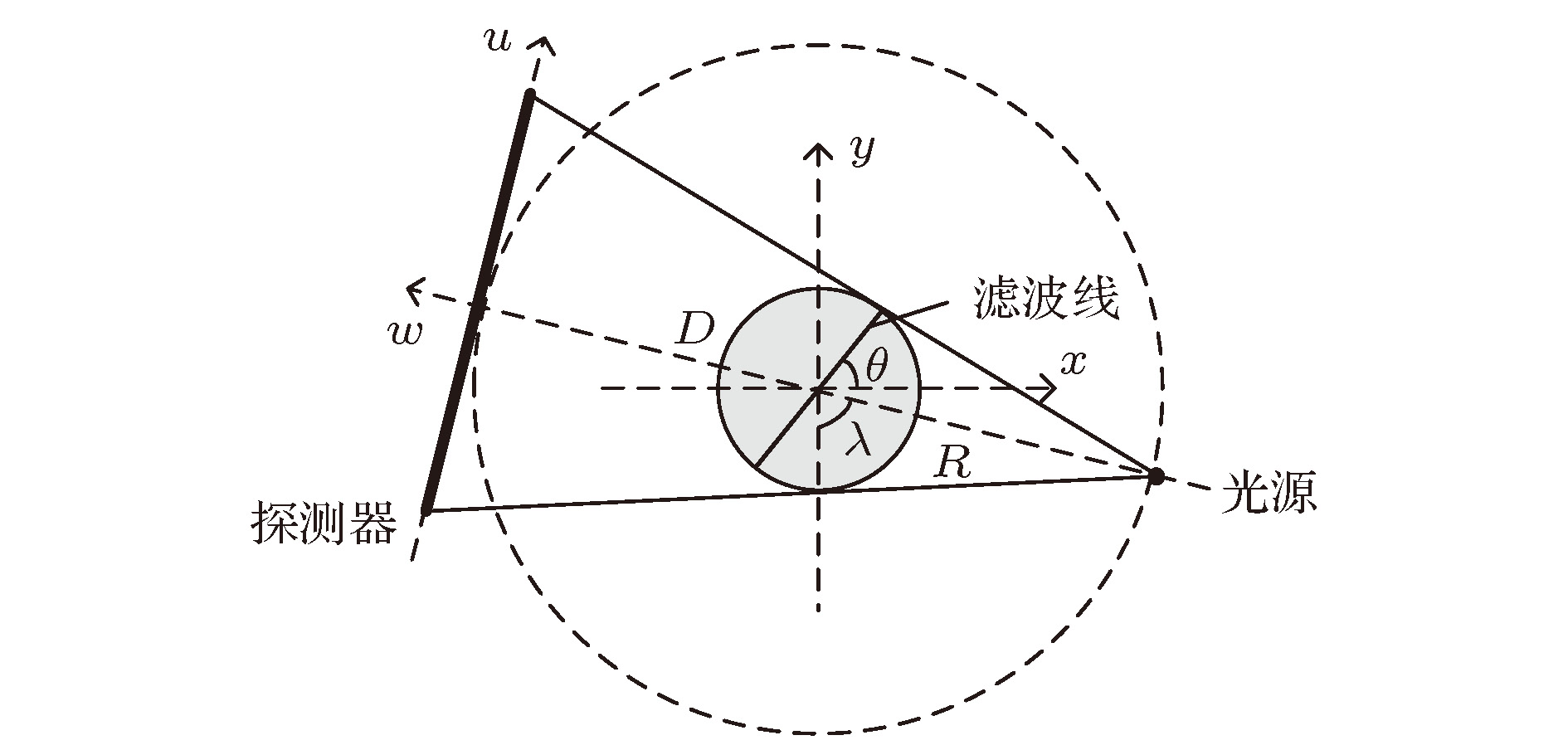
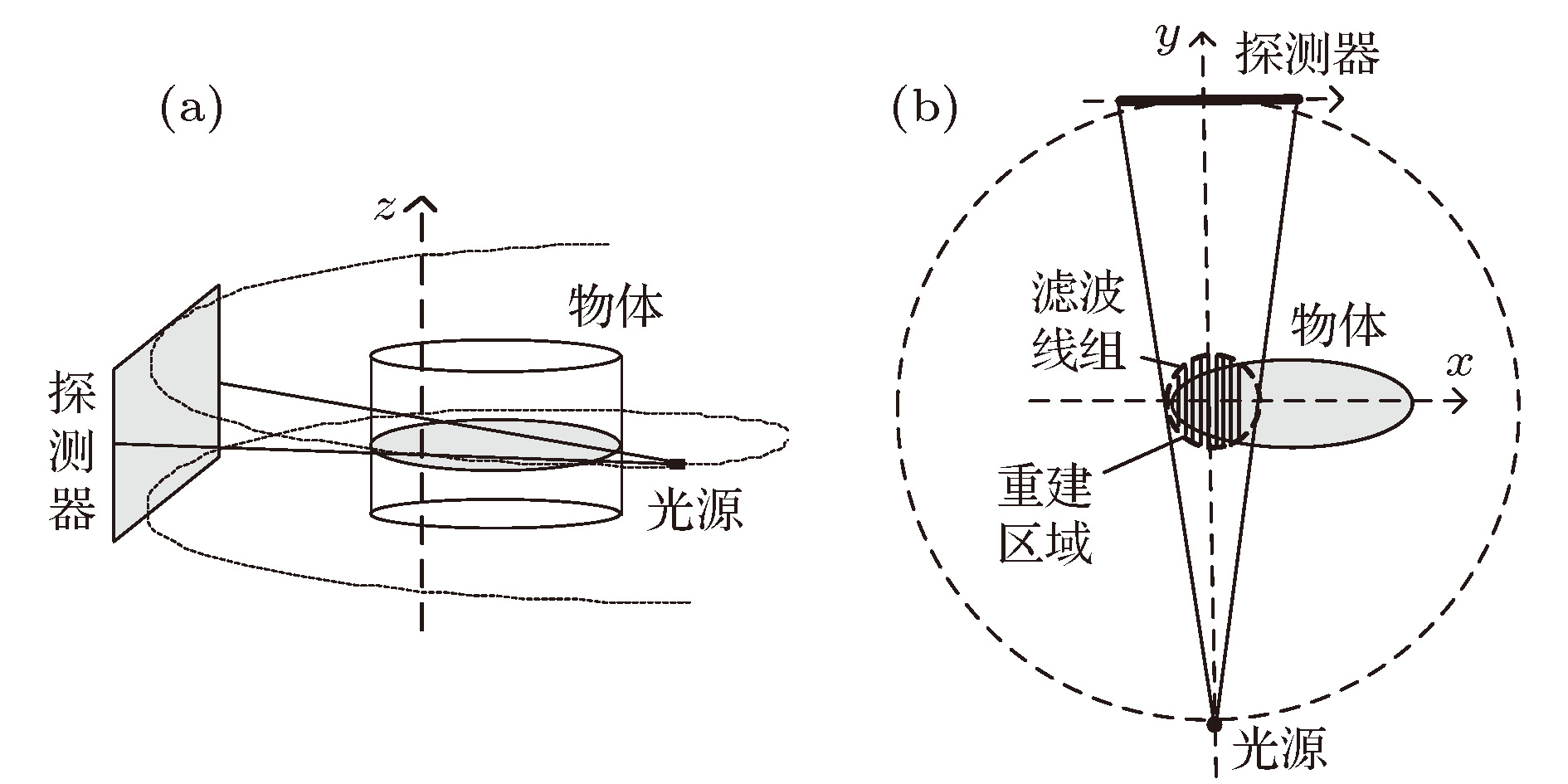
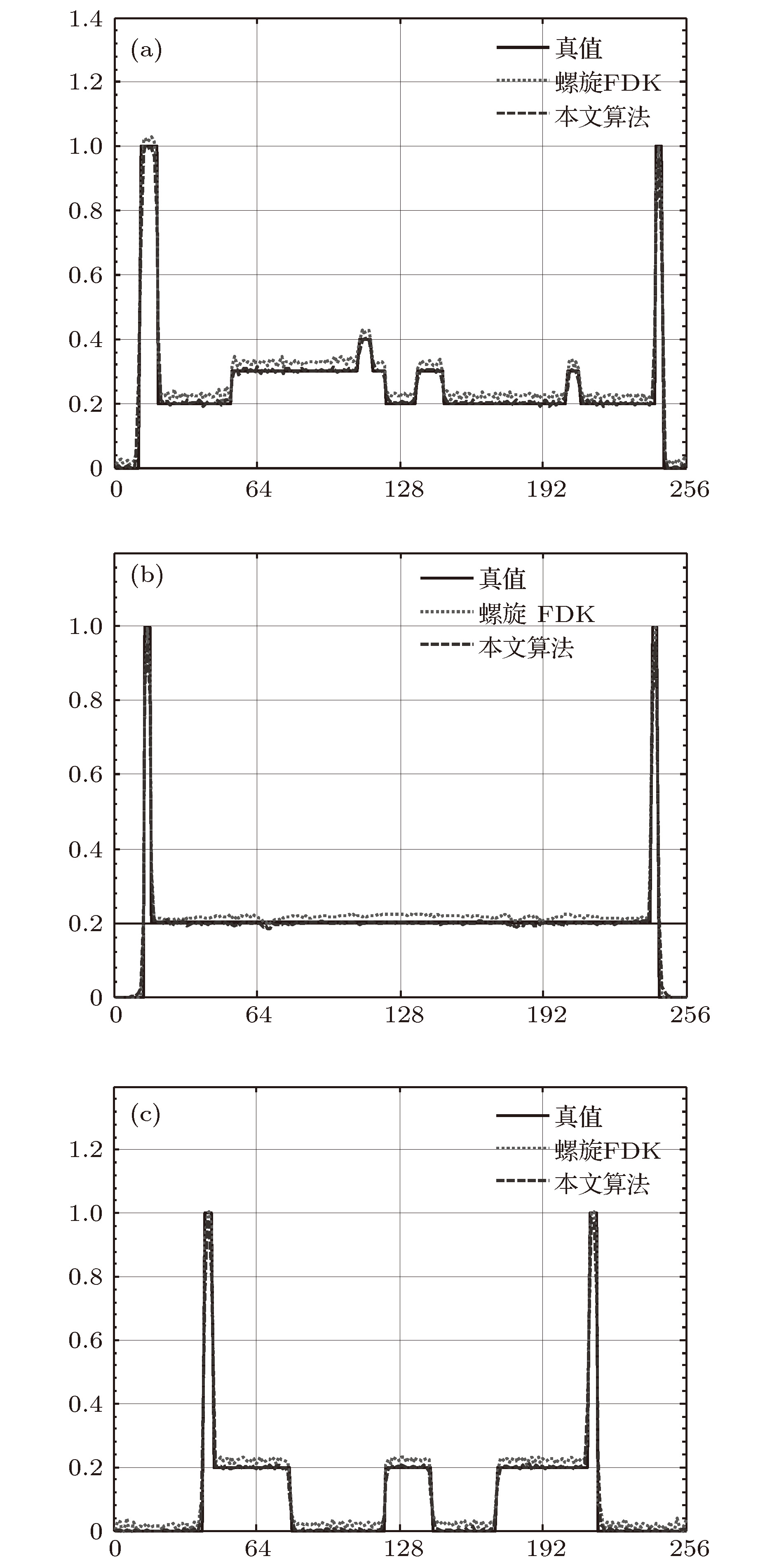
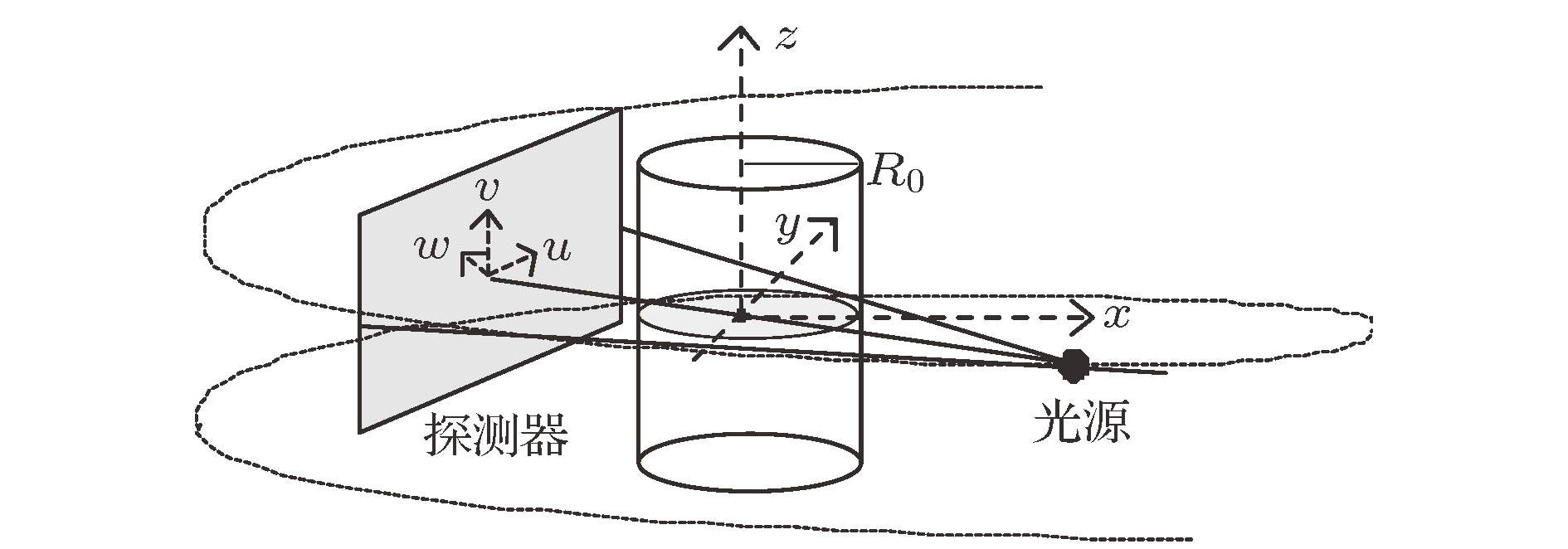
-
Dose reduction becomes one of the hot research fields in the most commonly used helical computed tomography (CT) for clinical diagnostic. Local imaging using a collimator can effectively lower the CT radiation dose by reducing the direct irradiation area. Due to the limitation of the exposing area, the projection data used for local imaging reconstruction are usually truncated, resulting in local reconstruction problems. The key in local image reconstruction is how to deal with the horizontal truncation of the projection data. The helical cone beam back-projection filtration (BPF) algorithm only needs to ensure the integrity of the projections of PI line to realize the reconstruction of the entire PI line, making local reconstruction possible. Due to the complexity and irregularity of the spatial distribution of PI lines, the existing helical BPF algorithms can only realize local surface reconstruction, whereas the local volume reconstruction is difficult. For the BPF algorithm in designing the PI line and the sampling points in helical cone beam CT and the difficulty in local volume reconstruction, the tilted fan-beam back-projection filtration (TFB-BPF) reconstruction algorithm is proposed by utilizing the weighted correction and coordinate expansion, based on the circular fan beam BPF. The algorithm divides the reconstruction area into several slices, constructs the inclined fan beam geometry for each layer, and slice-by-slice reconstruction is conducted by using the weighted modified tilted fan beam BPF algorithm. The most powerful feature of the algorithm is that the filter line, equivalent to the PI line in the original helical BPF algorithm, is selected in a two-dimensional plane. Therefore, it is more concise and efficient and can be applied to the reconstruction of local volume regions. In this paper, the helical cone beam CT imaging geometry and the original helical cone beam BPF algorithm are introduced. Then, the TFB-BPF reconstruction algorithm is deduced. Experimental results show that the algorithm can effectively realize the local volume reconstruction and overall improved image quality without obvious truncation artifacts.
-
Keywords:
- cone-beam computed tomography /
- helical trajectory /
- local reconstruction /
- back-projection filtration
[1] Huda W 2015 Curr. Raio. Rpt. 3 80
Google Scholar
[2] Wang J, Liang Z G, Lu H B, Xing L 2010 Curr. Med. Imaging Rev. 6 72
Google Scholar
[3] Ohno Y, Yaguchi A, Okazaki T, Aoyagi K, Yamagata H, Sugihara N, Koyama H, Yoshikawa T, Sugimura K 2016 Eur. J. Radio. 85 1375
Google Scholar
[4] Zheng X, Ravishankar S, Long Y, Fessler J A 2018 IEEE Trans. Med. Imaging 37 1498
[5] Shi Y Y, Yu H Y, Zhang Y B, Liu R, Kalra M, Wang G, Mou X Q 2017 the 14th International Meeting on Fully Three-Dimensional Image Reconstruction in Radiology and Nuclear Medicine Xi’An, China, June 18−23, 2017 p837
[6] Maruyama S, Fukushima Y, Miyamae Y, Koizumi K 2018 Radio. Phys. Tech. 11 235
Google Scholar
[7] Kataria B, Althén J N, Smedby Ö, Persson A, Sökjer H, Sandborg M 2018 Eur. Radiol. 28 2464
Google Scholar
[8] Yang Q, Yan P, Zhang Y, Yu H, Shi Y, Mou X Q, Kalra M K, Zhang Y, Sun L, Wang G 2018 IEEE Trans. Med. Imaging 37 1348
[9] Yang X, Andrade V D, Scullin W, Dyer E L, Kasthuri N, Carlo F D 2018 Sci. Rep. 8 2575
Google Scholar
[10] Yan B, Deng L, Han Y, Zhang F, Wang X Ch, Li L 2014 Chin. Phys. C 38 108201
Google Scholar
[11] 韩玉, 李磊, 闫镔, 席晓琦, 胡国恩 2015 64 058704
Google Scholar
Han Y, Li L, Yan B, Xi X Q, Hu G E 2015 Acta. Phys. Sin. 64 058704
Google Scholar
[12] Han Y, Yan B, Li L, Xi X Q, Hu G E 2014 IEEE Trans. Nucl. Sci. 61 2753
Google Scholar
[13] Hashemi S M, Beheshti S, Patrick R G, Narinder S P, Richard S C 2015 Comp. Math. Meth. Med. 2015 161797
Google Scholar
[14] Sidky E Y, Kao C, Pan X C 2006 J. X-ray Sci. Tech. 14 119
[15] Sidky E Y, Pan X C 2008 Phys. Med. Biol. 53 4777
Google Scholar
[16] Sidky E Y, Chartrand R, Boone J M, Pan X C 2014 IEEE J. Trans. Eng. Heal. Med. 2 1
[17] Courdurier M, Noo F, Defrise M, Kudo H 2008 Inverse. Probl. 24 065001
Google Scholar
[18] Kudo H, Courdurier M, Noo F, Defrise M 2008 Phys. Med. Biol. 53 2207
Google Scholar
[19] Ye Y, Yu H, Wei Y, Wang G 2007 J. Biol. Imaging 1 2
[20] Yu H, Ye Y, Wang G 2008 J. X-Ray Sci. Technol. 16 243
[21] Sidky E Y, Kraemer D N, Roth E G, Ullberg C, Reiser I S, Pan X 2014 J. Med. Imaging 1 031007
Google Scholar
[22] Shi Y, Mou X 2016 Proc. SPIE 9967 99671N
Google Scholar
[23] Zhao Y, Brun E, Coan P, Huang Z, Sztrókay A, Diemoz P C, Liebhardt S, Mittone A, Gasilov S, Miao J, Bravin A 2012 PNAS 109 18290
Google Scholar
[24] Zanette I, Bech M, Rack A, Duc G L, Tafforeau P, David C, Mohr J, Pfeiffer F. Weitkamp T 2012 Natl. Acad. Sci. 109 10199
Google Scholar
[25] Turbell H, Danielsson P E 1998 IEEE Nuclear Science Symposium and Medical Imaging Conference Toronto, Canada November 8−14, 1998 p8
[26] Noo F, Defrise M, Clackdoyle R 1999 Phys. Med. Biol. 44 561
Google Scholar
[27] Tang X Y, Hsieh J, Roy A N, Dutta S, Samsonov D, Hagiwara A 2006 Phys. Med. Biol. 5 855
[28] Han Y, Yan B, Yu Ch Q, Li L, Li J X, Bao S L 2012 Chin. Phys. B 21 068701
Google Scholar
[29] Katsevich A 2002 SIAM J. Appl. Math. 62 2012
[30] Zou Y, Pan X 2004 Phys. Med. Biol. 49 941
Google Scholar
[31] Zou Y, Pan X 2004 Phys. Med. Biol. 49 383
Google Scholar
[32] Zou Y, Pan X 2004 Phys. Med. Biol. 49 2717
Google Scholar
[33] Noo F, Clackdoyle R, Pack J D 2004 Phys. Med. Biol. 49 3903
Google Scholar
[34] Yu L F, Zou Y, Sidky E Y 2006 IEEE Trans. Med. Imag. 25 869
Google Scholar
[35] Wang G, Lin T H, Cheng P C, Shinozaki D M, Kim H G 1991 Proc. SPIE 1556 99
[36] Shepp L A, Logan B F 1974 IEEE Trans. Nucl. Sci. 21 21
-
图 1 螺旋锥束CT成像几何
Figure 1. Imaging geometry of helical cone-beam CT.
图 2 圆轨迹扇束CT成像几何
Figure 2. Imaging geometry of circular fan beam CT.
图 3 螺旋锥束CT几何中的待重建平面和虚拟滤波线
Figure 3. Reconstruction planes and virtual filtering lines in helical cone beam CT geometry.
图 4 单个待重建平面螺旋锥束成像几何
Figure 4. Imaging geometry of single reconstruction plane.
图 5 局部体区域成像示意图 (a)侧视图; (b)俯视图
Figure 5. Imaging schematic for local volume: (a) Side view; (b) top view.
图 6 无截断投影重建图像 (a), (b), (c)真值; (d), (e), (f)螺旋FDK算法; (g), (h), (i)本文算法
Figure 6. Reconstruction image without truncation: (a), (b), (c) True value; (d), (e), (f) helical FDK algorithm; (g), (h), (i) the proposed algorithm.
图 8 带噪声无截断投影重建图像 (a), (b), (c)螺旋FDK算法; (d), (e), (f)本文算法
Figure 8. Reconstruction image without truncated projections: (a), (b), (c) With helical FDK algorithm; (d), (e), (f) the proposed algorithm.
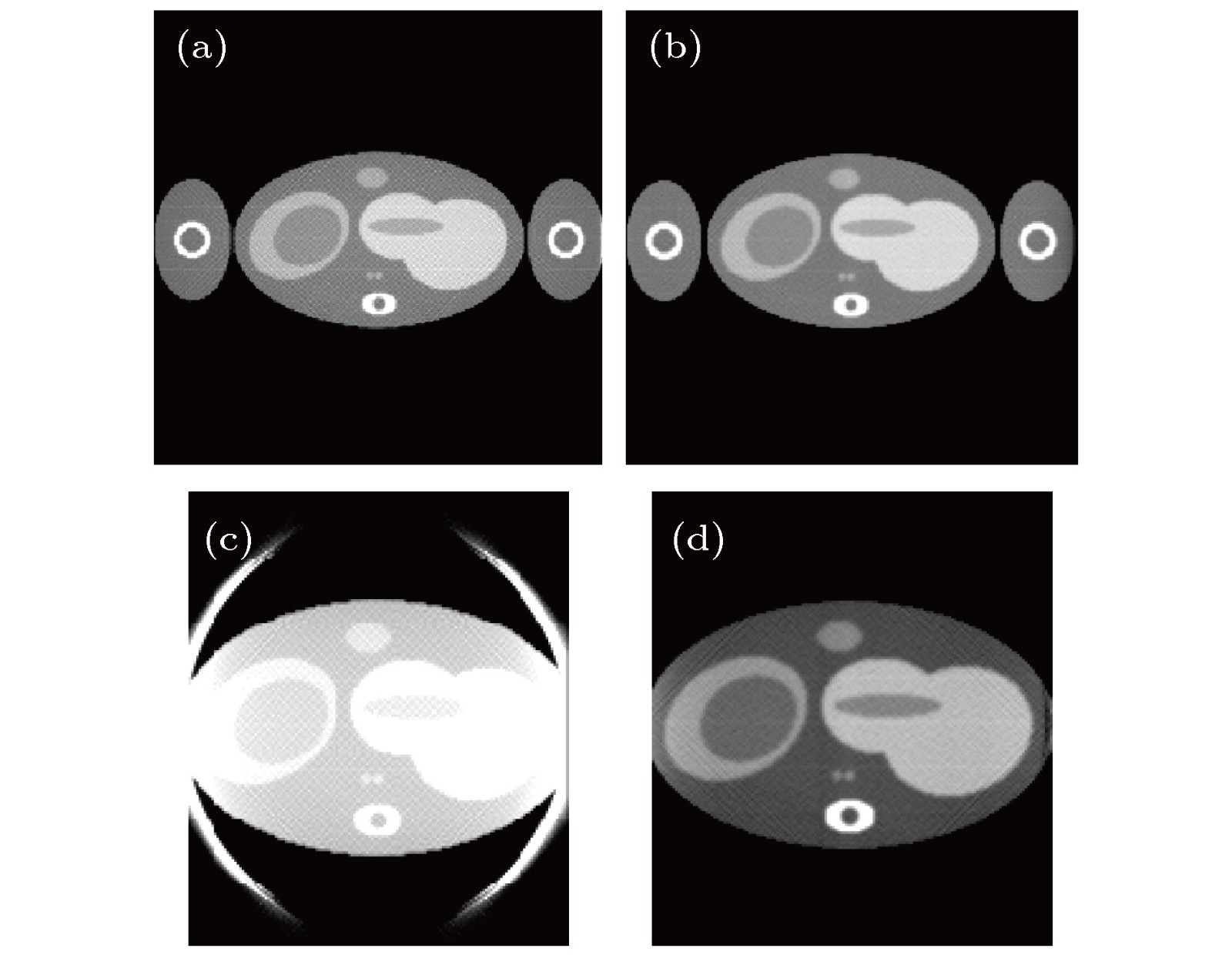
图 9 Popeye体模局部成像实验结果 (a)无截断投影数据, 螺旋FDK算法; (b)无截断投影数据, 本文算法; (c)有截断投影数据, 螺旋FDK算法; (d)有截断投影数据, 本文算法
Figure 9. Local reconstruction results for the Popeye phantom: (a) Without the truncated projection data, the helical FDK algoritm; (b) without the truncated projection data, the proposed algorithm; (c) with the truncated projection data, the helical FDK algoritm; (d) with the truncated projection data, the proposed algorithm.
-
[1] Huda W 2015 Curr. Raio. Rpt. 3 80
Google Scholar
[2] Wang J, Liang Z G, Lu H B, Xing L 2010 Curr. Med. Imaging Rev. 6 72
Google Scholar
[3] Ohno Y, Yaguchi A, Okazaki T, Aoyagi K, Yamagata H, Sugihara N, Koyama H, Yoshikawa T, Sugimura K 2016 Eur. J. Radio. 85 1375
Google Scholar
[4] Zheng X, Ravishankar S, Long Y, Fessler J A 2018 IEEE Trans. Med. Imaging 37 1498
[5] Shi Y Y, Yu H Y, Zhang Y B, Liu R, Kalra M, Wang G, Mou X Q 2017 the 14th International Meeting on Fully Three-Dimensional Image Reconstruction in Radiology and Nuclear Medicine Xi’An, China, June 18−23, 2017 p837
[6] Maruyama S, Fukushima Y, Miyamae Y, Koizumi K 2018 Radio. Phys. Tech. 11 235
Google Scholar
[7] Kataria B, Althén J N, Smedby Ö, Persson A, Sökjer H, Sandborg M 2018 Eur. Radiol. 28 2464
Google Scholar
[8] Yang Q, Yan P, Zhang Y, Yu H, Shi Y, Mou X Q, Kalra M K, Zhang Y, Sun L, Wang G 2018 IEEE Trans. Med. Imaging 37 1348
[9] Yang X, Andrade V D, Scullin W, Dyer E L, Kasthuri N, Carlo F D 2018 Sci. Rep. 8 2575
Google Scholar
[10] Yan B, Deng L, Han Y, Zhang F, Wang X Ch, Li L 2014 Chin. Phys. C 38 108201
Google Scholar
[11] 韩玉, 李磊, 闫镔, 席晓琦, 胡国恩 2015 64 058704
Google Scholar
Han Y, Li L, Yan B, Xi X Q, Hu G E 2015 Acta. Phys. Sin. 64 058704
Google Scholar
[12] Han Y, Yan B, Li L, Xi X Q, Hu G E 2014 IEEE Trans. Nucl. Sci. 61 2753
Google Scholar
[13] Hashemi S M, Beheshti S, Patrick R G, Narinder S P, Richard S C 2015 Comp. Math. Meth. Med. 2015 161797
Google Scholar
[14] Sidky E Y, Kao C, Pan X C 2006 J. X-ray Sci. Tech. 14 119
[15] Sidky E Y, Pan X C 2008 Phys. Med. Biol. 53 4777
Google Scholar
[16] Sidky E Y, Chartrand R, Boone J M, Pan X C 2014 IEEE J. Trans. Eng. Heal. Med. 2 1
[17] Courdurier M, Noo F, Defrise M, Kudo H 2008 Inverse. Probl. 24 065001
Google Scholar
[18] Kudo H, Courdurier M, Noo F, Defrise M 2008 Phys. Med. Biol. 53 2207
Google Scholar
[19] Ye Y, Yu H, Wei Y, Wang G 2007 J. Biol. Imaging 1 2
[20] Yu H, Ye Y, Wang G 2008 J. X-Ray Sci. Technol. 16 243
[21] Sidky E Y, Kraemer D N, Roth E G, Ullberg C, Reiser I S, Pan X 2014 J. Med. Imaging 1 031007
Google Scholar
[22] Shi Y, Mou X 2016 Proc. SPIE 9967 99671N
Google Scholar
[23] Zhao Y, Brun E, Coan P, Huang Z, Sztrókay A, Diemoz P C, Liebhardt S, Mittone A, Gasilov S, Miao J, Bravin A 2012 PNAS 109 18290
Google Scholar
[24] Zanette I, Bech M, Rack A, Duc G L, Tafforeau P, David C, Mohr J, Pfeiffer F. Weitkamp T 2012 Natl. Acad. Sci. 109 10199
Google Scholar
[25] Turbell H, Danielsson P E 1998 IEEE Nuclear Science Symposium and Medical Imaging Conference Toronto, Canada November 8−14, 1998 p8
[26] Noo F, Defrise M, Clackdoyle R 1999 Phys. Med. Biol. 44 561
Google Scholar
[27] Tang X Y, Hsieh J, Roy A N, Dutta S, Samsonov D, Hagiwara A 2006 Phys. Med. Biol. 5 855
[28] Han Y, Yan B, Yu Ch Q, Li L, Li J X, Bao S L 2012 Chin. Phys. B 21 068701
Google Scholar
[29] Katsevich A 2002 SIAM J. Appl. Math. 62 2012
[30] Zou Y, Pan X 2004 Phys. Med. Biol. 49 941
Google Scholar
[31] Zou Y, Pan X 2004 Phys. Med. Biol. 49 383
Google Scholar
[32] Zou Y, Pan X 2004 Phys. Med. Biol. 49 2717
Google Scholar
[33] Noo F, Clackdoyle R, Pack J D 2004 Phys. Med. Biol. 49 3903
Google Scholar
[34] Yu L F, Zou Y, Sidky E Y 2006 IEEE Trans. Med. Imag. 25 869
Google Scholar
[35] Wang G, Lin T H, Cheng P C, Shinozaki D M, Kim H G 1991 Proc. SPIE 1556 99
[36] Shepp L A, Logan B F 1974 IEEE Trans. Nucl. Sci. 21 21
DownLoad:
Catalog
Metrics
- Abstract views: 14594
- PDF Downloads: 118
- Cited By: 0
