声表面波-自旋波耦合及磁声非互易性器件

黄铭贤; 胡文彬; 白飞明

doi:10.7498/aps.73.20240462

摘要

声表面波是激发和控制自旋波的一种新兴手段, 不仅激励效率高, 而且传输长度可以达到毫米量级, 通过引入磁声耦合还可以打破时空反演对称性, 实现声表面波的非互易传播. 本文对不同类型的磁声耦合的物理机制进行了梳理, 对比了磁弹性耦合、自旋-涡度耦合(包括非磁性层注入交变自旋流和磁性材料自身的Barnett效应), 以及磁-旋转耦合在不同模式的声表面波激发下的等效驱动磁场, 讨论了这些等效驱动场的角度依赖性, 以及相应功率吸收的频率依赖性. 这为在实际应用中区分和利用各种磁声耦合机制提供了理论支持. 此外, 本文还介绍了利用磁声耦合实现声表面波非互易性传输的两种主流手段, 包括利用手性失配效应和引入具有非互易性自旋波色散关系的磁结构, 对比并讨论了它们各自的物理机制和优劣势, 希望为设计和发展基于磁声耦合的固态声学隔离器、环形器提供参考.

关键词:

Abstract

Surface acoustic wave (SAW) is a new means of exciting and controlling spin wave (SW), which has not only high excitation efficiency, but also long transmission length up to millimeter order. Based on the SAW-SW coupling (phonon-magnon coupling), a wide variety of new devices and applications such as high-sensitivity weak magnetic field sensors, energy-efficient spintronic devices, solid-state acoustic isolators, and nonreciprocal phase shifters, have been realized. Therefore, it is of great value to study the physical mechanism of magneto-acoustic coupling, develop new magneto-acoustic coupling effects, and improve the efficiency of magneto-acoustic coupling. In this work, different types of physical mechanisms of magneto-acoustic coupling are reviewed. The effective driven magnetic fields of magnetoelastic coupling, spin-vorticity coupling (including injection of alternating spin current from a non-magnetic layer and Barnett effect inside magnetic material itself), and magneto-rotation coupling under different modes of SAW excitation are compared. The angular dependence of these driven fields and the frequency dependence of the corresponding power absorption are discussed, which provides theoretical support for distinguishing and utilizing various magneto-acoustic coupling in practical applications. In addition, we also introduce two methods to realize nonreciprocal SAW transmission by magneto-acoustic coupling, including the helicity mismatch effect and nonreciprocal spin-wave dispersion magnetic structures, and discuss their physical mechanisms as well as advantages and disadvantages. For such magneto-acoustic nonreciprocal devices, the properties of higher isolation, lower insertion loss and wider bandwidth are always desired. In order to improve the properties of the devices, it is important to find magnetic structures with stronger SW nonreciprocity, reduce the insertion loss introduced by magnetic structure, and fully consider the effective driven field characteristics of different modes of SAW. We hope that this review can serve as a guide for future design and development of solid acoustic isolators and circulators in the RF and microwave frequency bands.

Keywords:

作者及机构信息

1.
电子科技大学电子科学与工程学院, 成都　610054

2.
电子科技大学, 电子薄膜与集成器件全国重点实验室, 成都　611731

通信作者: 白飞明, fmbai@uestc.edu.cn

基金项目: 国家自然科学基金(批准号: 61871081, 61271031)和四川省自然科学基金(批准号: 2022NSFSC0040)资助的课题.

Authors and contacts

1.
School of Electronic Science and Engineering, University of Electronic Science and Technology of China, Chengdu 610054, China

2.
State Key Laboratory of Electronic Thin Films and Integrated Devices, University of Electronic Science and Technology of China, Chengdu 611731, China

Corresponding author: Bai Fei-Ming, fmbai@uestc.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 61871081, 61271031) and the Natural Science Foundation of Sichuan Province of China (Grant No. 2022NSFSC0040).

文章全文 : translate this paragraph

参考文献

[1]	Takahashi S, Maekawa S 2008 J. Phys. Soc. Jpn. 77 031009 Google Scholar
[2]	Stancil D D, Prabhakar A 2009 Quantum theory of spin waves (New York: Springer Press
[3]	Uchida K I, Saitoh E 2016 Spin current (New York: John Wiley & Sons Press
[4]	Uchida K I, Adachi H, Kajiwara Y, Maekawa S, Saitoh E 2013 Solid State Phys. 64 1 Google Scholar
[5]	Adachi H, Uchida K I, Saitoh E, Maekawa S 2013 Rep. Prog. Phys. 76 036501 Google Scholar
[6]	Kruglyak V V, Demokritov S O, Grundler D 2010 J. Phys. D: Appl. Phys. 43 264001 Google Scholar
[7]	Chumak A V, Vasyuchka V I, Serga A A, Hillebrands B 2015 Nat. Phys. 11 453 Google Scholar
[8]	Reed K W, Owens J M, Carter R L 1985 Circuits Syst. Signal Press. 4 157 Google Scholar
[9]	Kim S K, Lee K S, Han D S 2009 Appl. Phys. Lett. 95 082507 Google Scholar
[10]	Wang Q, Zhong Z Y, Jin L C, Tang X L, Bai F M, Zhang H W, Beach G S D 2013 J. Magn. Magn. Mater. 340 23 Google Scholar
[11]	Chumak A V, Vasyuchka V I, Serga A A, Kostylev M P, Tiberkevich V S, Hillebrands B 2012 Phys. Rev. Lett. 108 257207 Google Scholar
[12]	Nakane R, Tanaka G, Hirose A 2018 IEEE Access 6 4462 Google Scholar
[13]	Sluka V, Schneider T, Gallardo R A, Kákay A, Weigand M, Warnatz T, Mattheis R, Roldán-Molina A, Landeros P, Tiberkevich V, Slavin A, Schütz G, Erbe A, Deac A, Lindner J, Raabe J, Fassbender J, Wintz S 2019 Nat. Nanotechnol. 14 328 Google Scholar
[14]	Jamali M, Kwon J, Seo S, Lee K J, Yang H 2013 Sci. Rep. 3 3160 Google Scholar
[15]	Chumak A, Serga A, Hillebrands B 2014 Nat. Commun. 5 4700 Google Scholar
[16]	Nikitin A A, Ustinov A B, Semenov A A 2015 Appl. Phys. Lett. 106 102405 Google Scholar
[17]	Vlaminck V, Bailleul M 2008 Science 322 410 Google Scholar
[18]	Auld B A 1973 Acoustic Fields and Waves in Solids (New York: John Wiley and Sons Press
[19]	Lewis M F 1985 Rayleigh-Wave Theory and Application (New York: Springer Press
[20]	Casals B, Statuto N, Foerster M, Hernández-Mínguez A, Cichelero R, Manshausen P, Mandziak A, Aballe L, Hernàndez J M, Macià F 2020 Phys. Rev. Lett. 124 137202 Google Scholar
[21]	Babu N K P, Trzaskowska A, Graczyk P, Centała G, Mieszczak S, Głowiński H, Zdunek M, Mielcarek S, W Kłos J 2021 Nano Lett. 21 946 Google Scholar
[22]	Küß M, Heigl M, Flacke L, Hefele A, Hörner A, Weiler M, Albrecht M, Wixforth A 2021 Phys. Rev. Appl. 15 034046 Google Scholar
[23]	Huang M X, Hu W B, Zhang H W, Bai F M 2023 J. Appl. Phys. 133 223902 Google Scholar
[24]	Zhang S, Levy P M, Fert A 2002 Phys. Rev. Lett. 88 236601 Google Scholar
[25]	Weiler M, Huebl H, Goerg F S, Czeschka F D, Gross R, Goennenwein S T B 2012 Phys. Rev. Lett. 108 176601 Google Scholar
[26]	Labanowski D, Jung A, Salahuddin S 2016 Appl. Phys. Lett. 108 022905 Google Scholar
[27]	Weiler M, Dreher L, Heeg C, Huebl H, Gross R, Brandt M S, Goennenwein S T B 2011 Phys. Rev. Lett. 106 117601 Google Scholar
[28]	Dreher L, Weiler M, Pernpeintner M, Huebl H, Gross R, Brandt M S, Goennenwein S T B 2012 Phys. Rev. B 86 134415 Google Scholar
[29]	Sasaki R, Nii Y, Iguchi Y, Onose Y 2017 Phys. Rev. B 95 020407 Google Scholar
[30]	Sasaki R, Nii Y, Onose Y 2021 Nat. Commun. 12 2599 Google Scholar
[31]	Tateno S, Nozaki Y 2020 Phys. Rev. Appl. 13 034074 Google Scholar
[32]	Hernández-Mínguez A, Macià F, Hernàndez J M, Herfort J, Santos P V 2020 Phys. Rev. Appl. 13 044018 Google Scholar
[33]	Küß M, Heigl M, Flacke L, Hörner A, Weiler M, Albrecht M, Wixforth A 2020 Phys. Rev. Lett. 125 217203 Google Scholar
[34]	Küß M, Heigl M, Flacke L, Hörner A, Weiler M, Wixforth A, Albrecht M 2021 Phys. Rev. Appl. 15 034060 Google Scholar
[35]	Verba R, Lisenkov I, Krivorotov I, Tiberkevich V, Slavin A 2018 Phys. Rev. Appl. 9 064014 Google Scholar
[36]	Verba R, Tiberkevich V, Slavin A 2019 Phys. Rev. Appl. 12 054061 Google Scholar
[37]	Shah P J, Bas D A, Lisenkov I, Matyushov A, Sun N X, Page M R 2020 Sci. Adv. 6 eabc5648 Google Scholar
[38]	Verba R, Bankowski E N, Meitzler T J, Tiberkevich V, Slavin A 2021 Adv. Electron. Mater. 7 2100263 Google Scholar
[39]	Bas D A, Verba R, Shah P J, Leontsev S, Matyushov A, Newburger M J, Sun N X, Tyberkevich V, Slavin A, Page M R 2022 Phys. Rev. Appl. 18 044003 Google Scholar
[40]	Küß M, Hassan M, Kunz Y, Hörner A, Weiler M, Albrecht M 2023 Phys. Rev. B 107 024424 Google Scholar
[41]	Küß M, Hassan M, Kunz Y, Hörner A, Weiler M, Albrecht M 2023 Phys. Rev. B 107 214412 Google Scholar
[42]	Küß M, Glamsch S, Kunz Y, Hörner A, Weiler M, Albrecht M 2023 ACS Appl. Electron. Mater. 5 5103 Google Scholar
[43]	Huang M X, Liu Y Y, Hu W B, Wu Y T, Wang W, He W, Zhang H W, Bai F M 2024 Phys. Rev. Appl. 21 014035 Google Scholar
[44]	Chudnovsky E M, Jaafar R 2016 Phys. Rev. Appl. 5 031002 Google Scholar
[45]	Kobayashi D, Yoshikawa T, Matsuo M, Iguchi R, Maekawa S, Saitoh E, Nozaki Y 2017 Phys. Rev. Lett. 119 077202 Google Scholar
[46]	Takahashi R, Matsuo M, Ono M, Harii K, Chudo H, Okayasu S, Ieda J, Takahashi S, Maekawa S, Saitoh E 2015 Nat. Phys. 12 52 Google Scholar
[47]	Matsuo M, Ieda J I, Harii K, Saitoh E, Maekawa S 2013 Phys. Rev. B 87 180402 Google Scholar
[48]	Matsuo M, Ohnuma Y, Maekawa S 2017 Phys. Rev. B 96 020401 Google Scholar
[49]	Tateno S, Okano G, Matsuo M, Nozaki Y 2020 Phys. Rev. B 102 104406 Google Scholar
[50]	Kurimune Y, Matsuo M, Maekawa S, Nozaki Y 2020 Phys. Rev. B 102 174413 Google Scholar
[51]	Huang M X, Hu W B, Zhang H W, Bai F M 2023 Phys. Rev. B 107 134401 Google Scholar
[52]	Kurimune Y, Matsuo M, Nozaki Y 2020 Phys. Rev. Lett. 124 217205 Google Scholar
[53]	Xu M, Yamamoto K, Puebla J, Baumgaertl K, Rana B, Miura K, Takahashi H, Grundler D, Maekawa S, Otani Y 2020 Sci. Adv. 6 eabb1724 Google Scholar
[54]	Landau L D, Lifshitz E M 1935 Phys. Z. Sowietunion 8 153
[55]	Gilbert T L 1955 Phys. Rev. 100 1243
[56]	Einstein A, de Haas W J 1915 Verhandlungen 17 152
[57]	Barnett S J 1915 Phys. Rev. 6 239 Google Scholar
[58]	Maekawa S, Tachiki M 1976 AIP Conf. Proc. 29 542 Google Scholar
[59]	Eshbach J R, Damon R W 1960 Phys. Rev. 118 1208 Google Scholar
[60]	Melkov G A, Vasyuchka V I, Lazovskiy V V, Tiberkevich V S, Slavin A N 2006 Appl. Phys. Lett. 89 252510 Google Scholar
[61]	Mruczkiewicz M, Krawczyk M, Gubbiotti G, Tacchi S, Filimonov Yu A, Kalyabin D V, Lisenkov I V, Nikitov S A 2013 New J. Phys. 15 113023 Google Scholar
[62]	Verba R, Tiberkevich V, Bankowski E, Meitzler T, Melkov G, Slavin A 2013 Appl. Phys. Lett. 103 082407 Google Scholar
[63]	Lisenkov I, Tyberkevych V, Slavin A, Bondarenko P, Ivanov B A, Bankowski E, Meitzler T, Nikitov S 2014 Phys. Rev. B 90 104417 Google Scholar
[64]	Dmytriiev O, Meitzler T, Bankowski E, Slavin A, Tiberkevich V 2010 J. Phys.: Condens. Matter 22 136001 Google Scholar
[65]	Gallardo R A, Alvarado-Seguel P, Schneider T, Gonzalez-Fuentes C, Roldán-Molina A, Lenz K, Lindner J, Landeros P 2019 New J. Phys. 21 033026 Google Scholar
[66]	Matsumoto H, Kawada T, Ishibashi M, Kawaguchi M, Hayashi M 2022 Appl. Phys. Express 15 063003 Google Scholar
[67]	Hu W B, Huang M X, Wu Y T, Jia Y N, Wang W, Bai F M 2024 Appl. Phys. Lett. 124 182405 Google Scholar
[68]	Küß M, Glamsch S, Hörner A, Albrecht M 2024 ACS Appl. Electron. Mater. 6 1790 Google Scholar
[69]	Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2015 Science 349 405 Google Scholar
[70]	Lachance-Quirion D, Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Nakamura Y 2017 Sci. Adv. 3 e1603150 Google Scholar
[71]	Satzinger K J, Zhong Y P, Chang H S, Peairs G A, Bienfait A, Chou M H, Cleland A Y, Conner C R, DumurÉ, Grebel J, Gutierrez I, November B H, Povey R G, Whiteley S J, Awschalom D D, Schuster D I, Cleland A N 2018 Nature 563 661 Google Scholar
[72]	Whiteley S J, Wolfowicz G, Anderson C P, Bourassa A, Ma H, Ye M, Koolstra G, Satzinger K J, Holt M V, Heremans F J, Cleland A N, Schuster D I, Galli G, Awschalom D D 2019 Nat. Phys. 15 490 Google Scholar
[73]	An K, Litvinenko A N, Kohno R, Fuad A A, Naletov V V, Vila L, Ebels U, de Loubens G, Hurdequint H, Beaulieu N, Ben Youssef J, Vukadinovic N, Bauer G E W, Slavin A N, Tiberkevich V S, Klein O 2020 Phys. Rev. B 101 060407(R Google Scholar
[74]	Chu Y, Kharel P, Yoon T, Frunzio L, Rakich P T, Schoelkopf R J 2018 Nature 563 666 Google Scholar
[75]	Bombeck M, Salasyuk A S, Glavin B A, Scherbakov A V, Brüggemann C, Yakovlev D R, Sapega V F, Liu X, Furdyna J K, Akimov A V, Bayer M 2012 Phys. Rev. B 85 195324 Google Scholar
[76]	Scherbakov A V, Salasyuk A S, Akimov A V, Liu X, Bombeck M, Brüggemann C, Yakovlev D R, Sapega V F, Furdyna J K, Bayer M 2010 Phys. Rev. Lett. 105 117204 Google Scholar
[77]	Polewczyk V, Dumesnil K, Lacour D, Moutaouekkil M, Mjahed H, Tiercelin N, Petit Watelot S, Mishra H, Dusch Y, Hage-Ali S, Elmazria O, Montaigne F, Talbi A, Bou Matar O, Hehn M 2017 Phys. Rev. Appl. 8 024001 Google Scholar
[78]	Liu X, Tong B, Ou-Yang J, Yang X, Chen S, Zhang Y, Zhu B 2018 Appl. Phys. Lett. 113 082402 Google Scholar
[79]	Kittmann A, Durdaut P, Zabel S, Reermann J, Schmalz J, Spetzler B, Meyners D, Sun N X, McCord J, Gerken M, Schmidt G, Höft M, Köchel R, Faupel F, Quandt E 2018 Sci. Rep. 8 278 Google Scholar
[80]	Hu W B, Huang M X, Xie H P, Zhang H W, Bai F M 2023 Phys. Rev. Appl. 19 014010 Google Scholar
[81]	Hu W B, Wang Y D, Huang M X, Zhang H W, Bai F M 2023 Appl. Phys. Lett. 123 012406 Google Scholar
[82]	Thevenard L, Camara I S, Majrab S, Bernard M, Rovillain P, Lemaître A, Gourdon C, Duquesne J Y 2016 Phys. Rev. B 93 134430 Google Scholar
[83]	Davis S, Baruth A, Adenwalla S 2010 Appl. Phys. Lett. 97 232507 Google Scholar
[84]	Al Misba W, Rajib M M, Bhattacharya D, Atulasimha J 2020 Phys. Rev. Appl. 14 014088 Google Scholar
[85]	Biswas A K, Bandyopadhyay S, Atulasimha J 2013 Appl. Phys. Lett. 103 232401 Google Scholar
[86]	Roe A, Bhattacharya D, Atulasimha J 2019 Appl. Phys. Lett. 115 112405 Google Scholar
[87]	Yang H F, Garcia-Sanchez F, Hu X K, Sievers S, Böhnert T, Costa J D, Tarequzzaman M, Ferreira R, Bieler M, Schumacher H W 2018 Appl. Phys. Lett. 113 072403 Google Scholar
[88]	Zhang D L, Zhu J, Qu T, Lattery D M, Victora R H, Wang X, Wang J P 2020 Sci. Adv. 6 eabb4607 Google Scholar
[89]	Iihama S, Xu Y, Deb M, Malinowski G, Hehn M, Gorchon J, Fullerton E E, Mangin S 2018 Adv. Mater. 30 1804004 Google Scholar
[90]	van Hees Y L W, van de Meugheuvel P, Koopmans B, Lavrijsen R 2020 Nat. Commun. 11 3835 Google Scholar
[91]	Edrington W, Singh U, Dominguez M A, Alexander J R, Nepal R, Adenwalla S 2018 Appl. Phys. Lett. 112 052402 Google Scholar
[92]	Dean J, Bryan M T, Cooper J D, Virbule A, Cunningham J E, Hayward T J 2015 Appl. Phys. Lett. 107 142405 Google Scholar
[93]	Wei Y, Li X, Gao R, Wu H, Wang X, Zeng Z, Wang J, Liu Q 2020 J. Magn. Magn. Mater. 502 166546 Google Scholar
[94]	Chen C, Fu S, Han L, Su R, Liu P, Chen R, Zhu W, Liao L, Pan F, Song C 2022 Adv. Electron. Mater. 8 2200593 Google Scholar
[95]	Yokouchi T, Sugimoto S, Rana B, Seki S, Ogawa N, Kasai S, Otani Y 2020 Nat. Nanotechnol. 15 361 Google Scholar
[96]	Yang W G, Schmidt H 2020 Appl. Phys. Lett. 116 212401 Google Scholar
[97]	Yang W G, Jaris M, Berk C, Schmidt H 2019 Phys. Rev. B 99 104434 Google Scholar
[98]	Matsuda O, Tsutsui K, Vaudel G, Pezeril T, Fujita K, Gusev V 2020 Phys. Rev. B 101 224307 Google Scholar
[99]	Chen R, Chen C, Han L, Liu P, Su R, Zhu W, Zhou Y, Pan F, Song C 2023 Nat. Commun. 14 4427 Google Scholar

施引文献

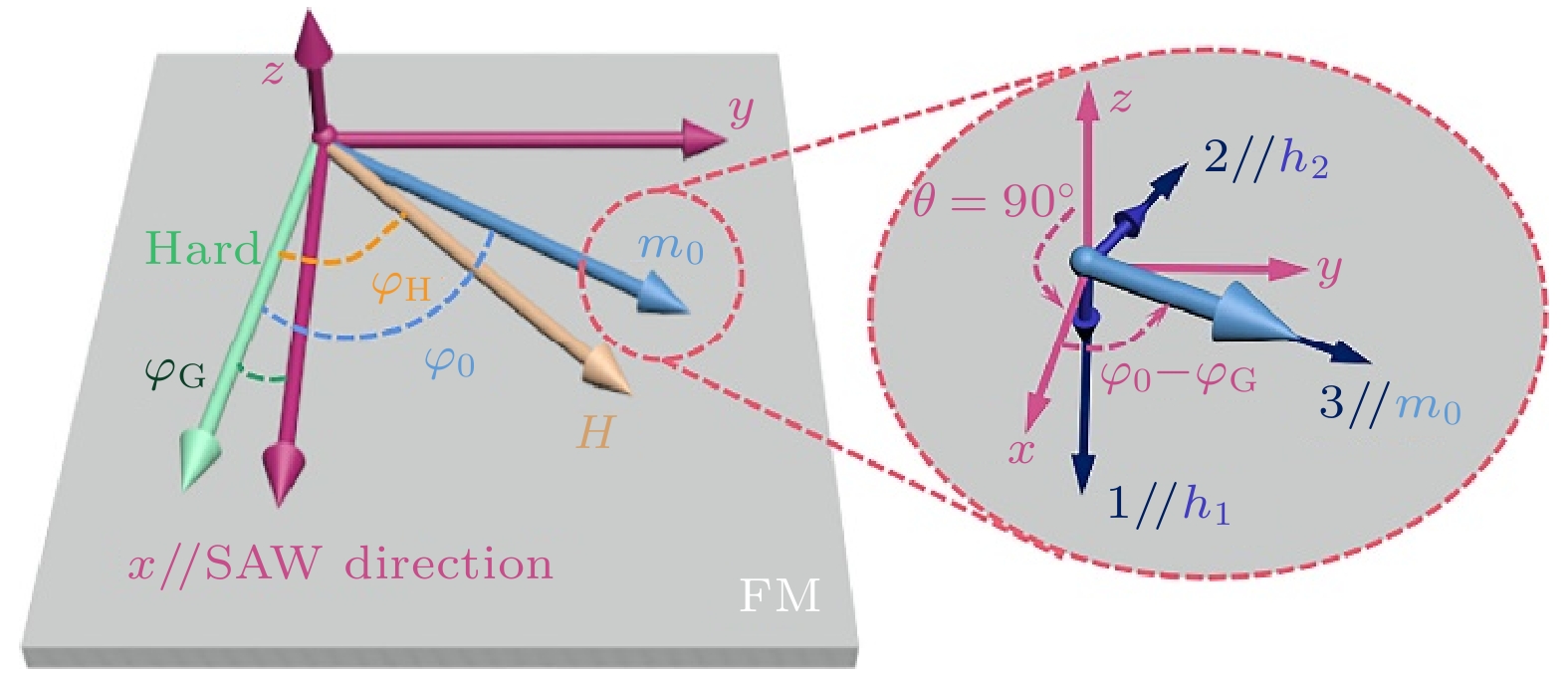
图 1 求解LLG方程的坐标系设置. 插图显示了所采用的两个坐标系之间的关系. (x, y, z)坐标系由声表面波的传播方向、磁膜的面内横向方向和法线方向组成. (1, 2, 3)坐标系可以通过沿洋红色虚线箭头旋转(x, y, z)坐标系获得, 其中3轴平行于平衡状态下的磁化m₀方向, 而1轴和2轴分别对于h₁和h₂方向^[51]

Fig. 1. Coordinate system setting for solving LLG equation. The inset shows relation between the two coordinate systems employed. The (x, y, z) coordinate system consists of the propagation direction of the SAW, the transverse in-plane direction, and the normal of the FM film. In the (1, 2, 3) coordinate system, which can be obtained by rotating the (x, y, z) coordinate system along the magenta dotted arrows, the 3-axis is parallel to the m₀ direction, whereas the 1- and 2-axis parallel to the h₁ and h₂ directions, respectively^[51]

下载: 全尺寸图片幻灯片

图 2 (a) 弹性应变驱动铁磁共振的实验设计与坐标系统说明, 右边展示了镍薄膜中的应变ε^[27]; (b) 声表面波传输的幅值和相位随外加磁场的大小和方向的变化^[27]; (c) 相干弹性波产生自旋泵浦的实验装置及坐标系说明^[25]; (d), (e) ΔP_IDT和ΔV_DC关于SAW(空心符号)以及EMW(实心符号)脉冲的检测结果^[25]

Fig. 2. (a) Illustration of elastically driven ferromagnetic resonance experimental setup and coordinate system. The close up to the right shows the strain ε in the nickel thin film^[27]. (b) Evolution of the amplitude and phase for SAW transmission as a function of the magnitude and orientation of the external magnetic field^[27]. (c) Illustration of spin pumping with coherent elastic waves experimental setup and coordinate system^[25]. (d), (e) ΔP_IDT and ΔV_DC for the detection of the SAW (open symbols) and the EMW (solid symbols) pulses^[25].

下载: 全尺寸图片幻灯片

图 3 (a)—(c) 瑞利波、SH波和LL波的应变幅值ε_xx, ε_xy和ε_xz的有限元仿真结果; (d) Al (5 nm)/Ni (10 nm)/LiTaO₃的器件结构示意图^[22]; (e) 瑞利波在4.47 GHz下ΔS₂₁, ΔS₁₂以及ΔS₂₁ – ΔS₁₂的实验数据^[22]; (f) SH波在3.47 GHz下ΔS₂₁, ΔS₁₂以及ΔS₂₁–ΔS₁₂的实验数据^[22]; (g) Ni (20 nm)/ST切石英的器件结构示意图^[23]; (h), (i) R3和LL3在不同外加磁场下的归一化功率吸收测试图^[23]

Fig. 3. (a)–(c) The FEM eigenfrequency simulation results of the magnitude of the strain ε_xx, ε_xy and ε_xz for R-, SH- and LL-waves; (d) schematic illustration of the experimental setup for Al (5 nm)/Ni (10 nm)/LiTaO₃^[22]; (e) the experimental data ΔS₂₁, ΔS₁₂ and ΔS₂₁ – ΔS₁₂ of the R wave at 4.47 GHz^[22]; (f) the experimental data ΔS₂₁, ΔS₁₂ and ΔS₂₁–ΔS₁₂ of the SH wave at 3.47 GHz^[22]; (g) schematic experimental setup for Ni (20 nm)/Quartz ST^[23]; (h), (i) polar plots of measured field-dependent normalized power absorption of the R3 (h) and LL3 (i)^[23].

下载: 全尺寸图片幻灯片

图 4 (a) SAW诱导自旋流产生的机制示意图^[47]; (b) 导体表面产生的自旋积累^[47]; (c), (d) 在R-SAW激励下通过SVC产生的SC (c)以及由SC注入导致的SWR产生MW吸收(d)的示意图^[45]; (e) 测试SWR产生的MW吸收的实验设置图^[45]; (f) Cu/NiFe/LiNbO₃器件的光学照片^[45]; (g) 归一化MW吸收在SAWs谐振峰处的角度依赖性^[45]; (h) 归一化MW吸收在SAWs谐振峰处随Cu层厚度的变化^[45]; (i) RSAW诱导Cu(200 nm)/ NiFe(20 nm)/Pt(10 nm)中自旋泵浦的实验设置^[49]; (j) 微波吸收随频率以及外加磁场的变化^[49]; (k) P₂₁在外加磁场为20 mT时随频率的变化^[49]; (l) 微波吸收以及 (m) 霍尔电压在SAW基频处随外加磁场的变化^[49]

Fig. 4. (a) Snapshot of mechanical generation of spin current induced by SAW^[47]; (b) spin accumulation induced on the surface^[47]; (c), (d) schematic illustrations of (c) SC generation via SVC in R-SAW and (d) MW absorption owing to SWR excitation caused by SC injection^[45]; (e) schematic experimental setup for measuring MW absorption caused by the SWR excitation^[45]; (f) optical photograph of the Cu/NiFe/LiNbO₃ device^[45]; (g) angular dependence of the peak value of normalized MW absorption^[45]; (h) Cu thickness dependence of the peak value of normalized MW absorption^[45]; (i) experimental setup of RSAW-induced spin pumping in Cu(200 nm) /NiFe(20 nm)/Pt(10 nm)^[49]; (j) color plots of microwave absorption in the external field versus the frequency plane^[49]; (k) P₂₁ at 20 mT as a function of frequency^[49]; (l) microwave absorptions and (m) Hall voltages measured at each fundamental frequency of the RSAW^[49].

下载: 全尺寸图片幻灯片

图 5 (a), (b) 由R-SAW (a)和SH-SAW (b)通过SVC激发NM/FM结构中的自旋波共振(SWR)的示意图^[51]; (c), (d) R-SAW (c)和SH-SAW (d)激发的SWR吸收功率$ {P_{{\mathrm{abs}}}} $随外加磁场变化的计算极坐标图^[51]; (e) 不同频率的SH-SAWs激发下归一化功率吸收随外加磁场的变化^[51]; (f) SH-SAWs激发的归一化吸收功率的频率依赖性^[51]

Fig. 5. (a), (b) Schematic illustration of SWR in the NM/FM structure excited by the R-SAW (a) and SH-SAW (b) via SVC^[51]; (c), (d) polar plot of the calculated SWR power absorption $ {P_{{\mathrm{abs}}}} $ excited by R-SAW (c) and SH-SAW (d) as a function of external magnetic fields^[51]; (e) field dependent normalized power absorption of SH-SAWs measured at different frequencies^[51]; (f) the frequency dependent normalized power absorption of SH-SAWs^[51].

下载: 全尺寸图片幻灯片

图 6 (a) 瑞利波在铁磁体激发的Barnett场的示意图^[52]; (b) Barnett场引起的SAW功率吸收随外加磁场以及角度的变化示意图^[52]; (c) 在NiFe/Cu, NiFe/Pt, NiFe/Ti, NiFe (ϕ = 0)和Ni (ϕ = π/4)结构中声功率吸收的R-SAW频率依赖性^[50]; (d) 磁-旋转耦合的示意图^[53]; (e) 在自旋波共振条件下, ±k方向传播的SAW功率吸收P_±k对比^[53]

Fig. 6. (a) Schematic illustration of Barnett field excited by Rayleigh waves in a ferromagnet^[52]; (b) the angular dependence of SAW power absorption in NiFe films caused by Barnett field^[52]; (c) R-SAW-frequency dependence of the MW absorption in NiFe/Cu, NiFe/Pt, NiFe/Ti, NiFe (ϕ = 0) and Ni (ϕ = π/4)^[50]; (d) schematics of the magneto-rotation coupling^[53]; (e) attenuation of acoustic waves near a spin-wave resonance condition for SAW propagating along +k and –k directions^[53].

下载: 全尺寸图片幻灯片

图 7 (a) 沉积在LiNiO₃衬底上的Si/Ni双层膜结构通过磁弹性耦合产生非互易性^[31]; (b) P_–H/P_+H随ε_xz/ε_xx的变化^[31]; (c) Ni(20 nm)/Si(400 nm)双层膜结构在外加磁场沿π/6处的归一化吸收功率ΔP ^norm[31]; (d) P_–H/P_+H随Si层厚度的变化^[31]

Fig. 7. (a) Experimental setup of nonreciprocal SW generation via magnetoelastic coupling in a Si/Ni bilayer deposited on LiNiO₃ substrate^[31]; (b) P_–H/P_+H as a function of ε_xz/ε_xx^[31]; (c) ΔP ^norm of Ni(20 nm)/Si(400 nm) bilayer with the external field at π/6^[31]; (d) P_–H/P_+H as a function of Si thickness^[31].

下载: 全尺寸图片幻灯片

图 8 (a), (b)两种非互易SW与AW发生磁弹性耦合的方法说明, 其中左列为AWs和SW的色散曲线, 图(a)中的插图展示了不同大小的能隙Δf; 右列为不同方向的AW传输参数^[36]

Fig. 8. (a), (b) An illustration of two methods of inducing nonreciprocity of an AW by magnetoelastic coupling with a SW. Left column, spectra of AWs and SWs, the inset in panel (a) shows the opening of the magnetoelastic gap of different Δf; right column, AW transmission rates in opposite directions^[36].

下载: 全尺寸图片幻灯片

图 9 (a) LiNbO₃/CoFeB和LiNbO₃/CoFeB/Pt器件结构示意图; (b), (c) CoFeB(2 nm)及CoFeB(2 nm)/Pt两种结构对应的SAW传输曲线图^[33]

Fig. 9. (a) Schematic illustration of the experimental setup for LiNbO₃/CoFeB and LiNbO₃/CoFeB/Pt devices; (b), (c) the SAW transmission curves of CoFeB(2 nm) and CoFeB(2 nm)/Pt respectively^[33].

下载: 全尺寸图片幻灯片

图 10 (a) 60°时计算得到的FeGaB(20 nm)/Al₂O₃(5 nm)/FeGaB(20 nm)结构磁弹性波的正向和反向传播的线性损耗随随外加磁场的变化^[37]; (b) CoFeB(16 nm)/Ru(0.55 nm)/CoFeB(5 nm)结构的SAW传输参数ΔS_ij在5.08 GHz沿ϕ_H= 29°随外磁场大小的变化^[42]; (c) NiFeCu/FeCoSiB磁弹双层膜结构示意图^[43]; (d) 在2.33 GHz下沿φ_H – φ_G = 5°改变磁场测得的FeCoSiB(10 nm)/NiFeCu(10 nm)双层膜样品的$\left| {{S_{ij}}} \right|$^[43]

Fig. 10. (a) Calculated linear loss of FeGaB(20 nm)/Al₂O₃(5 nm)/FeGaB(20 nm) for the magnetoelastic wave as a function of the applied magnetic field for forward and backward propagation for H_G = 60°^[37]; (b) change of the SAW transmission ΔS_ij of a CoFeB(16 nm)/Ru(0.55 nm)/CoFeB(5 nm) magnetic bilayer sample as a function of magnitude of the external magnetic field at 5.08 GHz along ϕ_H= 29°^[42]; (c) a NiFeCu/FeCoSiB magnetoelastic bilayer structure^[43]; (d) measured $\left| {{S_{ij}}} \right|$ of FeCoSiB(10 nm)/NiFeCu(10 nm) bilayer under different magnetic fields at 2.33 GHz (SH₉) along angles φ_H – φ_G = 5°^[43].

下载: 全尺寸图片幻灯片

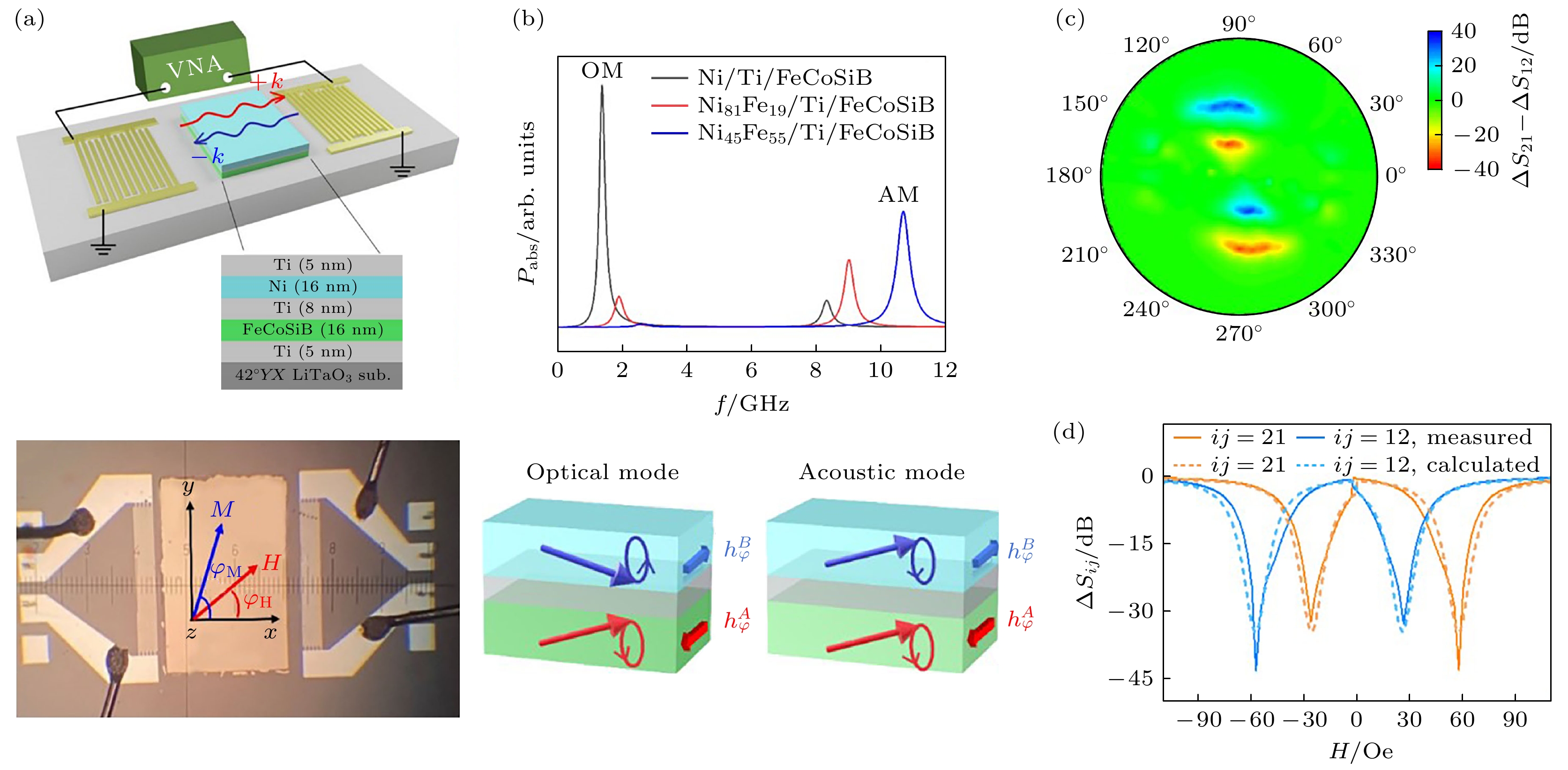
图 11 (a) 基于Ni(16 nm)/Ti(8 nm)/FeCoSiB(16 nm)异质结构的SH-SAW延迟线示意图和实物图^[67]; (b) Ni/Ti/FeCoSiB, Ni₈₁Fe₁₉/Ti/FeCoSiB和Ni₄₅Fe₅₅/Ti/FeCoSiB三种构型薄膜的SAW功率吸收计算结果, 下图示意性画出了Ni/Ti/FeCoSiB结构中的光学模和声学模的磁化进动, 其中顶层和底层中的等效驱动场总是反平行的^[67]; (c) 在2.33 GHz下沿不同方向施加磁场测试得到的 ΔS₂₁–ΔS₁₂^[67]; (d) 沿φ_H = 90°改变磁场的ΔS_ij测试(实线)和计算(虚线)结果^[67]

Fig. 11. (a) Schematic illustration and optical image of a SH-SAW delay line based on a Ni(16 nm)/Ti(8 nm)/FeCoSiB(16 nm) heterostructure^[67]; (b) calculated normalized SAW power absorption for Ni/Ti/FeCoSiB, Ni₈₁Fe₁₉/Ti/FeCoSiB and Ni₄₅Fe₅₅/Ti/FeCoSiB configurations, and the insets on the lower panel illustrate optical and acoustic resonance modes for the anti-magnetostrictive Ni/Ti/FeCoSiB configuration, where the effective driving fields in the top and bottom layers are always antiparallel^[67]; (c) polar plots of the measured nonreciprocal transmission ΔS₂₁–ΔS₁₂ as a function of applied field $ H $ and field angle $ {\varphi }_{H} $^[67]; (d) measured (solid lines) and calculated (dashed lines) field-dependent ΔS_ij along φ_H = 90°^[67].

下载: 全尺寸图片幻灯片

图 12 (a) 实现宽频非互易磁弹性耦合的方法说明. 左图为声表面波(绿色实线)和非互易自旋波(蓝色虚线)的色散曲线; 右图为不同方向的传输参数随频率的变化^[36]; (b)扣除背底后的传输参数ΔS₂₁随外加磁场的变化^[68]; (c)宽频范围内, 在饱和磁场–400 mT和最佳偏置磁场–13.8 mT下, 沿ϕ_H= 85.5°角度方向测得的声表面波传输参数幅值Mag[S_ij(f )]^[68]

Fig. 12. (a) Description of the method for realizing broadband non-reciprocal magnetoelastic coupling: left column, spectra of SAWs and SWs; right column, SAW transmission rates in opposite directions^[36]. (b) The background-corrected transmission ΔS₂₁ of counter-propagating waves as a function of the external magnetic field magnitude^[68]. (c) Over a wide range of frequencies, the SAW transmission magnitude Mag[S_ij(f )] at –13.8 mT and –400 mT along ϕ_H= 85.5°^[68].

下载: 全尺寸图片幻灯片

表 1 在各种模式SAWs激发下的三种不同类型磁声耦合的特征

Table 1. Comparison of three magnon-phonon coupling characteristics excited by different types of SAWs.

耦合类型	SAWs模式	应变场分量	方向	相位	等效驱动磁场的角度依赖性	功率吸收的频率依赖性
磁弹性耦合	R^[28]	ε_xx	面内	i	$ \sin 2\left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f
	R^[28]	ε_xz	面外	1	$ \cos \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f ³
	SH^[22]	ε_xy	面内	/	$ \cos 2\left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f
	LL^[23]	ε_xx	面内	/	$ \sin 2\left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f
自旋-涡度耦合-非磁性层	R^[45]	$ J_{\mathrm{s}}^Y $	面外	/	$ \cos \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f⁷
	SH^[51]	$ J_{\mathrm{s}}^X $	面外	i	$ \sin \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f⁷
	SH^[51]	$ J_{\mathrm{s}}^Z $	面内	1	1	f⁵
自旋-涡度耦合-Barnett场	R^[52]	Ω^y	面内	/	$ \cos \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f³
	SH^[52]	Ω^x	面内	i	$ \sin \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f³
	SH^[52]	Ω^z	面外	1	1	f⁵
磁-旋转耦合	R^[53]	$ {\omega _{xz}} $	面外	/	$ \cos \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f³
磁-旋转耦合	SH^[53]	$ {\omega _{yz}} $	面外	/	$ \sin \left( {{\varphi _0} - {\varphi _{\mathrm{G}}}} \right) $	f³
注: “/”表示在只有一种驱动场分量的情况下, 无相对的相位差异. fⁿ表示其与频率的n次方成正比.

下载: 导出CSV

表 2 文献报道的磁声器件的SAWs传输非互易性ΔS_±、磁声插入损耗IL_Δ和声延迟线本身的插入损耗IL₀

Table 2. Reported SAWs transmission nonreciprocity ΔS_±, magnetoacoustic insertion loss IL_Δ, and insertion loss of the acoustic delay line IL₀ for magnetoacoustic hybrid devices in literature.

磁结构/nm	非互易起源	f/GHz	l_f/mm	IL₀/dB	IL_Δ/l_f /(dB·mm^–1)	ΔS_±/lf /(dB·mm^–1)	Ref.
Ni(30)	HME	2.24	0.8	47	0.34	0.03	[29]
Ni(20)/Si(400)	HME	1.85	0.4	N/A	0.003	0.03	[31]
CoFeB(5)/Pt	HME, iDMI	6.77	0.75	71	22	28	[33]
FeGaB(20)/Al₂O₃(5)/FeGaB(20)	IDC	1.435	2.2	55	4	22	[37]
NiFe(20)/Au(5)/CoFeB(5)	IDC, HME	6.87	0.5	89	1.6	74	[34]
CoFeB(16)/Ru(0.55)/CoFeB(5)	IDC	5.08	0.15	81	0.9	250	[42]
FeCoSiB(10)/NiFeCu(10)	IDC	2.33	0.5	54	30	60	[43]
Ni(16)/Ti(8)/FeCoSiB(16)	IDC	2.33	0.5	51	4	80	[67]
CoFeB(16)/Ru(0.55)/CoFeB(14)	IDC	2.8—7	0.1	60	0.8	50	[68]

下载: 导出CSV

map

[1]	Takahashi S, Maekawa S 2008 J. Phys. Soc. Jpn. 77 031009 Google Scholar
[2]	Stancil D D, Prabhakar A 2009 Quantum theory of spin waves (New York: Springer Press
[3]	Uchida K I, Saitoh E 2016 Spin current (New York: John Wiley & Sons Press
[4]	Uchida K I, Adachi H, Kajiwara Y, Maekawa S, Saitoh E 2013 Solid State Phys. 64 1 Google Scholar
[5]	Adachi H, Uchida K I, Saitoh E, Maekawa S 2013 Rep. Prog. Phys. 76 036501 Google Scholar
[6]	Kruglyak V V, Demokritov S O, Grundler D 2010 J. Phys. D: Appl. Phys. 43 264001 Google Scholar
[7]	Chumak A V, Vasyuchka V I, Serga A A, Hillebrands B 2015 Nat. Phys. 11 453 Google Scholar
[8]	Reed K W, Owens J M, Carter R L 1985 Circuits Syst. Signal Press. 4 157 Google Scholar
[9]	Kim S K, Lee K S, Han D S 2009 Appl. Phys. Lett. 95 082507 Google Scholar
[10]	Wang Q, Zhong Z Y, Jin L C, Tang X L, Bai F M, Zhang H W, Beach G S D 2013 J. Magn. Magn. Mater. 340 23 Google Scholar
[11]	Chumak A V, Vasyuchka V I, Serga A A, Kostylev M P, Tiberkevich V S, Hillebrands B 2012 Phys. Rev. Lett. 108 257207 Google Scholar
[12]	Nakane R, Tanaka G, Hirose A 2018 IEEE Access 6 4462 Google Scholar
[13]	Sluka V, Schneider T, Gallardo R A, Kákay A, Weigand M, Warnatz T, Mattheis R, Roldán-Molina A, Landeros P, Tiberkevich V, Slavin A, Schütz G, Erbe A, Deac A, Lindner J, Raabe J, Fassbender J, Wintz S 2019 Nat. Nanotechnol. 14 328 Google Scholar
[14]	Jamali M, Kwon J, Seo S, Lee K J, Yang H 2013 Sci. Rep. 3 3160 Google Scholar
[15]	Chumak A, Serga A, Hillebrands B 2014 Nat. Commun. 5 4700 Google Scholar
[16]	Nikitin A A, Ustinov A B, Semenov A A 2015 Appl. Phys. Lett. 106 102405 Google Scholar
[17]	Vlaminck V, Bailleul M 2008 Science 322 410 Google Scholar
[18]	Auld B A 1973 Acoustic Fields and Waves in Solids (New York: John Wiley and Sons Press
[19]	Lewis M F 1985 Rayleigh-Wave Theory and Application (New York: Springer Press
[20]	Casals B, Statuto N, Foerster M, Hernández-Mínguez A, Cichelero R, Manshausen P, Mandziak A, Aballe L, Hernàndez J M, Macià F 2020 Phys. Rev. Lett. 124 137202 Google Scholar
[21]	Babu N K P, Trzaskowska A, Graczyk P, Centała G, Mieszczak S, Głowiński H, Zdunek M, Mielcarek S, W Kłos J 2021 Nano Lett. 21 946 Google Scholar
[22]	Küß M, Heigl M, Flacke L, Hefele A, Hörner A, Weiler M, Albrecht M, Wixforth A 2021 Phys. Rev. Appl. 15 034046 Google Scholar
[23]	Huang M X, Hu W B, Zhang H W, Bai F M 2023 J. Appl. Phys. 133 223902 Google Scholar
[24]	Zhang S, Levy P M, Fert A 2002 Phys. Rev. Lett. 88 236601 Google Scholar
[25]	Weiler M, Huebl H, Goerg F S, Czeschka F D, Gross R, Goennenwein S T B 2012 Phys. Rev. Lett. 108 176601 Google Scholar
[26]	Labanowski D, Jung A, Salahuddin S 2016 Appl. Phys. Lett. 108 022905 Google Scholar
[27]	Weiler M, Dreher L, Heeg C, Huebl H, Gross R, Brandt M S, Goennenwein S T B 2011 Phys. Rev. Lett. 106 117601 Google Scholar
[28]	Dreher L, Weiler M, Pernpeintner M, Huebl H, Gross R, Brandt M S, Goennenwein S T B 2012 Phys. Rev. B 86 134415 Google Scholar
[29]	Sasaki R, Nii Y, Iguchi Y, Onose Y 2017 Phys. Rev. B 95 020407 Google Scholar
[30]	Sasaki R, Nii Y, Onose Y 2021 Nat. Commun. 12 2599 Google Scholar
[31]	Tateno S, Nozaki Y 2020 Phys. Rev. Appl. 13 034074 Google Scholar
[32]	Hernández-Mínguez A, Macià F, Hernàndez J M, Herfort J, Santos P V 2020 Phys. Rev. Appl. 13 044018 Google Scholar
[33]	Küß M, Heigl M, Flacke L, Hörner A, Weiler M, Albrecht M, Wixforth A 2020 Phys. Rev. Lett. 125 217203 Google Scholar
[34]	Küß M, Heigl M, Flacke L, Hörner A, Weiler M, Wixforth A, Albrecht M 2021 Phys. Rev. Appl. 15 034060 Google Scholar
[35]	Verba R, Lisenkov I, Krivorotov I, Tiberkevich V, Slavin A 2018 Phys. Rev. Appl. 9 064014 Google Scholar
[36]	Verba R, Tiberkevich V, Slavin A 2019 Phys. Rev. Appl. 12 054061 Google Scholar
[37]	Shah P J, Bas D A, Lisenkov I, Matyushov A, Sun N X, Page M R 2020 Sci. Adv. 6 eabc5648 Google Scholar
[38]	Verba R, Bankowski E N, Meitzler T J, Tiberkevich V, Slavin A 2021 Adv. Electron. Mater. 7 2100263 Google Scholar
[39]	Bas D A, Verba R, Shah P J, Leontsev S, Matyushov A, Newburger M J, Sun N X, Tyberkevich V, Slavin A, Page M R 2022 Phys. Rev. Appl. 18 044003 Google Scholar
[40]	Küß M, Hassan M, Kunz Y, Hörner A, Weiler M, Albrecht M 2023 Phys. Rev. B 107 024424 Google Scholar
[41]	Küß M, Hassan M, Kunz Y, Hörner A, Weiler M, Albrecht M 2023 Phys. Rev. B 107 214412 Google Scholar
[42]	Küß M, Glamsch S, Kunz Y, Hörner A, Weiler M, Albrecht M 2023 ACS Appl. Electron. Mater. 5 5103 Google Scholar
[43]	Huang M X, Liu Y Y, Hu W B, Wu Y T, Wang W, He W, Zhang H W, Bai F M 2024 Phys. Rev. Appl. 21 014035 Google Scholar
[44]	Chudnovsky E M, Jaafar R 2016 Phys. Rev. Appl. 5 031002 Google Scholar
[45]	Kobayashi D, Yoshikawa T, Matsuo M, Iguchi R, Maekawa S, Saitoh E, Nozaki Y 2017 Phys. Rev. Lett. 119 077202 Google Scholar
[46]	Takahashi R, Matsuo M, Ono M, Harii K, Chudo H, Okayasu S, Ieda J, Takahashi S, Maekawa S, Saitoh E 2015 Nat. Phys. 12 52 Google Scholar
[47]	Matsuo M, Ieda J I, Harii K, Saitoh E, Maekawa S 2013 Phys. Rev. B 87 180402 Google Scholar
[48]	Matsuo M, Ohnuma Y, Maekawa S 2017 Phys. Rev. B 96 020401 Google Scholar
[49]	Tateno S, Okano G, Matsuo M, Nozaki Y 2020 Phys. Rev. B 102 104406 Google Scholar
[50]	Kurimune Y, Matsuo M, Maekawa S, Nozaki Y 2020 Phys. Rev. B 102 174413 Google Scholar
[51]	Huang M X, Hu W B, Zhang H W, Bai F M 2023 Phys. Rev. B 107 134401 Google Scholar
[52]	Kurimune Y, Matsuo M, Nozaki Y 2020 Phys. Rev. Lett. 124 217205 Google Scholar
[53]	Xu M, Yamamoto K, Puebla J, Baumgaertl K, Rana B, Miura K, Takahashi H, Grundler D, Maekawa S, Otani Y 2020 Sci. Adv. 6 eabb1724 Google Scholar
[54]	Landau L D, Lifshitz E M 1935 Phys. Z. Sowietunion 8 153
[55]	Gilbert T L 1955 Phys. Rev. 100 1243
[56]	Einstein A, de Haas W J 1915 Verhandlungen 17 152
[57]	Barnett S J 1915 Phys. Rev. 6 239 Google Scholar
[58]	Maekawa S, Tachiki M 1976 AIP Conf. Proc. 29 542 Google Scholar
[59]	Eshbach J R, Damon R W 1960 Phys. Rev. 118 1208 Google Scholar
[60]	Melkov G A, Vasyuchka V I, Lazovskiy V V, Tiberkevich V S, Slavin A N 2006 Appl. Phys. Lett. 89 252510 Google Scholar
[61]	Mruczkiewicz M, Krawczyk M, Gubbiotti G, Tacchi S, Filimonov Yu A, Kalyabin D V, Lisenkov I V, Nikitov S A 2013 New J. Phys. 15 113023 Google Scholar
[62]	Verba R, Tiberkevich V, Bankowski E, Meitzler T, Melkov G, Slavin A 2013 Appl. Phys. Lett. 103 082407 Google Scholar
[63]	Lisenkov I, Tyberkevych V, Slavin A, Bondarenko P, Ivanov B A, Bankowski E, Meitzler T, Nikitov S 2014 Phys. Rev. B 90 104417 Google Scholar
[64]	Dmytriiev O, Meitzler T, Bankowski E, Slavin A, Tiberkevich V 2010 J. Phys.: Condens. Matter 22 136001 Google Scholar
[65]	Gallardo R A, Alvarado-Seguel P, Schneider T, Gonzalez-Fuentes C, Roldán-Molina A, Lenz K, Lindner J, Landeros P 2019 New J. Phys. 21 033026 Google Scholar
[66]	Matsumoto H, Kawada T, Ishibashi M, Kawaguchi M, Hayashi M 2022 Appl. Phys. Express 15 063003 Google Scholar
[67]	Hu W B, Huang M X, Wu Y T, Jia Y N, Wang W, Bai F M 2024 Appl. Phys. Lett. 124 182405 Google Scholar
[68]	Küß M, Glamsch S, Hörner A, Albrecht M 2024 ACS Appl. Electron. Mater. 6 1790 Google Scholar
[69]	Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2015 Science 349 405 Google Scholar
[70]	Lachance-Quirion D, Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Nakamura Y 2017 Sci. Adv. 3 e1603150 Google Scholar
[71]	Satzinger K J, Zhong Y P, Chang H S, Peairs G A, Bienfait A, Chou M H, Cleland A Y, Conner C R, DumurÉ, Grebel J, Gutierrez I, November B H, Povey R G, Whiteley S J, Awschalom D D, Schuster D I, Cleland A N 2018 Nature 563 661 Google Scholar
[72]	Whiteley S J, Wolfowicz G, Anderson C P, Bourassa A, Ma H, Ye M, Koolstra G, Satzinger K J, Holt M V, Heremans F J, Cleland A N, Schuster D I, Galli G, Awschalom D D 2019 Nat. Phys. 15 490 Google Scholar
[73]	An K, Litvinenko A N, Kohno R, Fuad A A, Naletov V V, Vila L, Ebels U, de Loubens G, Hurdequint H, Beaulieu N, Ben Youssef J, Vukadinovic N, Bauer G E W, Slavin A N, Tiberkevich V S, Klein O 2020 Phys. Rev. B 101 060407(R Google Scholar
[74]	Chu Y, Kharel P, Yoon T, Frunzio L, Rakich P T, Schoelkopf R J 2018 Nature 563 666 Google Scholar
[75]	Bombeck M, Salasyuk A S, Glavin B A, Scherbakov A V, Brüggemann C, Yakovlev D R, Sapega V F, Liu X, Furdyna J K, Akimov A V, Bayer M 2012 Phys. Rev. B 85 195324 Google Scholar
[76]	Scherbakov A V, Salasyuk A S, Akimov A V, Liu X, Bombeck M, Brüggemann C, Yakovlev D R, Sapega V F, Furdyna J K, Bayer M 2010 Phys. Rev. Lett. 105 117204 Google Scholar
[77]	Polewczyk V, Dumesnil K, Lacour D, Moutaouekkil M, Mjahed H, Tiercelin N, Petit Watelot S, Mishra H, Dusch Y, Hage-Ali S, Elmazria O, Montaigne F, Talbi A, Bou Matar O, Hehn M 2017 Phys. Rev. Appl. 8 024001 Google Scholar
[78]	Liu X, Tong B, Ou-Yang J, Yang X, Chen S, Zhang Y, Zhu B 2018 Appl. Phys. Lett. 113 082402 Google Scholar
[79]	Kittmann A, Durdaut P, Zabel S, Reermann J, Schmalz J, Spetzler B, Meyners D, Sun N X, McCord J, Gerken M, Schmidt G, Höft M, Köchel R, Faupel F, Quandt E 2018 Sci. Rep. 8 278 Google Scholar
[80]	Hu W B, Huang M X, Xie H P, Zhang H W, Bai F M 2023 Phys. Rev. Appl. 19 014010 Google Scholar
[81]	Hu W B, Wang Y D, Huang M X, Zhang H W, Bai F M 2023 Appl. Phys. Lett. 123 012406 Google Scholar
[82]	Thevenard L, Camara I S, Majrab S, Bernard M, Rovillain P, Lemaître A, Gourdon C, Duquesne J Y 2016 Phys. Rev. B 93 134430 Google Scholar
[83]	Davis S, Baruth A, Adenwalla S 2010 Appl. Phys. Lett. 97 232507 Google Scholar
[84]	Al Misba W, Rajib M M, Bhattacharya D, Atulasimha J 2020 Phys. Rev. Appl. 14 014088 Google Scholar
[85]	Biswas A K, Bandyopadhyay S, Atulasimha J 2013 Appl. Phys. Lett. 103 232401 Google Scholar
[86]	Roe A, Bhattacharya D, Atulasimha J 2019 Appl. Phys. Lett. 115 112405 Google Scholar
[87]	Yang H F, Garcia-Sanchez F, Hu X K, Sievers S, Böhnert T, Costa J D, Tarequzzaman M, Ferreira R, Bieler M, Schumacher H W 2018 Appl. Phys. Lett. 113 072403 Google Scholar
[88]	Zhang D L, Zhu J, Qu T, Lattery D M, Victora R H, Wang X, Wang J P 2020 Sci. Adv. 6 eabb4607 Google Scholar
[89]	Iihama S, Xu Y, Deb M, Malinowski G, Hehn M, Gorchon J, Fullerton E E, Mangin S 2018 Adv. Mater. 30 1804004 Google Scholar
[90]	van Hees Y L W, van de Meugheuvel P, Koopmans B, Lavrijsen R 2020 Nat. Commun. 11 3835 Google Scholar
[91]	Edrington W, Singh U, Dominguez M A, Alexander J R, Nepal R, Adenwalla S 2018 Appl. Phys. Lett. 112 052402 Google Scholar
[92]	Dean J, Bryan M T, Cooper J D, Virbule A, Cunningham J E, Hayward T J 2015 Appl. Phys. Lett. 107 142405 Google Scholar
[93]	Wei Y, Li X, Gao R, Wu H, Wang X, Zeng Z, Wang J, Liu Q 2020 J. Magn. Magn. Mater. 502 166546 Google Scholar
[94]	Chen C, Fu S, Han L, Su R, Liu P, Chen R, Zhu W, Liao L, Pan F, Song C 2022 Adv. Electron. Mater. 8 2200593 Google Scholar
[95]	Yokouchi T, Sugimoto S, Rana B, Seki S, Ogawa N, Kasai S, Otani Y 2020 Nat. Nanotechnol. 15 361 Google Scholar
[96]	Yang W G, Schmidt H 2020 Appl. Phys. Lett. 116 212401 Google Scholar
[97]	Yang W G, Jaris M, Berk C, Schmidt H 2019 Phys. Rev. B 99 104434 Google Scholar
[98]	Matsuda O, Tsutsui K, Vaudel G, Pezeril T, Fujita K, Gusev V 2020 Phys. Rev. B 101 224307 Google Scholar
[99]	Chen R, Chen C, Han L, Liu P, Su R, Zhu W, Zhou Y, Pan F, Song C 2023 Nat. Commun. 14 4427 Google Scholar

[1]	陈崇, 马铭远, 潘峰, 宋成. 磁声耦合: 物理、材料与器件. , 2024, 73(5): 058502. doi: 10.7498/aps.73.20231908
[2]	刘想, 王希光, 李志雄, 郭光华. 铁磁畴壁中自旋极化电流诱导的左旋极化自旋波. , 2024, 73(14): 147501. doi: 10.7498/aps.73.20240651
[3]	谭自豪, 孙小伟, 宋婷, 温晓东, 刘禧萱, 刘子江. 球形复合柱表面波声子晶体的带隙特性仿真. , 2021, 70(14): 144301. doi: 10.7498/aps.70.20210165
[4]	闫健, 任志伟, 钟智勇. Y₃Fe₅O₁₂-CoFeB自旋波定向耦合器中的自旋波. , 2021, 70(18): 187501. doi: 10.7498/aps.70.20210507
[5]	吕刚, 张红, 侯志伟. 具有倾斜极化层的自旋阀结构中磁翻转以及磁振荡模式的微磁模拟. , 2018, 67(17): 177502. doi: 10.7498/aps.67.20180947
[6]	秦晨, 余辉, 叶乔波, 卫欢, 江晓清. 基于绝缘体上硅的一种改进的Mach-Zehnder声光调制器. , 2016, 65(1): 014304. doi: 10.7498/aps.65.014304
[7]	郝娟, 周广刚, 马跃, 黄文奇, 张鹏, 卢贵武. 高温条件下Ga3PO7晶体热学及声表面波性质的理论研究. , 2016, 65(11): 113101. doi: 10.7498/aps.65.113101
[8]	吕刚, 曹学成, 秦羽丰, 王林辉, 厉桂华, 高峰, 孙丰伟, 张红. 椭圆纳米盘中磁涡旋结构的方位角自旋波模式. , 2015, 64(21): 217501. doi: 10.7498/aps.64.217501
[9]	曾伟, 王海涛, 田贵云, 胡国星, 汪文. 研究激光激发的声表面波与材料近表面缺陷的振荡效应. , 2015, 64(13): 134302. doi: 10.7498/aps.64.134302
[10]	钱莉荣, 杨保和. ZnO薄膜/金刚石在不同激励条件下声表面波特性的计算与分析. , 2013, 62(11): 117701. doi: 10.7498/aps.62.117701
[11]	周振凯, 韦利明, 丰杰. ZnO/Diamond/Si结构中声表面波传播特性分析. , 2013, 62(10): 104601. doi: 10.7498/aps.62.104601
[12]	侯小娟, 云国宏, 白宇浩, 白那日苏, 周文平. 量子自旋波本征值及易轴型各向异性对其的影响. , 2011, 60(5): 056805. doi: 10.7498/aps.60.056805
[13]	李宏, 郭华忠, 路川, 李玲, 高洁. 声表面波单电子输运器件中量子线的电学特性研究. , 2008, 57(9): 5863-5868. doi: 10.7498/aps.57.5863
[14]	王敬时, 徐晓东, 刘晓峻, 许钢灿. 利用激光超声技术研究表面微裂纹缺陷材料的低通滤波效应. , 2008, 57(12): 7765-7769. doi: 10.7498/aps.57.7765
[15]	杨光, Santos Paulo V.. 磁控溅射制备ZnO薄膜及其声表面波特性. , 2007, 56(6): 3515-3520. doi: 10.7498/aps.56.3515
[16]	赵兴东, 谢征微, 张卫平. 玻色凝聚的原子自旋链中的非线性自旋波. , 2007, 56(11): 6358-6366. doi: 10.7498/aps.56.6358
[17]	肖夏, 尤学一, 姚素英. 表征超大规模集成电路互连纳米薄膜硬度特性的声表面波的频散特性. , 2007, 56(4): 2428-2433. doi: 10.7498/aps.56.2428
[18]	杨光, P. V. Santos. 声表面波对GaAs(110)量子阱发光特性的调制. , 2006, 55(8): 4327-4331. doi: 10.7498/aps.55.4327
[19]	王佐卿, 周素华, 汪承浩. 声表面波在声栅上的Bragg衍射. , 1983, 32(2): 156-167. doi: 10.7498/aps.32.156
[20]	朱砚磬, 王志强. 自旋波-声子耦合对反铁磁体红外吸收谱的影响. , 1966, 22(3): 360-370. doi: 10.7498/aps.22.360

计量

文章访问数: 1989
PDF下载量: 118
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板