幂律流体顶盖驱动流中的颗粒运动

杨晓峰; 刘姣; 单方; 柴振华; 施保昌

doi:10.7498/aps.73.20240164

摘要

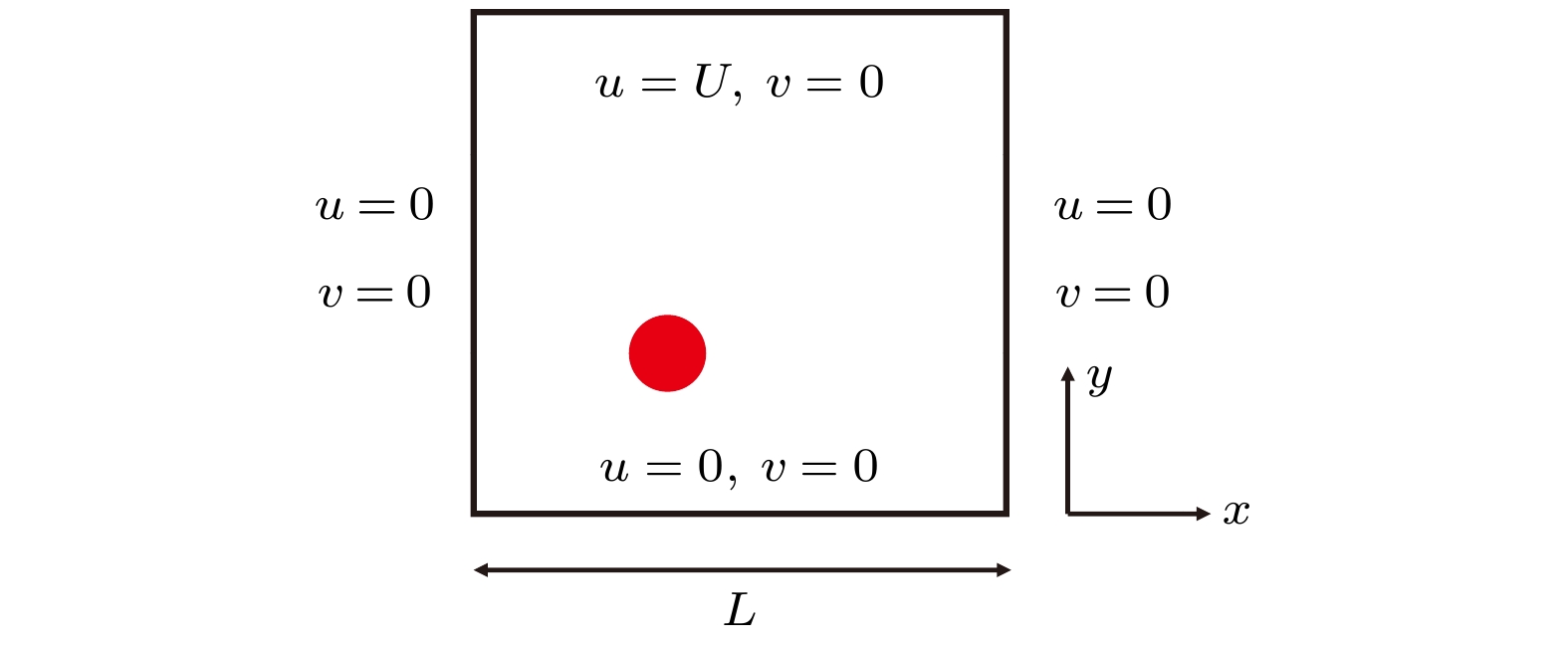
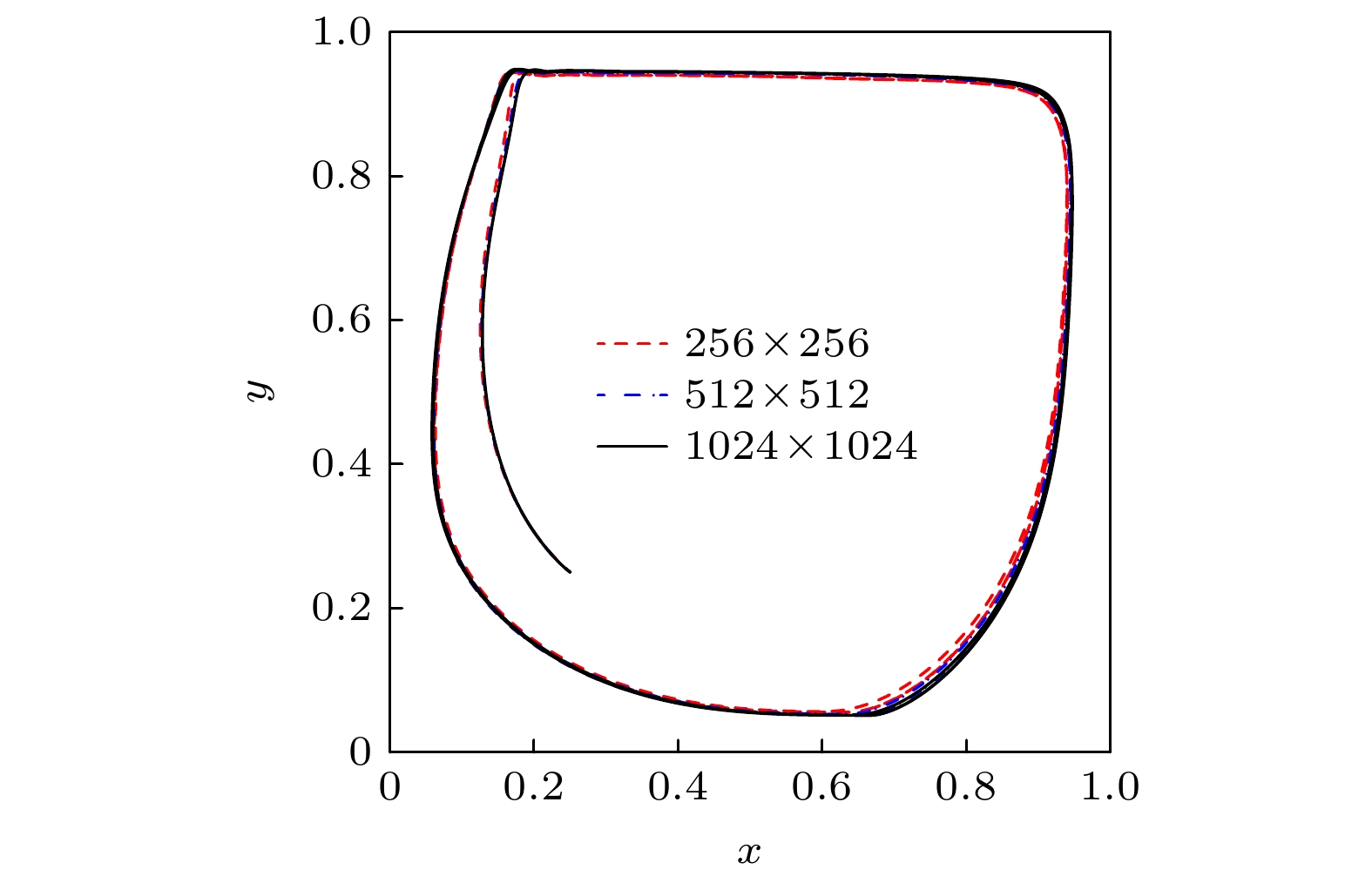
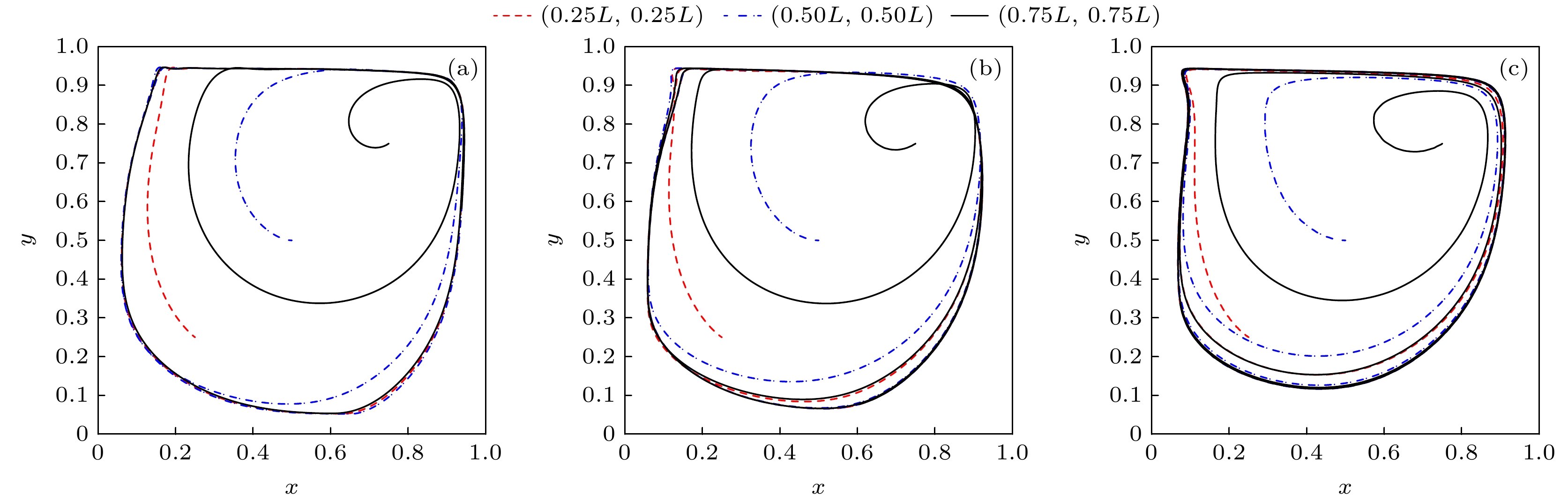
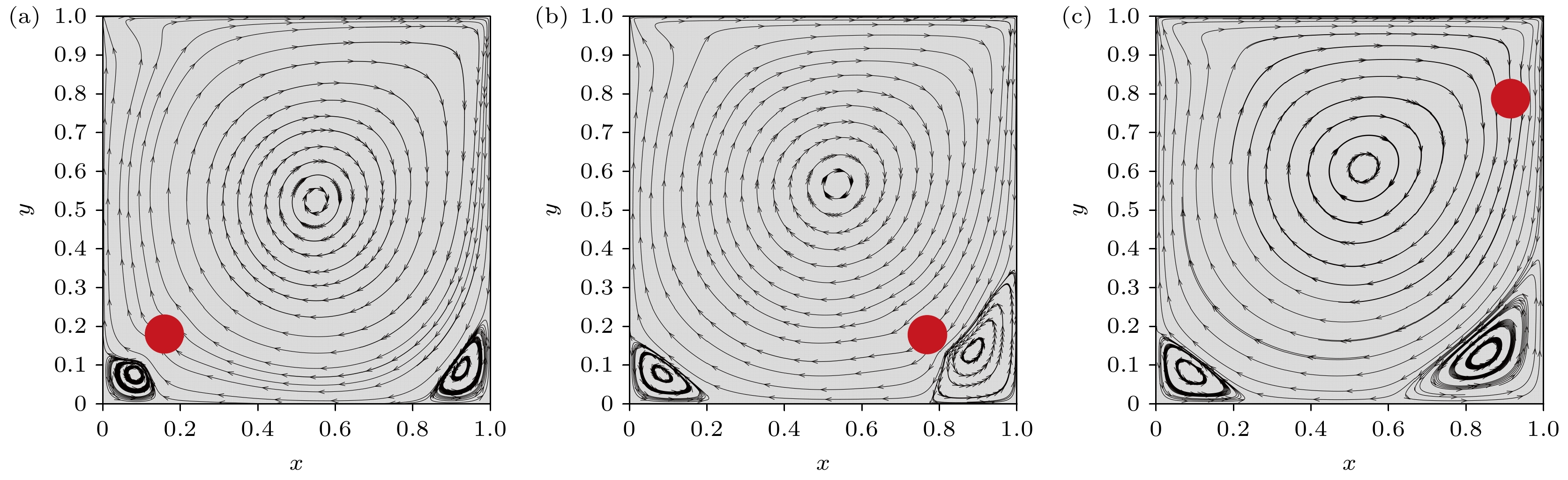
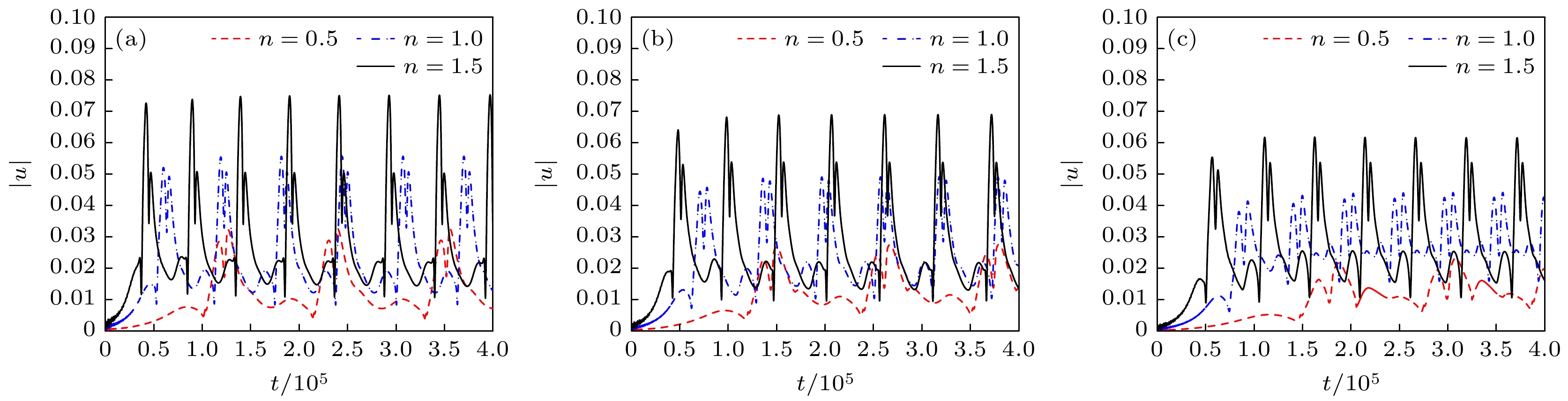
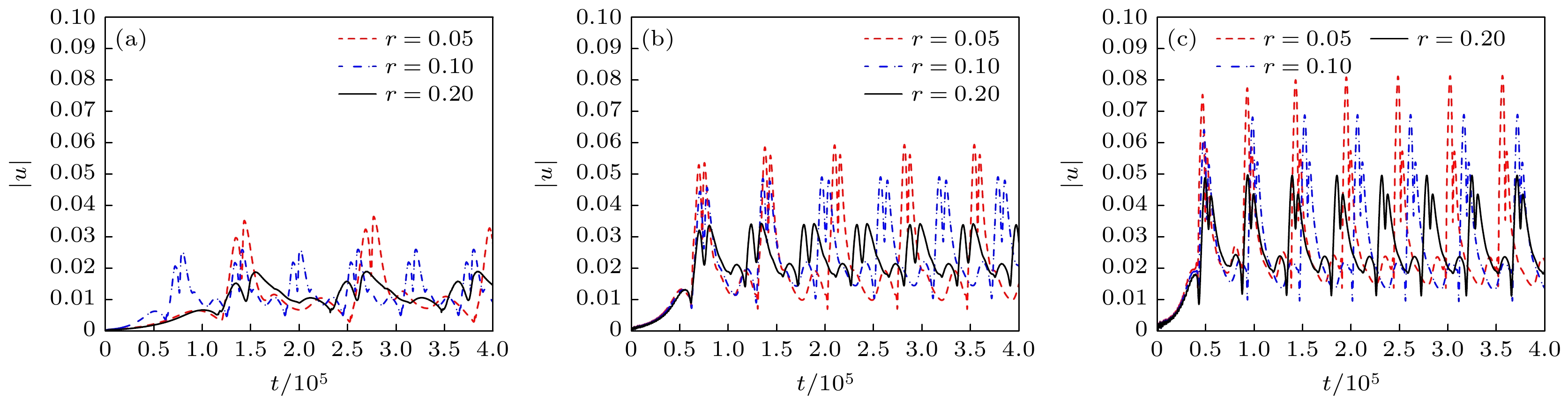
采用扩散界面格子Boltzmann模型研究了圆形颗粒在幂律流体方腔流中的运动, 重点分析了初始位置、幂律指数、颗粒大小对圆形颗粒在幂律流体顶盖驱动流中的运动的影响. 数值结果表明不同初始位置的圆形颗粒最终均能稳定在极限环上运动. 不同幂律指数的幂律流体对圆形颗粒运动的极限环有明显影响, 对于剪切增稠流体, 颗粒的运动速度明显增大, 极限环半径明显缩小; 对于剪切稀化流体, 左下角和右下角的次级涡收缩, 极限环向方腔的右下角移动. 圆形颗粒较小时, 颗粒的运动速度较快, 运动半径较大, 运动轨迹更接近流体的流线; 圆形颗粒较大时, 受到边界的限制, 极限环半径较小, 运动速度较慢. 此外, 还讨论了颗粒对顶盖驱动流主涡涡心位置的影响, 颗粒会将主涡涡心推向远离颗粒的方向.

关键词:

Abstract

In this paper, the motion of a circular particle in a lid-driven square cavity with the power-law fluid is studied by using the diffuse interface lattice Boltzmann method, and the study mainly considers the effects of the particle's initial position, the power-law index, the Reynolds number, and the particle size. The numerical results show that the circular particle is first in a centrifugal motion under the effect of inertia, and it finally moves steadily on the limit cycle. Furthermore, it is also found that the initial position of the particle has no influence on the limit cycle. For a shear-thinning fluid flow, the limit cycle moves towards the bottom right corner of the square cavity. Moreover, the particle velocity is small, and the period of the particle motion is long. On the other hand, in the case of shear-thickening fluid flow, the limit cycle moves towards the top left corner of the cavity. In addition, the particle velocity is large, and the period of the particle motion is short.With the increase of Reynolds number, the limit cycle moves towards the bottom right corner of the square cavity, which is caused by a strong fluid flow field. Meanwhile, the particle velocity becomes larger, and the period of the particle motion is shorter. With the increase of particle size, the effect of confinement of the cavity boundary becomes significant, and the circular particle is pushed towards the center of the cavity. In this case, the limit cycle shrinks towards the center of the cavity. The circular particle squeezes the secondary vortices, especially when the circular particle is located in the bottom left, bottom right and top left corners. Additionally, the appearance of the circular particle has a significant influence on the position of the primary vortex, which changes periodically near the position of the primary vortex without the particle. It is also observed that the influence of the circular particle becomes more significant as its size increases and the power-law index decreases.

Keywords:

作者及机构信息

1.
华中科技大学数学与统计学院, 武汉　430074

2.
华中科技大学, 工程建模与科学计算湖北重点实验室, 武汉　430074

3.
华中科技大学数学与应用学科交叉创新研究院, 武汉　430074

4.
南昌大学数学与计算机学院, 南昌　330031

通信作者: 柴振华, hustczh@hust.edu.cn

基金项目: 国家自然科学基金(批准号: 12072127, 123B2018)、华中科技大学交叉研究支持计划(批准号: 2023JCJY002, 2024JCYJ001)和中央高校基本科研业务费(批准号: YCJJ20241101, 2023JY-CXJJ046)资助的课题.

Authors and contacts

1.
School of Mathematics and Statistics, Huazhong University of Science and Technology, Wuhan 430074, China

2.
Hubei Key Laboratory of Engineering Modeling and Scientific Computing, Huazhong University of Science and Technology, Wuhan 430074, China

3.
Institute of Interdisciplinary Research for Mathematics and Applied Science, Huazhong University of Science and Technology, Wuhan 430074, China

4.
School of Mathematics and Computer Sciences, Nanchang University, Nanchang 330031, China

Corresponding author: Chai Zhen-Hua, hustczh@hust.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12072127, 123B2018), the Interdisciplinary Research Program of Huazhong University of Science and Technology, China (Grant Nos. 2023JCJY002, 2024JCYJ001), and the Fundamental Research Fund for the Central Universities, China (Grant Nos. YCJJ20241101, 2023JY-CXJJ046).

文章全文 : translate this paragraph

参考文献

[1]	Chhabra R P 2006 Bubbles, Drops, and Particles in Non-Newtonian Fluids (Boca Raton: CRC Press) pp40-74
[2]	朱克勤 2006 力学与实践 28 1 Google Scholar Zhu K Q 2006 Mech. Eng. 28 1 Google Scholar
[3]	Saramito P, Roquet N 2001 Comput. Methods Appl. Mech. Eng. 190 5391 Google Scholar
[4]	Neofytou P 2005 Adv. Eng. Software 36 664 Google Scholar
[5]	Rafiee A 2008 Anziam J. 49 411 Google Scholar
[6]	Bell B C, Surana K S 1994 Int. J. Numer. Methods Fluids 18 127 Google Scholar
[7]	Papanastasiou T C, Boudouvis A G 1997 Comput. Geotech. 64 677
[8]	Papanastasiou T C 1987 J. Rheol. 31 385 Google Scholar
[9]	Roquet N, Saramito P 2008 J. Non-Newtonian Fluid Mech. 155 101 Google Scholar
[10]	Tazangi H R, Goharrizi A S, Javaran E J 2021 Korea-Aust. Rheol. J. 33 293 Google Scholar
[11]	Schreiber R, Keller H B 1983 J. Comput. Phys. 49 310 Google Scholar
[12]	Botella O, Peyret R 1998 Comput. Fluids 27 421 Google Scholar
[13]	Erturk E, Corke T C 2005 Int. J. Numer. Methods Fluids 48 747 Google Scholar
[14]	Mendu S S, Das P K 2012 J. Non-Newtonian Fluid Mech. 175–176 10
[15]	Li Q X, Hong N, Shi B C, Chai Z H 2014 Commun. Comput. Phys. 15 265 Google Scholar
[16]	Aguirre A, Castillo E, Cruchaga M, Codina R, Baiges J 2018 J. Non-Newton. Fluid Mech. 257 22 Google Scholar
[17]	Alleborn N, Raszillier H, Durst F 1999 Int. J. Heat Mass Trans. 42 833 Google Scholar
[18]	Stephen T, Nathan E 2007 Geology 35 1027
[19]	Zhong H C, Zhou J, Du Z X, Xie L 2018 J. Aerosol Sci. 121 31 Google Scholar
[20]	Madankan R, Pouget S, Singla P, Bursik M, Dehn J, Jones M, Patra A, Pavolonis M, Pitman E B, Singh T, Webley P 2014 J. Comput. Phys. 271 39 Google Scholar
[21]	Yue G X, Cai R X, Lu J F, Zhang H 2017 Powder Technol. 316 18 Google Scholar
[22]	He W C, Lv X W, Pan F F, Li X Q, Yan Z M 2019 Powder Technol. 356 1087 Google Scholar
[23]	Sidik N A C, Attarzadeh S M R 2011 Int. J. Mech. 5 123
[24]	Romanò F, Kuhlmann H C 2017 Theor. Comput. Fluid Dyn. 31 427 Google Scholar
[25]	Hu J J, Sun D K, Mao S H, Wu H M, Yu S Y, Xu M S 2022 J. Comput. Theor. Transp. 51 222 Google Scholar
[26]	Hu J J 2021 Int. J. Mod. Phys. C 32 1
[27]	Hu J J 2020 Phys. Fluids 32 222
[28]	Safdari A, Kim K C 2014 Comput. Math. Appl. 68 606 Google Scholar
[29]	Francescò R, Hendrik H C 2017 Theor. Comput. Fluid Dyn. 31 1
[30]	Chen S, Doolen G D 1998 Rev. Fluid Mech. 30 329 Google Scholar
[31]	Succi S 2001 The Lattice Boltzmann Equation for Fluid Dynamics and Beyond (Oxford: Oxford University Press) pp3-38
[32]	Guo Z L, Shu C 2013 Lattice Boltzmann Method and Its Applications in Engineering (Singapore: World Scientific Publishing Co.) pp10-21
[33]	Krüger T, Kusumaatmaja H, Silva G, Shardt O, Kuzmin A, Viggen E M 2017 The Lattice Boltzmann Method: Principles and Practice (Switzerland: Springer International Publishing) pp61-65
[34]	Feng J, Hu H H, Joseph D D 1994 J. Fluid Mech. 277 271 Google Scholar
[35]	Inamuro T, Maeba K, Ogino F 2000 Int. J. Multiphase. Flow 26 1981 Google Scholar
[36]	Shao X, Yu Z, Sun B 2008 Phys. Fluids 20 103307 Google Scholar
[37]	Noble D R, Torczynski J R 1998 Int. J. Modern Phys. C 9 1189 Google Scholar
[38]	Liu J, Huang C S, Chai Z H, Shi B C 2022 Comput. Fluids 233 105240 Google Scholar
[39]	Liu J, Chai Z H, Shi B C 2022 Phys. Rev. E 106 015306 Google Scholar
[40]	Boyd J, Buick J, Green S 2006 J. Phys. A 39 14241 Google Scholar
[41]	Tang G H, Li X F, He Y L, Tao W Q 2009 J. Non-Newton. Fluid Mech. 157 133 Google Scholar
[42]	Chai Z H, Shi B C, Guo Z L, Rong F M 2011 J. Non-Newton. Fluid Mech. 166 332 Google Scholar
[43]	Guo Z L, Zheng C G, Shi B C 2002 Chin. Phys. 11 366 Google Scholar

施引文献

图 1 不同幂律指数下的中心线速度分布($ Re = 100 $)　(a)垂直速度; (b) 水平速度

Fig. 1. The velocity profiles along the centerlines at different power-law indices ($ Re = 100 $): (a) The vertical velocity profile; (b) the horizontal velocity profile.

下载: 全尺寸图片幻灯片

图 2 圆形颗粒在顶盖驱动流中运动的示意图

Fig. 2. The schematic of a circular particle moving in a lid-driven square cavity.

下载: 全尺寸图片幻灯片

图 3 不同雷诺数下圆形颗粒的极限环

Fig. 3. The limit cycles of a circular particle at different Reynolds numbers.

下载: 全尺寸图片幻灯片

图 4 $ Re = 1000 $时中心线速度分布对比　(a)垂直速度; (b)水平速度

Fig. 4. The comparisons of the velocity profiles along the centerlines at $ Re = 1000 $: (a) The vertical velocity profile; (b) the horizontal velocity profile.

下载: 全尺寸图片幻灯片

图 5 圆形颗粒在不同网格尺寸顶盖驱动流中的运动轨迹($ N_{x}\times N_{y} = 256\times 256 $, $ 512\times 512 $, $ 1024\times 1024 $)

Fig. 5. The trajectories of a circular particle moving in a lid-driven cavity with three different grid sizes ($ N_{x}\times $$ N_{y} = 256\times 256 $, $ 512\times 512 $, $ 1024\times 1024 $).

下载: 全尺寸图片幻灯片

图 6 圆形颗粒在幂律流体顶盖驱动流中的运动轨迹　(a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $

Fig. 6. The trajectories of the circular particle moving in the lid-driven cavity flows under different power-law indices and different initial positions: (a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $n = 1.5 $.

下载: 全尺寸图片幻灯片

图 7 圆形颗粒在幂律流体顶盖驱动流中运动的极限环　(a) $ Re = 500 $; (b) $ Re = 1000 $; (c) $ Re = 2000 $

Fig. 7. The limit cycles of the circular particle moving in the power-law lid-driven cavity flows with different power-law indices and Reynolds numbers: (a) $ Re = 500 $; (b) $ Re = 1000 $; (c) $ Re = 2000 $.

下载: 全尺寸图片幻灯片

图 8 幂律流体顶盖驱动流的流线及圆形颗粒的位置　(a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $

Fig. 8. The streamlines of power-law fluid flows and the positions of the circular particle under different power-law indices: (a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $.

下载: 全尺寸图片幻灯片

图 9 不同幂律指数($ n = 0.5 $, $ 1.0 $和$ 1.5 $)下圆形颗粒的速度　(a) $ Re = 500 $; (b) $Re = 1000 $; (c) $Re = 2000 $

Fig. 9. The evolutions of particle velocity under different power-law indices: (a) $ Re = 500 $; (b) $ Re = 1000 $; (c) $Re = 2000 $.

下载: 全尺寸图片幻灯片

图 10 不同颗粒尺寸和幂律指数下颗粒运动的极限环　(a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $

Fig. 10. The limit cycles of the moving particle in the power-law lid-driven cavity flows with different particle sizes and power-law indices: (a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $.

下载: 全尺寸图片幻灯片

图 11 不同颗粒尺寸和幂律指数下颗粒的运动速度　(a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $

Fig. 11. The evolutions of particle velocity under different particle sizes and power-law indices: (a) $ n = 0.5 $; (b) $ n = 1.0 $; (c) $ n = 1.5 $.

下载: 全尺寸图片幻灯片

图 12 不同幂律指数下幂律流体顶盖驱动流(Re = 1000, r = 0.2)的圆形颗粒位置和流线　(a) n = 0.5; (b) n = 1.0; (c) n = 1.5

Fig. 12. The positions of circular particle and the streamlines of the power-law lid-driven cavity flows (Re = 1000, r = 0.2) with different power-law indices: (a) n = 0.5; (b) n = 1.0; (c) n = 1.5.

下载: 全尺寸图片幻灯片

图 13 主涡涡心位置的水平、竖直坐标坐标随时间变化图　(a) $ x $轴坐标, $ r = 0.1 $; (b) $ y $轴坐标, $ r = 0.1 $; (c) $ x $轴坐标, $ r = 0.2 $; (d) $ y $轴坐标, $ r = 0.2 $

Fig. 13. The evolutions of the x-axis and y-axis coordinates of position of primary vortex: (a) x-axis, $ r = 0.1 $; (b) y-axis, $ r = 0.1 $; (c) x-axis, $ r = 0.2 $; (d) y-axis, $ r = 0.2 $.

下载: 全尺寸图片幻灯片

map

[1]	Chhabra R P 2006 Bubbles, Drops, and Particles in Non-Newtonian Fluids (Boca Raton: CRC Press) pp40-74
[2]	朱克勤 2006 力学与实践 28 1 Google Scholar Zhu K Q 2006 Mech. Eng. 28 1 Google Scholar
[3]	Saramito P, Roquet N 2001 Comput. Methods Appl. Mech. Eng. 190 5391 Google Scholar
[4]	Neofytou P 2005 Adv. Eng. Software 36 664 Google Scholar
[5]	Rafiee A 2008 Anziam J. 49 411 Google Scholar
[6]	Bell B C, Surana K S 1994 Int. J. Numer. Methods Fluids 18 127 Google Scholar
[7]	Papanastasiou T C, Boudouvis A G 1997 Comput. Geotech. 64 677
[8]	Papanastasiou T C 1987 J. Rheol. 31 385 Google Scholar
[9]	Roquet N, Saramito P 2008 J. Non-Newtonian Fluid Mech. 155 101 Google Scholar
[10]	Tazangi H R, Goharrizi A S, Javaran E J 2021 Korea-Aust. Rheol. J. 33 293 Google Scholar
[11]	Schreiber R, Keller H B 1983 J. Comput. Phys. 49 310 Google Scholar
[12]	Botella O, Peyret R 1998 Comput. Fluids 27 421 Google Scholar
[13]	Erturk E, Corke T C 2005 Int. J. Numer. Methods Fluids 48 747 Google Scholar
[14]	Mendu S S, Das P K 2012 J. Non-Newtonian Fluid Mech. 175–176 10
[15]	Li Q X, Hong N, Shi B C, Chai Z H 2014 Commun. Comput. Phys. 15 265 Google Scholar
[16]	Aguirre A, Castillo E, Cruchaga M, Codina R, Baiges J 2018 J. Non-Newton. Fluid Mech. 257 22 Google Scholar
[17]	Alleborn N, Raszillier H, Durst F 1999 Int. J. Heat Mass Trans. 42 833 Google Scholar
[18]	Stephen T, Nathan E 2007 Geology 35 1027
[19]	Zhong H C, Zhou J, Du Z X, Xie L 2018 J. Aerosol Sci. 121 31 Google Scholar
[20]	Madankan R, Pouget S, Singla P, Bursik M, Dehn J, Jones M, Patra A, Pavolonis M, Pitman E B, Singh T, Webley P 2014 J. Comput. Phys. 271 39 Google Scholar
[21]	Yue G X, Cai R X, Lu J F, Zhang H 2017 Powder Technol. 316 18 Google Scholar
[22]	He W C, Lv X W, Pan F F, Li X Q, Yan Z M 2019 Powder Technol. 356 1087 Google Scholar
[23]	Sidik N A C, Attarzadeh S M R 2011 Int. J. Mech. 5 123
[24]	Romanò F, Kuhlmann H C 2017 Theor. Comput. Fluid Dyn. 31 427 Google Scholar
[25]	Hu J J, Sun D K, Mao S H, Wu H M, Yu S Y, Xu M S 2022 J. Comput. Theor. Transp. 51 222 Google Scholar
[26]	Hu J J 2021 Int. J. Mod. Phys. C 32 1
[27]	Hu J J 2020 Phys. Fluids 32 222
[28]	Safdari A, Kim K C 2014 Comput. Math. Appl. 68 606 Google Scholar
[29]	Francescò R, Hendrik H C 2017 Theor. Comput. Fluid Dyn. 31 1
[30]	Chen S, Doolen G D 1998 Rev. Fluid Mech. 30 329 Google Scholar
[31]	Succi S 2001 The Lattice Boltzmann Equation for Fluid Dynamics and Beyond (Oxford: Oxford University Press) pp3-38
[32]	Guo Z L, Shu C 2013 Lattice Boltzmann Method and Its Applications in Engineering (Singapore: World Scientific Publishing Co.) pp10-21
[33]	Krüger T, Kusumaatmaja H, Silva G, Shardt O, Kuzmin A, Viggen E M 2017 The Lattice Boltzmann Method: Principles and Practice (Switzerland: Springer International Publishing) pp61-65
[34]	Feng J, Hu H H, Joseph D D 1994 J. Fluid Mech. 277 271 Google Scholar
[35]	Inamuro T, Maeba K, Ogino F 2000 Int. J. Multiphase. Flow 26 1981 Google Scholar
[36]	Shao X, Yu Z, Sun B 2008 Phys. Fluids 20 103307 Google Scholar
[37]	Noble D R, Torczynski J R 1998 Int. J. Modern Phys. C 9 1189 Google Scholar
[38]	Liu J, Huang C S, Chai Z H, Shi B C 2022 Comput. Fluids 233 105240 Google Scholar
[39]	Liu J, Chai Z H, Shi B C 2022 Phys. Rev. E 106 015306 Google Scholar
[40]	Boyd J, Buick J, Green S 2006 J. Phys. A 39 14241 Google Scholar
[41]	Tang G H, Li X F, He Y L, Tao W Q 2009 J. Non-Newton. Fluid Mech. 157 133 Google Scholar
[42]	Chai Z H, Shi B C, Guo Z L, Rong F M 2011 J. Non-Newton. Fluid Mech. 166 332 Google Scholar
[43]	Guo Z L, Zheng C G, Shi B C 2002 Chin. Phys. 11 366 Google Scholar

[1]	赖瑶瑶, 陈鑫梦, 柴振华, 施保昌. 基于格子Boltzmann方法的钉扎螺旋波反馈控制. , 2024, 73(4): 040502. doi: 10.7498/aps.73.20231549
[2]	许鑫萌, 娄钦. 剪切增稠幂律流体中单气泡上升动力学行为的格子Boltzmann方法研究. , 2024, 73(13): 134701. doi: 10.7498/aps.73.20240394
[3]	刘程, 梁宏. 三相流体的轴对称格子 Boltzmann 模型及其在 Rayleigh-Plateau 不稳定性的应用. , 2023, 72(4): 044701. doi: 10.7498/aps.72.20221967
[4]	陈百慧, 施保昌, 汪垒, 柴振华. 基于GPU的二维梯形空腔流的格子Boltzmann模拟与分析. , 2023, 72(15): 154701. doi: 10.7498/aps.72.20230430
[5]	马聪, 刘斌, 梁宏. 耦合界面张力的三维流体界面不稳定性的格子Boltzmann模拟. , 2022, 71(4): 044701. doi: 10.7498/aps.71.20212061
[6]	张恒, 任峰, 胡海豹. 基于格子Boltzmann方法的幂律流体二维顶盖驱动流转捩研究. , 2021, 70(18): 184703. doi: 10.7498/aps.70.20210451
[7]	胡嘉懿, 张文欢, 柴振华, 施保昌, 汪一航. 三维不可压缩流的12速多松弛格子Boltzmann模型. , 2019, 68(23): 234701. doi: 10.7498/aps.68.20190984
[8]	李洋, 苏婷, 梁宏, 徐江荣. 耦合界面力的两相流相场格子Boltzmann模型. , 2018, 67(22): 224701. doi: 10.7498/aps.67.20181230
[9]	周光雨, 陈力, 张鸿雁, 崔海航. 基于格子Boltzmann方法的自驱动Janus颗粒扩散泳力. , 2017, 66(8): 084703. doi: 10.7498/aps.66.084703
[10]	段娟, 陈耀钦, 朱庆勇. 微扩张管道内幂律流体非定常电渗流动. , 2016, 65(3): 034702. doi: 10.7498/aps.65.034702
[11]	陶实, 王亮, 郭照立. 微尺度振荡Couette流的格子Boltzmann模拟. , 2014, 63(21): 214703. doi: 10.7498/aps.63.214703
[12]	史冬岩, 王志凯, 张阿漫. 任意复杂流-固边界的格子Boltzmann处理方法. , 2014, 63(7): 074703. doi: 10.7498/aps.63.074703
[13]	曾建邦, 李隆键, 蒋方明. 气泡成核过程的格子Boltzmann方法模拟. , 2013, 62(17): 176401. doi: 10.7498/aps.62.176401
[14]	毛威, 郭照立, 王亮. 热对流条件下颗粒沉降的格子Boltzmann方法模拟. , 2013, 62(8): 084703. doi: 10.7498/aps.62.084703
[15]	郭亚丽, 徐鹤函, 沈胜强, 魏兰. 利用格子Boltzmann方法模拟矩形腔内纳米流体Raleigh-Benard对流. , 2013, 62(14): 144704. doi: 10.7498/aps.62.144704
[16]	苏进, 欧阳洁, 王晓东. 耦合不可压流场输运方程的格子Boltzmann方法研究. , 2012, 61(10): 104702. doi: 10.7498/aps.61.104702
[17]	曾建邦, 李隆键, 廖全, 陈清华, 崔文智, 潘良明. 格子Boltzmann方法在相变过程中的应用. , 2010, 59(1): 178-185. doi: 10.7498/aps.59.178
[18]	石自媛, 胡国辉, 周哲玮. 润湿性梯度驱动液滴运动的格子Boltzmann模拟. , 2010, 59(4): 2595-2600. doi: 10.7498/aps.59.2595
[19]	张超英, 李华兵, 谭惠丽, 刘慕仁, 孔令江. 椭圆柱体在牛顿流体中运动的格子Boltzmann方法模拟. , 2005, 54(5): 1982-1987. doi: 10.7498/aps.54.1982
[20]	吕晓阳, 李华兵. 用格子Boltzmann方法模拟高雷诺数下的热空腔黏性流. , 2001, 50(3): 422-427. doi: 10.7498/aps.50.422

计量

文章访问数: 624
PDF下载量: 30
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

幂律流体顶盖驱动流中的颗粒运动