基于最优控制理论的国产光抽运小铯钟频率控制算法

宋会杰; 董绍武; 王翔; 姜萌; 章宇; 郭栋; 张继海

doi:10.7498/aps.73.20231866

摘要

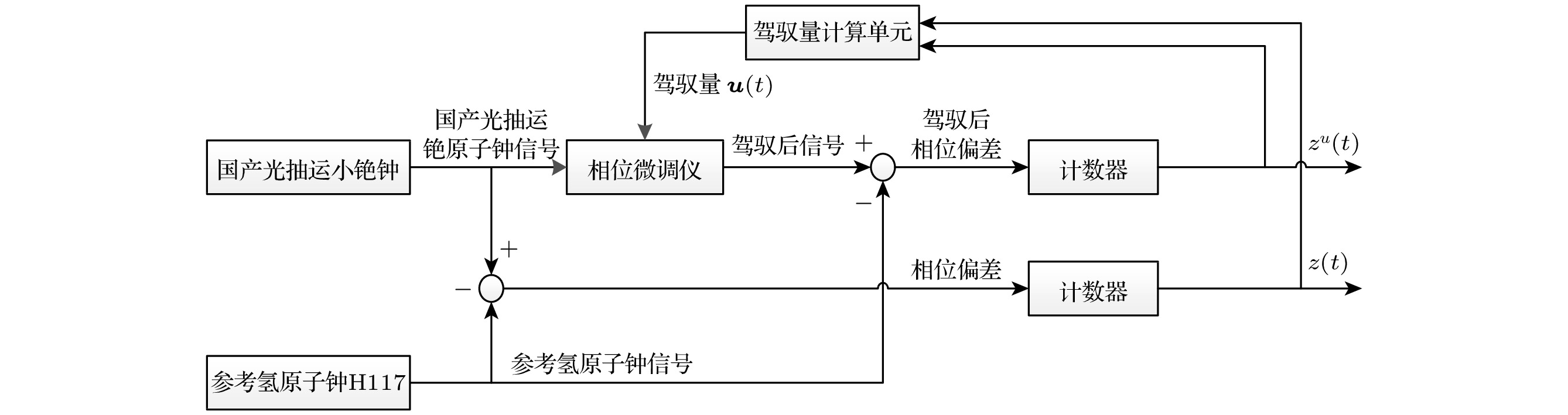
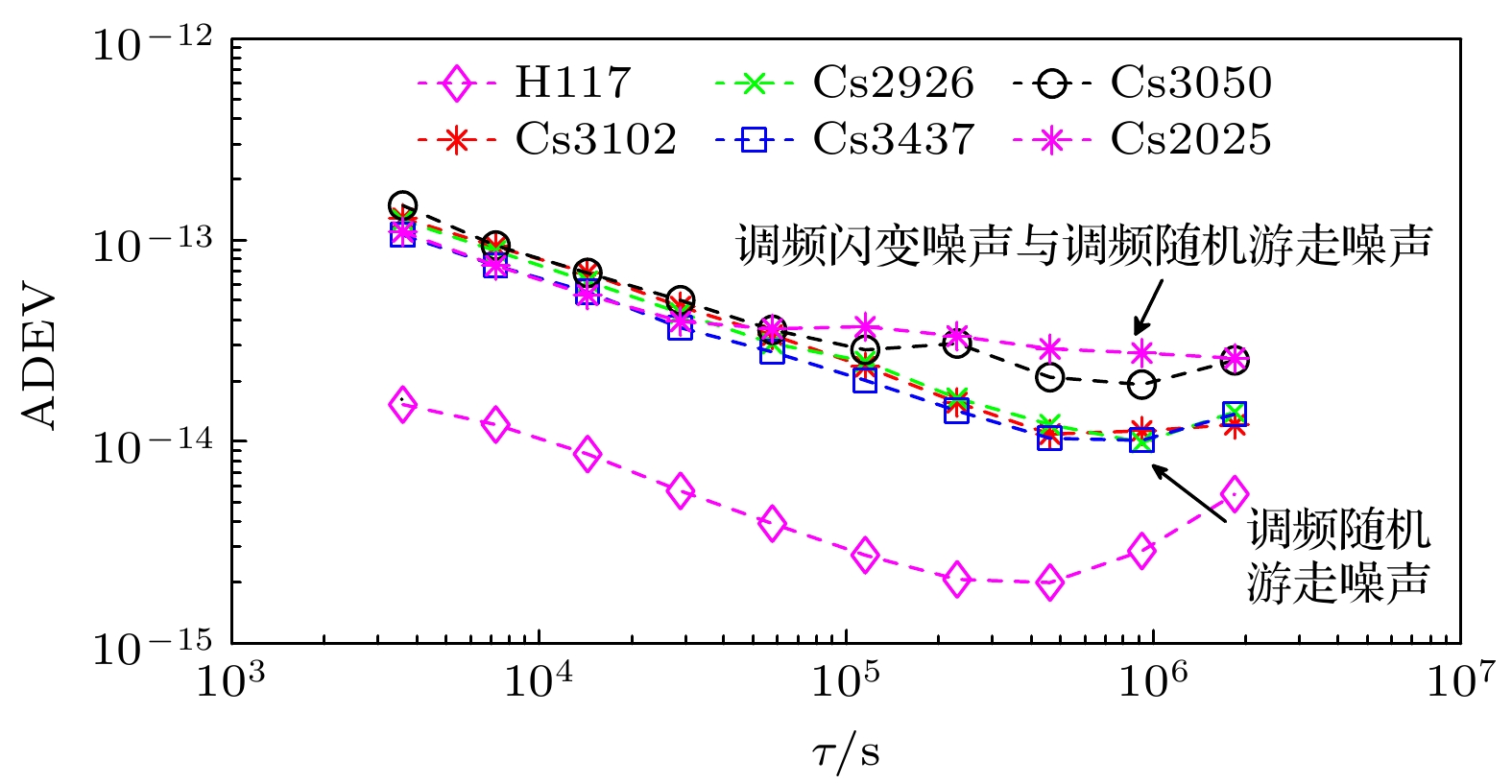
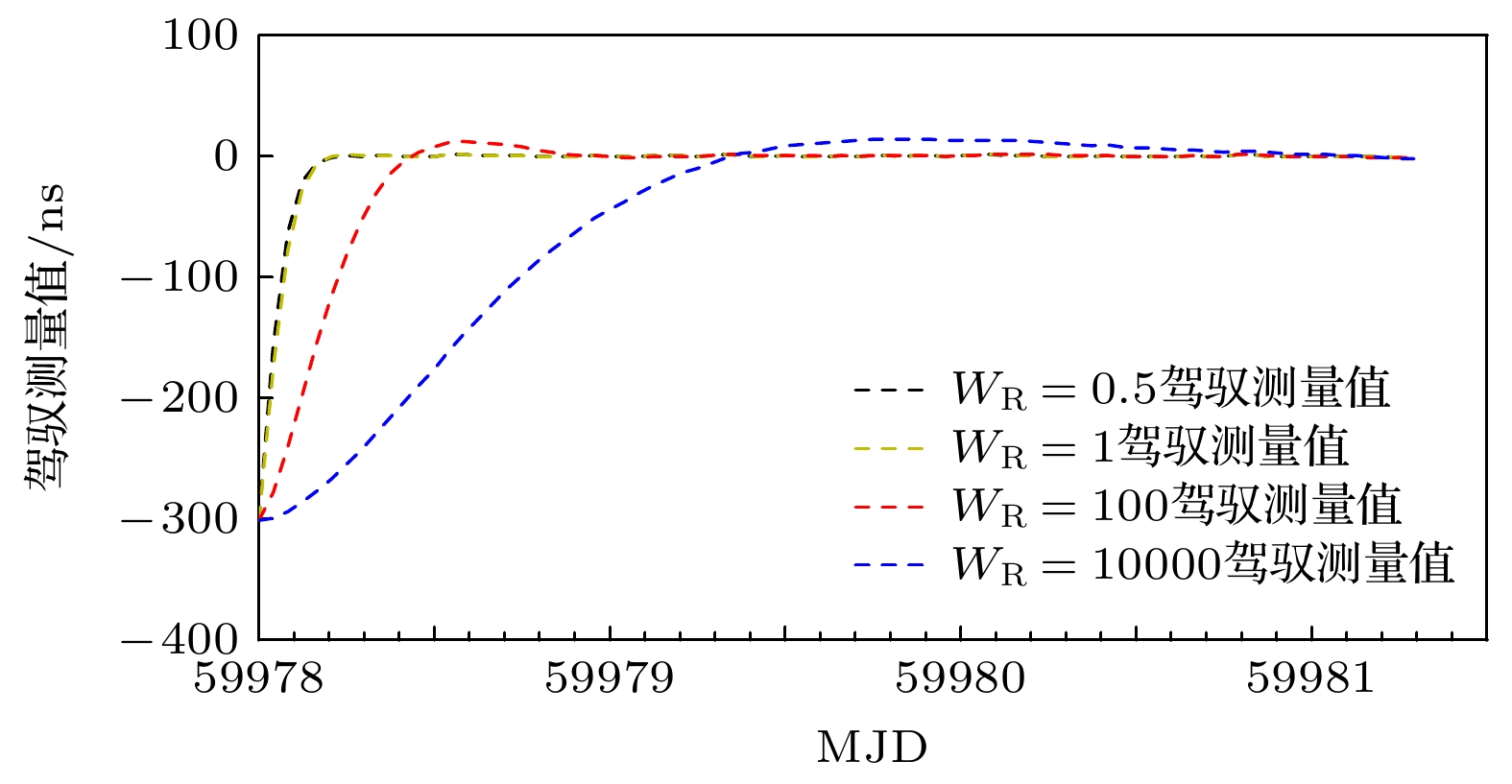
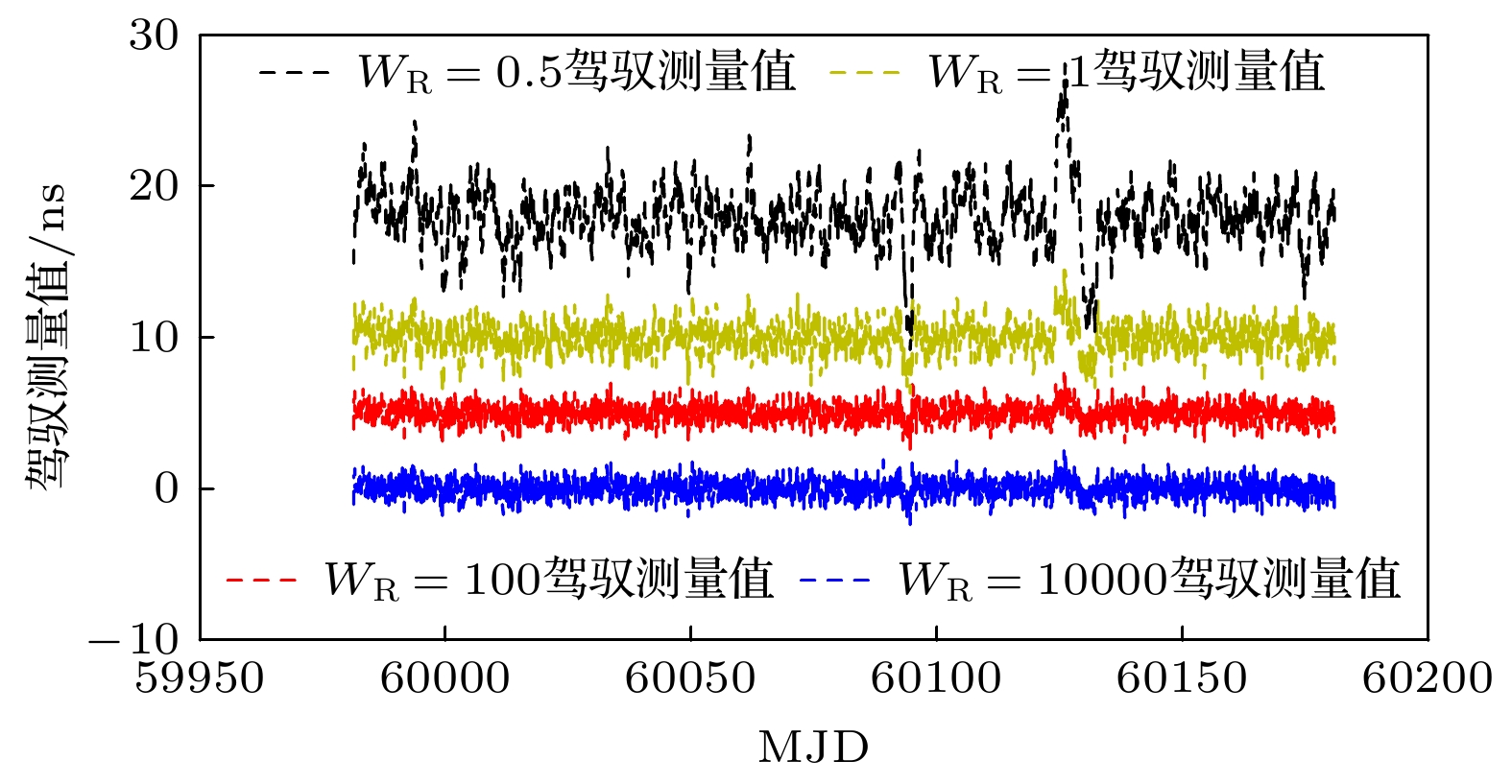
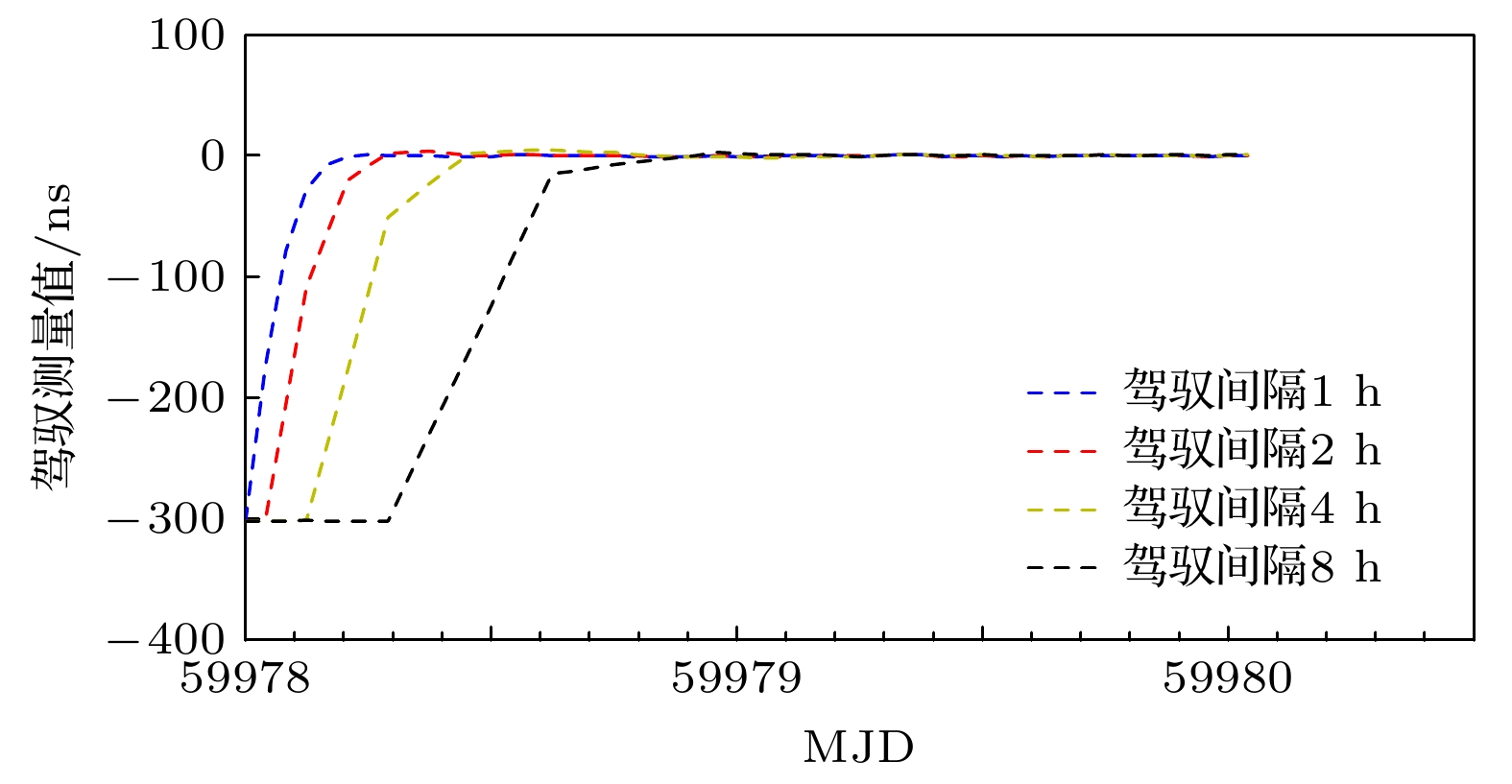
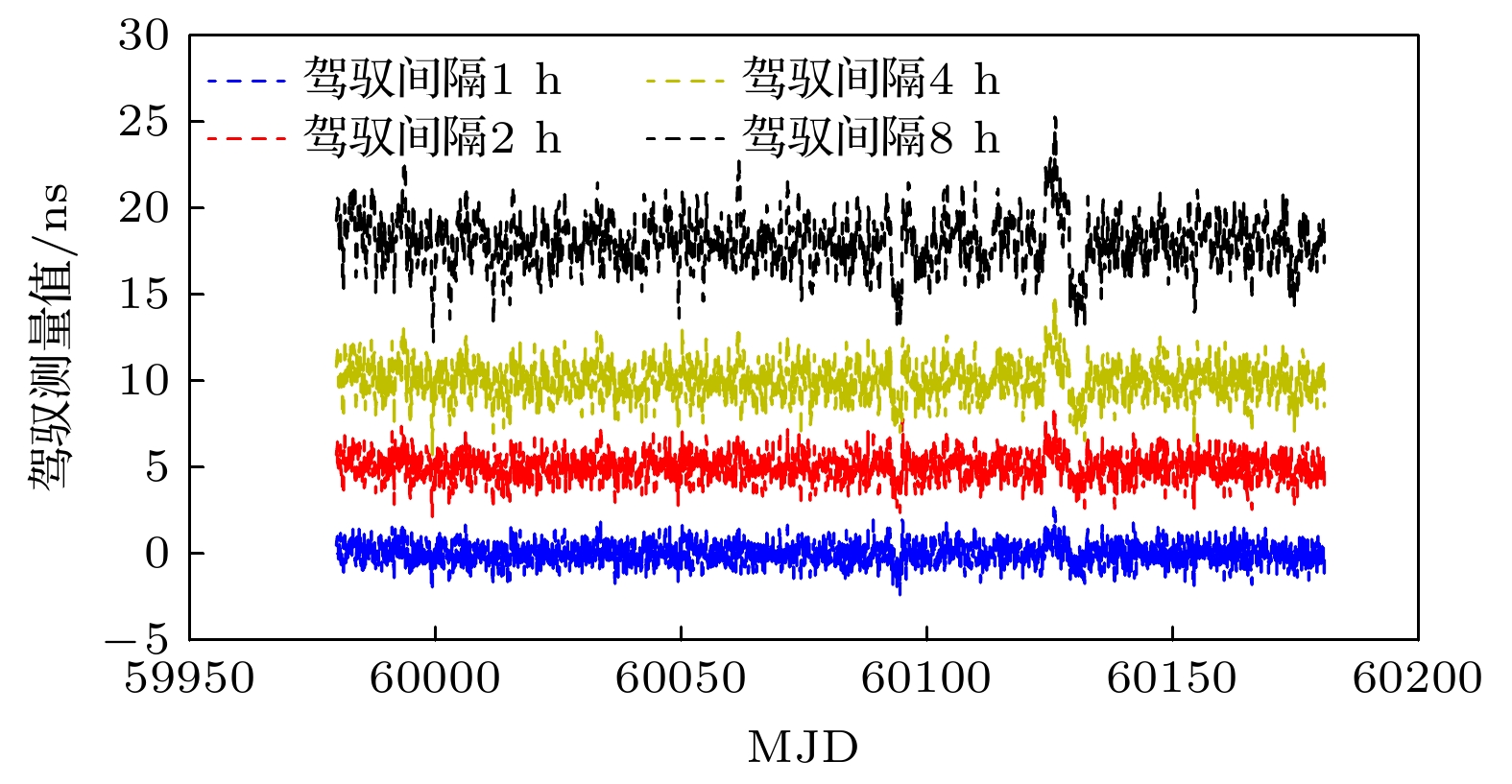
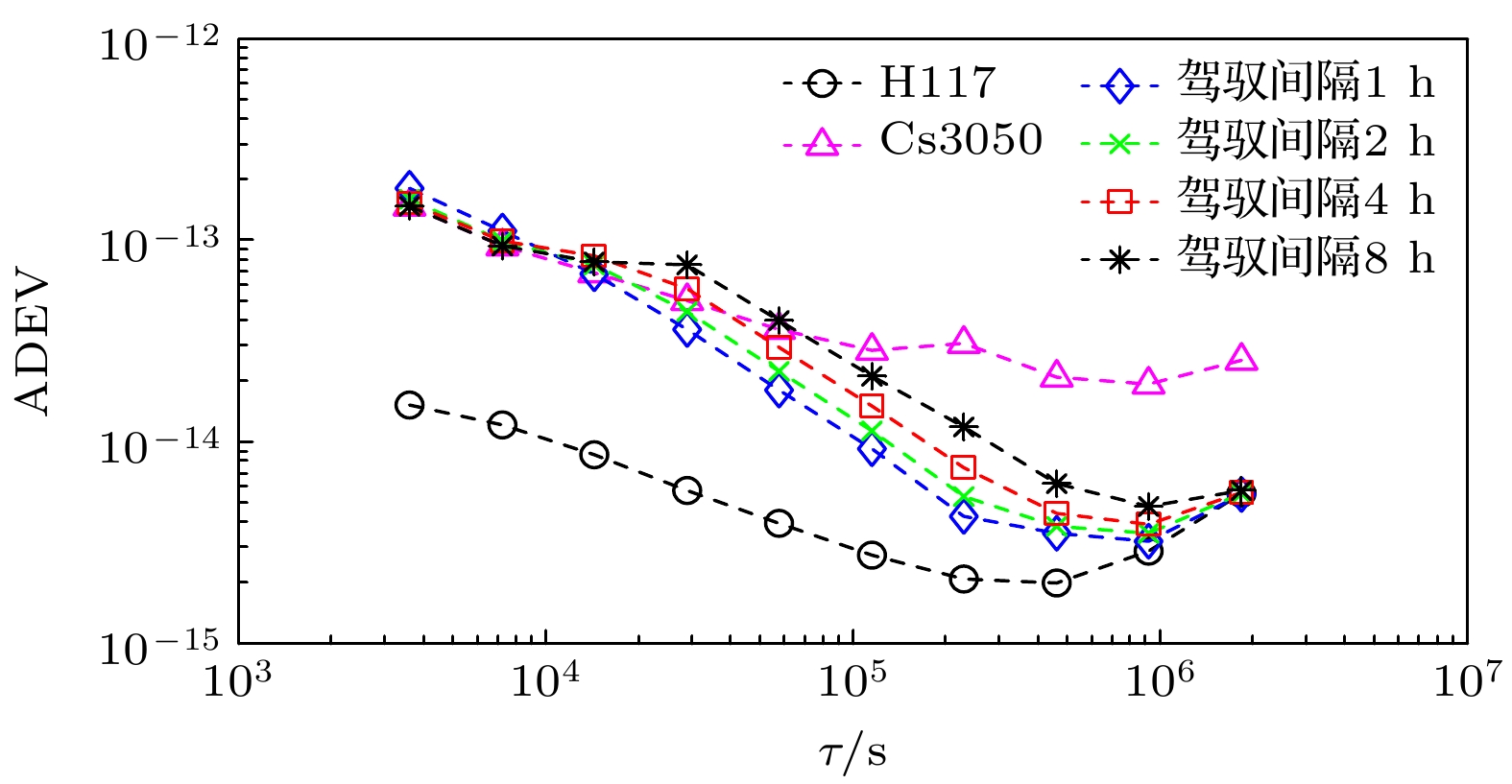
原子钟频率控制是时间保持工作中的关键技术. 当前守时工作中的频率控制主要针对国外微波钟采用开环控制算法, 但由于国产光抽运小铯钟(下称国产钟)的工作原理和性能不同于国外同类型原子钟, 因此该算法不能很好适应国产钟. 为了提升我国标准时间的自主性和安全性, 本文基于国产钟的噪声特性, 在最优控制理论的框架下研究了线性二次高斯控制算法, 该算法属于闭环控制算法, 从同步时间、频率控制准确度和频率控制稳定度方面研究国产钟性能, 最后分析了不同控制间隔对国产钟性能的影响. 结果表明随着二次损失函数中约束矩阵$ {{{W}}_{\text{R}}} $的增大, 同步时间延长, 控制准确度降低, 控制短期稳定度提高. $ {{{W}}_{\text{R}}} $相同情况下, 随着控制间隔的增大, 同步时间延长, 控制准确度降低, 控制短期稳定度提高, 对于$ {{{W}}_{\text{R}}} =1$时, 控制间隔为1 h的同步时间为5小时, 控制准确度为1.83 ns, 1 h的Allan偏差为1.81×10^–13; 控制间隔为8 h的同步时间为28 h, 控制准确度为4.48 ns, 1 h的Allan偏差为1.48×10^–13. 控制国产光抽运小铯钟的中长期稳定度都得到提高.

关键词:

Abstract

Frequency control of atomic clock is a key technology in time keeping operation. At present, the open-loop control algorithm is mainly used for the frequency control of foreign microwave clock, but the working principle and performance of domestic optically pumped small cesium clock (hereinafter referred to as domestic clock) are different from those of foreign atomic clock of the same type, so the algorithm cannot be well adapted to domestic clock. In order to improve the autonomy and security of the national standard time, based on the noise characteristics of domestic clock, in this work, the linear quadratic Gaussian control algorithm is studied in the framework of optimal control theory. This algorithm belongs to closed-loop control algorithm. The performance of domestic clock is studied from the aspects of synchronization time, frequency control accuracy and frequency control stability. Finally, the influence of different control intervals on the performance of domestic clock is analyzed. The results show that with the increase of the constraint matrix $ {{{W}}_{\text{R}}} $ in the quadratic loss function, the synchronization time increases, the control accuracy decreases, and the control short-term stability increases. When $ {{{W}}_{\text{R}}} $ is the same, with the increase of control interval, the synchronization time increases, the control accuracy decreases, and the control short-term stability increases. When $ {W_{\text{R}}} = 1 $, the synchronization time with control interval of 1 h is 5 h, the control accuracy is 1.83 ns, and the Allan deviation of 1 hour is 1.81×10^–13. When the control interval is 8 h, the synchronization time is 28 h, the control accuracy is 4.48 ns, and the Allan deviation of 1 h is 1.48×10^–13. The medium-term stability and long-term stability of domestic optically pumped small cesium clock are both improved.

Keywords:

作者及机构信息

1.
中国科学院国家授时中心, 时间频率基准实验室, 西安　710600

2.
时间基准及应用重点实验室(中国科学院), 西安　710600

3.
中国科学院大学天文与空间科学学院, 北京　100049

4.
西安工程大学电子信息学院, 西安　710048

5.
西安科技大学机械工程学院, 西安　710054

通信作者: 董绍武, sdong@ntsc.ac.cn

基金项目: 中国科学院 “西部之光”人才培养计划(批准号: XAB2021YN22)、北京无线电计量测试研究所开放基金(批准号: JLJK2021001A002)和陕西省自然科学基础研究计划(批准号: 2022JQ-014)资助的课题.

Authors and contacts

1.
National Time Service Center, Chinese Academy of Sciences, Xi’an 710600, China

2.
Key Laboratory of Time Reference and Applications, Chinese Academy of Sciences, Xi’an 710600, China

3.
School of Astronomy and Space Science, University of Chinese Academy of Sciences, Beijing 100049, China

4.
School of Electronics and information, Xi’an Polytechnic University, Xi’an 710048, China

5.
School of Mechanical Engineering, Xi’an University of Science and Technology, Xi’an 710054, China

Corresponding author: Dong Shao-Wu, sdong@ntsc.ac.cn

Funds: Project supported by the Western Light Project of Chinese Academy of Sciences (Grant No. XAB2021YN22), the Open Fund of Beijing Institute of Radio Metrology and Testing (Grant No. JLJK2021001A002), and the Natural Science Basic Research Program of Shaanxi Province, China (Grant No. 2022JQ-014).

文章全文 : translate this paragraph

参考文献

[1]	Godel M, Schmidt T D, Furthner J 2019 Metrologia 56 3 Google Scholar
[2]	Schmidt T D, Trainotti C, Furthner J 2019 Proceedings of the Precise Time and Time Interval Meeting ( ION PTTI 2019), Reston, Virginia, January 28–31, 2019 p290
[3]	Galleani L, Signorile G, Formichella V, Sesia I 2020 Metrologia 57 3 Google Scholar
[4]	Arias E, Panfilo G, Petit G 2011 Metrologia 48 S145 Google Scholar
[5]	McCarthy D 2011 Metrologia 48 S132 Google Scholar
[6]	Koppang P A 2016 Metrologia 53 R60 Google Scholar
[7]	陈法喜, 赵侃, 李立波, 郭宝龙 2022 71 230702 Google Scholar Chen F X, Zhao K, Li L B, Guo B L 2022 Acta Phys. Sin. 71 230702 Google Scholar
[8]	Song H J, Dong S W, Wu W J, Jiang M, Wang W X 2018 Metrologia 55 350 Google Scholar
[9]	韩孟纳, 童明雷 2023 72 079701 Google Scholar Han M N, Tong M L 2023 Acta Phys. Sin. 72 079701 Google Scholar
[10]	Kaczmarek J, Miczulski W, Koziol M, Czubla A 2013 IEEE Trans. Instrum. Meas 65 2828 Google Scholar
[11]	Panfilo G, Harmegnies A, Tisserand L 2012 Metrologia 49 49 Google Scholar
[12]	宋会杰, 董绍武, 屈俐俐, 王翔, 广伟 2017 仪器仪表学报 38 1809 Google Scholar Song H J, Dong S W, Qu L L, Wang X, Guang W 2017 Chin. J. Sci. Instrum. 38 1809 Google Scholar
[13]	Panfilo G, Harmegnies A, Tisserand L 2014 Metrologia 51 285 Google Scholar
[14]	宋会杰, 董绍武, 王翔, 章宇, 王燕平 2020 69 170201 Google Scholar Song H J, Dong S W, Wang X, Zhang Y, Wang Y P 2020 Acta Phys. Sin. 69 170201 Google Scholar
[15]	宋会杰, 董绍武, 王燕平, 安卫, 侯娟 2019 武汉大学学报(信息科学版) 44 1205 Google Scholar Song H J, Dong S W, Wang Y P, An W, Hou J 2019 Geomat. Inf. Sci. Wuhan Univ. 44 1205 Google Scholar
[16]	Panfilo G, Arias E F 2010 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 57 140 Google Scholar
[17]	Tavella P, Thomas C 1991 Metrologia 28 2 Google Scholar
[18]	刘云, 王文海, 贺德晶, 周勇壮, 沈咏, 邹宏新 2023 72 184202 Google Scholar Liu Y, Wang W H, He D J, Zhou Y Z, Shen Y, Zou H X 2023 Acta Phys. Sin. 72 184202 Google Scholar
[19]	梁悦, 谢勇辉, 陈鹏飞, 帅涛, 裴雨贤, 徐昊天, 赵阳, 夏天, 潘晓燕, 张朋军, 林传富 2023 72 013702 Google Scholar Liang Y, Xie Y H, Chen P F, Shuai T, Pei Y X, Xu H T, Zhao Y, Xia T, Pan X Y, Zhang P J, Lin C F 2023 Acta Phys. Sin. 72 013702 Google Scholar
[20]	邵晓东, 韩海年, 魏志义 2021 70 134204 Google Scholar Shao X D, Han H N, Wei Z Y 2021 Acta Phys. Sin. 70 134204 Google Scholar
[21]	Galleani L 2008 Metrologia 45 S175 Google Scholar
[22]	Tavella P 2008 Metrologia 45 S183 Google Scholar
[23]	Yao J, Parker T E, Ashby N, Levine J 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 65 127 Google Scholar

施引文献

图 1 铯原子钟与氢原子钟的Allan偏差曲线

Fig. 1. Allan deviation curves of cesium atomic clock and hydrogen atomic clock.

下载: 全尺寸图片幻灯片

图 2 国产钟的控制结构图

Fig. 2. Control structure diagram of domestic clock.

下载: 全尺寸图片幻灯片

图 3 不同$ {{{W}}_{\text{R}}} $值的控制同步时间比较

Fig. 3. Comparison of control synchronization time with different $ {{{W}}_{\text{R}}} $ values.

下载: 全尺寸图片幻灯片

图 4 同步后不同$ {{{W}}_{\text{R}}} $值的控制测量值

Fig. 4. Control measurements of different $ {{{W}}_{\text{R}}} $ values after synchronization

下载: 全尺寸图片幻灯片

图 5 约束矩阵$ {{{W}}_{\text{R}}} $取不同值时的控制国产钟的稳定度

Fig. 5. Stability of the controlled domestic clock with different values of the constrained matrix $ {{{W}}_{\text{R}}} $.

下载: 全尺寸图片幻灯片

图 6 约束矩阵$ {{{W}}_{\text{R}}} $取不同值时控制测量值的稳定度

Fig. 6. Stability of control measurement with different values of the constrained matrix $ {{{W}}_{\text{R}}} $.

下载: 全尺寸图片幻灯片

图 7 不同控制间隔的同步时间比较

Fig. 7. Comparison of synchronization time with different control interval.

下载: 全尺寸图片幻灯片

图 8 同步后不同控制间隔的测量值

Fig. 8. Measurements at different control intervals after synchronization.

下载: 全尺寸图片幻灯片

图 9 基于不同控制间隔的国产钟的稳定度

Fig. 9. Stability of the domestic clock based on different control intervals.

下载: 全尺寸图片幻灯片

图 10 基于不同控制间隔的控制测量值的稳定度

Fig. 10. Stability of control measurements based on different control intervals.

下载: 全尺寸图片幻灯片

图 11 控制后Cs3050与UTCr的相位偏差

Fig. 11. Phase deviation of the controlled Cs3050 from the UTCr.

下载: 全尺寸图片幻灯片

图 12 自由运行Cs3050与驾驭后Cs3050相对于UTCr的频率稳定度

Fig. 12. Frequency stability of free running Cs3050 and controlled Cs3050 relatived to UTCr.

下载: 全尺寸图片幻灯片

表 1 同步后不同$ {{{W}}_{\text{R}}} $值的3倍标准差($ 3\sigma $)

Table 1. Three times standard deviation of different $ {{{W}}_{\text{R}}} $values after synchronization.

	$ {{{W}}_{\text{R}}} $
	1/2	1	10²	10⁴
$ 3\sigma $的值/ns	1.76	1.83	3.03	6.26

下载: 导出CSV

表 2 同步后不同控制间隔测量值的3倍标准差($ 3\sigma $)

Table 2. Three times standard deviation of different control intervals after synchronization.

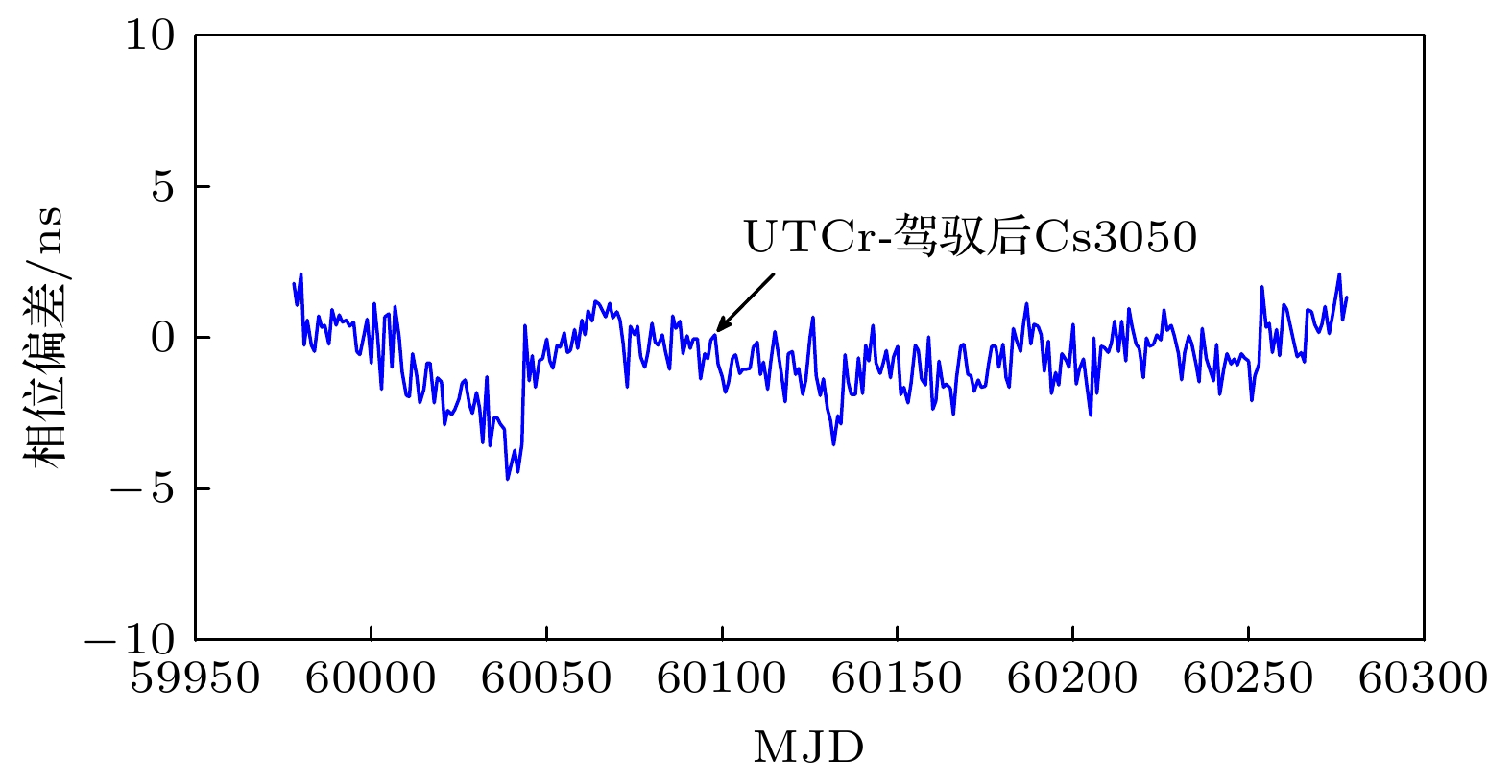
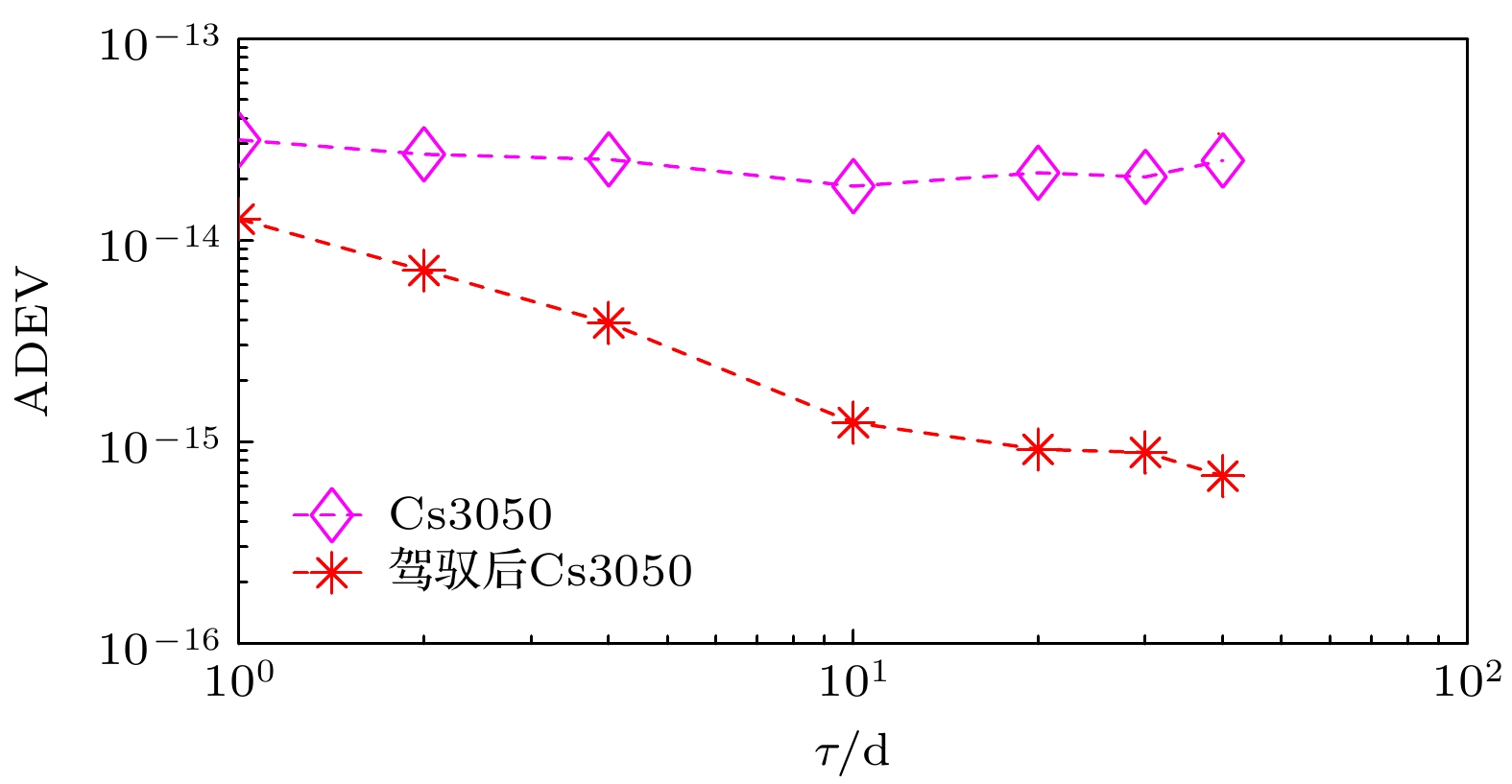
	控制间隔/h
	1	2	4	8
$ 3\sigma $的值/ns	1.83	2.29	3.10	4.48

下载: 导出CSV

表 3 自由运行Cs3050与驾驭后Cs3050相对于UTCr的频率稳定度

Table 3. Frequency stability of free running Cs3050 and controlled Cs3050 relative to UTCr.

	取样间隔/d
	10	20	30	40
Allan偏差 (Cs3050)	1.85×10^–14	2.16×10^–14	2.10×10^–14	2.50×10^–14
Allan偏差 (控制Cs3050)	1.24×10^–15	9.17×10^–16	8.86×10^–16	6.73×10^–16

下载: 导出CSV

表 4 自由运行 Cs3050相对于UTCr的频率漂移

Table 4. Frequency drift of free-running Cs3050 with respect to UTCr.

估计周期 (MJD)	59978—60007	60008—60037	60038—60067	60068—60097	60098—60127
频率漂移/(ns·d^–1)	0.1938	–0.0236	0.0446	–0.2062	0.1859

估计周期 (MJD)	60128—60157	60158—60187	60188—60217	60218—60247	60248—60277
频率漂移/(ns·d^–1)	–0.0374	–0.1158	0.3041	0.0457	0.1263

下载: 导出CSV

表 5 控制Cs3050相对于UTCr的频率漂移

Table 5. Frequency drift of controlled Cs3050 relative to UTCr.

估计周期(MJD)	59978—60007	60008—60037	60038—60067	60068—60097	60098—60127
频率漂移/(ns·d^–1)	0.0050	0.0010	–0.0131	0.0031	0.0016

估计周期(MJD)	60128—60157	60158—60187	60188—60217	60218—60247	60248—60277
频率漂移/(ns·d^–1)	–0.0088	0.0082	0.0143	–0.0029	–0.0003

下载: 导出CSV

map

[1]	Godel M, Schmidt T D, Furthner J 2019 Metrologia 56 3 Google Scholar
[2]	Schmidt T D, Trainotti C, Furthner J 2019 Proceedings of the Precise Time and Time Interval Meeting ( ION PTTI 2019), Reston, Virginia, January 28–31, 2019 p290
[3]	Galleani L, Signorile G, Formichella V, Sesia I 2020 Metrologia 57 3 Google Scholar
[4]	Arias E, Panfilo G, Petit G 2011 Metrologia 48 S145 Google Scholar
[5]	McCarthy D 2011 Metrologia 48 S132 Google Scholar
[6]	Koppang P A 2016 Metrologia 53 R60 Google Scholar
[7]	陈法喜, 赵侃, 李立波, 郭宝龙 2022 71 230702 Google Scholar Chen F X, Zhao K, Li L B, Guo B L 2022 Acta Phys. Sin. 71 230702 Google Scholar
[8]	Song H J, Dong S W, Wu W J, Jiang M, Wang W X 2018 Metrologia 55 350 Google Scholar
[9]	韩孟纳, 童明雷 2023 72 079701 Google Scholar Han M N, Tong M L 2023 Acta Phys. Sin. 72 079701 Google Scholar
[10]	Kaczmarek J, Miczulski W, Koziol M, Czubla A 2013 IEEE Trans. Instrum. Meas 65 2828 Google Scholar
[11]	Panfilo G, Harmegnies A, Tisserand L 2012 Metrologia 49 49 Google Scholar
[12]	宋会杰, 董绍武, 屈俐俐, 王翔, 广伟 2017 仪器仪表学报 38 1809 Google Scholar Song H J, Dong S W, Qu L L, Wang X, Guang W 2017 Chin. J. Sci. Instrum. 38 1809 Google Scholar
[13]	Panfilo G, Harmegnies A, Tisserand L 2014 Metrologia 51 285 Google Scholar
[14]	宋会杰, 董绍武, 王翔, 章宇, 王燕平 2020 69 170201 Google Scholar Song H J, Dong S W, Wang X, Zhang Y, Wang Y P 2020 Acta Phys. Sin. 69 170201 Google Scholar
[15]	宋会杰, 董绍武, 王燕平, 安卫, 侯娟 2019 武汉大学学报(信息科学版) 44 1205 Google Scholar Song H J, Dong S W, Wang Y P, An W, Hou J 2019 Geomat. Inf. Sci. Wuhan Univ. 44 1205 Google Scholar
[16]	Panfilo G, Arias E F 2010 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 57 140 Google Scholar
[17]	Tavella P, Thomas C 1991 Metrologia 28 2 Google Scholar
[18]	刘云, 王文海, 贺德晶, 周勇壮, 沈咏, 邹宏新 2023 72 184202 Google Scholar Liu Y, Wang W H, He D J, Zhou Y Z, Shen Y, Zou H X 2023 Acta Phys. Sin. 72 184202 Google Scholar
[19]	梁悦, 谢勇辉, 陈鹏飞, 帅涛, 裴雨贤, 徐昊天, 赵阳, 夏天, 潘晓燕, 张朋军, 林传富 2023 72 013702 Google Scholar Liang Y, Xie Y H, Chen P F, Shuai T, Pei Y X, Xu H T, Zhao Y, Xia T, Pan X Y, Zhang P J, Lin C F 2023 Acta Phys. Sin. 72 013702 Google Scholar
[20]	邵晓东, 韩海年, 魏志义 2021 70 134204 Google Scholar Shao X D, Han H N, Wei Z Y 2021 Acta Phys. Sin. 70 134204 Google Scholar
[21]	Galleani L 2008 Metrologia 45 S175 Google Scholar
[22]	Tavella P 2008 Metrologia 45 S183 Google Scholar
[23]	Yao J, Parker T E, Ashby N, Levine J 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 65 127 Google Scholar

[1]	梁悦, 谢勇辉, 陈鹏飞, 帅涛, 裴雨贤, 徐昊天, 赵阳, 夏天, 潘晓燕, 张朋军, 林传富. 氢原子钟双选态束光学系统仿真分析. , 2023, 72(1): 013702. doi: 10.7498/aps.72.20221363
[2]	陈泽锐, 刘光存, 俞振华. 谐振子势阱中双费米原子光钟的碰撞频移. , 2021, 70(18): 180602. doi: 10.7498/aps.70.20210243
[3]	张玉燕, 殷东哲, 温银堂, 罗小元. 基于自适应Kalman滤波的平面阵列电容成像. , 2021, 70(11): 118102. doi: 10.7498/aps.70.20210442
[4]	宋会杰, 董绍武, 王翔, 章宇, 王燕平. 原子钟噪声变化时改进的Kalman滤波时间尺度算法. , 2020, 69(17): 170201. doi: 10.7498/aps.69.20191920
[5]	卢晓同, 李婷, 孔德欢, 王叶兵, 常宏. 锶原子光晶格钟碰撞频移的测量. , 2019, 68(23): 233401. doi: 10.7498/aps.68.20191147
[6]	李婷, 卢晓同, 张强, 孔德欢, 王叶兵, 常宏. 锶原子光晶格钟黑体辐射频移评估. , 2019, 68(9): 093701. doi: 10.7498/aps.68.20182294
[7]	郭阳, 尹默娟, 徐琴芳, 王叶兵, 卢本全, 任洁, 赵芳婧, 常宏. 锶原子光晶格钟自旋极化谱线的探测. , 2018, 67(7): 070601. doi: 10.7498/aps.67.20172759
[8]	林弋戈, 方占军. 锶原子光晶格钟. , 2018, 67(16): 160604. doi: 10.7498/aps.67.20181097
[9]	徐琴芳, 尹默娟, 孔德欢, 王叶兵, 卢本全, 郭阳, 常宏. 光梳主动滤波放大实现锶原子光钟二级冷却光源. , 2018, 67(8): 080601. doi: 10.7498/aps.67.20172733
[10]	张曦, 刘慧, 姜坤良, 王进起, 熊转贤, 贺凌翔, 吕宝龙. 利用传输腔技术实现镱原子光钟光晶格场的频率稳定. , 2017, 66(16): 164205. doi: 10.7498/aps.66.164205
[11]	张星, 张奕, 张建伟, 张建, 钟础宇, 黄佑文, 宁永强, 顾思洪, 王立军. 894nm高温垂直腔面发射激光器及其芯片级铯原子钟系统的应用. , 2016, 65(13): 134204. doi: 10.7498/aps.65.134204
[12]	贾梦源, 赵刚, 侯佳佳, 谭巍, 邱晓东, 马维光, 张雷, 董磊, 尹王保, 肖连团, 贾锁堂. 双重频率锁定的腔衰荡吸收光谱技术及信号处理. , 2016, 65(12): 128701. doi: 10.7498/aps.65.128701
[13]	尹毅, 张奕, 谭伯仲, 陈杰华, 顾思洪. 芯片原子钟相干布居囚禁谱线特性研究. , 2015, 64(3): 034207. doi: 10.7498/aps.64.034207
[14]	林旭, 罗志才. 一种新的卫星钟差Kalman滤波噪声协方差估计方法. , 2015, 64(8): 080201. doi: 10.7498/aps.64.080201
[15]	刘洋洋, 廉保旺, 赵宏伟, 刘亚擎. Kalman滤波辅助的室内伪卫星相对定位算法. , 2014, 63(22): 228402. doi: 10.7498/aps.63.228402
[16]	吴长江, 阮军, 陈江, 张辉, 张首刚. 应用于铯原子喷泉钟的二维磁光阱研制. , 2013, 62(6): 063201. doi: 10.7498/aps.62.063201
[17]	高峰, 王叶兵, 田晓, 许朋, 常宏. 锶原子三重态谱线的观测及在光钟中的应用. , 2012, 61(17): 173201. doi: 10.7498/aps.61.173201
[18]	张路, 钟苏川, 彭皓, 罗懋康. 乘性二次噪声驱动的线性过阻尼振子的随机共振. , 2012, 61(13): 130503. doi: 10.7498/aps.61.130503
[19]	徐志君, 李鹏华. 玻色凝聚原子云的二次干涉及其放大效应. , 2007, 56(10): 5607-5612. doi: 10.7498/aps.56.5607
[20]	柯熙政, 吴振森. 原子钟噪声中的混沌现象及其统计特性. , 1998, 47(9): 1436-1449. doi: 10.7498/aps.47.1436

计量

文章访问数: 1610
PDF下载量: 51
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板