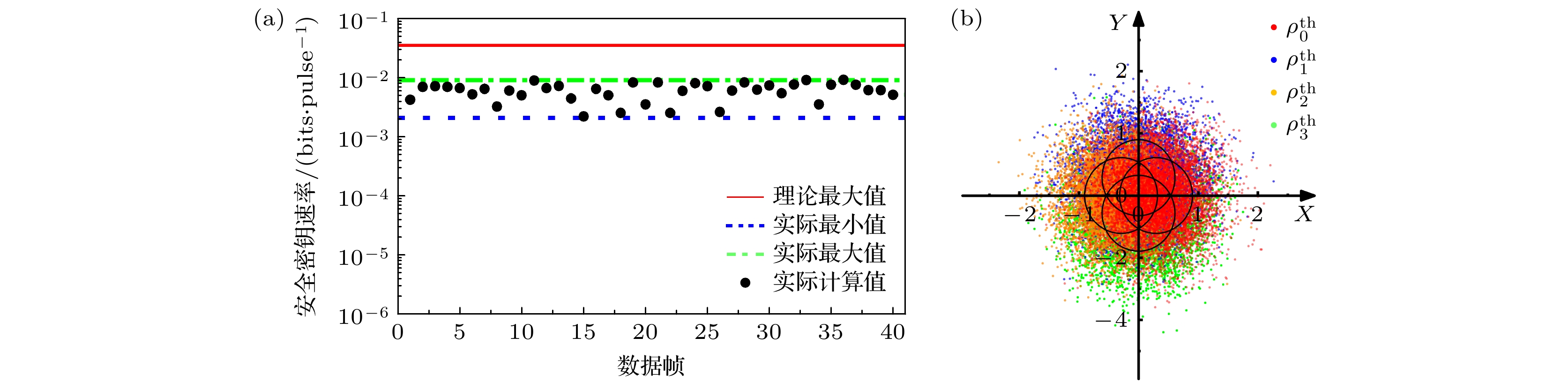
-
在连续变量量子密钥分发系统中, 同步技术是确保通信双方时钟和数据一致的关键技术. 本文通过巧妙设计发送端和接收端仪器的硬件时序, 采用时域差拍探测方式和峰值采集技术, 实验实现了可硬件同步的四态离散调制连续变量量子密钥分发. 通信双方在设计好的硬件同步时序下可实现时钟的恢复和数据的自动对齐, 无需借助软件算法实现数据的对齐. 本文采用了加拿大滑铁卢大学 Norbert Lütkenhaus研究组提出的针对连续变量离散调制协议的安全密钥速率计算方法. 该方法需计算出接收端所测各种平移热态的一阶矩和二阶(非中心)矩, 以此为约束条件结合凸优化算法可计算出安全密钥速率. 计算过程中无需假设信道为线性信道, 无需额外噪声的估算. 密钥分发系统重复频率为10 MHz, 传输距离为25 km, 平均安全密钥比特率为24 kbit/s. 本文提出的硬件同步方法无需过采样和软件帧同步, 减小了系统的复杂度和计算量, 在一定程度上降低了系统所需的成本、功耗和体积, 有效地增强了连续变量量子密钥分发的实用性.
-
关键词:
- 连续变量量子密钥分发 /
- 硬件同步 /
- 四态离散调制 /
- 时域差拍探测
In the case of continuous-variable quantum key distribution (CV-QKD) systems, synchronization is a key technology that ensures that both the transmitter and receiver obtain corresponding data synchronously. By designing an ingenious time sequence for the transmitter and receiver and using the peaking value acquisition technique and time domain heterodyne detection, we experimentally realize a four-state discrete modulation CV-QKD with a repetition rate of 10 MHz, transmitting over a distance of 25 km. With well-designed time sequence of hardware, Alice and Bob can obtain corresponding data automatically without using numerous software calculation methods. The secure key rates are calculated by using the method proposed by the Lütkenhaus group at the University of Waterloo in Canada. In the calculation, we first estimate the first and the second moment by using the measured quadratures of displaced thermal states, followed by calculating the secret key rate by using the convex optimization method through the reconstruction of the moments. There is no need to assume a linear quantum transmission channel to estimate the excess noise. Finally, secure key rates of 0.0022—0.0091 bit/pulse are achieved, and the excess noise is between 0.016 and 0.103. In this study, first, we introduce the prepare-and-measure scheme and the entanglement-based scheme of the four-state discrete modulation protocol. The Wigner images of the four coherent states on Alice’s side, and four displaced thermal states on Bob’s side are presented. Second, the design of hardware synchronization time series is introduced comprehensively. Third, the CV-QKD experiment setup is introduced and the time sequence is verified. Finally, the calculation method of secure key rate using the first and the second moment of quadrature is explained in detail. The phase space distribution of quadratures is also presented. The secret key rate ranges between 0.0022 and 0.0091 bits/pulse, and the equivalent excess noise are between 0.016 and 0.103. The average secret key bit rate is 24 kbit/s. During the experiment, the first and the second moment of the quantum state at the receiver end are found to fluctuate owing to the finite-size effect. This effect reduces the value of the secure key rate and limits the transmission distance of the CV-QKD system. In conclusion, four-state discrete modulation CV-QKD based on hardware synchronization is designed and demonstrated. The proposed hardware synchronization method can effectively reduce the cost, size, and power consumption. In the future, the finite-size effect will be investigated theoretically and experimentally to improve the performance of system. -
Keywords:
- continuous variable quantum key distribution /
- hardware synchronization /
- four-state discrete modulation /
- time domain heterodyne detection
[1] Xu F, Ma X, Zhang Q, Lo H K, Pan J W 2020 Rev. Mod. Phys. 92 025002
Google Scholar
[2] Pirandola S, Andersen U L, Banchi L, Berta M, Bunandar D, Colbeck R, Englund D, Gehring T, Lupo C, Ottaviani, Pereira J L, Razavi M, Shamsul Shaari J, Tomamichel M, Usenko V C, Vallone G, Villoresi P, Wallden P 2020 Adv. Opt. Photonics 12 1012
Google Scholar
[3] Fan-Yuan G J, Lu F L, Wang S, Yin Z Q, He D Y, Zhou Z, Teng J, Chen W, Guo G C, Han Z F 2021 Photonics Res. 9 1881
Google Scholar
[4] Liu H, Jiang C, Zhu H T, Zou M, Yu Z W, Hu X L, Xu H, Ma S, Han Z, Chen J P, Dai Y, Tang S B, Zhang W, Li H, You L, Wang Z, Hua Y, Hu H, Zhang H, Zhou F, Zhang Q, Wang X B, Chen T Y, Pan J W 2021 Phys. Rev. Lett. 126 250502
Google Scholar
[5] Grosshans F, Van Assche G, Wenger J, Brouri R, Cerf N J, Grangier P 2003 Nature 421 238
Google Scholar
[6] Xu H, Hu X L, Jiang C, Yu Z W, Wang X B 2023 Phys. Rev. Res. 5 023069
Google Scholar
[7] Jiang C, Yu Z W, Hu X L, Wang X B 2023 Natl. Sci. Rev. 10 186
Google Scholar
[8] Yin J, Li Y H, Liao S K, Yang M, Cao Y, Zhang Y, Ren J G, Cai W Q, Liu W Y, Li S L, Shu R, Huang Y M, Deng L, Li L, Zhang Q, Liu N L, Chen Y A, Lu C Y, Wang X B, Xu F H, Wang J Y, Peng C Z, Ekert A K, Pan J W 2020 Nature 582 501
Google Scholar
[9] Fang X T, Zeng P, Liu H, Zou M, Wu W J, Tang Y L, Sheng Y J, Zhang W, Li L, Li M J, Chen H A, Zhang Q, Peng C Z, Ma X, Chen T Y, Pan J W 2020 Nat. Photonics 14 422
Google Scholar
[10] Zhu H T, Huang Y Z, Liu H, Zeng P, Zou M, Dai Y Q, Tang S B, Li H, You L X, Wang Z, Chen Y A, Ma X F, Chen T Y, Pan J W 2023 Phys. Rev. Lett. 130 030801
Google Scholar
[11] Du Y Q, Zhu X, Hua X, Zhao Z G, Hu X, Qian Y, Xiao X, Wei K J 2023 Chip 2 100039
Google Scholar
[12] Wei K J, Li W, Tan H, Li Y, Min H, Zhang W J, Li H, You L X, Wang Z, Jiang X, Chen T Y, Liao S K, Peng C Z, Xu F H, Pan J W 2020 Phys. Rev. X 10 031030
Google Scholar
[13] Huang P, Wang T, Huang D, Zeng G H 2022 Symmetry 14 568
Google Scholar
[14] Wang H, Pan Y, Shao Y, Pi Y D, Ye T, Li Y, Zhang T, Liu J L, Yang J, Ma L, Huang W, Xu B J 2023 Opt. Express 31 5577
Google Scholar
[15] Sun S H, Xu F H 2021 New J. Phys. 23 023011
Google Scholar
[16] Sun S H 2021 Phys. Rev. A 104 022423
Google Scholar
[17] Huang P, Huang J Z, Zhang Z S, Zeng G H 2018 Phys. Rev. A 97 042311
Google Scholar
[18] Zhang Y C, Li Z Y, Yu S, Gu W Y, Peng X, Guo H 2014 Phys. Rev. A 90 052325
Google Scholar
[19] Qi B, Gunther H, Evans P G, Williams B P, Camacho R M, Peters N A 2020 Phys. Rev. Appl. 13 054065
Google Scholar
[20] Tian Y, Wang P, Liu J Q, Du S N, Liu W Y, Lu Z G, Wang X Y, Li Y M 2022 Optica 9 492
Google Scholar
[21] Tian Y, Zhang Y, Liu S S, Wang P, Lu Z G, Wang X Y, Li Y M 2023 Opt. Lett. 48 2953
Google Scholar
[22] Wang T, Xu Y, Zhao H, Li L, Huang P, Zeng G H 2023 Opt. Lett. 48 719
Google Scholar
[23] Wang P, Zhang Y, Lu Z G, Wang X Y, Li Y M 2023 New J. Phys. 25 023019
Google Scholar
[24] Du S N, Wang P, Liu J Q, Tian Y, Li Y M 2023 Photonics Res. 11 463
Google Scholar
[25] Chen J P, Zhang C, Liu Y, Jiang C, Zhang W, Han Z Y, Ma S Z, Hu X L, Li Y H, Liu H, Zhou F, Jiang H F, Chen T Y, Li H, You L X, Wang Z, Wang X B, Zhang Q, Pan J W 2021 Nat. Photonics 15 570
Google Scholar
[26] Wang S, Yin Z Q, He D Y, Chen W, Wang R Q, Ye P, Zhou Y, Fan-Yuan G J, Wang F X, Chen W, Zhu Y G, Morozov P V, Divochiy A V, Zhou Z, Guo G C, Han F Z 2022 Nat. Photonics 16 154
Google Scholar
[27] Liu Y, Zhang W J, Jiang C, Chen J P, Zhang C, Pan W X, Ma D, Dong H, Xiong J M, Zhang C J, Li H, Chen T Y, You L X, Wang X B, Zhang Q, Pan J W 2023 Phys. Rev. Lett. 130 210801
Google Scholar
[28] Pan Y, Wang H, Shao Y, Pi Y D, Liu B, Huang W, Xu B J 2022 Opt. Lett. 47 3307
Google Scholar
[29] Wang H, Li Y, Pi Y D, Pan Y, Shao Y, Ma L, Zhang Y C, Yang J, Zhang Tao, Huang W, Xu B J 2022 Commun. Phys. 5 162
Google Scholar
[30] Hajomer A A E, Bruynsteen C, Derkach I, Jain N, Bomhals A, Bastiaens S, Andersen U L, Yin X, Gehring T 2023 arXiv: 2305.19642v1[quant-ph]
[31] Zhang Y C, Chen Z Y, Pirandola S, Wang X Y, Zhou C, Chu B J, Zhao Y J, Xu B J, Yu S, Guo H 2020 Phys. Rev. Lett. 125 010502
Google Scholar
[32] Zhang G, Haw J Y, Cai H, Xu F H, Assad S M, Fitzsimons J F, Zhou X, Zhang Y, Yu S, Wu J, Ser W, Kwek L C, Liu A Q 2019 Nat. Photonics 13 839
Google Scholar
[33] Wang X Y, Jia Y X, Guo X B, Liu J Q, Wang S F, Liu W Y, Sun F Y, Zou J, Li Y M 2022 Chin. Opt. Lett. 20 041301
Google Scholar
[34] Jia Y X, Wang X Y, Hu X, Hua X, Zhang Y, Guo X B, Zhang S X, Xiao X, Yu S H, Zou J, Li Y M 2023 New J. Phys. 25 103030
Google Scholar
[35] Li L, Wang T, Li X H, Huang P, Guo Y Y, Lu L J, Zhou L J, Zeng G H 2023 Photonics Res. 11 504
Google Scholar
[36] Leverrier A, Grangier P 2009 Phys. Rev. Lett. 102 180504
Google Scholar
[37] Leverrier A, Grosshans F, Grangier P 2010 Phys. Rev. A 81 062343
Google Scholar
[38] Lin J, Upadhyaya T, Lutkenhaus N 2019 Phys. Rev. X 9 041064
Google Scholar
[39] Ghorai S, Grangier P, Diamanti E, Leverrier A 2019 Phys. Rev. X 9 021059
Google Scholar
[40] Lupo C, Ouyang Y K 2022 PRX Quantum 3 010341
Google Scholar
[41] Ma H X, Huang P, Bai D Y, Wang T, Wang S Y, Bao W S, Zeng G H 2019 Phys. Rev. A 99 022322
Google Scholar
[42] Liu W B, Li C L, Xie Y M, Weng C X, Gu J, Cao X Y, Lu Y S, Li B H, Yin H L, Chen Z B 2021 PRX Quantum 2 040334
Google Scholar
[43] Wang X Y, Bai Z L, Wang S F, Li Y M, Peng K C 2013 Chin. Phys. Lett. 30 010305
Google Scholar
[44] Pereira D, Almeida M, Facao M F, Pinto A N, Silva N A 2022 Opt. Lett. 47 3948
Google Scholar
[45] Kleis S, Rueckmann M, Schaeffe C G 2017 Opt. Lett. 42 1588
Google Scholar
[46] Milovancev D, Vokic N, Laudenbach F, Pacher C, Hübel H, Schrenk B 2021 J. Lightwave Technol. 39 3445
Google Scholar
[47] Li H S, Wang C, Huang P, Huang D, Wang T, Zeng G H 2016 Opt. Express 24 20481
Google Scholar
[48] Wang C, Huang P, Huang D, Lin D K, Zeng G H 2016 Phys. Rev. A 93 022315
Google Scholar
[49] 刘友明, 汪超, 黄端, 黄鹏, 冯晓毅, 彭进业, 曹正文, 曾贵华 2015 光学学报 35 0106006
Google Scholar
Liu Y M, Wang C, Huang D, Huang P, Feng X Y, Peng J Y, Cao Z W, Zeng G H 2015 Acta Opt. Sin. 35 0106006
Google Scholar
[50] Lin J, Lütkenhaus N 2020 Phys. Rev. Appl. 14 064030
Google Scholar
[51] Lodewyck J, Bloch M, Garcia-Patron R, Fossier S, Karpov E, Diamanti E, Debuisschert T, Cerf N J, Tualle-Brouri R, McLaughlin S W, Grangier P 2007 Phys. Rev. A 76 042305
Google Scholar
[52] Wang X Y, Liu J Q, Li X F, Li Y M 2015 IEEE J. Quantum Electron. 51 5200206
Google Scholar
[53] Wang X Y, Liu W Y, Wang P, Li Y M 2017 Phys. Rev. A 95 062330
Google Scholar
[54] Du S N, Li Z Y, Liu W Y, Wang X Y, Li Y M 2018 J. Opt. Soc. Am. B 35 481
Google Scholar
[55] Wang X Y, Guo X B, Jia Y X, Zhang Y, Lu Z G, Liu J Q, Li Y M 2023 J. Lightwave Technol. 41 5518
Google Scholar
[56] Qi B, Lougovski P, Pooser R, Grice W, Bobrek M 2015 Phys. Rev. X 5 041009
Google Scholar
[57] 刘建强, 王旭阳, 白增亮, 李永民 2016 65 100303
Google Scholar
Liu J Q, Wang X Y, Bai Z L, Li Y M 2016 Acta Phys. Sin. 65 100303
Google Scholar
-
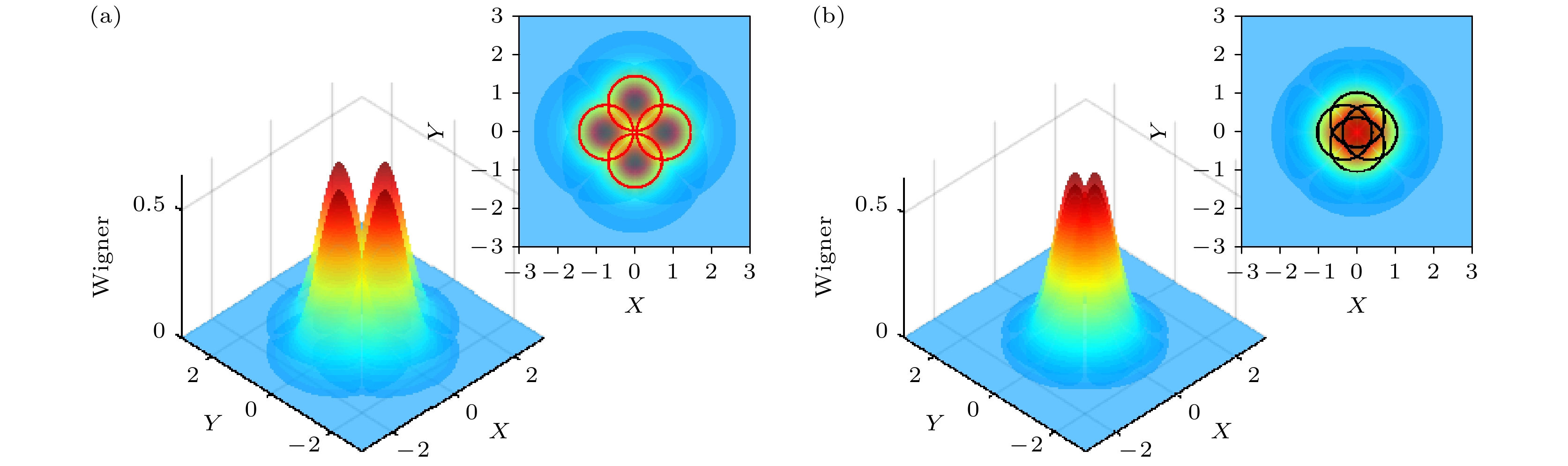
图 1 发送端和接收端的量子态在相空间中的Wigner函数图形 (a) 发送端Alice制备的四个相干态的Wigner函数图形和其俯视图; (b) 接收端Bob接收到的四个平移热态的Wigner函数图形和其俯视图
Fig. 1. Wigner pictures of the quantum states of Alice and Bob: (a) The Wigner pictures of four coherent states prepared by Alice and their top view; (b) the Wigner functions of four displaced thermal states received by Bob and their top view.
图 2 CV-QKD系统的电信号时序图 (a) 发送端Alice的电信号时序图; (b) 接收端Bob的电信号时序图
Fig. 2. Timing diagrams of the CV-QKD system: (a) The timing diagram of Alice; (b) the timing diagram of Bob.
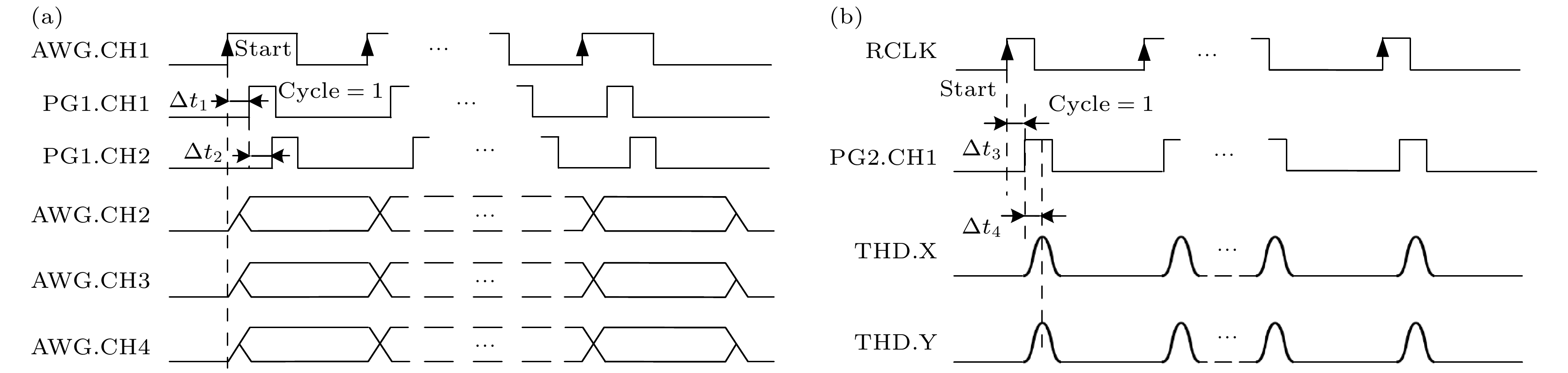
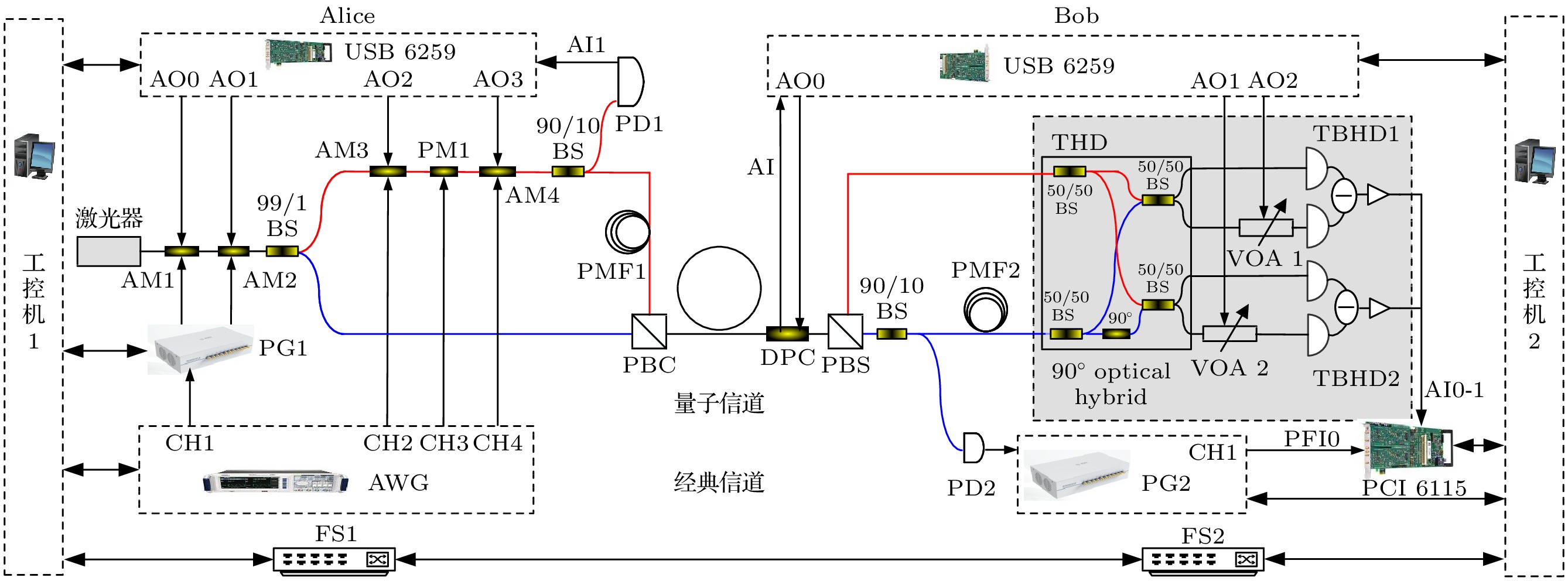
图 3 基于硬件同步方案的四态离散调制CV-QKD系统光路图. AM, 振幅调制器; PM, 相位调制器; PG, 脉冲发生器; AWG, 任意波形发生器; PD, 光电探测器; PMF, 保偏光纤; PBC, 偏振合束器; PBS, 偏振合束器; DPC, 动态偏振控制器; VOA, 可调光衰减器; THD, 时域差拍探测器; TBHD, 时域平衡零拍探测器; FS, 光纤交换机
Fig. 3. Scheme of the four-state discrete modulation CV-QKD system based on the hardware synchronization method. AM, amplitude modulator; PM, phase modulator; PG, pulse generator; AWG, arbitrary waveform generator; PD, photodetector; PMF, polarization maintaining fiber; PBC, polarization beam combiner; PBS, polarization beam splitter; DPC, dynamic polarization controller; VOA, variable optical attenuator; THD, time domain heterodyne detector; TBHD, time domain balanced homodyne detector; FS, fiber switch.
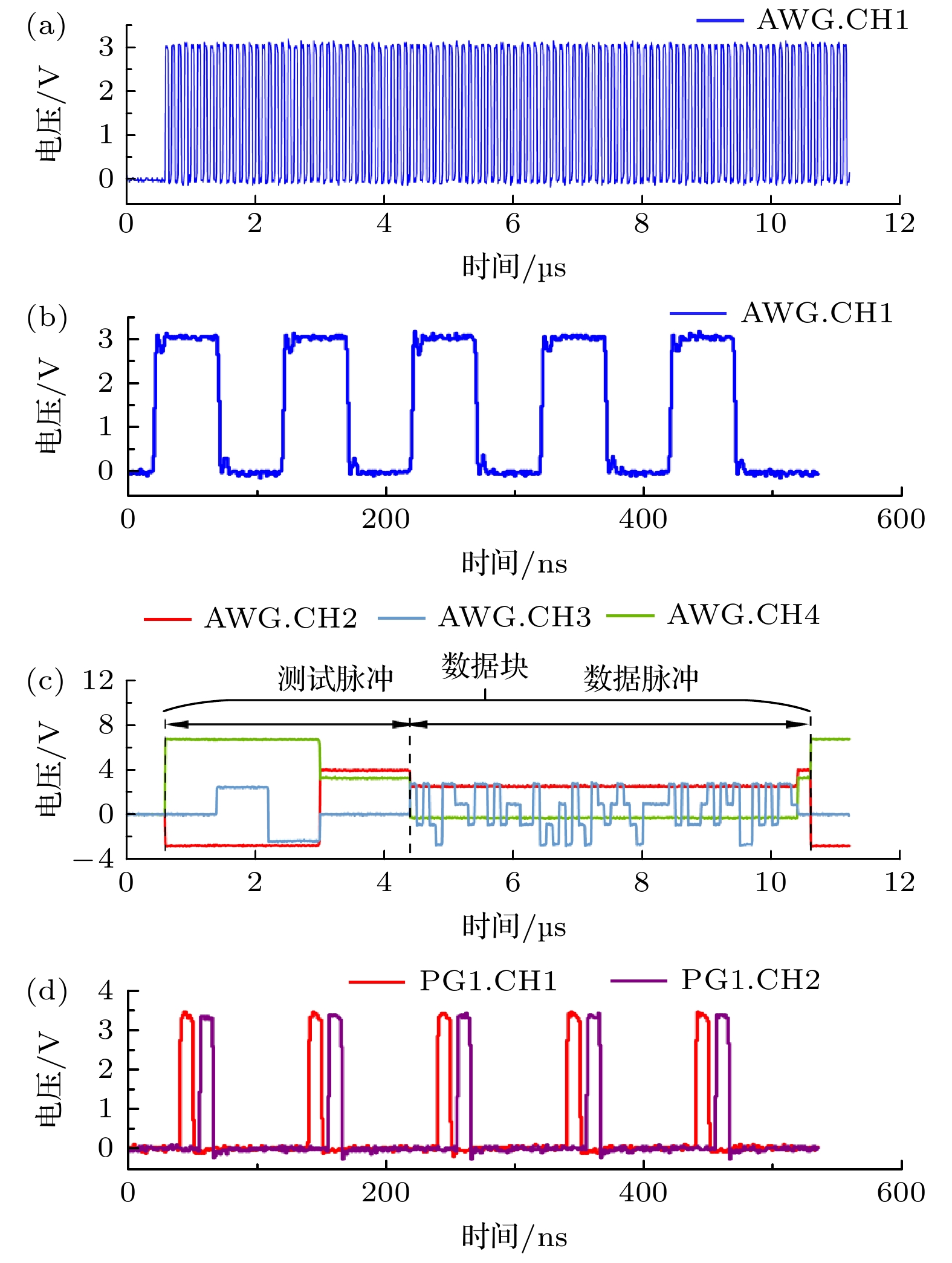
图 4 发送端Alice的各种信号波形 (a) AWG.CH1输出的时钟信号波形; (b) 图(a)的展开波形; (c) AWG.CH2-4输出的一个数据块的调制信号波形; (d) PG1.CH1-2输出的脉冲信号波形
Fig. 4. Various waveform at Alice’s side: (a) The waveform of clock signals generated by AWG.CH1; (b) the expanded waveform of panel (a); (c) the waveform of one block modulated signals generated by AWG.CH2-4; (d) the waveform of pulse signals generated by PG1.CH1-2.
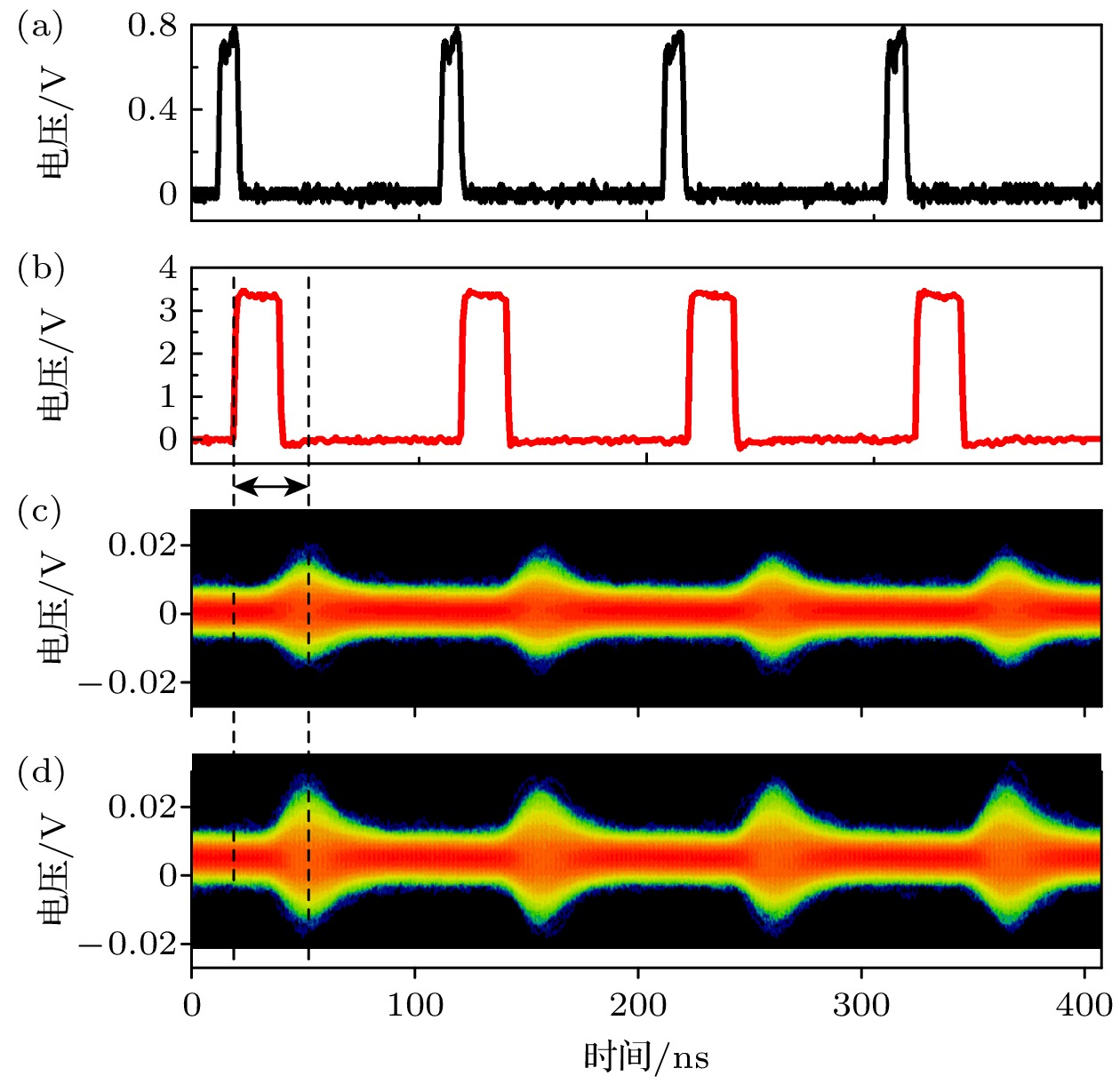
图 5 接收端Bob的各种信号波形 (a) PD2输出的恢复时钟信号波形; (b) PG 2.CH1输出的时钟信号波形; (c) THD输出的散粒噪声色温图; (d) THD输出的平移热态的色温图
Fig. 5. Various waveform at Bob’s side: (a) The waveform of recovery clock signals generated by PD2; (b) the waveform of clock signals generated by PG2.CH1; (c) the color temperature waveform of the shot noise generated by THD; (d) the color temperature waveform of the displaced thermal states generated by THD.
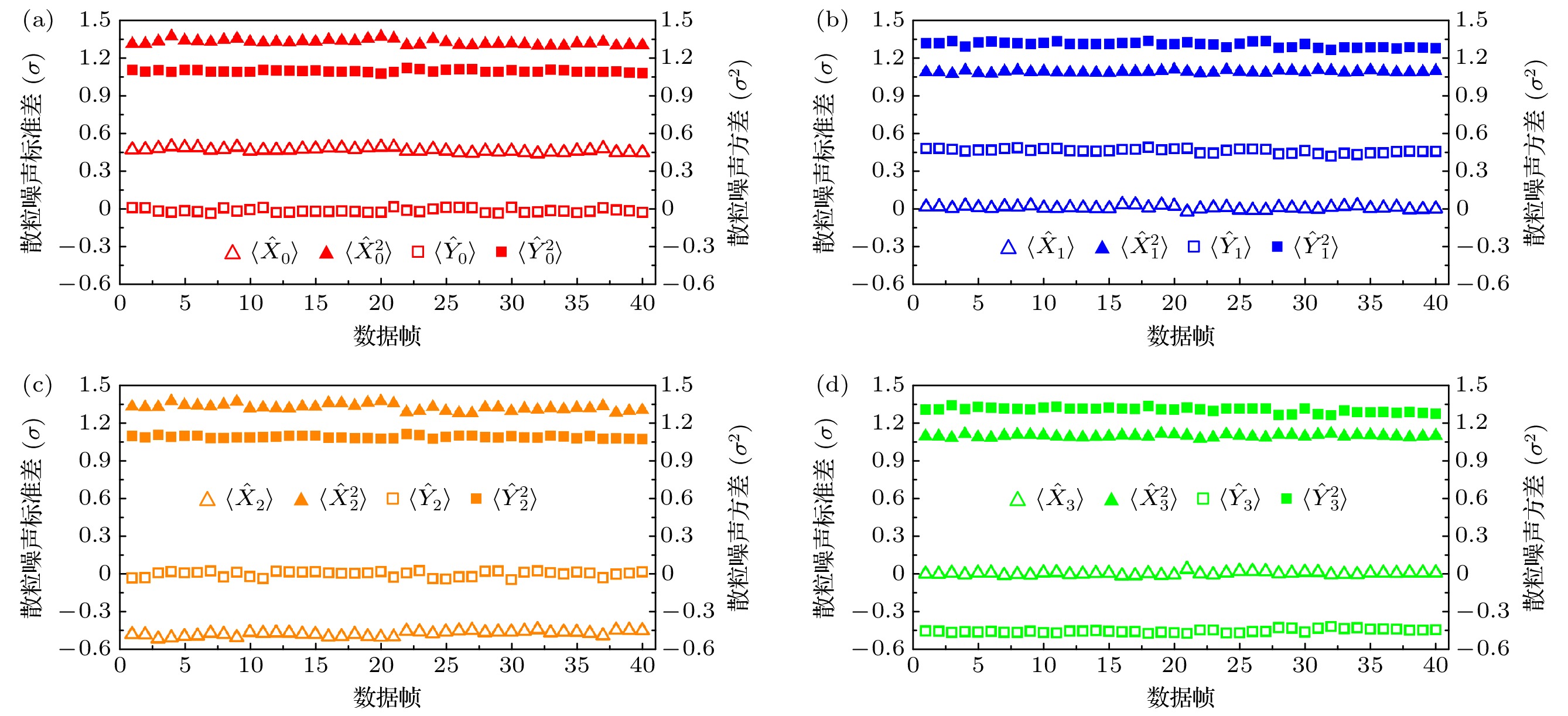
图 6 各平移热态正交分量的一阶矩和二阶矩的测量值 (a) 平移热态$ \rho _0^{{\text{th}}} $测量值; (b) 平移热态$ \rho _1^{{\text{th}}} $测量值; (c) 平移热态$ \rho _2^{{\text{th}}} $测量值; (d) 平移热态$ \rho _3^{{\text{th}}} $测量值
Fig. 6. Measurement results of the first and second moments of quadratures of the displaced thermal states: (a) Measurement results of displaced thermal state $ \rho _0^{{\text{th}}} $; (b) measurement results of displaced thermal state $ \rho _1^{{\text{th}}} $; (c) measurement results of displaced thermal state $ \rho _2^{{\text{th}}} $; (d) measurement results of displaced thermal state $ \rho _{3}^{{\text{th}}} $.
图 7 安全密钥速率和正交分量值的相空间分布图 (a) 每帧数据的安全密钥速率; (b) 平移热态正交分量值的相空间分布图
Fig. 7. Secure key rates and the phase space distribution of quadratures: (a) The secret key rate of each frame; (b) the phase space distribution of quadratures of displaced thermal states.
表 1 正交分量一阶矩和二阶矩的相关统计量
Table 1. Statistical quantities of the first and second moments of quadratures.
$ \langle {{{\hat X}_0}} \rangle $ $ \langle {{{\hat X}^2}_0} \rangle $ $ \langle {{{\hat Y}_0}} \rangle $ $ \langle {{{\hat Y}^2}_0} \rangle $ $ \langle {{{\hat X}_1}} \rangle $ $ \langle {{{\hat X}^2}_1} \rangle $ $ \langle {{{\hat Y}_1}} \rangle $ $ \langle {{{\hat Y}^2}_1} \rangle $ 最大值 0.494 1.37 0.017 1.12 0.037 1.11 0.492 1.34 最小值 0.438 1.29 –0.035 1.07 –0.021 1.07 0.421 1.27 均值 0.467 1.32 –0.012 1.09 0.012 1.09 0.470 1.31 方差 2.35×10–4 4.09×10–4 2.37×10–4 8.69×10–5 1.58×10–4 7.22×10–5 2.81×10–4 3.98×10–4 期望值 0.470 1.31 –9.09×10–5 1.08 –2.44×10–4 1.08 0.4710 1.30 $ \langle {{{\hat X}_2}} \rangle $ $ \langle {{{\hat X}^2}_2} \rangle $ $ \langle {{{\hat Y}_2}} \rangle $ $ \langle {{{\hat Y}^2}_2} \rangle $ $ \langle {{{\hat X}_3}} \rangle $ $ \langle {{{\hat X}^2}_3} \rangle $ $ \langle {{{\hat Y}_3}} \rangle $ $ \langle {{{\hat Y}^2}_3} \rangle $ 最大值 –0.444 1.38 0.024 1.11 0.034 1.11 –0.425 1.34 最小值 –0.514 1.28 –0.047 1.07 –0.018 1.08 –0.478 1.26 均值 –0.477 1.33 –0.002 1.09 –0.007 1.10 –0.458 1.30 方差 3.86×10–4 6.51×10–4 4.74×10–4 1.01×10–4 1.07×10–4 9.70×10–5 1.90×10–4 3.90×10–4 期望值 –0.469 1.31 –1.56×10–4 1.08 –3.11×10–4 1.09 –0.472 1.30 
下载: 导出CSV
-
[1] Xu F, Ma X, Zhang Q, Lo H K, Pan J W 2020 Rev. Mod. Phys. 92 025002
Google Scholar
[2] Pirandola S, Andersen U L, Banchi L, Berta M, Bunandar D, Colbeck R, Englund D, Gehring T, Lupo C, Ottaviani, Pereira J L, Razavi M, Shamsul Shaari J, Tomamichel M, Usenko V C, Vallone G, Villoresi P, Wallden P 2020 Adv. Opt. Photonics 12 1012
Google Scholar
[3] Fan-Yuan G J, Lu F L, Wang S, Yin Z Q, He D Y, Zhou Z, Teng J, Chen W, Guo G C, Han Z F 2021 Photonics Res. 9 1881
Google Scholar
[4] Liu H, Jiang C, Zhu H T, Zou M, Yu Z W, Hu X L, Xu H, Ma S, Han Z, Chen J P, Dai Y, Tang S B, Zhang W, Li H, You L, Wang Z, Hua Y, Hu H, Zhang H, Zhou F, Zhang Q, Wang X B, Chen T Y, Pan J W 2021 Phys. Rev. Lett. 126 250502
Google Scholar
[5] Grosshans F, Van Assche G, Wenger J, Brouri R, Cerf N J, Grangier P 2003 Nature 421 238
Google Scholar
[6] Xu H, Hu X L, Jiang C, Yu Z W, Wang X B 2023 Phys. Rev. Res. 5 023069
Google Scholar
[7] Jiang C, Yu Z W, Hu X L, Wang X B 2023 Natl. Sci. Rev. 10 186
Google Scholar
[8] Yin J, Li Y H, Liao S K, Yang M, Cao Y, Zhang Y, Ren J G, Cai W Q, Liu W Y, Li S L, Shu R, Huang Y M, Deng L, Li L, Zhang Q, Liu N L, Chen Y A, Lu C Y, Wang X B, Xu F H, Wang J Y, Peng C Z, Ekert A K, Pan J W 2020 Nature 582 501
Google Scholar
[9] Fang X T, Zeng P, Liu H, Zou M, Wu W J, Tang Y L, Sheng Y J, Zhang W, Li L, Li M J, Chen H A, Zhang Q, Peng C Z, Ma X, Chen T Y, Pan J W 2020 Nat. Photonics 14 422
Google Scholar
[10] Zhu H T, Huang Y Z, Liu H, Zeng P, Zou M, Dai Y Q, Tang S B, Li H, You L X, Wang Z, Chen Y A, Ma X F, Chen T Y, Pan J W 2023 Phys. Rev. Lett. 130 030801
Google Scholar
[11] Du Y Q, Zhu X, Hua X, Zhao Z G, Hu X, Qian Y, Xiao X, Wei K J 2023 Chip 2 100039
Google Scholar
[12] Wei K J, Li W, Tan H, Li Y, Min H, Zhang W J, Li H, You L X, Wang Z, Jiang X, Chen T Y, Liao S K, Peng C Z, Xu F H, Pan J W 2020 Phys. Rev. X 10 031030
Google Scholar
[13] Huang P, Wang T, Huang D, Zeng G H 2022 Symmetry 14 568
Google Scholar
[14] Wang H, Pan Y, Shao Y, Pi Y D, Ye T, Li Y, Zhang T, Liu J L, Yang J, Ma L, Huang W, Xu B J 2023 Opt. Express 31 5577
Google Scholar
[15] Sun S H, Xu F H 2021 New J. Phys. 23 023011
Google Scholar
[16] Sun S H 2021 Phys. Rev. A 104 022423
Google Scholar
[17] Huang P, Huang J Z, Zhang Z S, Zeng G H 2018 Phys. Rev. A 97 042311
Google Scholar
[18] Zhang Y C, Li Z Y, Yu S, Gu W Y, Peng X, Guo H 2014 Phys. Rev. A 90 052325
Google Scholar
[19] Qi B, Gunther H, Evans P G, Williams B P, Camacho R M, Peters N A 2020 Phys. Rev. Appl. 13 054065
Google Scholar
[20] Tian Y, Wang P, Liu J Q, Du S N, Liu W Y, Lu Z G, Wang X Y, Li Y M 2022 Optica 9 492
Google Scholar
[21] Tian Y, Zhang Y, Liu S S, Wang P, Lu Z G, Wang X Y, Li Y M 2023 Opt. Lett. 48 2953
Google Scholar
[22] Wang T, Xu Y, Zhao H, Li L, Huang P, Zeng G H 2023 Opt. Lett. 48 719
Google Scholar
[23] Wang P, Zhang Y, Lu Z G, Wang X Y, Li Y M 2023 New J. Phys. 25 023019
Google Scholar
[24] Du S N, Wang P, Liu J Q, Tian Y, Li Y M 2023 Photonics Res. 11 463
Google Scholar
[25] Chen J P, Zhang C, Liu Y, Jiang C, Zhang W, Han Z Y, Ma S Z, Hu X L, Li Y H, Liu H, Zhou F, Jiang H F, Chen T Y, Li H, You L X, Wang Z, Wang X B, Zhang Q, Pan J W 2021 Nat. Photonics 15 570
Google Scholar
[26] Wang S, Yin Z Q, He D Y, Chen W, Wang R Q, Ye P, Zhou Y, Fan-Yuan G J, Wang F X, Chen W, Zhu Y G, Morozov P V, Divochiy A V, Zhou Z, Guo G C, Han F Z 2022 Nat. Photonics 16 154
Google Scholar
[27] Liu Y, Zhang W J, Jiang C, Chen J P, Zhang C, Pan W X, Ma D, Dong H, Xiong J M, Zhang C J, Li H, Chen T Y, You L X, Wang X B, Zhang Q, Pan J W 2023 Phys. Rev. Lett. 130 210801
Google Scholar
[28] Pan Y, Wang H, Shao Y, Pi Y D, Liu B, Huang W, Xu B J 2022 Opt. Lett. 47 3307
Google Scholar
[29] Wang H, Li Y, Pi Y D, Pan Y, Shao Y, Ma L, Zhang Y C, Yang J, Zhang Tao, Huang W, Xu B J 2022 Commun. Phys. 5 162
Google Scholar
[30] Hajomer A A E, Bruynsteen C, Derkach I, Jain N, Bomhals A, Bastiaens S, Andersen U L, Yin X, Gehring T 2023 arXiv: 2305.19642v1[quant-ph]
[31] Zhang Y C, Chen Z Y, Pirandola S, Wang X Y, Zhou C, Chu B J, Zhao Y J, Xu B J, Yu S, Guo H 2020 Phys. Rev. Lett. 125 010502
Google Scholar
[32] Zhang G, Haw J Y, Cai H, Xu F H, Assad S M, Fitzsimons J F, Zhou X, Zhang Y, Yu S, Wu J, Ser W, Kwek L C, Liu A Q 2019 Nat. Photonics 13 839
Google Scholar
[33] Wang X Y, Jia Y X, Guo X B, Liu J Q, Wang S F, Liu W Y, Sun F Y, Zou J, Li Y M 2022 Chin. Opt. Lett. 20 041301
Google Scholar
[34] Jia Y X, Wang X Y, Hu X, Hua X, Zhang Y, Guo X B, Zhang S X, Xiao X, Yu S H, Zou J, Li Y M 2023 New J. Phys. 25 103030
Google Scholar
[35] Li L, Wang T, Li X H, Huang P, Guo Y Y, Lu L J, Zhou L J, Zeng G H 2023 Photonics Res. 11 504
Google Scholar
[36] Leverrier A, Grangier P 2009 Phys. Rev. Lett. 102 180504
Google Scholar
[37] Leverrier A, Grosshans F, Grangier P 2010 Phys. Rev. A 81 062343
Google Scholar
[38] Lin J, Upadhyaya T, Lutkenhaus N 2019 Phys. Rev. X 9 041064
Google Scholar
[39] Ghorai S, Grangier P, Diamanti E, Leverrier A 2019 Phys. Rev. X 9 021059
Google Scholar
[40] Lupo C, Ouyang Y K 2022 PRX Quantum 3 010341
Google Scholar
[41] Ma H X, Huang P, Bai D Y, Wang T, Wang S Y, Bao W S, Zeng G H 2019 Phys. Rev. A 99 022322
Google Scholar
[42] Liu W B, Li C L, Xie Y M, Weng C X, Gu J, Cao X Y, Lu Y S, Li B H, Yin H L, Chen Z B 2021 PRX Quantum 2 040334
Google Scholar
[43] Wang X Y, Bai Z L, Wang S F, Li Y M, Peng K C 2013 Chin. Phys. Lett. 30 010305
Google Scholar
[44] Pereira D, Almeida M, Facao M F, Pinto A N, Silva N A 2022 Opt. Lett. 47 3948
Google Scholar
[45] Kleis S, Rueckmann M, Schaeffe C G 2017 Opt. Lett. 42 1588
Google Scholar
[46] Milovancev D, Vokic N, Laudenbach F, Pacher C, Hübel H, Schrenk B 2021 J. Lightwave Technol. 39 3445
Google Scholar
[47] Li H S, Wang C, Huang P, Huang D, Wang T, Zeng G H 2016 Opt. Express 24 20481
Google Scholar
[48] Wang C, Huang P, Huang D, Lin D K, Zeng G H 2016 Phys. Rev. A 93 022315
Google Scholar
[49] 刘友明, 汪超, 黄端, 黄鹏, 冯晓毅, 彭进业, 曹正文, 曾贵华 2015 光学学报 35 0106006
Google Scholar
Liu Y M, Wang C, Huang D, Huang P, Feng X Y, Peng J Y, Cao Z W, Zeng G H 2015 Acta Opt. Sin. 35 0106006
Google Scholar
[50] Lin J, Lütkenhaus N 2020 Phys. Rev. Appl. 14 064030
Google Scholar
[51] Lodewyck J, Bloch M, Garcia-Patron R, Fossier S, Karpov E, Diamanti E, Debuisschert T, Cerf N J, Tualle-Brouri R, McLaughlin S W, Grangier P 2007 Phys. Rev. A 76 042305
Google Scholar
[52] Wang X Y, Liu J Q, Li X F, Li Y M 2015 IEEE J. Quantum Electron. 51 5200206
Google Scholar
[53] Wang X Y, Liu W Y, Wang P, Li Y M 2017 Phys. Rev. A 95 062330
Google Scholar
[54] Du S N, Li Z Y, Liu W Y, Wang X Y, Li Y M 2018 J. Opt. Soc. Am. B 35 481
Google Scholar
[55] Wang X Y, Guo X B, Jia Y X, Zhang Y, Lu Z G, Liu J Q, Li Y M 2023 J. Lightwave Technol. 41 5518
Google Scholar
[56] Qi B, Lougovski P, Pooser R, Grice W, Bobrek M 2015 Phys. Rev. X 5 041009
Google Scholar
[57] 刘建强, 王旭阳, 白增亮, 李永民 2016 65 100303
Google Scholar
Liu J Q, Wang X Y, Bai Z L, Li Y M 2016 Acta Phys. Sin. 65 100303
Google Scholar
下载:
计量
- 文章访问数: 2259
- PDF下载量: 82
- 被引次数: 0
