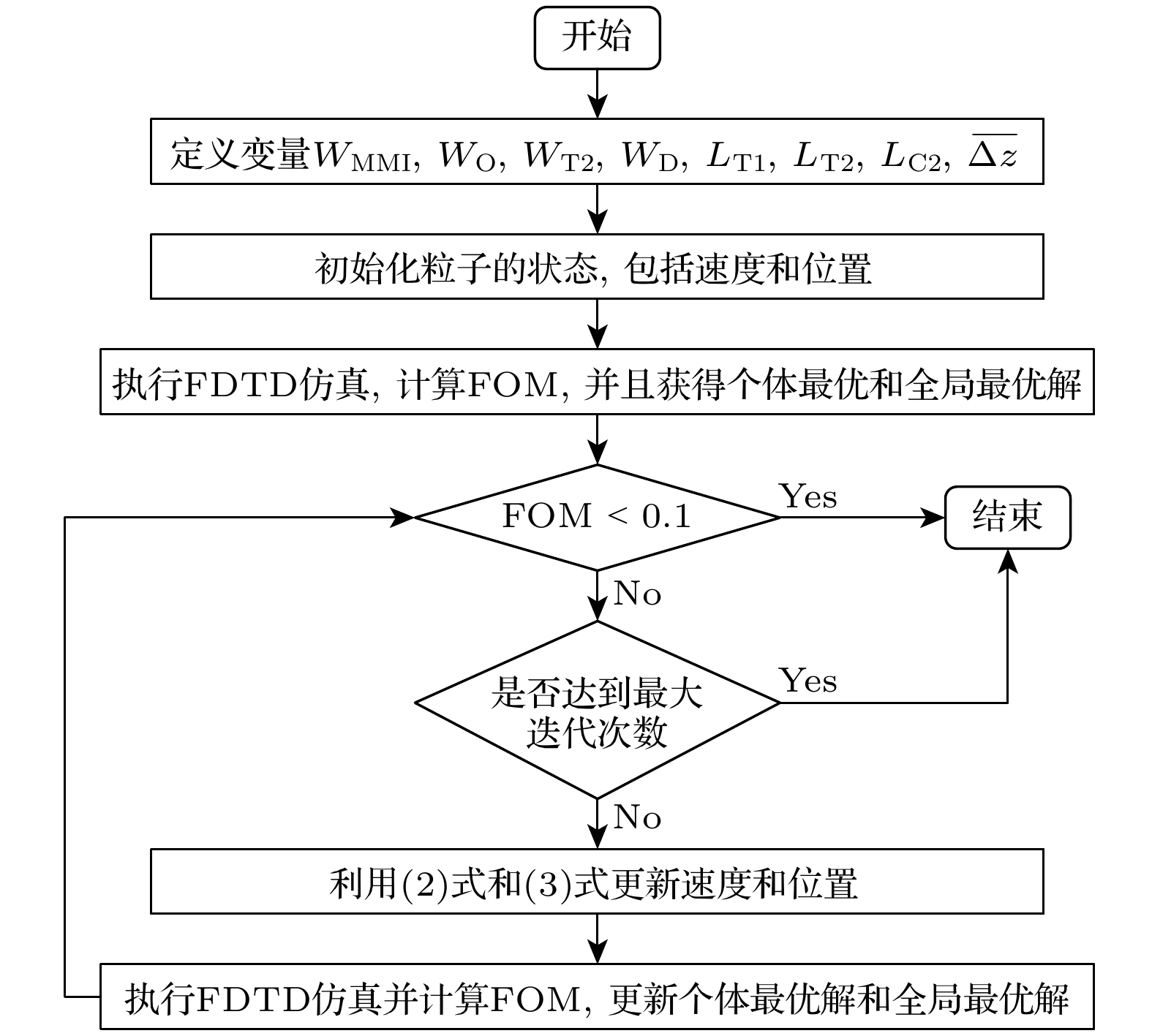
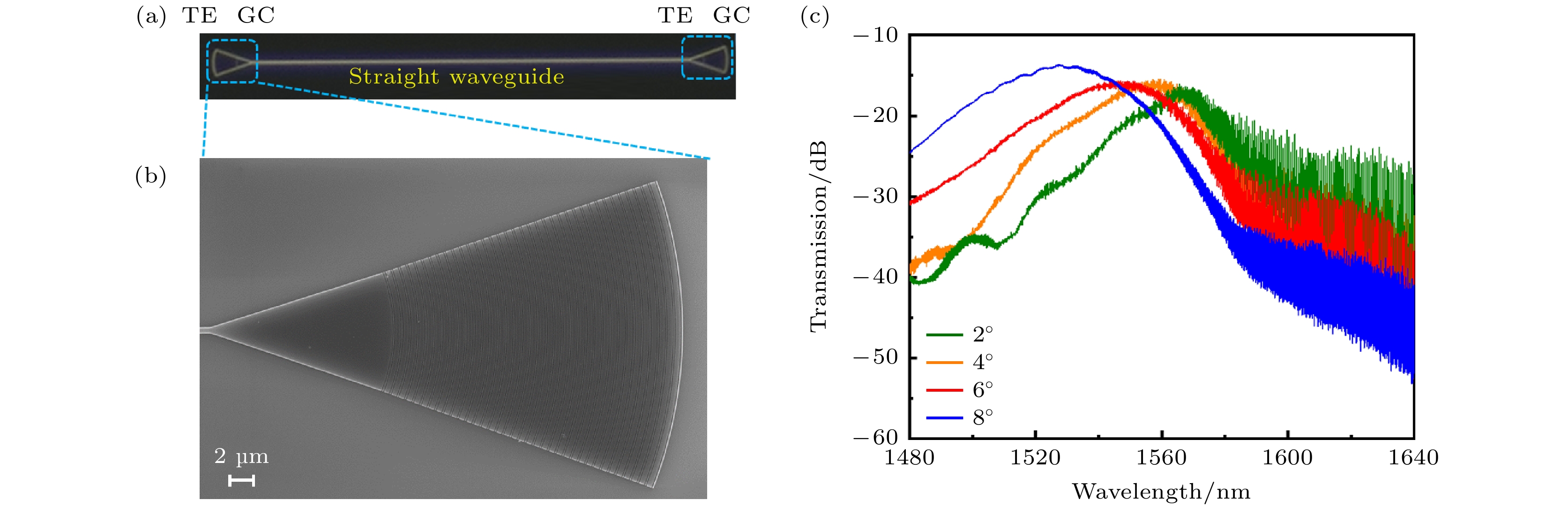
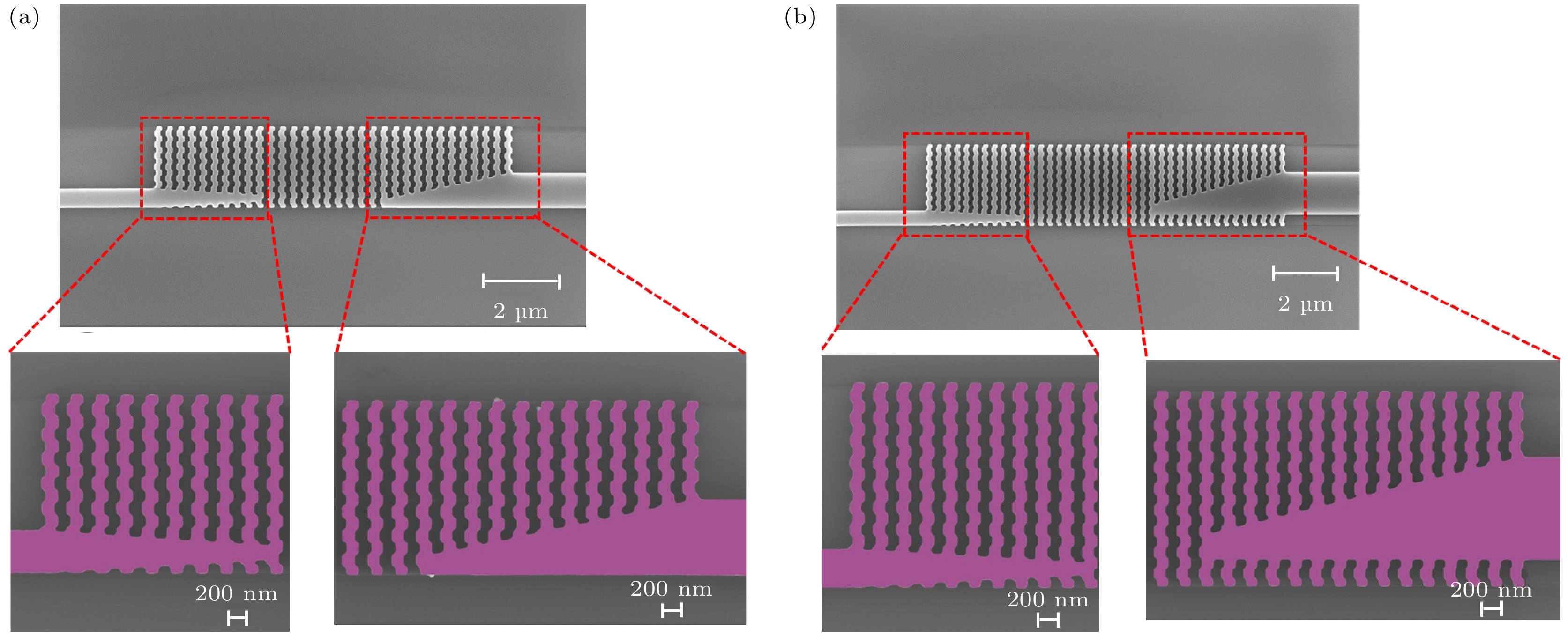
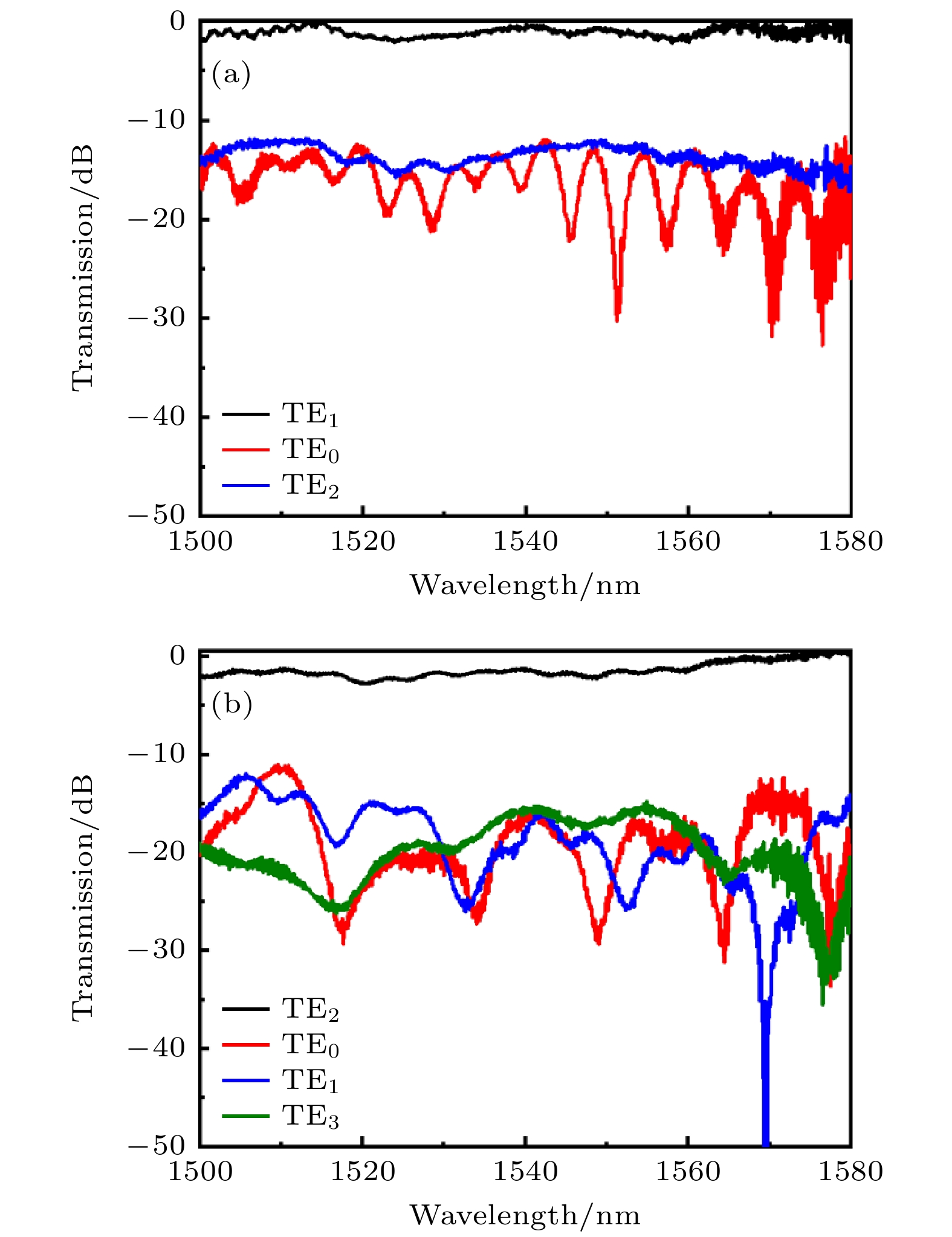
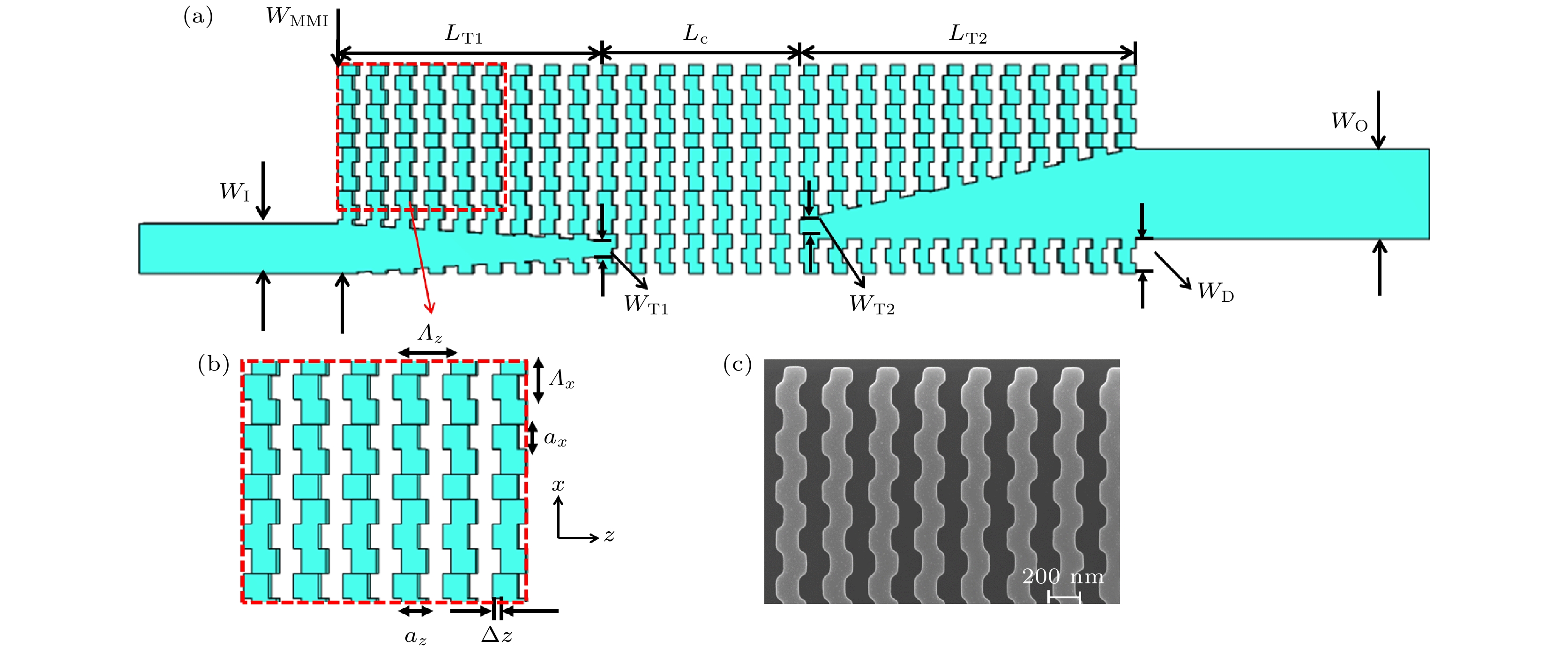
-
亚波长光栅可以等效为均匀介质, 具备可控的双折射、色散和各向异性等优势, 有利于设计高性能的光子器件. 尽管目前传统的亚波长光栅结构只需要单步刻蚀, 然而通常需要100 nm及以下的制造分辨率, 这对当前主流的晶圆级硅光子芯片制造技术来说比较困难. 亚波长光栅的各向异性可以通过引入砖砌型拓扑结构来进一步设计, 从而在设计中提供额外的自由度, 同时还可以降低制造分辨率需求(> 100 nm). 本文提出并研究了基于硅基砖砌型亚波长光栅的紧凑型TE0-TE1和TE0-TE2模式转换器, 其中砖砌型亚波长光栅的最小特征尺寸为145 nm. 实现了TE0模式到TE1模式和TE2模式的转换, 转换区域长度分别为9.39 µm和11.27 µm. 测试结果表明, 在68 nm (1512—1580 nm, 受限于激光器调谐范围和光栅耦合器)带宽内, 插损和串扰分别小于2.5 dB和–10 dB.Facing the increasing capacity requirements of on-chip optical interconnects, mode division multiplexing technology (MDM), which fully uses the different spatial eigenmodes at the same wavelength as independent channels to transmit optical signals, has attracted tremendous interest. Mode-order converter that can convert the fundamental mode into high-order mode is a key component in MDM system. However, it is still very challenging to achieve compact mode-order converters with high performances. Subwavelength grating (SWG) can be equivalent to homogenous material, which has the prominent advantages such as controlling over birefringence, dispersion and anisotropy, thus making photonic devices possess high performance. Wheras the conventional SWG only needs single-etch step, but the implementation of SWG structure usually requires a fabrication resolution on the order of 100 nm and below, which is difficult for current wafer-scale fabrication technology. The anisotropic response of SWG can be further engineered by introducing bricked topology structure, providing an additional degree of freedom in the design. Meanwhile, the requirement for fabrication resolution can also be reduced (> 100 nm). In this work, we experimentally demonstrate compact TE0-TE1 mode-order converter and TE0-TE2 mode-order converter by using a bricked subwavelength grating (BSWG) based on a silicon-on-insulator (SOI) with the BSWG having a minimum feature size of 145 nm. In the proposed mode-order converter, a quasi-TE0 mode is generated in the BSWG region, which can be regarded as an effective bridge between the two TE modes to be converted. Flexible mode conversion can be realized by only choosing appropriate structural parameters for specific mode transitions between input/output modes and the quasi-TE0 mode. By combining three-dimensional (3D) finite difference time domain (FDTD) and particle swarm optimization (PSO) method, TE0-TE1 mode-order converter and TE0-TE2 mode-order converter are optimally designed. They can convert TE0 mode into TE1 and TE2 mode with conversion length of 9.39 µm and 11.27 µm, respectively. The simulation results show that the insertion loss of < 1 dB and crosstalk of < –15 dB are achieved for both TE0-TE1 mode-order converter and TE0-TE2 mode-order converter, their corresponding working bandwidths being 128 nm (1511–1639 nm) and 126 nm (1527–1653 nm), respectively. The measurement results indicate that insertion loss and crosstalk are, respectively, less than 2.5 dB and –10 dB in a bandwidth of 68 nm (1512–1580 nm, limited by the laser tuning range and grating coupler).
-
Keywords:
- subwavelength grating /
- mode-order converter /
- silicon-on-insulator /
- anisotropy
[1] Luo L W, Ophir N, Chen C P, Gabrielli L H, Poitras C B, Bergmen K, and Lipson M 2014 Nat. Commun. 5 3069
Google Scholar
[2] Li C L, Liu D J, and Dai D X 2019 Nanophotonics 8 227
Google Scholar
[3] Hsu Y, Chuang C Y, Wu X R, Chen G H, Hsu C W, Chang Y C, Chow C W, Chen J, Lai Y C, Yeh C H, Tsang H K 2018 IEEE Photonics Technol. L. 30 1052
Google Scholar
[4] Li H Q, Wang P J, Yang T J, Dai T, Wang G C, Li S Q, Chen W W, Yang J Y 2018 Opt. Laser Technol. 100 7
Google Scholar
[5] Pan T H and Tseng S Y 2015 Opt. Express 23 10405
Google Scholar
[6] Xu Y, Liu L P, Hu X, Dong Y, Zhang B, Ni Y 2022 Opt. Laser Technol. 151 108028
Google Scholar
[7] Ohana D, Levy U 2014 Opt. Express 22 27617
Google Scholar
[8] Gabrielli L H, Liu D, Johnson S G, Lipson M 2012 Nat. Commun. 3 1217
Google Scholar
[9] Xu H N, Shi Y C 2018 Laser Photonics Rev. 12 1700240
Google Scholar
[10] Chang W J, Lu L L Z, Ren X S, Lu L H, Cheng M F, Liu D M, Zhang M M 2018 IEEE Photonics J. 10 4501008
Google Scholar
[11] Garcia-Rodriguez D, Corral J L, Griol A, Llorente R 2017 Opt. Lett. 42 1221
Google Scholar
[12] Chen W W, Wang P J, Yang J Y 2014 IEEE Photonics Technol. L. 26 2043
Google Scholar
[13] Oner B B, Ustun K, Kurt H, Okyay A K, Turhan-Sayan G 2015 Opt. Express 23 3186
Google Scholar
[14] Chack D, Hassan S, Qasim M 2020 Appl. Opt. 59 3652
Google Scholar
[15] Liu L P, Xu Y, Wen L, Dong Y, Zhang B, Ni Y 2019 Appl. Optics 58 9075
Google Scholar
[16] Cheben P, Halir R, Schmid J H, Atwater H A, Smith D R 2018 Nature 560 565
Google Scholar
[17] Luque-González J M, Sánchez-Postigo A, Hadij-ElHouati A, Ortega-Moñux A, Wangüemert-Pérez J G, Schmid J H, Cheben P, Molina-Fernández I, Halir R, 2021 Nanophotonics 10 2765
Google Scholar
[18] Yu Z J, Xu H N, Liu D J, Li H, Shi Y C, Dai D X 2022 J. Lightwave Technol. 40 1784
Google Scholar
[19] Wu F, Liu T T, Long Y, Xiao S Y, Chen G Y 2023 Phys. Rev. B 107 165428
Google Scholar
[20] Mia M B, Jaidye N, Ahmed I, Ahmed S Z, Kim S 2023 Opt. Express 31 4140
Google Scholar
[21] He Y, Zhang Y, Zhu Q M, An S, Cao R Y, Guo X H, Qiu C Y, Su Y K 2018 J. Lightwave Technol. 36 5746
Google Scholar
[22] González-Andrade D, Gonzalo Wanguemert-Perez J, Velasco A V, Ortega-Monux A, Herrero-Bermello A, Molina-Fernandez I, Halir R, Cheben P 2018 IEEE Photonics J. 10 2201010
Google Scholar
[23] González-Andrade D, Dias A, Wanguemert-Perez J G, Ortega-Monux A, Molina-Fernandez I, Halir R, Cheben P, Velasco A V 2020 Opt. Laser Technol. 129 106297
Google Scholar
[24] Cheng Z, Wang J, Yang Z Y, Zhu L N, Yang Y Q, Huang Y Q, Ren X M 2019 Opt. Express 27 34434
Google Scholar
[25] Wang H W, Zhang Y, He Y, Zhu Q M, Sun L, Su Y K 2019 Adv. Opt. Mater. 7 1801191
Google Scholar
[26] Sun L, Hu R, Zhang Z H, He Y, Su Y K 2021 IEEE J. Sel. Top. Quant. 27 8100308
Google Scholar
[27] Luque-González J M, Ortega-Moñux A, Halir R, Schmid J H, Cheben P, Molina-Fernández I, Wangüemert-Pérez J G 2021 Laser Photonics Rev. 15 2000478
Google Scholar
[28] Lu M J, Deng C Y, Sun Y, Wang D Y, Huang L, Liu P C, Lin D D, Cheng W, Hu G H, Lin T, Yun B F, Cui Y P 2022 Opt. Express 30 24655
Google Scholar
[29] Luque-González J M, Herrero-Bermello A, Ortega-Moux A, Sánchez-Rodríguez M, Velasco A V, Schmid J H, Cheben P, Molina-Fernández I, Halir R. 2020 Opt. Lett. 45 3398
Google Scholar
[30] Yao R K, Li H X, Zhang B H, Chen W W, Wang P J, Dai S X, Liu Y X, Li J, Li Y, Fu Q, Dai T G, Yu H, Yang J Y, Pavesi L 2021 J. Lightwave Technol. 39 6253
Google Scholar
[31] Mao S Q, Hu J Z, Zhang H Y, Jiang W F 2022 IEEE J. Quantum Elect. 58 8400106
Google Scholar
-
图 1 (a) 模式转换器方案的结构示意图; (b), (c) BSWG的部分放大图与SEM图
Fig. 1. (a) Schematic of the mode converter; (b), (c) part enlarged view and SEM image of the BSWG.
图 3 (a) TE0-TE1模式转换器的透射光谱; (b) TE0-TE2模式转换器的透射光谱
Fig. 3. (a) Transmission spectra for the TE0-TE1 mode converter; (b) transmission spectra for the TE0-TE2 mode converter.
图 4 (a) 带有TE GC的直波导显微图; (b) TE GC的SEM图; (c) 不同光纤角度下TE耦合光栅的传输光谱
Fig. 4. (a) Microscope image of the reference straight waveguide with TE-type grating couplers; (b) SEM image of TE-type grating coupler; (c) transmission spectra of TE-type grating couplers under different fiber angles.
图 5 (a), (c) TE0-TE1模式转换器的测试方案显微图; (b), (d) TE0-TE2模式转换器的测试方案显微图以及TE1-TE3模式复用器的SEM图
Fig. 5. (a), (c) Microscope images of the measure schemes for TE0-TE1 mode converter; (b), (d) microscope images of the measure schemes for TE0-TE2 mode converter and SEM images of TE1-TE3 multiplexer.
图 6 TE0-TE1模式转换器(a)和TE0-TE2模式转换器(b)的SEM图及其放大的伪彩图
Fig. 6. SEM images and corresponding pseudocolor SEM images of TE0-TE1 mode converter (a) and TE0-TE2 mode converter (b).
图 7 模式转换器的插损和串扰的测试链路图
Fig. 7. Experimental setup for measuring the insertion loss and crosstalk of mode converter.
图 8 测试得到的器件传输谱 (a) TE0-TE1模式转换器; (b) TE0-TE2模式转换器
Fig. 8. Measured transmission spectra: (a) TE0-TE1 mode converter; (b) TE0-TE2 mode converter.
表 1 模式转换器的优化设计参数
Table 1. Optimized design parameters for the mode converter.
模式转换功能 WMMI/μm WO/μm WT2/μm WD/μm LT1/μm LT2/μm Lc/μm $ \overline {\Delta z} $ TE0-TE1 2.10 0.9 0.229 0 3.00 3.2 3.19 0.16 TE0-TE2 2.67 1.4 0.350 0.373 3.21 4.0 4.06 0.14 
下载: 导出CSV
表 2 模式(解)复用器的详细参数
Table 2. Detail parameters for the mode (de) multiplexer.
模式(解)
复用器总线波导
的宽度/μm接入波导
宽度/μm间隔/nm 耦合长度/μm TE1 0.835 0.4 200 15.5 TE2 1.29 0.406 200 21.3 TE3 1.63 0.38 200 18
下载: 导出CSV
-
[1] Luo L W, Ophir N, Chen C P, Gabrielli L H, Poitras C B, Bergmen K, and Lipson M 2014 Nat. Commun. 5 3069
Google Scholar
[2] Li C L, Liu D J, and Dai D X 2019 Nanophotonics 8 227
Google Scholar
[3] Hsu Y, Chuang C Y, Wu X R, Chen G H, Hsu C W, Chang Y C, Chow C W, Chen J, Lai Y C, Yeh C H, Tsang H K 2018 IEEE Photonics Technol. L. 30 1052
Google Scholar
[4] Li H Q, Wang P J, Yang T J, Dai T, Wang G C, Li S Q, Chen W W, Yang J Y 2018 Opt. Laser Technol. 100 7
Google Scholar
[5] Pan T H and Tseng S Y 2015 Opt. Express 23 10405
Google Scholar
[6] Xu Y, Liu L P, Hu X, Dong Y, Zhang B, Ni Y 2022 Opt. Laser Technol. 151 108028
Google Scholar
[7] Ohana D, Levy U 2014 Opt. Express 22 27617
Google Scholar
[8] Gabrielli L H, Liu D, Johnson S G, Lipson M 2012 Nat. Commun. 3 1217
Google Scholar
[9] Xu H N, Shi Y C 2018 Laser Photonics Rev. 12 1700240
Google Scholar
[10] Chang W J, Lu L L Z, Ren X S, Lu L H, Cheng M F, Liu D M, Zhang M M 2018 IEEE Photonics J. 10 4501008
Google Scholar
[11] Garcia-Rodriguez D, Corral J L, Griol A, Llorente R 2017 Opt. Lett. 42 1221
Google Scholar
[12] Chen W W, Wang P J, Yang J Y 2014 IEEE Photonics Technol. L. 26 2043
Google Scholar
[13] Oner B B, Ustun K, Kurt H, Okyay A K, Turhan-Sayan G 2015 Opt. Express 23 3186
Google Scholar
[14] Chack D, Hassan S, Qasim M 2020 Appl. Opt. 59 3652
Google Scholar
[15] Liu L P, Xu Y, Wen L, Dong Y, Zhang B, Ni Y 2019 Appl. Optics 58 9075
Google Scholar
[16] Cheben P, Halir R, Schmid J H, Atwater H A, Smith D R 2018 Nature 560 565
Google Scholar
[17] Luque-González J M, Sánchez-Postigo A, Hadij-ElHouati A, Ortega-Moñux A, Wangüemert-Pérez J G, Schmid J H, Cheben P, Molina-Fernández I, Halir R, 2021 Nanophotonics 10 2765
Google Scholar
[18] Yu Z J, Xu H N, Liu D J, Li H, Shi Y C, Dai D X 2022 J. Lightwave Technol. 40 1784
Google Scholar
[19] Wu F, Liu T T, Long Y, Xiao S Y, Chen G Y 2023 Phys. Rev. B 107 165428
Google Scholar
[20] Mia M B, Jaidye N, Ahmed I, Ahmed S Z, Kim S 2023 Opt. Express 31 4140
Google Scholar
[21] He Y, Zhang Y, Zhu Q M, An S, Cao R Y, Guo X H, Qiu C Y, Su Y K 2018 J. Lightwave Technol. 36 5746
Google Scholar
[22] González-Andrade D, Gonzalo Wanguemert-Perez J, Velasco A V, Ortega-Monux A, Herrero-Bermello A, Molina-Fernandez I, Halir R, Cheben P 2018 IEEE Photonics J. 10 2201010
Google Scholar
[23] González-Andrade D, Dias A, Wanguemert-Perez J G, Ortega-Monux A, Molina-Fernandez I, Halir R, Cheben P, Velasco A V 2020 Opt. Laser Technol. 129 106297
Google Scholar
[24] Cheng Z, Wang J, Yang Z Y, Zhu L N, Yang Y Q, Huang Y Q, Ren X M 2019 Opt. Express 27 34434
Google Scholar
[25] Wang H W, Zhang Y, He Y, Zhu Q M, Sun L, Su Y K 2019 Adv. Opt. Mater. 7 1801191
Google Scholar
[26] Sun L, Hu R, Zhang Z H, He Y, Su Y K 2021 IEEE J. Sel. Top. Quant. 27 8100308
Google Scholar
[27] Luque-González J M, Ortega-Moñux A, Halir R, Schmid J H, Cheben P, Molina-Fernández I, Wangüemert-Pérez J G 2021 Laser Photonics Rev. 15 2000478
Google Scholar
[28] Lu M J, Deng C Y, Sun Y, Wang D Y, Huang L, Liu P C, Lin D D, Cheng W, Hu G H, Lin T, Yun B F, Cui Y P 2022 Opt. Express 30 24655
Google Scholar
[29] Luque-González J M, Herrero-Bermello A, Ortega-Moux A, Sánchez-Rodríguez M, Velasco A V, Schmid J H, Cheben P, Molina-Fernández I, Halir R. 2020 Opt. Lett. 45 3398
Google Scholar
[30] Yao R K, Li H X, Zhang B H, Chen W W, Wang P J, Dai S X, Liu Y X, Li J, Li Y, Fu Q, Dai T G, Yu H, Yang J Y, Pavesi L 2021 J. Lightwave Technol. 39 6253
Google Scholar
[31] Mao S Q, Hu J Z, Zhang H Y, Jiang W F 2022 IEEE J. Quantum Elect. 58 8400106
Google Scholar
下载:
计量
- 文章访问数: 2730
- PDF下载量: 76
- 被引次数: 0
