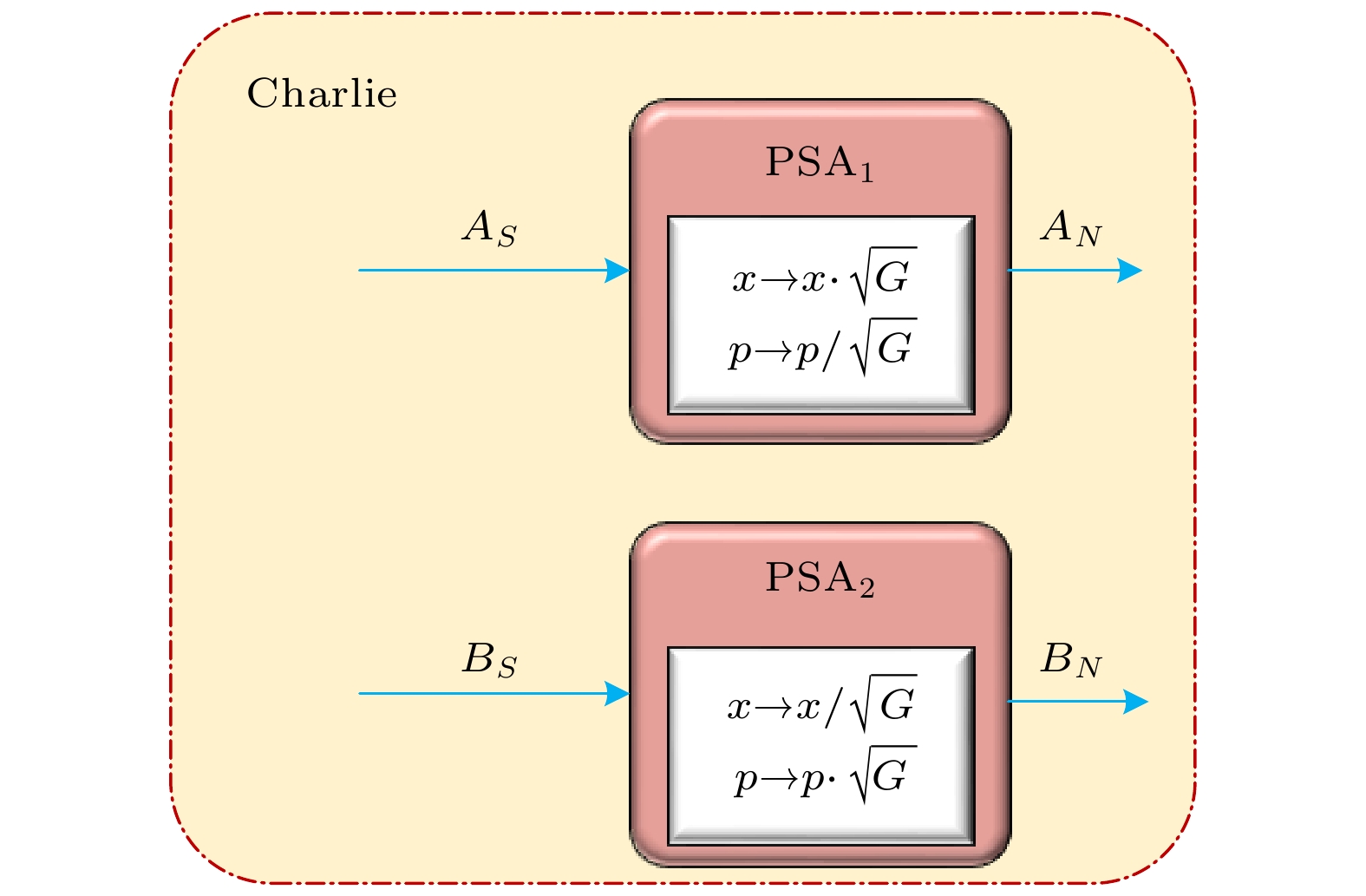
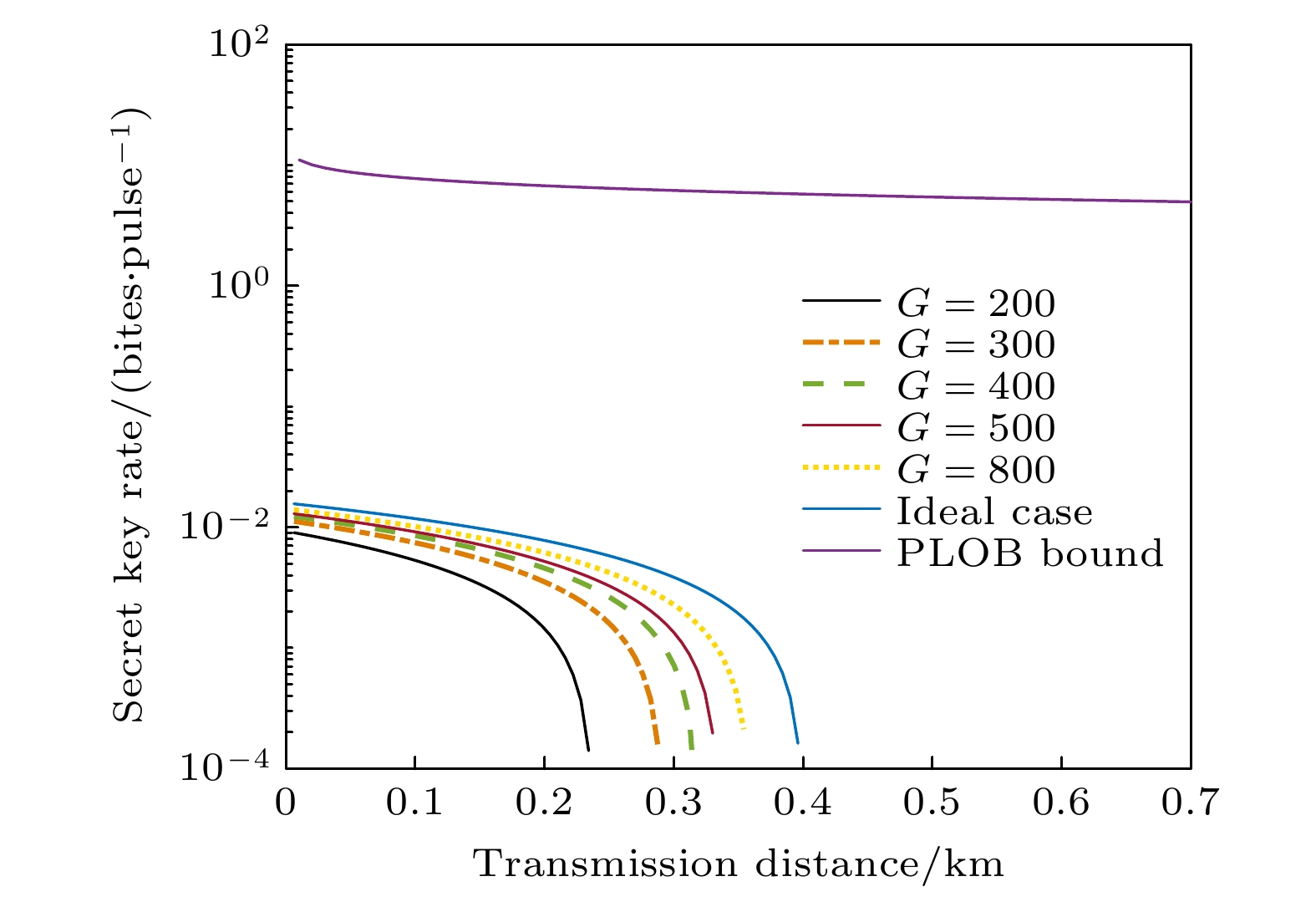
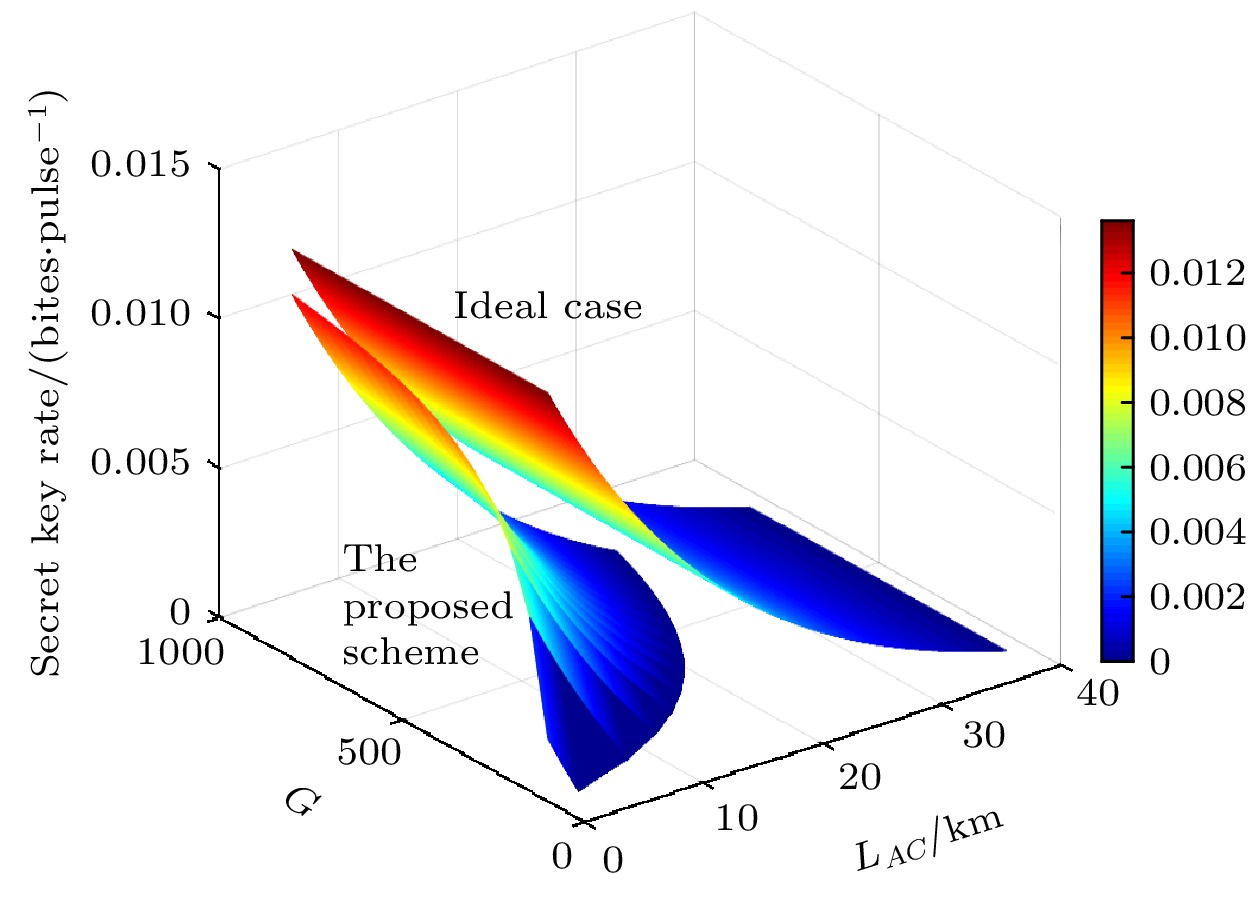
-
由于离散调制连续变量测量设备无关量子密钥分发方案与高效纠错码具有良好的兼容性, 因此即使在低信噪比条件下, 也具备较高的协商效率, 并且其实现条件相比于高斯调制方案更加简单. 然而, 实验中常用的零差探测器的量子效率仅为0.6, 这会严重影响离散调制连续变量测量设备无关量子密钥分发方案的实际应用性能. 鉴于此, 本文提出基于实际探测器补偿的离散调制连续变量测量设备无关量子密钥分发方案, 即在该方案中对两条量子信道的输出端各采用一个相位敏感放大器用于补偿相对应的实际零差探测器. 仿真结果表明采用相位敏感放大器能够很好地补偿实际零差探测器的量子效率, 有效提升基于实际探测器的离散调制连续变量测量设备无关量子密钥分发方案的密钥率和安全传输距离, 为推动离散调制连续变量测量设备无关量子密钥分发方案的实用化发展提供了一个有效而实用的方法.
-
关键词:
- 离散调制 /
- 连续变量 /
- 测量设备无关量子密钥分发 /
- 实际探测器补偿
Discrete modulation continuous variable measurement device independent quantum key distribution scheme has good compatibility with efficient error correction codes, which leads to high reconciliation efficiency even at low signal-to-noise ratio. Besides, the implementation of this protocol is simpler than that of Gaussian modulation scheme. However, the quantum efficiency of homodyne detector commonly used in the experiment is only 0.6, which will seriously affect the practical application performance of discrete modulation continuous variable measurement device independent quantum key distribution scheme. To solve this problem, we propose a discrete modulation continuous variable measurement device independent quantum key distribution scheme based on realistic detector compensation. In our scheme, for the outputs of two quantum channels, each adopts a phase sensitive amplifier to compensate for the corresponding realistic homodyne detector. The simulation results show that the phase sensitive amplifier can well compensate for the quantum efficiency of the realistic detector and effectively improve the performance of the discrete modulation continuous variable measurement device independent quantum key distribution scheme with realistic detector in terms of secret key rate and secure transmission distance. The proposed protocol provides an effective method for promoting the practical development of the discrete modulation continuous variable measurement device independent quantum key distribution scheme.-
Keywords:
- discrete modulation /
- continuous variable /
- measurement device independent quantum key distribution /
- realistic detector compensation
[1] Xu F, Ma X, Zhang Q, Lo H K, Pan J W 2020 Rev. Mod. Phys. 92 025002
 Google Scholar
Google Scholar
[2] Lo H K, Curty M, Tamaki K 2014 Nat. Photonics 8 595
 Google Scholar
Google Scholar
[3] Liu H, Jiang C, Zhu H T, Zou M, Yu Z W, Hu X L, Xu H, Ma S, Han Z, Chen J P, Dai Y, Tang S B, Zhang W, Li H, You L, Wang Z, Hua Y, Hu H, Zhang H, Zhou F, Zhang Q, Wang X B, Chen T Y, Pan J W 2021 Phys. Rev. Lett. 126 250502
 Google Scholar
Google Scholar
[4] Pirandola S, Andersen U L, Banchi L, Berta M, Bunandar D, Colbeck R, Englund D, Gehring T, Lupo C, Ottaviani C, Pereira J L, Razavi M, Shaari J S, Tomamichel M, Usenko V C, Vallone G, Villoresi P, Wallden P 2020 Adv. Opt. Photon. 12 1012
 Google Scholar
Google Scholar
[5] Chen J P, Zhang C, Liu Y, Jiang C, Zhang W J, Han Z Y, Ma S Z, Hu X L, Li Y H, Liu H, Zhou F, Jiang H F, Chen T Y, Li H, You L X, Wang Z, Wang X B, Zhang Q, Pan J W 2021 Nat. Photonics 15 570
 Google Scholar
Google Scholar
[6] Yin J, Li Y H, Liao S K, Yang M, Cao Y, Zhang L, Ren J G, Cai W Q, Liu W Y, Li S L, Shu R, Huang Y M, Deng L, Li L, Zhang Q, Liu N L, Chen Y A, Lu C Y, Wang X B, Xu F H, Wang J Y, Peng C Z, Ekert A K, Pan J W 2020 Nature 582 501
 Google Scholar
Google Scholar
[7] Fang X T, Zeng P, Liu H, Zou M, Wu W J, Tang Y L, Sheng Y J, Xiang Y, Zhang W, Li H, Wang Z, You L, Li M J, Chen H, Chen Y A, Zhang Q, Peng C Z, Ma X, Chen T Y, Pan J W 2020 Nat. Photonics 14 422
 Google Scholar
Google Scholar
[8] Laudenbach F, Pacher C, Fung C H F, Poppe A, Peev M, Schrenk B, Hentschel M, Walther P, Hübel H 2018 Adv. Quantum Technol. 1 1800011
 Google Scholar
Google Scholar
[9] Wu X D, Wang Y J, Zhong H, Liao Q, Guo Y 2019 Front. Phys. 14 41501
 Google Scholar
Google Scholar
[10] 钟海, 叶炜, 吴晓东, 郭迎 2021 70 020301
 Google Scholar
Google Scholar
Zhong H, Ye W, Wu X D, Guo Y 2021 Acta Phys. Sin 70 020301
 Google Scholar
Google Scholar
[11] Wu X, Wang Y, Guo Y, Zhong H, Huang D 2021 Phys. Rev. A 103 032604
 Google Scholar
Google Scholar
[12] Grosshans F, Grangier P 2002 Phys. Rev. Lett. 88 057902
 Google Scholar
Google Scholar
[13] Wang T, Zuo Z, Li L, Huang P, Guo Y, Zeng G 2022 Phys. Rev. Appl. 18 014064
 Google Scholar
Google Scholar
[14] Liu C, Zhu C, Nie M, Yang H, Pei C 2022 Opt. Express 30 14798
 Google Scholar
Google Scholar
[15] Jing F, Liu X, Wang X, Lu Y, Wu T, Li K, Dong C 2022 Opt. Express 30 8075
 Google Scholar
Google Scholar
[16] Sarmiento S, Etcheverry S, Aldama J, López I H, Vidarte L T, Xavier G B, Nolan D A, Stone J S, Li M J, Loeber D, Pruneri V 2022 New J. Phys. 24 063011
 Google Scholar
Google Scholar
[17] García-Patrón R, Cerf N J 2006 Phys. Rev. Lett. 97 190503
 Google Scholar
Google Scholar
[18] Navascués M, Grosshans F, Acín A 2006 Phys. Rev. Lett. 97 190502
 Google Scholar
Google Scholar
[19] Pirandola S, Braunstein S L, Lloyd S 2008 Phys. Rev. Lett. 101 200504
 Google Scholar
Google Scholar
[20] Renner R, Cirac J I 2009 Phys. Rev. Lett. 102 110504
 Google Scholar
Google Scholar
[21] Leverrier A, Grosshans F, Grangier P 2010 Phys. Rev. A 81 062343
 Google Scholar
Google Scholar
[22] Leverrier A 2015 Phys. Rev. Lett. 114 070501
 Google Scholar
Google Scholar
[23] Huang D, Huang P, Lin D, Zeng G 2016 Sci. Rep. 6 19201
 Google Scholar
Google Scholar
[24] Zhang Y, Chen Z, Pirandola S, Wang X, Zhou C, Chu B, Zhao Y, Xu B, Yu S, Guo H 2020 Phys. Rev. Lett. 125 010502
 Google Scholar
Google Scholar
[25] Jouguet P, Kunz-Jacques S, Leverrier A, Grangier P, Diamanti E 2013 Nat. Photonics 7 378
 Google Scholar
Google Scholar
[26] Huang D, Lin D, Wang C, Liu W, Fang S, Peng J, Huang P, Zeng G 2015 Opt. Express 23 17511
 Google Scholar
Google Scholar
[27] Huang D, Huang P, Li H, Wang T, Zhou Y, Zeng G 2016 Opt. Lett. 41 3511
 Google Scholar
Google Scholar
[28] Filip R 2008 Phys. Rev. A 77 022310
 Google Scholar
Google Scholar
[29] Yuan Z L, Dynes J F, Shields A J 2010 Nat. Photonics 4 800
 Google Scholar
Google Scholar
[30] Jouguet P, Kunz-Jacques S, Diamanti E 2013 Phys. Rev. A 87 062313
 Google Scholar
Google Scholar
[31] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 88 022339
 Google Scholar
Google Scholar
[32] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 87 052309
 Google Scholar
Google Scholar
[33] Qin H, Kumar R, Alléaume R 2016 Phys. Rev. A 94 012325
 Google Scholar
Google Scholar
[34] Braunstein S L, Pirandola S 2012 Phys. Rev. Lett. 108 130502
 Google Scholar
Google Scholar
[35] Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503
 Google Scholar
Google Scholar
[36] Wang X B 2013 Phys. Rev. A 87 012320
 Google Scholar
Google Scholar
[37] Xu F, Curty M, Qi B, Lo H K 2013 New J. Phys. 15 113007
 Google Scholar
Google Scholar
[38] Curty M, Xu F, Cui W, Lim C C W, Tamaki K, Lo H K 2014 Nat. Commun. 5 3732
 Google Scholar
Google Scholar
[39] Lupo C, Ottaviani C, Papanastasiou P, Pirandola S 2018 Phys. Rev. Lett. 120 220505
 Google Scholar
Google Scholar
[40] Ferreira da S T, Vitoreti D, Xavier G B, do Amaral G C, Temporao G P, von derWeid J P 2013 Phys. Rev. A 88 052303
 Google Scholar
Google Scholar
[41] Cao Y, Li Y H, Yang K X, Jiang Y F, Li S L, Hu X L, Abulizi M, Li C L, Zhang W, Sun Q C, Liu W Y, Jiang X, Liao S K, Ren J G, Li H, You L, Wang Z, Yin J, Lu C Y, Wang X B, Zhang Q, Peng C Z, Pan J W 2020 Phys. Rev. Lett. 125 260503
 Google Scholar
Google Scholar
[42] Xu F, Qi B, Liao Z, Lo H K 2013 Appl. Phys. Lett. 103 061101
 Google Scholar
Google Scholar
[43] Li Z, Zhang Y C, Xu F, Peng X, Guo H 2014 Phys. Rev. A 89 052301
 Google Scholar
Google Scholar
[44] Ma X C, Sun S H, Jiang M S, Gui M, Liang L M 2014 Phys. Rev. A 89 042335
 Google Scholar
Google Scholar
[45] Zhang Y C, Li Z, Yu S, Gu W, Peng X, Guo H 2014 Phys. Rev. A 90 052325
 Google Scholar
Google Scholar
[46] Wu X D, Wang Y J, Huang D, Guo Y 2020 Front. Phys. 15 31601
 Google Scholar
Google Scholar
[47] Pirandola S, Ottaviani C, Spedalieri G, Weedbrook C, Braunstein S L, Lloyd S, Gehring T, Jacobsen C S, Andersen U L 2015 Nat. Photonics 9 397
 Google Scholar
Google Scholar
[48] Richardson T J, Shokrollahi M A, Urbanke R 2001 IEEE Trans. Inf. Theory 47 619
 Google Scholar
Google Scholar
[49] Leverrier A, Alléaume R, Boutros J, Zémor G, Grangier P 2008 Phys. Rev. A 77 042325
 Google Scholar
Google Scholar
[50] Jouguet P, Kunz-Jacques S, Leverrier A 2011 Phys. Rev. A 84 062317
 Google Scholar
Google Scholar
[51] Milicevic M, Chen F, Zhang L M, Gulak P. G 2018 npj Quantum Inf. 4 21
 Google Scholar
Google Scholar
[52] Ma H X, Huang P, Bai D Y, Wang T, Wang S Y, Bao W S, Zeng G H 2019 Phys. Rev. A 99 022322
 Google Scholar
Google Scholar
[53] Lodewyck J, Bloch M, García-Patrón R, Fossier S, Karpov E, Diamanti E, Debuisschert T, Cerf N J, Tualle-Brouri R, McLaughlin S W, Grangier P 2007 Phys. Rev. A 76 042305
 Google Scholar
Google Scholar
[54] Leverrier A, Grangier P 2009 Phys. Rev. Lett. 102 180504
 Google Scholar
Google Scholar
[55] Polkinghorne R E S, Ralph T C 1999 Phys. Rev. Lett. 83 2095
 Google Scholar
Google Scholar
[56] Pirandola S 2013 New J. Phys. 15 113046
 Google Scholar
Google Scholar
[57] Fossier S, Diamanti E, Debuisschert T, Tualle-Brouri R, Grangier P 2009 J. Phys. B 42 114014
 Google Scholar
Google Scholar
[58] Pirandola S, Laurenza R, Ottaviani C, Banchi L 2017 Nat. Commun. 8 15043
 Google Scholar
Google Scholar
-
-
[1] Xu F, Ma X, Zhang Q, Lo H K, Pan J W 2020 Rev. Mod. Phys. 92 025002
 Google Scholar
Google Scholar
[2] Lo H K, Curty M, Tamaki K 2014 Nat. Photonics 8 595
 Google Scholar
Google Scholar
[3] Liu H, Jiang C, Zhu H T, Zou M, Yu Z W, Hu X L, Xu H, Ma S, Han Z, Chen J P, Dai Y, Tang S B, Zhang W, Li H, You L, Wang Z, Hua Y, Hu H, Zhang H, Zhou F, Zhang Q, Wang X B, Chen T Y, Pan J W 2021 Phys. Rev. Lett. 126 250502
 Google Scholar
Google Scholar
[4] Pirandola S, Andersen U L, Banchi L, Berta M, Bunandar D, Colbeck R, Englund D, Gehring T, Lupo C, Ottaviani C, Pereira J L, Razavi M, Shaari J S, Tomamichel M, Usenko V C, Vallone G, Villoresi P, Wallden P 2020 Adv. Opt. Photon. 12 1012
 Google Scholar
Google Scholar
[5] Chen J P, Zhang C, Liu Y, Jiang C, Zhang W J, Han Z Y, Ma S Z, Hu X L, Li Y H, Liu H, Zhou F, Jiang H F, Chen T Y, Li H, You L X, Wang Z, Wang X B, Zhang Q, Pan J W 2021 Nat. Photonics 15 570
 Google Scholar
Google Scholar
[6] Yin J, Li Y H, Liao S K, Yang M, Cao Y, Zhang L, Ren J G, Cai W Q, Liu W Y, Li S L, Shu R, Huang Y M, Deng L, Li L, Zhang Q, Liu N L, Chen Y A, Lu C Y, Wang X B, Xu F H, Wang J Y, Peng C Z, Ekert A K, Pan J W 2020 Nature 582 501
 Google Scholar
Google Scholar
[7] Fang X T, Zeng P, Liu H, Zou M, Wu W J, Tang Y L, Sheng Y J, Xiang Y, Zhang W, Li H, Wang Z, You L, Li M J, Chen H, Chen Y A, Zhang Q, Peng C Z, Ma X, Chen T Y, Pan J W 2020 Nat. Photonics 14 422
 Google Scholar
Google Scholar
[8] Laudenbach F, Pacher C, Fung C H F, Poppe A, Peev M, Schrenk B, Hentschel M, Walther P, Hübel H 2018 Adv. Quantum Technol. 1 1800011
 Google Scholar
Google Scholar
[9] Wu X D, Wang Y J, Zhong H, Liao Q, Guo Y 2019 Front. Phys. 14 41501
 Google Scholar
Google Scholar
[10] 钟海, 叶炜, 吴晓东, 郭迎 2021 70 020301
 Google Scholar
Google Scholar
Zhong H, Ye W, Wu X D, Guo Y 2021 Acta Phys. Sin 70 020301
 Google Scholar
Google Scholar
[11] Wu X, Wang Y, Guo Y, Zhong H, Huang D 2021 Phys. Rev. A 103 032604
 Google Scholar
Google Scholar
[12] Grosshans F, Grangier P 2002 Phys. Rev. Lett. 88 057902
 Google Scholar
Google Scholar
[13] Wang T, Zuo Z, Li L, Huang P, Guo Y, Zeng G 2022 Phys. Rev. Appl. 18 014064
 Google Scholar
Google Scholar
[14] Liu C, Zhu C, Nie M, Yang H, Pei C 2022 Opt. Express 30 14798
 Google Scholar
Google Scholar
[15] Jing F, Liu X, Wang X, Lu Y, Wu T, Li K, Dong C 2022 Opt. Express 30 8075
 Google Scholar
Google Scholar
[16] Sarmiento S, Etcheverry S, Aldama J, López I H, Vidarte L T, Xavier G B, Nolan D A, Stone J S, Li M J, Loeber D, Pruneri V 2022 New J. Phys. 24 063011
 Google Scholar
Google Scholar
[17] García-Patrón R, Cerf N J 2006 Phys. Rev. Lett. 97 190503
 Google Scholar
Google Scholar
[18] Navascués M, Grosshans F, Acín A 2006 Phys. Rev. Lett. 97 190502
 Google Scholar
Google Scholar
[19] Pirandola S, Braunstein S L, Lloyd S 2008 Phys. Rev. Lett. 101 200504
 Google Scholar
Google Scholar
[20] Renner R, Cirac J I 2009 Phys. Rev. Lett. 102 110504
 Google Scholar
Google Scholar
[21] Leverrier A, Grosshans F, Grangier P 2010 Phys. Rev. A 81 062343
 Google Scholar
Google Scholar
[22] Leverrier A 2015 Phys. Rev. Lett. 114 070501
 Google Scholar
Google Scholar
[23] Huang D, Huang P, Lin D, Zeng G 2016 Sci. Rep. 6 19201
 Google Scholar
Google Scholar
[24] Zhang Y, Chen Z, Pirandola S, Wang X, Zhou C, Chu B, Zhao Y, Xu B, Yu S, Guo H 2020 Phys. Rev. Lett. 125 010502
 Google Scholar
Google Scholar
[25] Jouguet P, Kunz-Jacques S, Leverrier A, Grangier P, Diamanti E 2013 Nat. Photonics 7 378
 Google Scholar
Google Scholar
[26] Huang D, Lin D, Wang C, Liu W, Fang S, Peng J, Huang P, Zeng G 2015 Opt. Express 23 17511
 Google Scholar
Google Scholar
[27] Huang D, Huang P, Li H, Wang T, Zhou Y, Zeng G 2016 Opt. Lett. 41 3511
 Google Scholar
Google Scholar
[28] Filip R 2008 Phys. Rev. A 77 022310
 Google Scholar
Google Scholar
[29] Yuan Z L, Dynes J F, Shields A J 2010 Nat. Photonics 4 800
 Google Scholar
Google Scholar
[30] Jouguet P, Kunz-Jacques S, Diamanti E 2013 Phys. Rev. A 87 062313
 Google Scholar
Google Scholar
[31] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 88 022339
 Google Scholar
Google Scholar
[32] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 87 052309
 Google Scholar
Google Scholar
[33] Qin H, Kumar R, Alléaume R 2016 Phys. Rev. A 94 012325
 Google Scholar
Google Scholar
[34] Braunstein S L, Pirandola S 2012 Phys. Rev. Lett. 108 130502
 Google Scholar
Google Scholar
[35] Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503
 Google Scholar
Google Scholar
[36] Wang X B 2013 Phys. Rev. A 87 012320
 Google Scholar
Google Scholar
[37] Xu F, Curty M, Qi B, Lo H K 2013 New J. Phys. 15 113007
 Google Scholar
Google Scholar
[38] Curty M, Xu F, Cui W, Lim C C W, Tamaki K, Lo H K 2014 Nat. Commun. 5 3732
 Google Scholar
Google Scholar
[39] Lupo C, Ottaviani C, Papanastasiou P, Pirandola S 2018 Phys. Rev. Lett. 120 220505
 Google Scholar
Google Scholar
[40] Ferreira da S T, Vitoreti D, Xavier G B, do Amaral G C, Temporao G P, von derWeid J P 2013 Phys. Rev. A 88 052303
 Google Scholar
Google Scholar
[41] Cao Y, Li Y H, Yang K X, Jiang Y F, Li S L, Hu X L, Abulizi M, Li C L, Zhang W, Sun Q C, Liu W Y, Jiang X, Liao S K, Ren J G, Li H, You L, Wang Z, Yin J, Lu C Y, Wang X B, Zhang Q, Peng C Z, Pan J W 2020 Phys. Rev. Lett. 125 260503
 Google Scholar
Google Scholar
[42] Xu F, Qi B, Liao Z, Lo H K 2013 Appl. Phys. Lett. 103 061101
 Google Scholar
Google Scholar
[43] Li Z, Zhang Y C, Xu F, Peng X, Guo H 2014 Phys. Rev. A 89 052301
 Google Scholar
Google Scholar
[44] Ma X C, Sun S H, Jiang M S, Gui M, Liang L M 2014 Phys. Rev. A 89 042335
 Google Scholar
Google Scholar
[45] Zhang Y C, Li Z, Yu S, Gu W, Peng X, Guo H 2014 Phys. Rev. A 90 052325
 Google Scholar
Google Scholar
[46] Wu X D, Wang Y J, Huang D, Guo Y 2020 Front. Phys. 15 31601
 Google Scholar
Google Scholar
[47] Pirandola S, Ottaviani C, Spedalieri G, Weedbrook C, Braunstein S L, Lloyd S, Gehring T, Jacobsen C S, Andersen U L 2015 Nat. Photonics 9 397
 Google Scholar
Google Scholar
[48] Richardson T J, Shokrollahi M A, Urbanke R 2001 IEEE Trans. Inf. Theory 47 619
 Google Scholar
Google Scholar
[49] Leverrier A, Alléaume R, Boutros J, Zémor G, Grangier P 2008 Phys. Rev. A 77 042325
 Google Scholar
Google Scholar
[50] Jouguet P, Kunz-Jacques S, Leverrier A 2011 Phys. Rev. A 84 062317
 Google Scholar
Google Scholar
[51] Milicevic M, Chen F, Zhang L M, Gulak P. G 2018 npj Quantum Inf. 4 21
 Google Scholar
Google Scholar
[52] Ma H X, Huang P, Bai D Y, Wang T, Wang S Y, Bao W S, Zeng G H 2019 Phys. Rev. A 99 022322
 Google Scholar
Google Scholar
[53] Lodewyck J, Bloch M, García-Patrón R, Fossier S, Karpov E, Diamanti E, Debuisschert T, Cerf N J, Tualle-Brouri R, McLaughlin S W, Grangier P 2007 Phys. Rev. A 76 042305
 Google Scholar
Google Scholar
[54] Leverrier A, Grangier P 2009 Phys. Rev. Lett. 102 180504
 Google Scholar
Google Scholar
[55] Polkinghorne R E S, Ralph T C 1999 Phys. Rev. Lett. 83 2095
 Google Scholar
Google Scholar
[56] Pirandola S 2013 New J. Phys. 15 113046
 Google Scholar
Google Scholar
[57] Fossier S, Diamanti E, Debuisschert T, Tualle-Brouri R, Grangier P 2009 J. Phys. B 42 114014
 Google Scholar
Google Scholar
[58] Pirandola S, Laurenza R, Ottaviani C, Banchi L 2017 Nat. Commun. 8 15043
 Google Scholar
Google Scholar
计量
- 文章访问数: 5908
- PDF下载量: 110
- 被引次数: 0
















 下载:
下载: