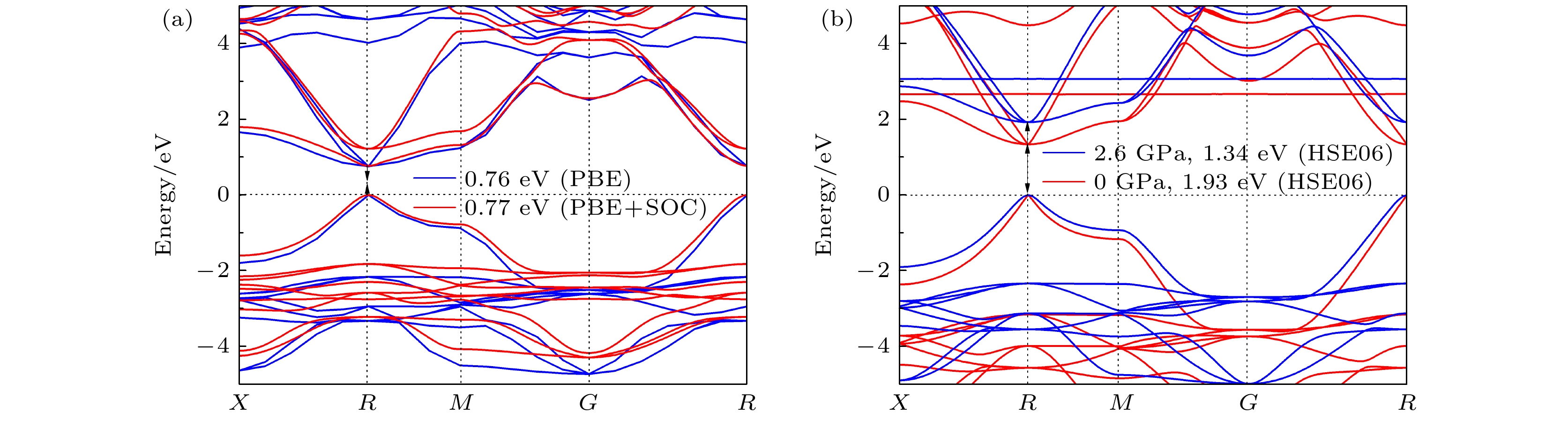
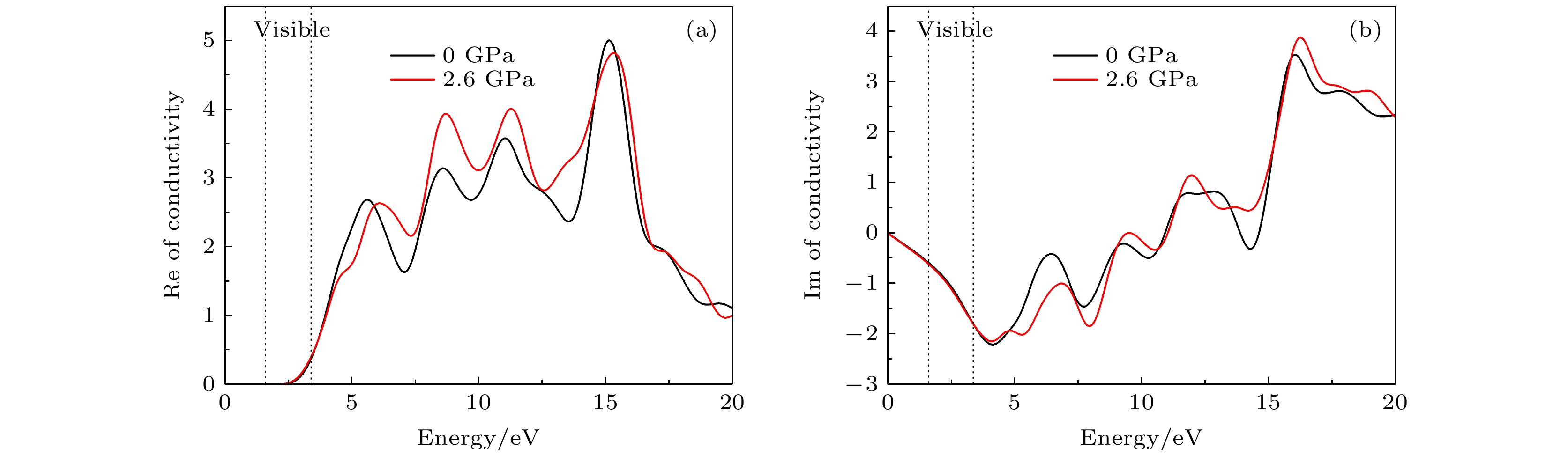
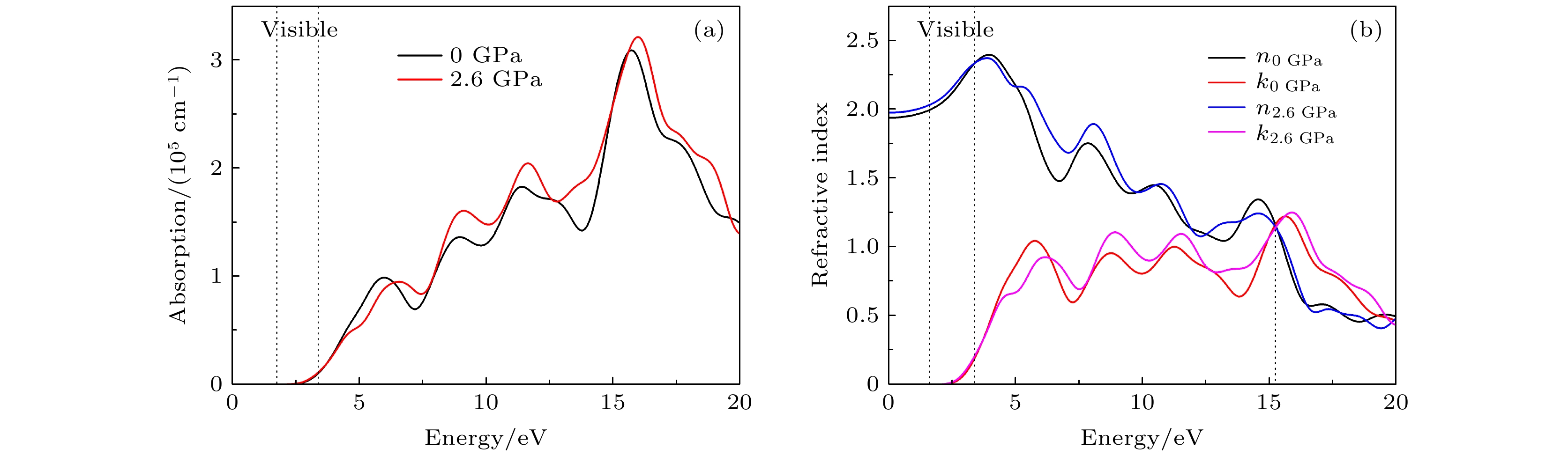
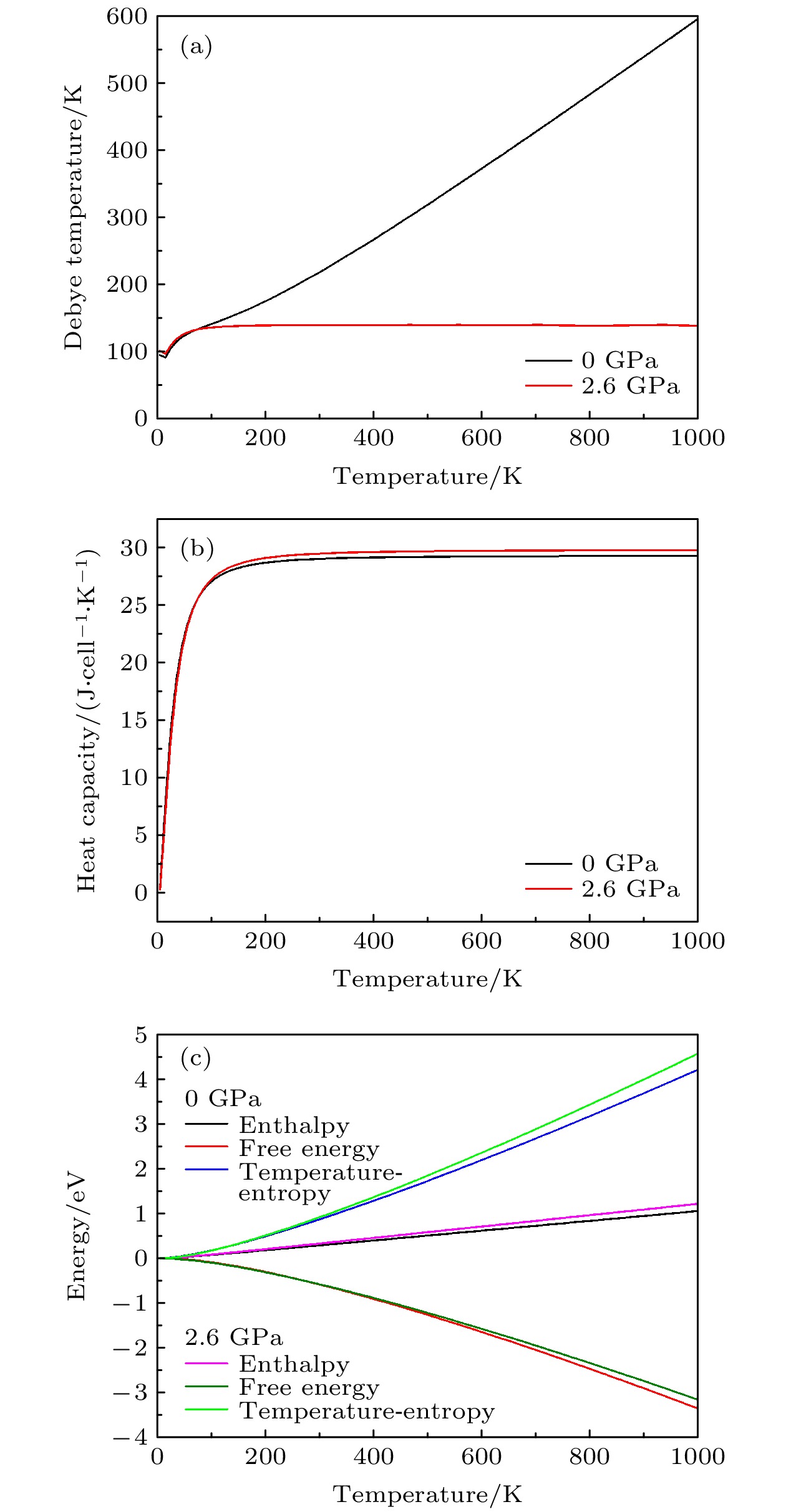
-
CsSnBr3作为一种重要的钙钛矿太阳能电池材料已经被广泛研究. 基于密度泛函理论, 利用第一性原理来研究在不同静水压力下CsSnBr3的光电性质. 结果发现, 当压力为2.6 GPa时, CsSnBr3具有最佳光学带隙值1.34 eV, 因此, 本文只对比研究CsSnBr3在0和2.6 GPa静水压力下的光电性质. 当压力为2.6 GPa时, CsSnBr3具有更大的介电值、电导率、吸收系数及折射率, 吸收光谱有红移现象, 且其电子和空穴的有效质量、激子的结合能都比较小, 这表明CsSnBr3是一种高效的光吸收材料. 通过Born-Huang 稳定标准判据, 容差因子T和声子谱有无虚频的三重计算, 发现在压力0和2.6 GPa下, CsSnBr3都是稳定的. 由加压前后CsSnBr3的弹性模量值可知他们都是偏软性的, 具有良好的延展性和各向异性. 加压后CsSnBr3的德拜温度和热容量很快趋于稳定, 与温度无关; 而焓和熵则随着温度升高而增加, 增加的幅度大于未加压的情况; 吉布斯自由能都呈现出降低的趋势, 未加压时降低得稍快. 本研究表明, 施加静水压力后的CsSnBr3是一种良好的光电材料, 适合用于钙钛矿太阳能电池.
-
关键词:
- 密度泛函理论 /
- CsSnBr3钙钛矿 /
- 静水压力 /
- 光电性质
As an important perovskite solar cell (PSC) material, CsSnBr3 has been widely studied. Based on the density functional theory (DFT), the photoelectric properties of CsSnBr3 are studied by using the first-principles at different hydrostatic pressures. It is found that CsSnBr3 has an optimal optical band gap value of 1.34 eV under a pressure of 2.6 GPa, so only the photoelectric properties of CsSnBr3 under the hydrostatic pressure of 0 GPa and 2.6 GPa are studied, respectively. When the pressure is 2.6 GPa, CsSnBr3 has larger values of dielectric, conductivity, absorption coefficient and refractive index, the red-shifted absorption spectrum, and relatively small effective mass of electron and hole and exciton binding energy, indicating that CsSnBr3 is an efficient light absorbing material. According to the triple calculations of Born-Huang stability standard criterion, the tolerance factor T and phonon spectrum with or without virtual frequency, it is found that CsSnBr3 is stable under the pressure of 0 GPa and 2.6 GPa. According to the elastic modulus value of CsSnBr3 before and after pressure, it can be seen that the CsSnBr3 is soft, with good ductility and anisotropy. The Debye temperature and heat capacity of CsSnBr3, soon after it has been pressured, tend to be stable and are independent of temperature. The enthalpy and entropy increase with temperature increasing, and the increased amplitude is larger than those of the unpressured CsSnBr3. Gibbs free energy shows a decreasing trend, and the decrease is slightly faster when unpressured. This study shows that CsSnBr3 is a good photoelectric material after having been pressured hydrostatically, which is suitable for perovskite solar cells.-
Keywords:
- density functional theory /
- CsSnBr3 perovskite /
- hydrostatic pressure /
- photoelectric properties
[1] Kojima A, Teshima K, Shirai Y, Miyasaka T 2009 J. Am. Chem. Soc. 131 6050
Google Scholar
[2] Kim H S, Lee C R, Im J H, Lee K B, Moehl T, Marchioro A, Moon S J, Humphry-Baker R, Yum J H, Moser J E, Gratzel M, Park N G 2012 Sci. Rep. 2 591
Google Scholar
[3] Lee M M, Teuscher J, Miyasaka T, Murakami T N, Snaith H J 2012 Science 338 643
Google Scholar
[4] Snaith H J 2013 J. Phys. Chem. Lett. 4 3623
Google Scholar
[5] Katan C, Mercier N, Even J 2019 Chem. Rev. 119 3140
Google Scholar
[6] Tan Z K, Moghaddam R S, Lai M L, Docampo P, Higler R, Deschler F, Price M, Sadhanala A, Pazos L M, Credgington D, Hanusch F, Bein T, Snaith H J, Friend R H 2014 Nat. Nanotechnol. 9 687
Google Scholar
[7] Jeon N J, Na H, Jung E H, Yang T Y, Lee Y G, Kim G, Shin H W, Seok S I, Lee J, Seo J 2018 Nat. Energy 3 682
Google Scholar
[8] Ehli C, Oelsner C, Guldi D M, Mateo-Alonso A, Prato M, Schmidt C, Backes C, Hauke F, Hirsch A 2009 Nat. Chem. 1 243
Google Scholar
[9] Piao Y M, Meany B, Powell L R, Valley N, Kwon H, Schatz G C, Wang Y H 2013 Nat. Chem. 5 840
Google Scholar
[10] Williams S T, Rajagopal A, Chueh C C, Jen A K Y 2016 J. Phys. Chem. Lett. 7 811
Google Scholar
[11] Boix P P, Agarwala S, Koh T M, Mathews N, Mhaisalkar S G 2015 J. Phys. Chem. Lett. 6 898
Google Scholar
[12] Saliba M, Matsui T, Domanski K, Seo J Y, Ummadisingu A, Zakeeruddin S M, Correa-Baena J P, Tress W R, Abate A, Hagfeldt A, Gratzel M 2016 Science 354 206
Google Scholar
[13] Kieslich G, Sun S J, Cheetham A K 2014 Chem. Sci. 5 4712
Google Scholar
[14] Sutton R J, Filip M R, Haghighirad A A, Sakai N, Wenger B, Giustino F, Snaith H J 2018 ACS Energy Lett. 3 1787
Google Scholar
[15] Wang K, Jin Z W, Liang L, Bian H, Bai D L, Wang H R, Zhang J R, Wang Q, Liu S Z 2018 Nat. Commun. 9 1
Google Scholar
[16] Sanehira E M, Marshall A R, Christians J A, Harvey S P, Ciesielski P N, Wheeler L M, Schulz P, Lin L Y, Beard M C, Luther J M 2017 Sci. Adv. 3 eaao4204
Google Scholar
[17] Perdew J P, Ruzsinszky A 2018 Eur. Phys. J. B 91 6
Google Scholar
[18] Cheng X R, Kuang X Y, Cheng H, Tian H, Yang S M, Yu M, Dou X L, Mao A J 2020 RSC Adv. 10 12432
Google Scholar
[19] Peedikakkandy L, Bhargava P 2016 RSC Adv. 6 19857
Google Scholar
[20] Ou T J, Yan J J, Xiao C H, Shen W S, Liu C L, L iu, X Z, Han Y H, Ma Y Z, Gao C X 2016 Nanoscale 8 11426
Google Scholar
[21] Jaffe A, Lin Y, Umeyama D, Beavers C, Voss J, Mao W, Karunadasa H 2017 ACS Energy Lett. 253 1549
Google Scholar
[22] Schwarz U, Wagner F, Syassen K, Hillebrecht H 1996 Phys. Rev. B 53 19
Google Scholar
[23] Gupta N, Thiele G, Seo D K, Whangbo M H, Hillebrecht H 1998 Inorg. Chem. 37 407
Google Scholar
[24] Jing H J, Sa RJ, Xu G 2019 Chem. Phys. Lett. 732 136642
Google Scholar
[25] Coduri M, Strobel T A, Szafranski M, Katrusiak A, Mahata A, Cova F, Bonomi S, Mosconi E, De Angelis F, Malavasi L 2019 J. Phys. Chem. Lett. 10 7398
Google Scholar
[26] Yalameha S, Saeidi P, Nourbakhsh Z, Vaez A, Ramazani A 2020 J. Appl. Phys. 127 085102
[27] Blöchl P E, Jepsen O, Andersen O K 1994 Phys. Rev. B 49 16223
Google Scholar
[28] Kohn W, Sham L J 1965 Phys. Rev. A 140 A1133
Google Scholar
[29] Segall M D, Lindan P J D, Probert M J, Pickard C J, Hasnip P J, Clark S J, Payne M C 2002 J. Phys. Condens. Matter 14 2717
Google Scholar
[30] Shockley W, Queisser H J 1961 J. Appl. Phys. 32 510
[31] Lang L, Yang J H, Liu H R, Xiang H J, Gong X G 2014 Phys. Lett. A 378 290
Google Scholar
[32] Qian J Y, Xu B, Tian W J 2016 Org. Electron. 37 61
Google Scholar
[33] Jung M C, Raga S R, Qi Y B 2016 RSC Adv. 6 2819
Google Scholar
[34] Gajdoš M, Hummer K, Kresse G, Furthmüller J, Bechstedt F 2006 Phys. Rev. B 73 045112
Google Scholar
[35] Sahin S, Ciftci Y O, Colakoglu K, Korozlu N 2012 J. Alloys Compd. 529 1
Google Scholar
[36] Saha S, Sinha T P, Mookerjee A 2000 Phys. Rev. B 62 13
Google Scholar
[37] Rodina A V, Dietrich M, Göldner A, Eckey L, Meyer B K 2001 Phys. Rev. B 64 115204
Google Scholar
[38] Manser J S, Christians J A, Kamat P V 2016 Chem. Rev. 116 12956
Google Scholar
[39] Galkowski K, Mitioglu A, Miyata A, Plochocka P, Portugall O, Eperon G E, Wang J T W, Stergiopoulos T, Stranks S D, Snaith H J, Nicholas R J 2016 Energy Environ. Sci. 9 962
Google Scholar
[40] De Wolf S, Holovsky J, Moon S J, Loper P, Niesen B, Ledinsky M, Haug F J, Yum J H, Ballif C 2014 J. Phys. Chem. Lett. 5 1035
Google Scholar
[41] Li B, Long R, Xia Y, Mi Q 2018 Angew. Chem. 57 13154
Google Scholar
[42] Born M 1955 Am. J. Phys. 23 474
Google Scholar
[43] Goldschmidt V M 1926 Naturwissenschaften 14 477
Google Scholar
[44] Li C H, Lu X G, Ding W Z, Feng L M, Gao Y H, Guo Z G 2008 Acta. Crystallogr., Sect. B 64 702
Google Scholar
[45] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[46] Ranganathan S I, Ostoja-Starzewski M 2008 J. Mech. Phys. Solids 56 2773
-
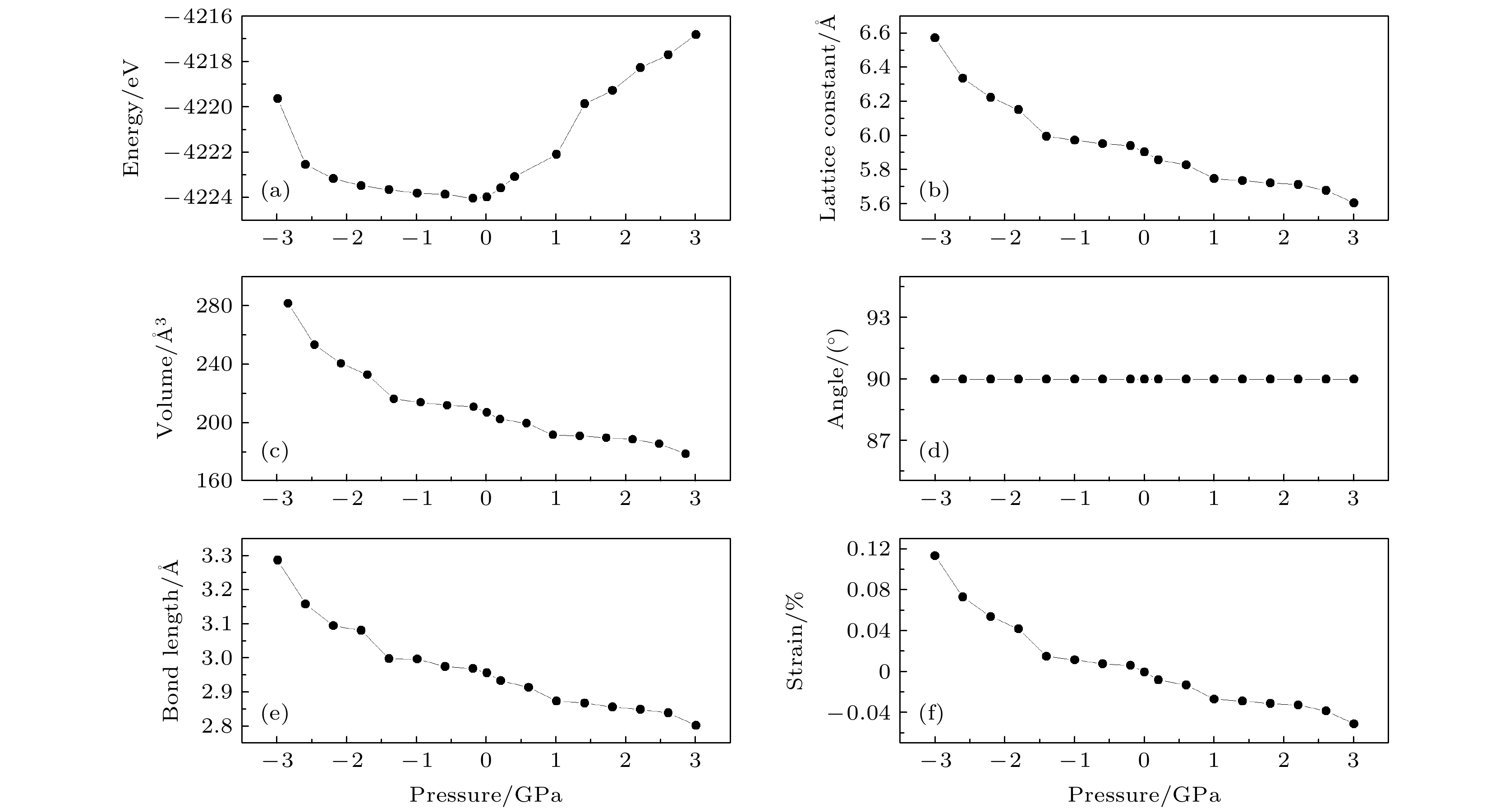
图 2 CsSnBr3在不同压力下的结构参数 (a)能量曲线; (b)晶格常数曲线; (c)体积曲线; (d)晶胞角曲线; (e) Sn—Br键长曲线; (f)应变曲线
Fig. 2. Structure parameters of CsSnBr3 under different pressure conditions: (a) Curve of energy; (b) curve of the lattice constant (c) curve of volume; (d) curve of cell angle; (e) curve of bond length of Sn—Br; (f) curve of the strain.
图 3 CsSnBr3在不同压力下的能带值
Fig. 3. Band gap of CsSnBr3 under different pressure conditions.
图 4 CsSnBr3的能带结构 (a)采用PBE和PBE + SOC计算得到的能带; (b) 0和2.6 GPa压力下采用HSE06计算得到的能带
Fig. 4. Band structures of CsSnBr3: (a) Band structure calculated by PBE and PBE + SOC; (b) band structure calculated by HSE06 at the pressure of 0 and 2.6 GPa.
图 5 CsSnBr3在 (a) 0 GPa和(b) 2.6 GPa压力下的态密度
Fig. 5. Density of states (DOS) of CsSnBr3 under the pressure of (a) 0 GPa and (b) 2.6 GPa.
图 7 在0和2.6 GPa压力下CsSnBr3介电函数的(a)实部和(b)虚部
Fig. 7. Dielectric function of CsSnBr3 of (a) real and (b) imaginary under the pressure of 0 and 2.6 GPa.
图 8 在0和2.6 GPa压力下CsSnBr3电导率的(a)实部和(b)虚部
Fig. 8. Conductivity of CsSnBr3 of (a) real and (b) imaginary under the pressure of 0 and 2.6 GPa.
图 9 (a) CsSnBr3在0和2.6 GPa压力下的吸收系数; (b) CsSnBr3在0和2.6 GPa压下的折射率n和消光系数k
Fig. 9. (a) Absorption of CsSnBr3 under the pressure of 0 and 2.6 GPa; (b) refractive index n and extinction coefficient k of CsSnBr3 under the pressure of 0 and 2.6 GPa.
图 10 CsSnBr3在(a) 0 GPa和(b) 2.6 GPa压力下的声子谱
Fig. 10. Phonon spectrum of CsSnBr3 under the pressure of (a) 0 GPa and (b) 2.6 GPa.
图 11 在0和2.6 GPa压力下CsSnBr3的热力学性质 (a)德拜温度; (b)热容量; (c)焓、温度-熵和吉布斯自由能
Fig. 11. Thermodynamic properties of CsSnBr3 of (a) Debye temperature, (b) heat capacity and (c) enthalpy, temperature-entropy and free energy under the pressure of 0 and 2.6 GPa.
表 1 Findit找到的CsSnBr3的晶格参数与几何优化后的对比
Table 1. Lattice parameters of CsSnBr3 with Findit compared with geometry optimization (GO).
a = b = c/Å α = β = γ/(°) V/Å3 Space group Findit 5.80 90.00 195.11 $Pm\bar3m$ GO 5.94 90.00 209.58 $ Pm\bar 3m $ 
下载: 导出CSV
表 2 在0和2.6 GPa压力下CsSnBr3的有效质量和激子结合能(质量的单位是自由电子的质量m0)
Table 2. Effective masses and exciton binding energy calculated for CsSnBr3 under the pressure of 0 and 2.6 GPa. Masses are given in units of the free electron mass m0.
Pressure/GPa me (R→X) me (R→M) me(R→G) ${\bar m_{\rm{e}}}$ mh (R→X) mh (R→M) mh (R→G) ${\bar m_{\rm{h}}}$ εs Eb /meV 0 0.523 0.524 0.184 0.410 0.072 0.075 0.072 0.073 3.8 58 2.6 0.418 0.418 0.143 0.326 0.052 0.063 0.051 0.055 3.9 42
下载: 导出CSV
表 3 在0和2.6 GPa压力下CsSnBr3的弹性常数、体积模量(B)、剪切模量(G)和弹性各向异性(A)
Table 3. Calculated elastic constant, bulk modulus (B), shear modulus (G) and elastic anisotropy (A) of CsSnBr3 under the pressure of 0 and 2.6 GPa.
Pressure/GPa C11 C12 C44 B G B/G A 0 37.40 6.32 5.21 16.68 8.22 2.03 0.34 2.6 67.36 11.56 5.20 30.17 10.99 2.73 0.19
下载: 导出CSV
表 4 CsSnBr3的各元素的离子半径
Table 4. Ionic radium of CsSnBr3.
Cs+ Sn2+ Br– T R/nm 0.167 0.112 0.196 0.83
下载: 导出CSV
-
[1] Kojima A, Teshima K, Shirai Y, Miyasaka T 2009 J. Am. Chem. Soc. 131 6050
Google Scholar
[2] Kim H S, Lee C R, Im J H, Lee K B, Moehl T, Marchioro A, Moon S J, Humphry-Baker R, Yum J H, Moser J E, Gratzel M, Park N G 2012 Sci. Rep. 2 591
Google Scholar
[3] Lee M M, Teuscher J, Miyasaka T, Murakami T N, Snaith H J 2012 Science 338 643
Google Scholar
[4] Snaith H J 2013 J. Phys. Chem. Lett. 4 3623
Google Scholar
[5] Katan C, Mercier N, Even J 2019 Chem. Rev. 119 3140
Google Scholar
[6] Tan Z K, Moghaddam R S, Lai M L, Docampo P, Higler R, Deschler F, Price M, Sadhanala A, Pazos L M, Credgington D, Hanusch F, Bein T, Snaith H J, Friend R H 2014 Nat. Nanotechnol. 9 687
Google Scholar
[7] Jeon N J, Na H, Jung E H, Yang T Y, Lee Y G, Kim G, Shin H W, Seok S I, Lee J, Seo J 2018 Nat. Energy 3 682
Google Scholar
[8] Ehli C, Oelsner C, Guldi D M, Mateo-Alonso A, Prato M, Schmidt C, Backes C, Hauke F, Hirsch A 2009 Nat. Chem. 1 243
Google Scholar
[9] Piao Y M, Meany B, Powell L R, Valley N, Kwon H, Schatz G C, Wang Y H 2013 Nat. Chem. 5 840
Google Scholar
[10] Williams S T, Rajagopal A, Chueh C C, Jen A K Y 2016 J. Phys. Chem. Lett. 7 811
Google Scholar
[11] Boix P P, Agarwala S, Koh T M, Mathews N, Mhaisalkar S G 2015 J. Phys. Chem. Lett. 6 898
Google Scholar
[12] Saliba M, Matsui T, Domanski K, Seo J Y, Ummadisingu A, Zakeeruddin S M, Correa-Baena J P, Tress W R, Abate A, Hagfeldt A, Gratzel M 2016 Science 354 206
Google Scholar
[13] Kieslich G, Sun S J, Cheetham A K 2014 Chem. Sci. 5 4712
Google Scholar
[14] Sutton R J, Filip M R, Haghighirad A A, Sakai N, Wenger B, Giustino F, Snaith H J 2018 ACS Energy Lett. 3 1787
Google Scholar
[15] Wang K, Jin Z W, Liang L, Bian H, Bai D L, Wang H R, Zhang J R, Wang Q, Liu S Z 2018 Nat. Commun. 9 1
Google Scholar
[16] Sanehira E M, Marshall A R, Christians J A, Harvey S P, Ciesielski P N, Wheeler L M, Schulz P, Lin L Y, Beard M C, Luther J M 2017 Sci. Adv. 3 eaao4204
Google Scholar
[17] Perdew J P, Ruzsinszky A 2018 Eur. Phys. J. B 91 6
Google Scholar
[18] Cheng X R, Kuang X Y, Cheng H, Tian H, Yang S M, Yu M, Dou X L, Mao A J 2020 RSC Adv. 10 12432
Google Scholar
[19] Peedikakkandy L, Bhargava P 2016 RSC Adv. 6 19857
Google Scholar
[20] Ou T J, Yan J J, Xiao C H, Shen W S, Liu C L, L iu, X Z, Han Y H, Ma Y Z, Gao C X 2016 Nanoscale 8 11426
Google Scholar
[21] Jaffe A, Lin Y, Umeyama D, Beavers C, Voss J, Mao W, Karunadasa H 2017 ACS Energy Lett. 253 1549
Google Scholar
[22] Schwarz U, Wagner F, Syassen K, Hillebrecht H 1996 Phys. Rev. B 53 19
Google Scholar
[23] Gupta N, Thiele G, Seo D K, Whangbo M H, Hillebrecht H 1998 Inorg. Chem. 37 407
Google Scholar
[24] Jing H J, Sa RJ, Xu G 2019 Chem. Phys. Lett. 732 136642
Google Scholar
[25] Coduri M, Strobel T A, Szafranski M, Katrusiak A, Mahata A, Cova F, Bonomi S, Mosconi E, De Angelis F, Malavasi L 2019 J. Phys. Chem. Lett. 10 7398
Google Scholar
[26] Yalameha S, Saeidi P, Nourbakhsh Z, Vaez A, Ramazani A 2020 J. Appl. Phys. 127 085102
[27] Blöchl P E, Jepsen O, Andersen O K 1994 Phys. Rev. B 49 16223
Google Scholar
[28] Kohn W, Sham L J 1965 Phys. Rev. A 140 A1133
Google Scholar
[29] Segall M D, Lindan P J D, Probert M J, Pickard C J, Hasnip P J, Clark S J, Payne M C 2002 J. Phys. Condens. Matter 14 2717
Google Scholar
[30] Shockley W, Queisser H J 1961 J. Appl. Phys. 32 510
[31] Lang L, Yang J H, Liu H R, Xiang H J, Gong X G 2014 Phys. Lett. A 378 290
Google Scholar
[32] Qian J Y, Xu B, Tian W J 2016 Org. Electron. 37 61
Google Scholar
[33] Jung M C, Raga S R, Qi Y B 2016 RSC Adv. 6 2819
Google Scholar
[34] Gajdoš M, Hummer K, Kresse G, Furthmüller J, Bechstedt F 2006 Phys. Rev. B 73 045112
Google Scholar
[35] Sahin S, Ciftci Y O, Colakoglu K, Korozlu N 2012 J. Alloys Compd. 529 1
Google Scholar
[36] Saha S, Sinha T P, Mookerjee A 2000 Phys. Rev. B 62 13
Google Scholar
[37] Rodina A V, Dietrich M, Göldner A, Eckey L, Meyer B K 2001 Phys. Rev. B 64 115204
Google Scholar
[38] Manser J S, Christians J A, Kamat P V 2016 Chem. Rev. 116 12956
Google Scholar
[39] Galkowski K, Mitioglu A, Miyata A, Plochocka P, Portugall O, Eperon G E, Wang J T W, Stergiopoulos T, Stranks S D, Snaith H J, Nicholas R J 2016 Energy Environ. Sci. 9 962
Google Scholar
[40] De Wolf S, Holovsky J, Moon S J, Loper P, Niesen B, Ledinsky M, Haug F J, Yum J H, Ballif C 2014 J. Phys. Chem. Lett. 5 1035
Google Scholar
[41] Li B, Long R, Xia Y, Mi Q 2018 Angew. Chem. 57 13154
Google Scholar
[42] Born M 1955 Am. J. Phys. 23 474
Google Scholar
[43] Goldschmidt V M 1926 Naturwissenschaften 14 477
Google Scholar
[44] Li C H, Lu X G, Ding W Z, Feng L M, Gao Y H, Guo Z G 2008 Acta. Crystallogr., Sect. B 64 702
Google Scholar
[45] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[46] Ranganathan S I, Ostoja-Starzewski M 2008 J. Mech. Phys. Solids 56 2773
下载:
计量
- 文章访问数: 5669
- PDF下载量: 137
- 被引次数: 0
