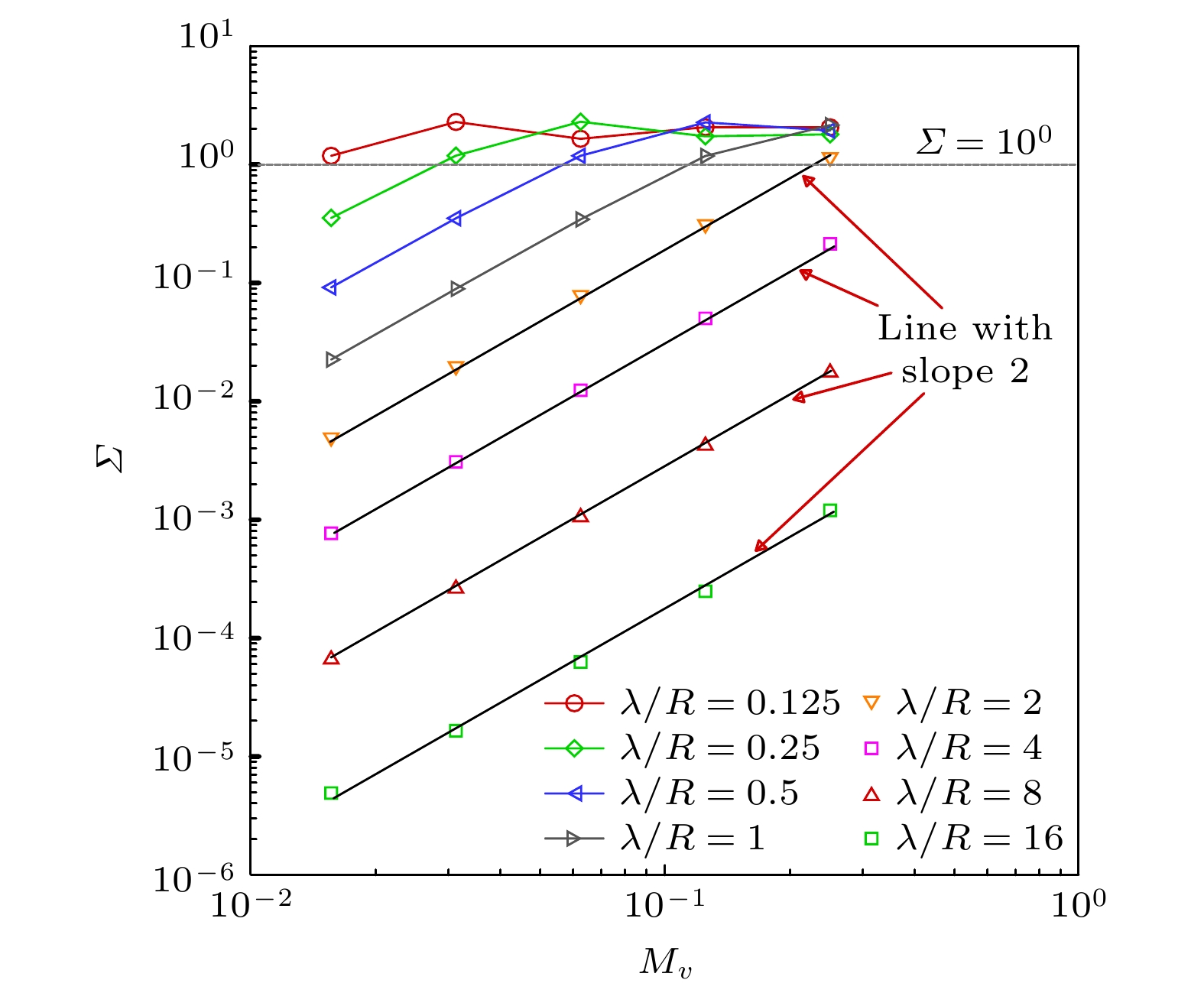
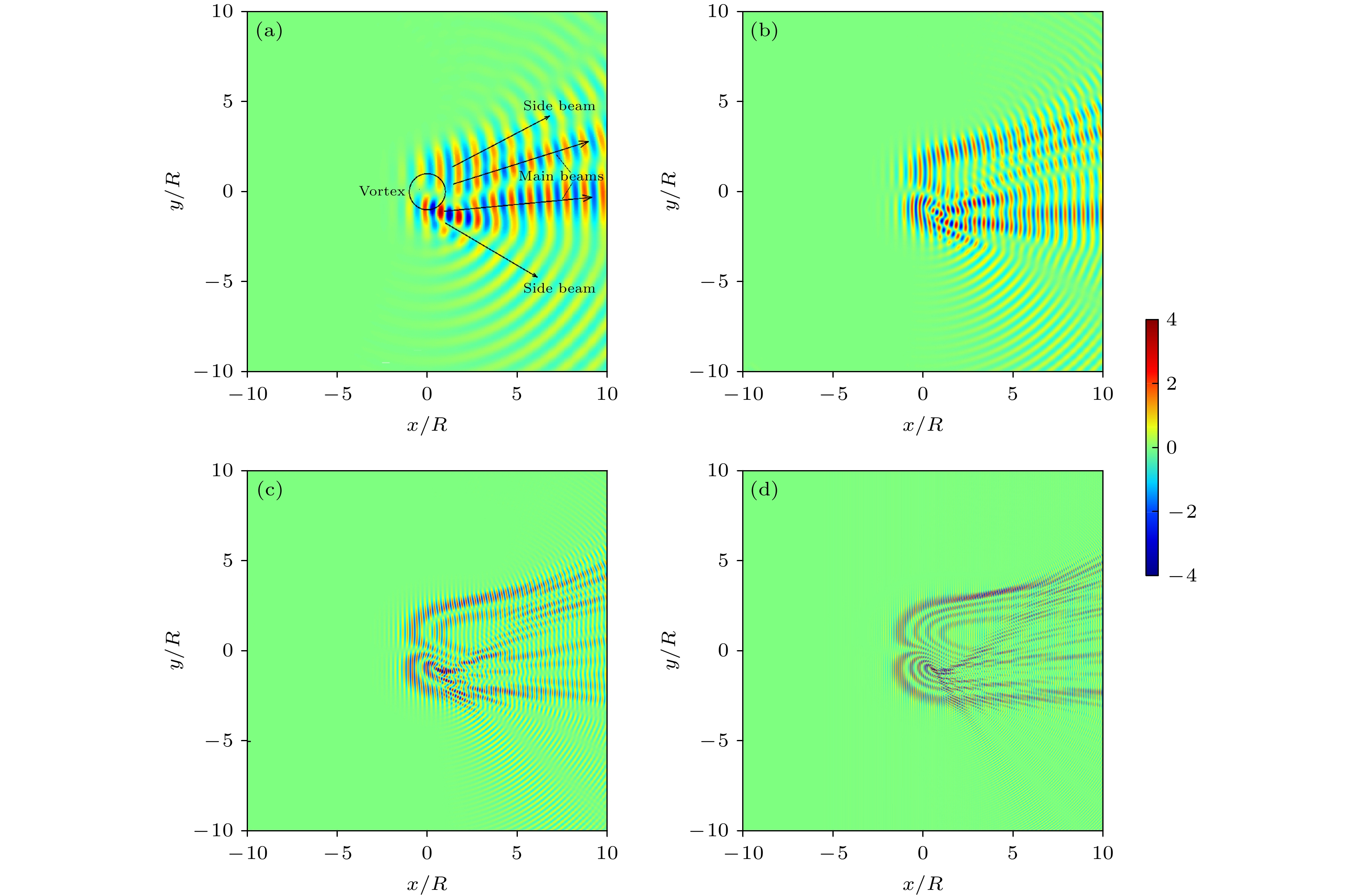
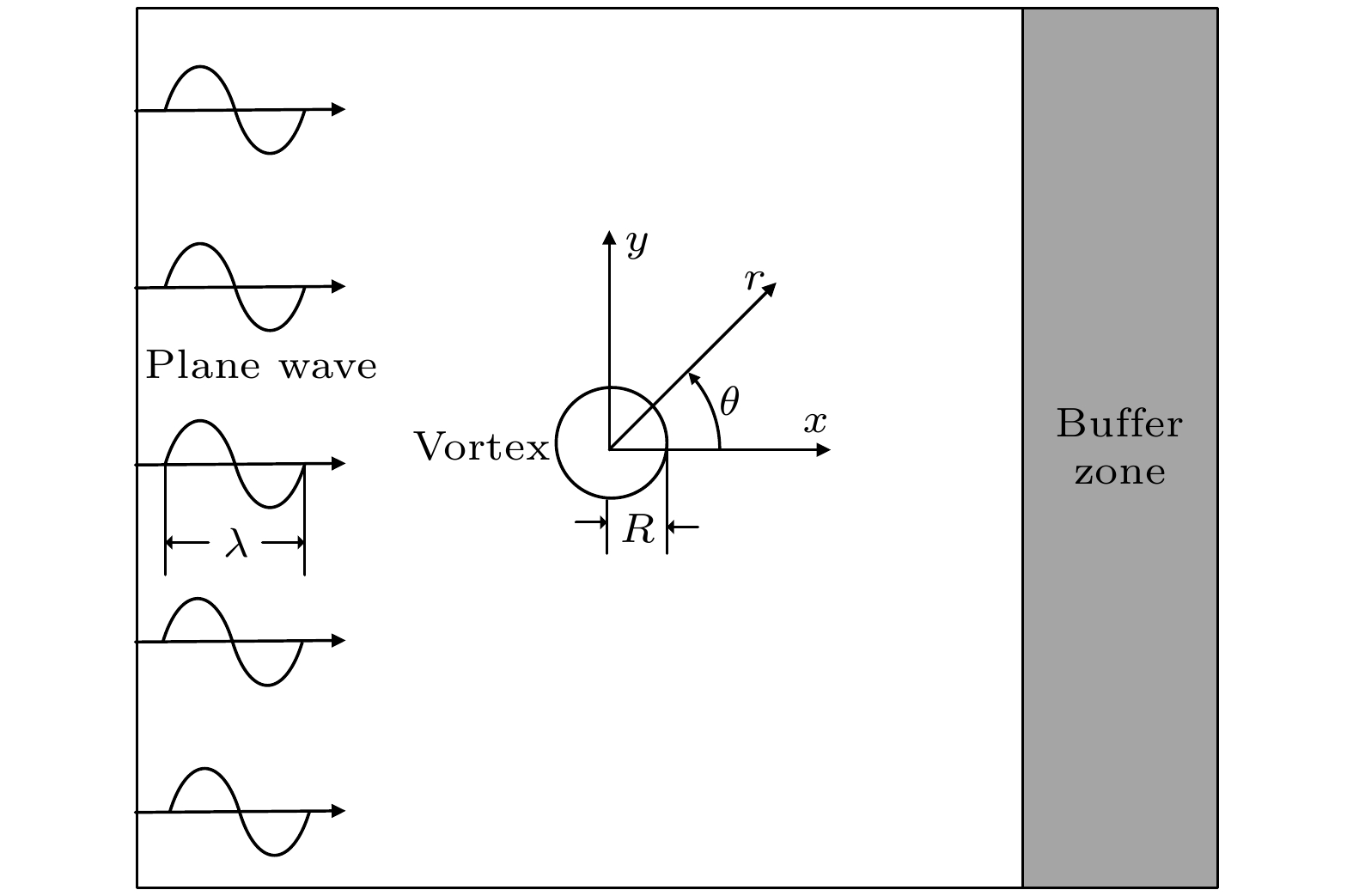
-
以声波为主要表现形式的膨胀过程和以旋涡为主要表现形式的剪切过程之间的非线性耦合问题一直以来都是流体力学的研究热点. 尤其是旋涡对声波的散射问题, 具有重要的科学意义与工程应用背景. 本文通过线性紧致格式直接数值求解二维欧拉方程, 获得了平面声波穿过均熵Taylor涡的散射特性. 与之前经典文献中的标准算例比较, 结果极其吻合, 直接验证了研究所采用的高精度高分辨率空间差分和时间推进格式以及远场无反射边界条件(缓冲区)的计算方法在时域同时解析动力学量和声学量(量级远远小于动力学量)的有效性. 通过引入散射截面, 将全区域的散射分为长波近似区、共振散射区和几何声学区. 针对每个子区域, 重点分析了无量纲尺度量旋涡强度和长度尺度比对散射声场的影响, 给出了散射声场关于上述两个关键无量纲参数的尺度律关系, 并且得到了极低马赫数极大波长时散射声场的分布函数. 在此基础上给出了关于旋涡声散射物理机制的一种解释.When acoustic waves propagate through a volume of vortical flows, the strong nonlinear scattering lead the amplitude, the frequency, and the phase of the incident waves to change obviously. As one of the most significant problems in the area of aeroacoustics, the scattering of acoustic waves by a vortical flow plays a main role in industrial applications and scientific research. In this study, we start from an elementary vortex model. The scattering of plane acoustic waves from a Taylor vortex is investigated by solving two-dimensional Euler equations numerically in the time domain. To resolve the small-amplitude acoustic waves, a sixth-order-accurate compact Padé scheme is used for spatial derivatives and a fourth-order-accurate Runge-Kutta scheme is used to advance the solution in time. To minimize the reflection of outgoing waves, a buffer zone is used at the computational boundary. The computations of scattered fields with very small amplitudes are found to be in excellent agreement with a benchmark provided by previous studies. Simulations for the scattering from a Taylor vortex reveal that the amplitude of the scattered fields is strongly influenced by two dimensionless quantities: the vortex strength
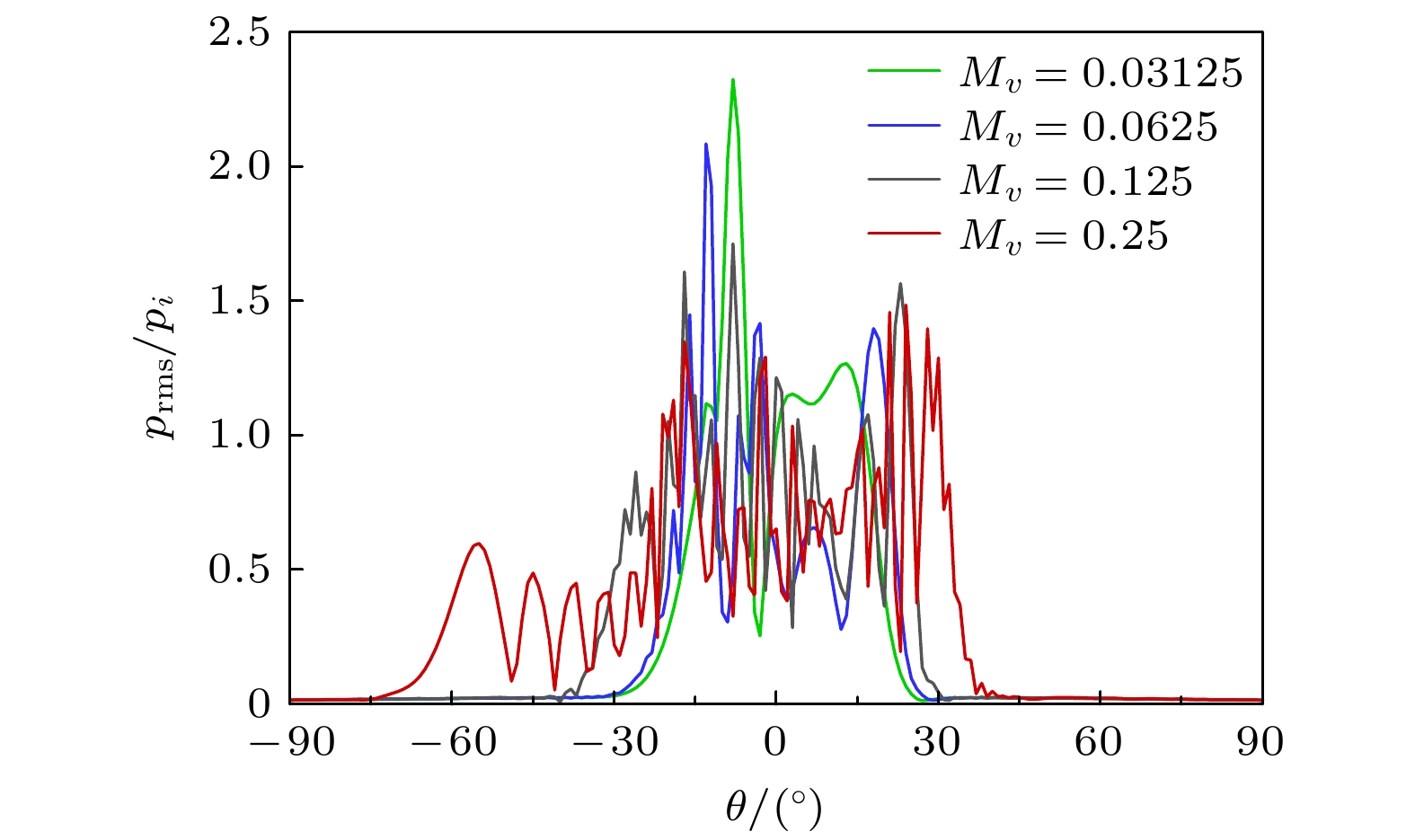
${M_v}$ and the length-scale ratio$\lambda /R$ . Based on a global analysis of scale effects of these two dimensionless quantities on the scattering cross-section, the whole scattering domain defined on the${M_v} - \lambda /R$ plane is divided into three subdomains. As the vortex strength${M_v}$ increases and the length-scale ratio$\lambda /R$ decreases, the acoustic scattering from a compact vortex goes through the long-wavelength domain, the resonance domain, and the geometrical acoustics domain in turn. The associated scattered fields with the increasing of intensity show more irregularities. The scattering in the long-wavelength domain possesses four primary beams described by half-sine functions, which scales as${M_v}{\left( {\lambda /R} \right)^{ - 2}}$ . In particular, the directivity of the scattered field with a very low Mach number and a very long wavelength behaves as${M_v}{\left( {\lambda /R} \right)^{ - 2}}\left| {\sin \left( {\theta /2} \right)} \right|$ . In the resonance domain, the beams in the opposite direction to the incident waves decay rapidly. The rest of two beams follow the${M_v}$ scaling. The scattered fields are concentrated around the direction of the incident wave in the geometrical acoustics domain, where the primary beams are surrounded by several small sub-beams. The physical mechanism of the acoustic scattering caused by a vortex involves two different mechanisms, namely nonlinear scattering effect and linear long-range refraction effect.-
Keywords:
- acoustic scattering /
- vortex strength /
- length-scale ratio /
- scaling laws
[1] Ferziger J H 1974 J. Acoust. Soc. Am. 56 1705
Google Scholar
[2] Lund F, Rojas C 1989 Physica D 37 508
Google Scholar
[3] 武从海, 马瑞轩, 王益民, 张树海 2020 空气动力学学报 38 1120
Wu C, Ma R, Wang Y, Zhang S 2020 Acta Aerodyn. Sin. 38 1120
[4] 李秀坤, 孟祥夏, 夏峙 2015 64 064302
Google Scholar
Li X K, Meng X X, Xia Z 2015 Acta Phys. Sin. 64 064302
Google Scholar
[5] 夏峙, 李秀坤 2015 64 094302
Google Scholar
Xia Z, Li X K 2015 Acta Phys. Sin. 64 094302
Google Scholar
[6] 杨阳, 李秀坤 2016 65 164301
Google Scholar
Yang Y, Li X K 2016 Acta Phys. Sin. 65 164301
Google Scholar
[7] Labbe R, Pinton J F 1998 Phys. Rev. Lett. 81 1413
Google Scholar
[8] Oljaca M, Gu X, Glezer A, Baffico M, Lund F 1998 Phys. Fluids 10 886
Google Scholar
[9] Powell A 1964 J. Acoust. Soc. Am. 36 177
Google Scholar
[10] Kraichnan R H 1953 J. Acoust. Soc. Am. 25 1096
Google Scholar
[11] Lighthill M J 1953 Proc. Cambridge Philos. Soc. 49 531
Google Scholar
[12] Amiet R K 1978 J. Sound Vib. 58 467
Google Scholar
[13] Obukhov A M 1941 Dokl. Akad. Nauk SSSR 30 616
[14] Howe M S 1973 J. Sound Vib. 27 455
Google Scholar
[15] Clifford S F, Brown E H 1974 J. Acoust. Soc. Am. 55 929
Google Scholar
[16] Ostashev V E, Salomons E M, Clifford S F, Lataitis R J, Wilson D K, Blanc-Benon P, Juva D 2001 J. Acoust. Soc. Am. 109 1894
Google Scholar
[17] Dallois L, Blanc-Benon P, Juvé D 2001 J. Comput. Acoust. 9 477
Google Scholar
[18] Fetter A L 1964 Phys. Rev. A 136 1488
Google Scholar
[19] Oshea S 1975 J. Sound Vib. 43 109
Google Scholar
[20] Colonius T, LELE S, Moin P 1991 29 th Aerospace Sciences Meeting Reno, United States, January 7–10, 1991 p494
[21] Colonius T, Lele S K, Moin P 1994 J. Fluid Mech. 260 271
Google Scholar
[22] Ford R, Smith S G L 1999 J. Fluid Mech. 386 305
Google Scholar
[23] Llewellyn Smith S G, Ford R 2001 Phys. Fluids 13 2876
Google Scholar
[24] Llewellyn Smith S G, Ford R 2001 Phys. Fluids 13 2890
Google Scholar
[25] Hattori Y, Llewellyn Smith S G 2002 J. Fluid Mech. 473 275
Google Scholar
[26] Howe M S 1999 J. Sound Vib. 227 1003
Google Scholar
[27] Kopiev V F, Belyaev I V 2010 J. Sound Vib. 329 1409
Google Scholar
[28] Candel S M 1979 J. Fluid Mech. 90 465
Google Scholar
[29] Clair V, Gabard G 2018 J. Fluid Mech. 841 50
Google Scholar
[30] Bodony D J 2006 J. Comput. Phys. 212 681
Google Scholar
[31] Mani A 2012 J. Comput. Phys. 231 704
Google Scholar
[32] Ke G, Li W, Zheng Z C 2015 21st AIAA/CEAS Aeroacoustics Conference Dallas, United States, June 22–26, 2015 p3267
-
图 3 不同长度尺度比下散射截面与旋涡强度的关系(对数坐标系)
Fig. 3. Scattering cross-section
$\varSigma $ potted against${M_v}$ at different$\lambda /R$ (Logarithmic coordinate system).
图 4 不同旋涡强度下散射截面与长度尺度比的关系(对数坐标系)
Fig. 4. Scattering cross-section
$\Sigma $ plotted against$\lambda /R$ at different${M_v}$ (Logarithmic coordinate system).
图 5 旋涡强度和长度尺度比共同对散射截面的影响(对数坐标系)
Fig. 5. Scattering cross-section as a function of
${M_v}$ and$\lambda /R$ (Logarithmic coordinate system).
图 6 t = 280时的散射声压, 其中
${M_v} \!=\! 0.125$ ,$\lambda /R \!=\!10$ Fig. 6. Snapshot of scattered pressure with
${M_v} = 0.125$ and$\lambda /R = 10$ at t = 280.
图 7 长波近似区的散射声压均方根指向性分布
Fig. 7. Directivity for root-mean-square pressure of the scattered fields in long-wavelength domain.
图 8 低马赫数大波长下的散射声压均方根指向性分布
Fig. 8. Directivity for root-mean-square pressure of the scattered fields with low Mach number and long wavelength.
图 9 共振散射区较大波长散射声场指向性分布
Fig. 9. Directivity for root-mean-square pressure of the scattered fields in resonance domain with relatively long wavelength.
图 10 t = 140时的散射声压, 其中
${M_v} \!=\! 0.125$ ,$\lambda /R \!=\! 4$ Fig. 10. Snapshot of scattered pressure with
${M_v} = 0.125$ and$\lambda /R = 4$ at t = 140
图 11 旋涡强度对共振散射区散射声场指向性的影响
Fig. 11. Directivity for root-mean-square pressure of the scattered fields in resonance domain at different vortex strength.
图 12 t = 60时的散射声压, 其中
${M_v} = 0.125$ ,$\lambda /R = 1$ Fig. 12. Snapshot of scattered pressure with
${M_v} = 0.125$ and$\lambda /R = 1$ at t = 60.
图 13 旋涡强度对共振散射区小波长散射声场指向性的影响
Fig. 13. Directivity for root-mean-square pressure of the scattered fields in resonance domain with relatively small wavelength at different vortex strength.
图 14 散射声压, 其中旋涡强度
${M_v} = 0.25$ (a)$\lambda /R = 1$ ; (b)$\lambda /R = 0.5$ ; (c)$\lambda /R = 0.25$ ; (d)$\lambda /R = 0.125$ Fig. 14. Snapshot of scattered pressure with
${M_v} = 0.25$ : (a)$\lambda /R = 1$ ; (b)$\lambda /R = 0.5$ ; (c)$\lambda /R = 0.25$ ; (d)$\lambda /R = 0.125$ .
图 15 不同旋涡强度对几何声学区散射声场指向性的影响
Fig. 15. Directivity for root-mean-square pressure of the scattered field in geometrical acoustics domain at different vortex strength.
-
[1] Ferziger J H 1974 J. Acoust. Soc. Am. 56 1705
Google Scholar
[2] Lund F, Rojas C 1989 Physica D 37 508
Google Scholar
[3] 武从海, 马瑞轩, 王益民, 张树海 2020 空气动力学学报 38 1120
Wu C, Ma R, Wang Y, Zhang S 2020 Acta Aerodyn. Sin. 38 1120
[4] 李秀坤, 孟祥夏, 夏峙 2015 64 064302
Google Scholar
Li X K, Meng X X, Xia Z 2015 Acta Phys. Sin. 64 064302
Google Scholar
[5] 夏峙, 李秀坤 2015 64 094302
Google Scholar
Xia Z, Li X K 2015 Acta Phys. Sin. 64 094302
Google Scholar
[6] 杨阳, 李秀坤 2016 65 164301
Google Scholar
Yang Y, Li X K 2016 Acta Phys. Sin. 65 164301
Google Scholar
[7] Labbe R, Pinton J F 1998 Phys. Rev. Lett. 81 1413
Google Scholar
[8] Oljaca M, Gu X, Glezer A, Baffico M, Lund F 1998 Phys. Fluids 10 886
Google Scholar
[9] Powell A 1964 J. Acoust. Soc. Am. 36 177
Google Scholar
[10] Kraichnan R H 1953 J. Acoust. Soc. Am. 25 1096
Google Scholar
[11] Lighthill M J 1953 Proc. Cambridge Philos. Soc. 49 531
Google Scholar
[12] Amiet R K 1978 J. Sound Vib. 58 467
Google Scholar
[13] Obukhov A M 1941 Dokl. Akad. Nauk SSSR 30 616
[14] Howe M S 1973 J. Sound Vib. 27 455
Google Scholar
[15] Clifford S F, Brown E H 1974 J. Acoust. Soc. Am. 55 929
Google Scholar
[16] Ostashev V E, Salomons E M, Clifford S F, Lataitis R J, Wilson D K, Blanc-Benon P, Juva D 2001 J. Acoust. Soc. Am. 109 1894
Google Scholar
[17] Dallois L, Blanc-Benon P, Juvé D 2001 J. Comput. Acoust. 9 477
Google Scholar
[18] Fetter A L 1964 Phys. Rev. A 136 1488
Google Scholar
[19] Oshea S 1975 J. Sound Vib. 43 109
Google Scholar
[20] Colonius T, LELE S, Moin P 1991 29 th Aerospace Sciences Meeting Reno, United States, January 7–10, 1991 p494
[21] Colonius T, Lele S K, Moin P 1994 J. Fluid Mech. 260 271
Google Scholar
[22] Ford R, Smith S G L 1999 J. Fluid Mech. 386 305
Google Scholar
[23] Llewellyn Smith S G, Ford R 2001 Phys. Fluids 13 2876
Google Scholar
[24] Llewellyn Smith S G, Ford R 2001 Phys. Fluids 13 2890
Google Scholar
[25] Hattori Y, Llewellyn Smith S G 2002 J. Fluid Mech. 473 275
Google Scholar
[26] Howe M S 1999 J. Sound Vib. 227 1003
Google Scholar
[27] Kopiev V F, Belyaev I V 2010 J. Sound Vib. 329 1409
Google Scholar
[28] Candel S M 1979 J. Fluid Mech. 90 465
Google Scholar
[29] Clair V, Gabard G 2018 J. Fluid Mech. 841 50
Google Scholar
[30] Bodony D J 2006 J. Comput. Phys. 212 681
Google Scholar
[31] Mani A 2012 J. Comput. Phys. 231 704
Google Scholar
[32] Ke G, Li W, Zheng Z C 2015 21st AIAA/CEAS Aeroacoustics Conference Dallas, United States, June 22–26, 2015 p3267
下载:
计量
- 文章访问数: 4634
- PDF下载量: 80
- 被引次数: 0
