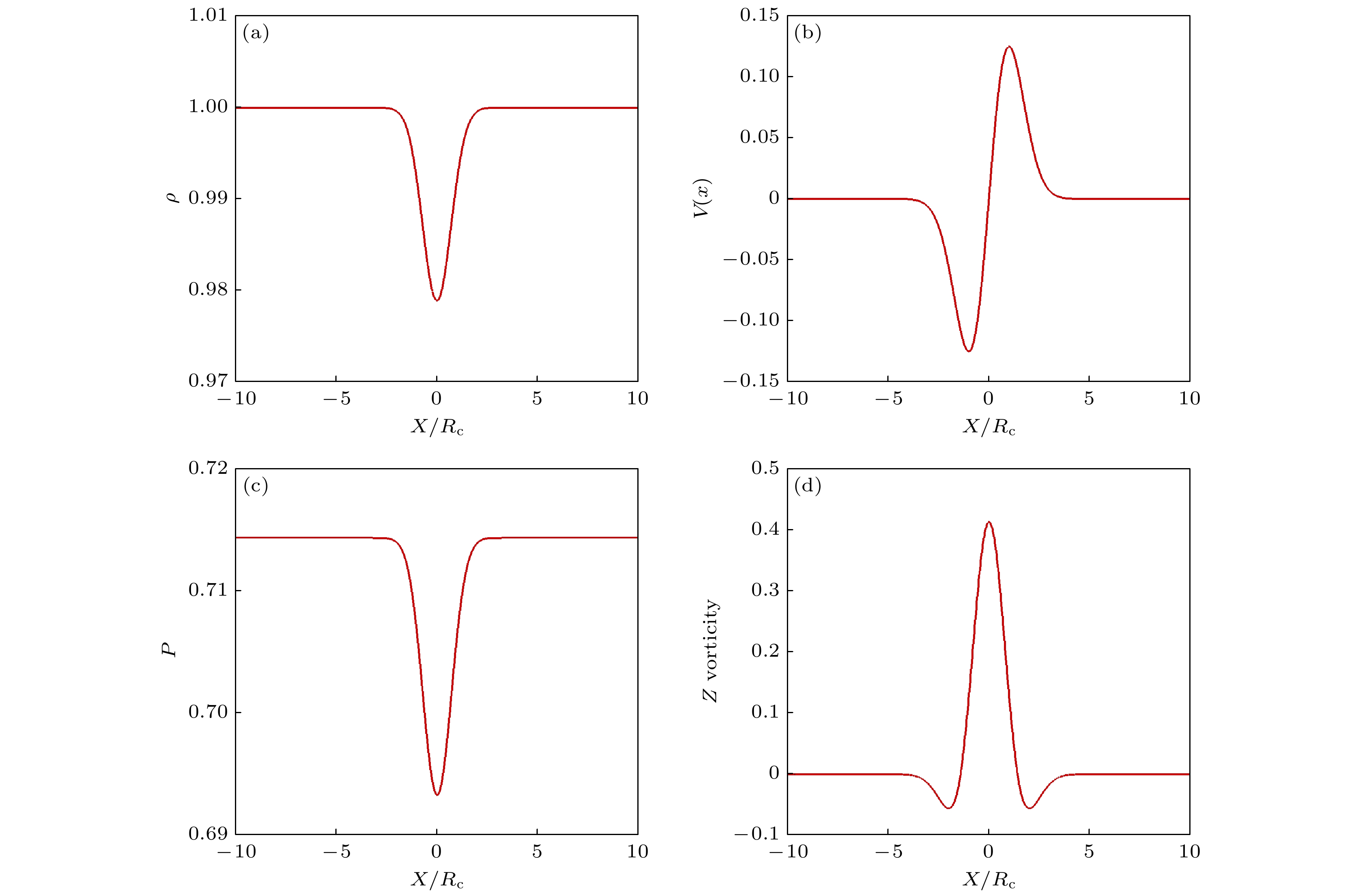
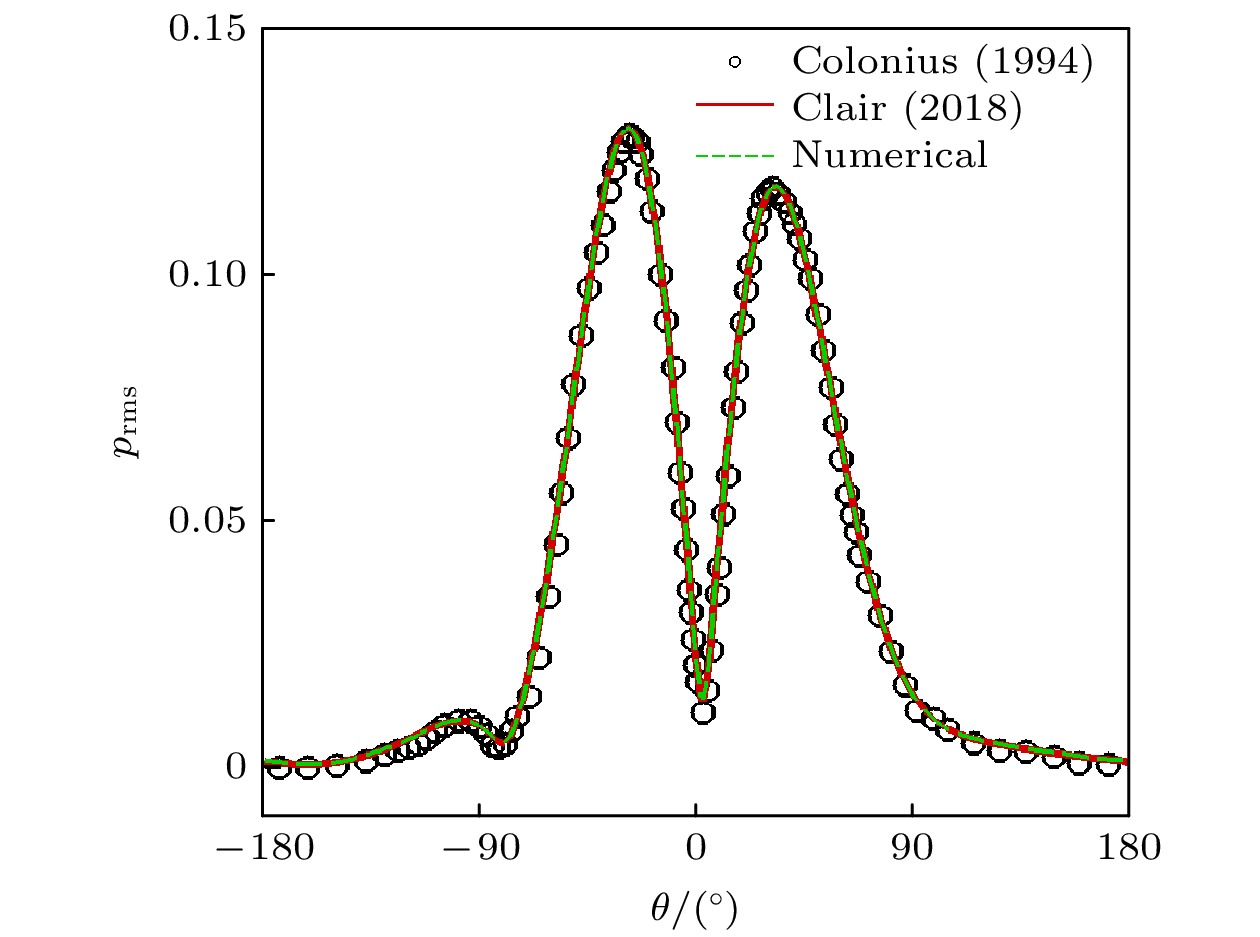
-
旋涡对声波的散射问题是声波在复杂流场中传播的基本问题, 在声源定位、声目标识别及探测、远场噪声预测等方面具有重要的学术研究价值和工程应用价值, 如飞行器的尾涡识别、探测及测距, 湍流剪切流中声目标预测, 声学风洞试验中声学测量和声源定位等. 声波穿过旋涡时会产生非线性散射现象, 其物理机理主要与声波波长和旋涡半径的长度尺度比相关. 本文采用高阶精度高分辨率线性紧致格式, 通过求解二维非定常Euler方程, 数值模拟了平面声波穿过静止等熵涡的物理问题. 通过引入声散射截面法, 分析了不同声波波长与旋涡半径的长度尺度比对声波脉动压强、声散射有效声压以及声散射能量的影响规律. 研究表明: 随着声波波长与旋涡半径的长度尺度比逐渐增加, 旋涡流场对声场的影响逐渐减弱, 声散射有效声压影响区域先逐渐增大随后逐渐减小, 声散射能量最大值呈现4种不同的变化阶段.The scattering of acoustic waves by a vortex is a fundamental problem of the acoustic waves propagation in complex flow field, which plays an important role in academic research and engineering application for sound source localization, acoustic target recognition and detection, the far field noise prediction, such as aircraft wake vortex identification, detection and ranging, acoustic target forecasting in turbulent shear flow, acoustic measurement and sound source localization in wind tunnel test, etc. The nonlinear scattering phenomenon occurs when acoustic wave passes through the vortex, which is mainly related to the length-scale ratio between the wavelength of acoustic wave and the core radius of the vortex. In this paper, a plane acoustic wave passing through a stationary isentropic vortex is numerically simulated by solving a two-dimensional compressible, unsteady Euler equation. A sixth-order linear compact finite difference scheme is employed for spatial discretization. Time integration is performed by a four-stage fourth-order Runge-Kutta method. The eighth-order spatial compact filter scheme is adopted to suppress high frequency errors. At the far field boundaries, buffer layer is applied to handle the outgoing acoustic wave. Under the matching condition, the accuracy of the numerical results is verified by comparing with the previous direct numerical simulation results. The acoustic scattering cross-section method is introduced to analyze the effects of different length-scale ratio on the acoustic pulsation pressure, acoustic scattering effective sound pressure and acoustic scattering energy. Scattering occurs when sound waves pass through the vortex, the acoustic field in front of the vortex is basically unaffected, and the acoustic wave front remains intact. A “vacuum” region is formed slightly below the acoustic field directly behind the vortex, and two primary interference bands and several secondary interference bands are formed on the upper and lower sides of the vortex. As the length-scale ratio increases, the sound scattering decreases and the influence of the vortex flow field on the acoustic field gradually weakens. The influence region of effective sound pressure of acoustic scattering is mainly concentrated behind the vortex. With the increase of the length scale ratio, the influence gradually increases and extends to the upstream, and then the influence region gradually decreases to the vicinity of the vortex. When the length scale ratio is greater than or equal to 6, the location of the maximum effective sound pressure of sound scattering jumps from the upper right to the lower right of the vortex. The influence of acoustic wave wavelength change on the acoustic scattering energy can be divided into three parts. With the increase of the length scale ratio, the maximum sound scattering energy presents four different stages.
-
Keywords:
- acoustic scattering /
- static isentropic vortex /
- acoustical scattering cross-section /
- length-scale ratio
[1] Colonius T, Lele S K 2004 Prog. Aerosp. Sci. 40 345
Google Scholar
[2] Ribner H S 1957 J. Acoust. Soc. Am. 29 435
Google Scholar
[3] Miles J W 1957 J. Acoust. Soc. Am. 29 226
Google Scholar
[4] Amiet R K 1975 AIAA Paper 75
Google Scholar
[5] Amiet R K 1978 J. Sound Vib. 58 467
Google Scholar
[6] Schlinker R H, Amiet R K 1980 NASA-CR-3371
[7] 张雪, 陈宝, 卢清华 2014 应用声学 33 433
Google Scholar
Zhang X, Chen B, Lu Q H 2014 J. Appl. Acoust. 33 433
Google Scholar
[8] Bogey C, Bailly C, Juvé D 2002 AIAA J. 40 235
Google Scholar
[9] 张军, 王勋年, 张俊龙, 卢翔宇, 陈正武 2018 实验流体力学 32 39
Google Scholar
Zhang J, Wang X N, Zhang J L, Lu X Y, Chen Z W 2018 J. Exp. Fluid Mech. 32 39
Google Scholar
[10] 张军, 陈鹏, 张俊龙, 卢翔宇 2018 航空动力学报 33 2458
Google Scholar
Zhang J, Chen P, Zhang J L, Lu X Y 2018 J. Aerosp. Power 33 2458
Google Scholar
[11] 倪章松, 张军, 王茂, 张俊龙 2020 航空动力学报 35 244
Google Scholar
Ni Z S, Zhang J, Wang M, Zhang J L 2020 J. Aerosp. Power 35 244
Google Scholar
[12] 王李璨, 陈荣钱, 尤延铖, 陈正武, 邱若凡 2019 西北工业大学学报 37 1148
Google Scholar
Wang L C, Chen R Q, You Y C, Chen Z W, Qiu R F 2019 J. Northwest. Polytech. Univ. 37 1148
Google Scholar
[13] Wang L C, Chen R Q, You Y C, Wu W J, Qiu R F 2019 Acta Acust. Acust. 105 732
Google Scholar
[14] Wang L C, Chen R Q, You Y C, Qiu R F 2020 J. Sound Vib. 492 115801
Google Scholar
[15] Candel S M 1979 J. Fluid Mech. 90 465
Google Scholar
[16] Colonius T, Lele S K, Moin P 1994 J. Fluid Mech. 260 271
Google Scholar
[17] Symons N P, Aldridge D F, Marlin D H, Wilson D K, Patton D G, Sullivan P P, Collier S L, Ostashev V E, Drob D P 2004 11th International Symposium on Long Range Sound Propagation
[18] Belyaev I V, Kopiev V F 2007 AIAA Paper 2007
Google Scholar
[19] Belyaev I V, Kopiev V F 2007 proceedings of the 7 th European Conference on Noise Control
[20] Karabasov S A, Kopiev V F, Goloviznin V M 2009 Proceedings of the 15th AIAA/CEAS Aeroacoustics Conference (30th AIAA Aeroacoustics Conference)
[21] Kopiev V F, Belyaev I V 2010 J. Sound Vib. 329 1409
Google Scholar
[22] Cheinet S, Ehrhardt L, Juve D, Blanc-Benon P 2012 J. Acoust. Soc. Am. 132 2198
Google Scholar
[23] Ke G Y, Li W, Zheng Z C 2015 AIAA Paper 2015
Google Scholar
[24] Clair V, Gabard G 2018 J. Fluid Mech. 841 50
Google Scholar
[25] Lele S K 1992 J. Comput. Phys. 103 16
Google Scholar
[26] Liu X L, Zhang S H, Zhang H X, Shu C W 2013 J. Comput. Phys. 248 235
Google Scholar
[27] 刘旭亮 2011 硕士学位论文 (绵阳: 中国空气动力研究与发展中心)
Liu X L 2011 M. S. Thesis (Mianyang: China Aerodynamics Research and Development Center) (in Chinese)
[28] Jiang G S, Shu C W 1996 J. Comput. Phys. 126 202
Google Scholar
[29] 王益民 2017 硕士学位论文 (绵阳: 中国空气动力研究与发展中心)
Wang Y M 2017 Master Dissertation (Mianyang: China Aerodynamics Research and Development Cen-ter) (in Chinese)
[30] Inoue O, Hattori Y 1999 J. Fluid Mech. 380 81
Google Scholar
[31] Zhang S H, Zhang Y T, Chi C W 2005 Phys. Fluids 17 116101
Google Scholar
[32] Robert H K 1953 J. Acoust. Soc. Am. 25 1096
Google Scholar
[33] Hattori Y, Llewellyn S S G 2002 J. Fluid Mech. 473 275
Google Scholar
[34] Shi J, Yang D S, Zhang H Y, Shi S G, Li S, Hu B 2017 Chin. Phys. B 26 074301
Google Scholar
[35] Wu J Z 1991 Adv. Mech. 21 430
Google Scholar
[36] Wu J Z 1992 Adv. Mech. 22 35
Google Scholar
-
图 1 平面声波穿过旋涡的示意图
Fig. 1. Schematic diagram of acoustic wave propagating through a vortex
图 2 静止等熵涡初始分布 (a) 密度; (b) 速度; (c) 压强; (d) 涡量
Fig. 2. Initial distribution of stationary isentropic vortices: (a) density; (b) velocity; (c)pressure; (d) vorticity.
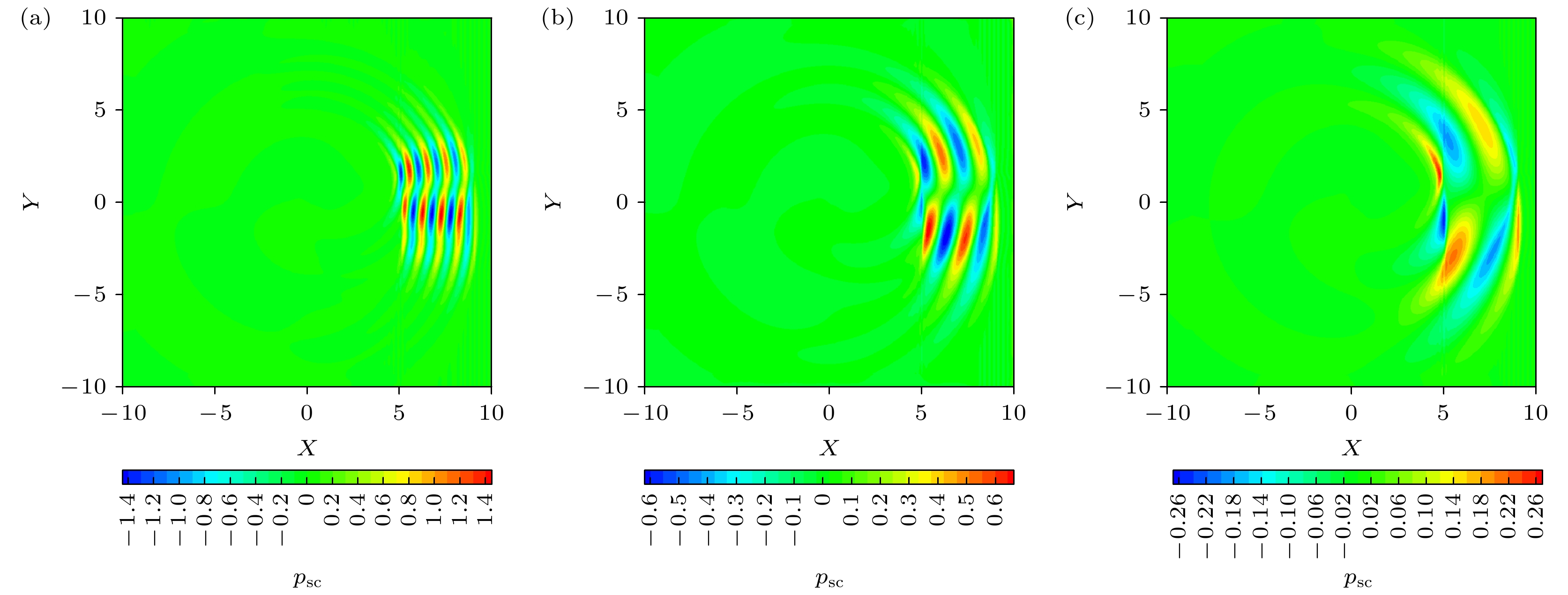
图 4 声波脉动压强随长度尺度比
$r_{\rm L}$ 的变化云图 (a)$ r_{\rm L} = 0.1 $ ; (b)$ r_{\rm L} = 0.5 $ ; (c)$ r_{\rm L} = 1.0 $ ; (d)$ r_{\rm L} = 5.0 $ ; (e)$ r_{\rm L} = 10.0 $ ; (f)$ r_{\rm L} = 20.0 $ Fig. 4. The contour of the change of acoustic wave pressure with the length-scale ratio
$ r_{\rm L} $ : (a)$ r_{\rm L} = 0.1 $ ; (b)$ r_{\rm L} = 0.5 $ ; (c)$ r_{\rm L} = 1.0 $ ; (d)$ r_{\rm L} = 5.0 $ ; (e)$ r_{\rm L} = 10.0 $ ; (f)$ r_{\rm L} = 20.0 $ .
图 5 散射有效声压
$ p_{\rm rms} $ 随长度尺度比$ r_{\rm L} $ 的变化云图 (a)$ r_{\rm L} = 0.1 $ ; (b)$ r_{\rm L} = 0.5 $ ; (c)$ r_{\rm L} = 1.0 $ ; (d)$ r_{\rm L} = 5.0 $ ; (e)$r_{\rm L} = $ $ 10.0$ ; (f)$ r_{\rm L} = 20.0 $ Fig. 5. The contour of the change of the root-mean-square of scattering pressure with the length-scale ratio
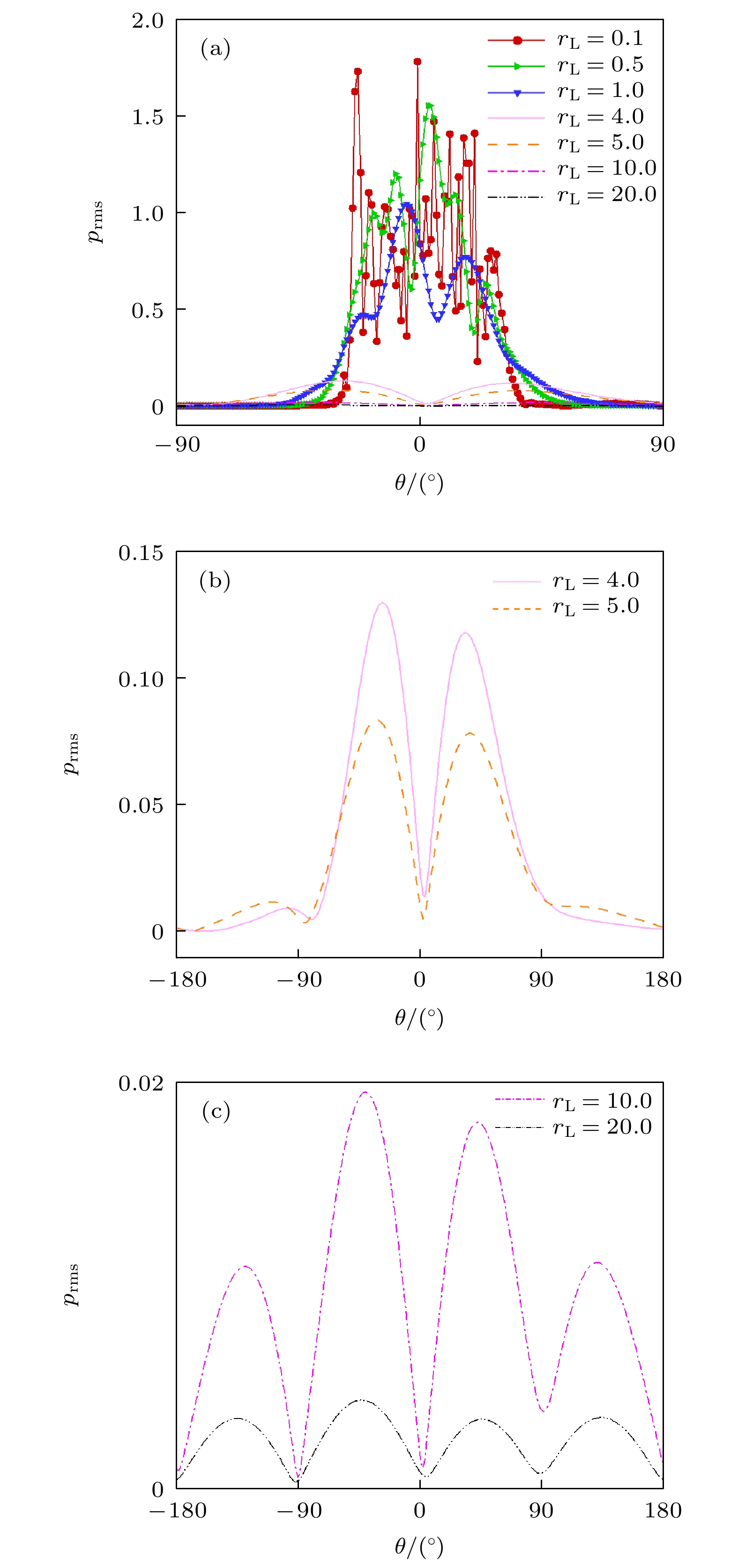
$ r_{\rm L} $ : (a)$ r_{\rm L} = 0.1 $ ; (b)$ r_{\rm L} = 0.5 $ ; (c)$ r_{\rm L} = 1.0 $ ; (d)$ r_{\rm L} = 5.0 $ ; (e)$ r_{\rm L} = 10.0 $ ; (f)$ r_{\rm L} = 20.0 $ .
图 6 散射有效声压在半径为
$ r = 8 R_{\rm c} $ 圆周上的分布 (a) 全局图; (b) 局部放大图1; (c) 局部放大图2Fig. 6. The distribution of root-mean-square pressure of scattered wave on a circle with radius
$ 8 R_{\rm c} $ : (a) Global; (b) zoomed 1; (c) zoomed 2.
图 7 散射有效声压最大值随长度尺度比的变化曲线 (a) 散射有效声压最大值; (b) 散射有效声压最大值点的半径; (c) 散射有效声压最大值点的角度
Fig. 7. The curve of the root-mean-square pressure of scattered wave with
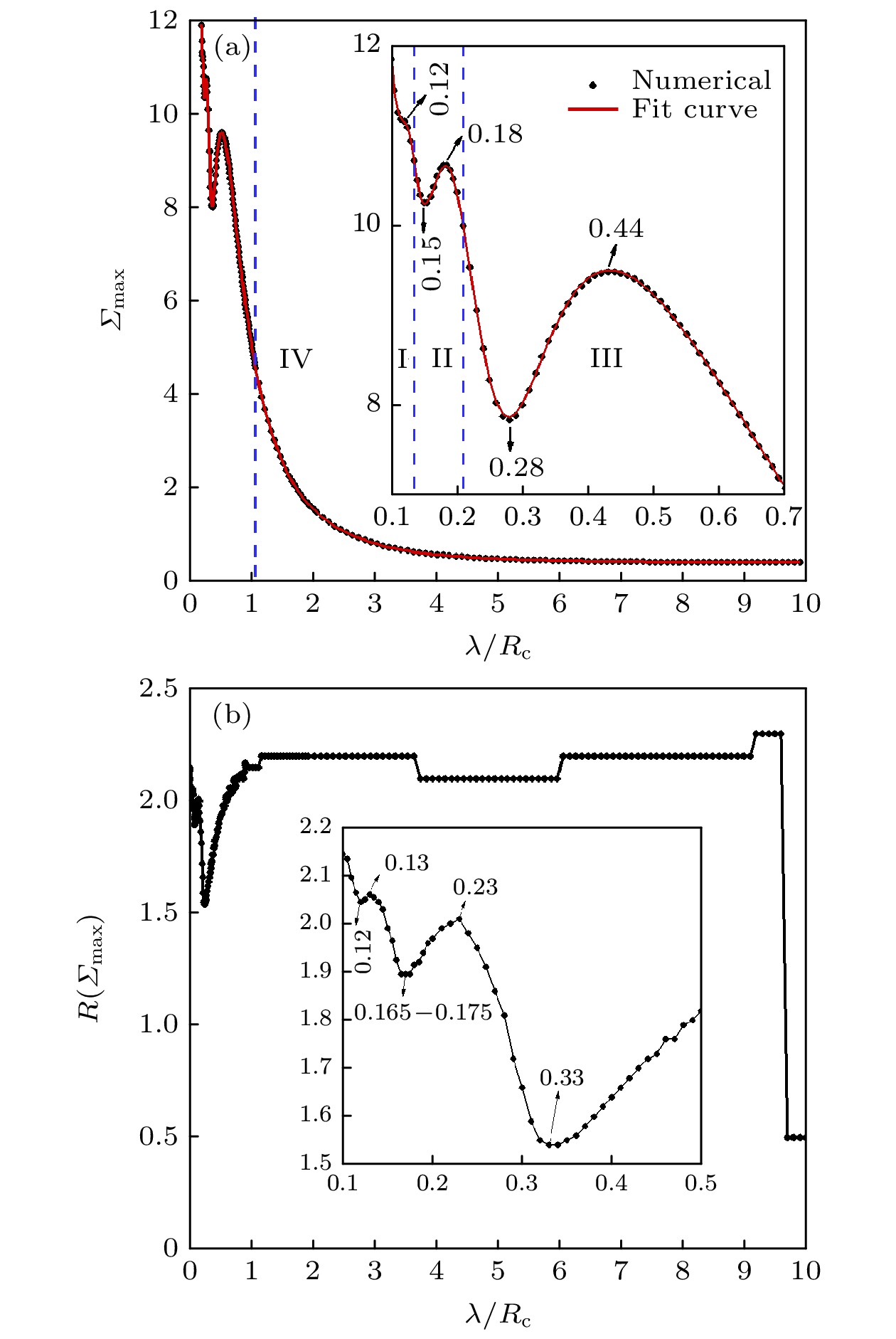
$r_{\rm L}$ value: (a)$ {p_{{\rm rms}\;{\rm max}}} $ ; (b)$ R\left({p_{{\rm rms}\;{\rm max}}} \right) $ ; (c)$ \theta \left( {p_{{\rm rms}\;{\rm max}}} \right) $ .
图 8 声散射能量
$ \varSigma $ 随观测半径$ R $ 的变化曲线 (a)$r_{\rm L} \in $ $ \left ( 0.1, \, 1.0 \right)$ ; (b)$ r_{\rm L} \in \left ( 2.0, \, 5.0 \right) $ ; (c)$ r_{\rm L} \in \left ( 6.0, \, 30.0 \right) $ Fig. 8. The curve of acoustical scattering cross-section with observed radius: (a)
$ r_{\rm L} \in \left ( 0.1, \, 1.0 \right) $ ; (b)$ r_{\rm L} \in \left ( 2.0, \, 5.0 \right) $ ; (c)$ r_{\rm L} \in \left ( 6.0, \, 30.0 \right) $ .
图 9 声散射能量随尺度比的变化曲线 (a)
$\varSigma_{\max }$ ; (b)$R\left(\varSigma_{\max }\right )$ Fig. 9. The curve of acoustical scattering cross-section with
$ r_{\rm L} $ value: (a)$\varSigma_{\max }$ ; (b)$R\left( \varSigma_{\max }\right )$ .
图 11 声散射压强云图 (a)
$ \lambda = 1 $ ; (b)$ \lambda = 2 $ ; (c)$ \lambda = 4 $ Fig. 11. The contour of sound scattering pressure: (a)
$ \lambda = 1 $ ; (b)$ \lambda = 2 $ ; (c)$ \lambda = 4 $ . -
[1] Colonius T, Lele S K 2004 Prog. Aerosp. Sci. 40 345
Google Scholar
[2] Ribner H S 1957 J. Acoust. Soc. Am. 29 435
Google Scholar
[3] Miles J W 1957 J. Acoust. Soc. Am. 29 226
Google Scholar
[4] Amiet R K 1975 AIAA Paper 75
Google Scholar
[5] Amiet R K 1978 J. Sound Vib. 58 467
Google Scholar
[6] Schlinker R H, Amiet R K 1980 NASA-CR-3371
[7] 张雪, 陈宝, 卢清华 2014 应用声学 33 433
Google Scholar
Zhang X, Chen B, Lu Q H 2014 J. Appl. Acoust. 33 433
Google Scholar
[8] Bogey C, Bailly C, Juvé D 2002 AIAA J. 40 235
Google Scholar
[9] 张军, 王勋年, 张俊龙, 卢翔宇, 陈正武 2018 实验流体力学 32 39
Google Scholar
Zhang J, Wang X N, Zhang J L, Lu X Y, Chen Z W 2018 J. Exp. Fluid Mech. 32 39
Google Scholar
[10] 张军, 陈鹏, 张俊龙, 卢翔宇 2018 航空动力学报 33 2458
Google Scholar
Zhang J, Chen P, Zhang J L, Lu X Y 2018 J. Aerosp. Power 33 2458
Google Scholar
[11] 倪章松, 张军, 王茂, 张俊龙 2020 航空动力学报 35 244
Google Scholar
Ni Z S, Zhang J, Wang M, Zhang J L 2020 J. Aerosp. Power 35 244
Google Scholar
[12] 王李璨, 陈荣钱, 尤延铖, 陈正武, 邱若凡 2019 西北工业大学学报 37 1148
Google Scholar
Wang L C, Chen R Q, You Y C, Chen Z W, Qiu R F 2019 J. Northwest. Polytech. Univ. 37 1148
Google Scholar
[13] Wang L C, Chen R Q, You Y C, Wu W J, Qiu R F 2019 Acta Acust. Acust. 105 732
Google Scholar
[14] Wang L C, Chen R Q, You Y C, Qiu R F 2020 J. Sound Vib. 492 115801
Google Scholar
[15] Candel S M 1979 J. Fluid Mech. 90 465
Google Scholar
[16] Colonius T, Lele S K, Moin P 1994 J. Fluid Mech. 260 271
Google Scholar
[17] Symons N P, Aldridge D F, Marlin D H, Wilson D K, Patton D G, Sullivan P P, Collier S L, Ostashev V E, Drob D P 2004 11th International Symposium on Long Range Sound Propagation
[18] Belyaev I V, Kopiev V F 2007 AIAA Paper 2007
Google Scholar
[19] Belyaev I V, Kopiev V F 2007 proceedings of the 7 th European Conference on Noise Control
[20] Karabasov S A, Kopiev V F, Goloviznin V M 2009 Proceedings of the 15th AIAA/CEAS Aeroacoustics Conference (30th AIAA Aeroacoustics Conference)
[21] Kopiev V F, Belyaev I V 2010 J. Sound Vib. 329 1409
Google Scholar
[22] Cheinet S, Ehrhardt L, Juve D, Blanc-Benon P 2012 J. Acoust. Soc. Am. 132 2198
Google Scholar
[23] Ke G Y, Li W, Zheng Z C 2015 AIAA Paper 2015
Google Scholar
[24] Clair V, Gabard G 2018 J. Fluid Mech. 841 50
Google Scholar
[25] Lele S K 1992 J. Comput. Phys. 103 16
Google Scholar
[26] Liu X L, Zhang S H, Zhang H X, Shu C W 2013 J. Comput. Phys. 248 235
Google Scholar
[27] 刘旭亮 2011 硕士学位论文 (绵阳: 中国空气动力研究与发展中心)
Liu X L 2011 M. S. Thesis (Mianyang: China Aerodynamics Research and Development Center) (in Chinese)
[28] Jiang G S, Shu C W 1996 J. Comput. Phys. 126 202
Google Scholar
[29] 王益民 2017 硕士学位论文 (绵阳: 中国空气动力研究与发展中心)
Wang Y M 2017 Master Dissertation (Mianyang: China Aerodynamics Research and Development Cen-ter) (in Chinese)
[30] Inoue O, Hattori Y 1999 J. Fluid Mech. 380 81
Google Scholar
[31] Zhang S H, Zhang Y T, Chi C W 2005 Phys. Fluids 17 116101
Google Scholar
[32] Robert H K 1953 J. Acoust. Soc. Am. 25 1096
Google Scholar
[33] Hattori Y, Llewellyn S S G 2002 J. Fluid Mech. 473 275
Google Scholar
[34] Shi J, Yang D S, Zhang H Y, Shi S G, Li S, Hu B 2017 Chin. Phys. B 26 074301
Google Scholar
[35] Wu J Z 1991 Adv. Mech. 21 430
Google Scholar
[36] Wu J Z 1992 Adv. Mech. 22 35
Google Scholar
下载:
计量
- 文章访问数: 4219
- PDF下载量: 89
- 被引次数: 0
