-
利用部分波展开法求解得到了Gauss声束入射下刚性和非刚性椭圆柱的声散射系数, 推导了一般情况下的声辐射力矩表达式. 在此基础上, 通过一系列数值仿真详细分析了离轴距离、入射角度和束腰半径对声辐射力矩的影响. 结果表明: 正向与负向声辐射力矩均可以在一定条件下存在; 低频情况下刚性椭圆柱比非刚性椭圆柱更容易产生较强的声辐射力矩; 特定频率的入射声场可以激发出非刚性椭圆柱不同阶的共振散射模式, 因而非刚性椭圆柱的声辐射力矩峰值与频率的关系更密切; 增加束腰半径有利于扩大散射截面, 进而增加椭圆柱的声辐射力矩. 该研究结果预期可以为利用声辐射力矩实现粒子的可控旋转和流体黏度的反演提供一定的理论指导.As one of the nonlinear effects of acoustic waves, the time-averaged acoustic radiation torque expression is derived from the transfer of angular momentum from the incident beam to the object. In recent years, the acoustic radiation torque has received substantial attention since it is the underlying principle of well-controlled particle rotations and spins, which provides a new degree of freedom in particle manipulation and acousto-fluidic applications in addition to the translational displacement caused by the acoustic radiation force. Cylindrical particles, such as fibers, carbon nanotubes and other surface acoustic wave devices, are commonly encountered in various applications. The acoustic scattering coefficients for an elliptical cylinder arbitrarily located at the field of Gauss beam in two-dimensions are computed based on the partial-wave series expansion method and the Graf’s additional theorem for cylindrical functions to obtain the off-axis beam shape coefficients. It is worth mentioning that both the rigid and non-rigid cylinders are considered in this work, which requires different boundary conditions at the cylinder surface. Moreover, the closed-form expression of the acoustic radiation torque in this case is derived. On this basis, several numerical simulations are performed with particular emphasis on the off-axis distance, the incident angle and the beam waist. The simulated results show that both the positive and negative acoustic radiation torque can exist under certain conditions, which means that 1) the elliptical cylinder can be rotated in either the clockwise or the counterclockwise direction, 2) rigid elliptical cylinders are more likely to experience a strong acoustic radiation torque than non-rigid elliptical cylinders at low frequencies, 3) the incident wave field with a specific frequency can excite a different resonance scattering mode for a non-rigid elliptical cylinder, therefore the acoustic radiation torque peak is more related to the beam frequency than to the elliptical cylinder’s location in the field, and 4) increasing the beam width can enlarge the scattering cross section area, and thus enhancing the acoustic radiation torque on the elliptical cylinder. The results in this study are expected to provide a theoretical guide for the controllable rotation of a particle and the viscosity inversion of fluid by using the acoustic radiation torque. The exact formalism presented here by using the multipole expansion method, which is valid for any frequency range, can be used to validate other approaches by using purely numerical methods.
-
Keywords:
- acoustic radiation torque /
- Gauss beam /
- elliptical cylinder /
- partial-wave series expansion method /
- acoustic manipulation
[1] Wu J R 1991 J. Acoust. Soc. Am. 89 2140
Google Scholar
[2] 黄先玉, 蔡飞燕, 李文成, 郑海荣, 何兆剑, 邓科, 赵鹤平 2017 66 044301
Google Scholar
Huang X Y, Cai F Y, Li W C, Zheng H R, He Z J, Deng K, Zhao H P 2017 Acta Phys. Sin. 66 044301
Google Scholar
[3] Ozcelik A, Rufo J, Guo F, Gu Y Y, Li P, Lata J 2018 Nat. Methods 15 1021
Google Scholar
[4] Baudoin M, Thomas J L 2020 Annu. Rev. Fluid Mech. 52 205
Google Scholar
[5] Lierke E G 1996 Acustica 82 220
[6] Yarin A L, Pfaffenlehner M, Tropea C 1998 J. Fluid Mech. 356 65
Google Scholar
[7] Chung S K, Trinh E H 1998 J. Cryst. Growth 194 384
Google Scholar
[8] Mitri F G, Garzon F H, Sinha D N 2011 Rev. Sci. Instrum. 82 034903
Google Scholar
[9] Maidanik G 1958 J. Acoust. Soc. Am. 30 620
Google Scholar
[10] Fan Z W, Mei D Q, Yang K J, Chen Z C 2008 J. Acoust. Soc. Am. 124 2727
Google Scholar
[11] Zhang L K, Marston P L 2011 J. Acoust. Soc. Am. 129 1679
Google Scholar
[12] Zhang L K, Marston P L 2011 Phys. Rev. E 84 065601
Google Scholar
[13] Silva G T, Lobo T P, Mitri F G 2012 EPL 97 54003
Google Scholar
[14] Mitri F G 2012 Phys. Rev. E 85 026602
Google Scholar
[15] Mitri F G, Lobo T P, Silva G T 2012 Phys. Rev. E 86 059902
Google Scholar
[16] Zhang L K 2018 Phys. Rev. Appl. 10 034039
Google Scholar
[17] Gong Z X, Marston P L 2019 Phys. Rev. Appl. 11 064022
Google Scholar
[18] Zeng Q, Li L L, Ma H L, Xu J H, Fan Y S, Wang H 2013 Appl. Phys. Lett. 102 213106
Google Scholar
[19] Yamahira S, Hatanaka S, Kuwabara M 2000 Jpn. J. Appl. Phys. 39 3683
Google Scholar
[20] Shilton R, Tan M K, Yeo L Y, Friend J R 2008 J. Appl. Phys. 104 014910
Google Scholar
[21] Hasheminejad S M, Sanaei R 2007 J. Comput. Acoust. 15 377
Google Scholar
[22] Wang J T, Dual J 2011 J. Acoust. Soc. Am. 129 3490
Google Scholar
[23] Mitri F G 2016 Phys. Fluids 28 077104
Google Scholar
[24] Mitri F G 2016 Wave Motion 66 31
Google Scholar
[25] Mitri F G 2017 J. Appl. Phys. 121 144901
Google Scholar
[26] Wang H B, Gao S, Qiao Y P, Liu J H, Liu X Z 2019 Phys. Fluids 31 047103
Google Scholar
[27] Mitri F G 2018 Appl. Phys. 124 054902
Google Scholar
[28] Mitri F G, Fellah Z E A, Silva G T 2014 J. Sound Vib. 333 7326
Google Scholar
[29] Qiao Y P, Zhang X F, Zhang G B 2017 J. Acoust. Soc. Am. 141 4633
Google Scholar
[30] Flax L, Dragonette L R, Uberall H 1978 J. Acoust. Soc. Am. 63 723
Google Scholar
[31] Werby M F, Uberall H, Nagl A, Brown S H, Dickey J W 1988 J. Acoust. Soc. Am. 84 1425
Google Scholar
[32] Wiegel F W 1979 Phys. Lett. A 70 112
Google Scholar
-
图 1 Gauss声束斜入射到无限长椭圆柱上
Fig. 1. Description for the interaction of a Gauss beam with an infinitely long elliptical cylinder.
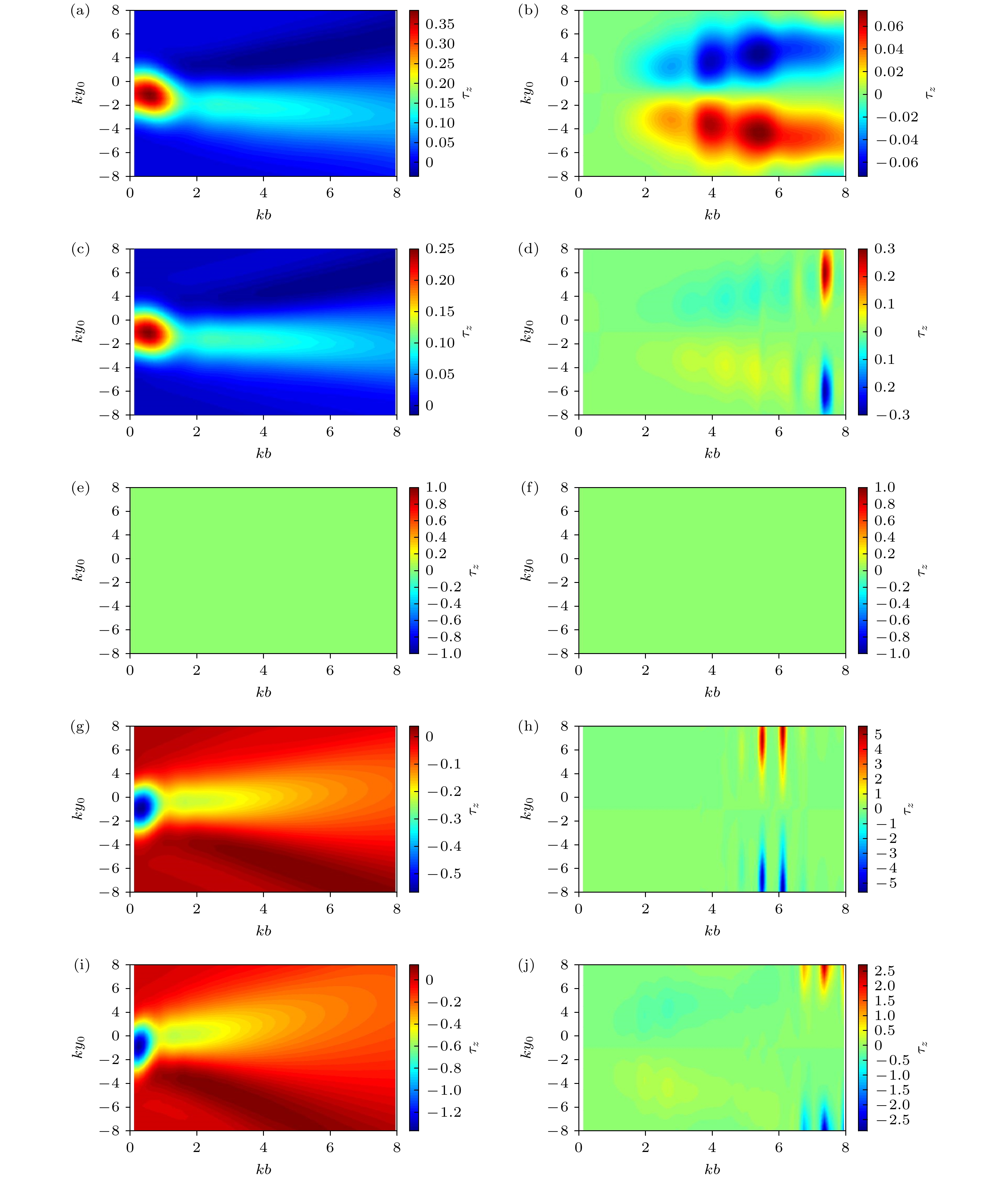
图 2 椭圆柱的声辐射力矩函数随kb和ky0的变化关系(kx0 = 0, kW0 = 3) (a) a/b = 1/2, 刚性; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, 刚性; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, 刚性; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, 刚性; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, 刚性; (j) a/b = 2, PDMS-TBE
Fig. 2. The acoustic radiation torque function plots for an elliptical cylinder versus kb and ky0 (kx0 = 0, kW0 = 3): (a) a/b = 1/2, rigid; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, rigid; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, rigid; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, rigid; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, rigid; (j) a/b = 2, PDMS-TBE.
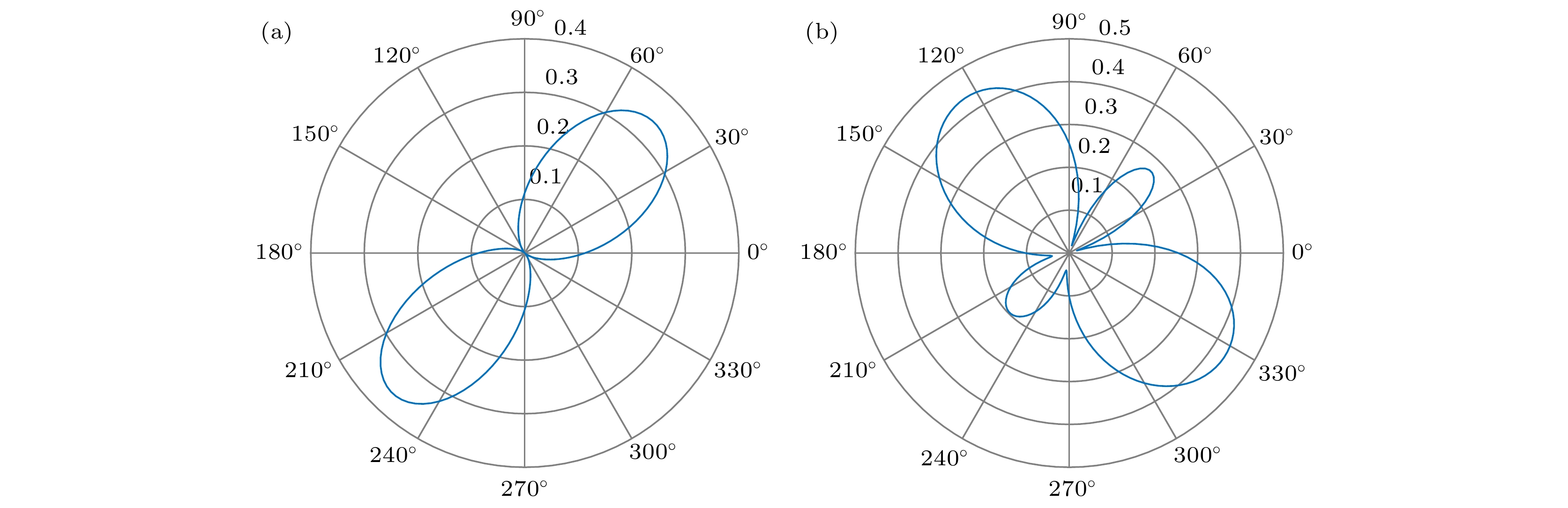
图 3 PDMS椭圆柱的共振散射函数幅值
$\left| {{f^{{\rm{res}}}}} \right|$ 随角度θ的变化关系$(kx_0 \!=\! 0, ky_0 \!=\! 6, \alpha \!=\! \pi/4, kW_0 \!=\! 3) ~~{\rm(a)}~ kb \!=\! 5.5; ~{\rm (b)}~ kb \!=\! 6.1$ Fig. 3. The resonance scattering function modulus
$\left| {{f^{{\rm{res}}}}} \right|$ for a PDMS-TBE elliptical cylinder versus the angle θ (kx0 = 0, ky0 = 6, α = π/4, kW0 = 3): (a) kb = 5.5; (b) kb = 6.1.
图 4 椭圆柱的声辐射力矩函数随kb和kx0的变化关系(ky0 = –3, kW0 = 3) (a) a/b = 1/2, 刚性; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, 刚性; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, 刚性; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, 刚性; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, 刚性; (j) a/b = 2, PDMS-TBE
Fig. 4. The acoustic radiation torque function plots for an elliptical cylinder versus kb and kx0 (ky0 = –3, kW0 = 3): (a) a/b = 1/2, rigid; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, rigid; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, rigid; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, rigid; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, rigid; (j) a/b = 2, PDMS-TBE.
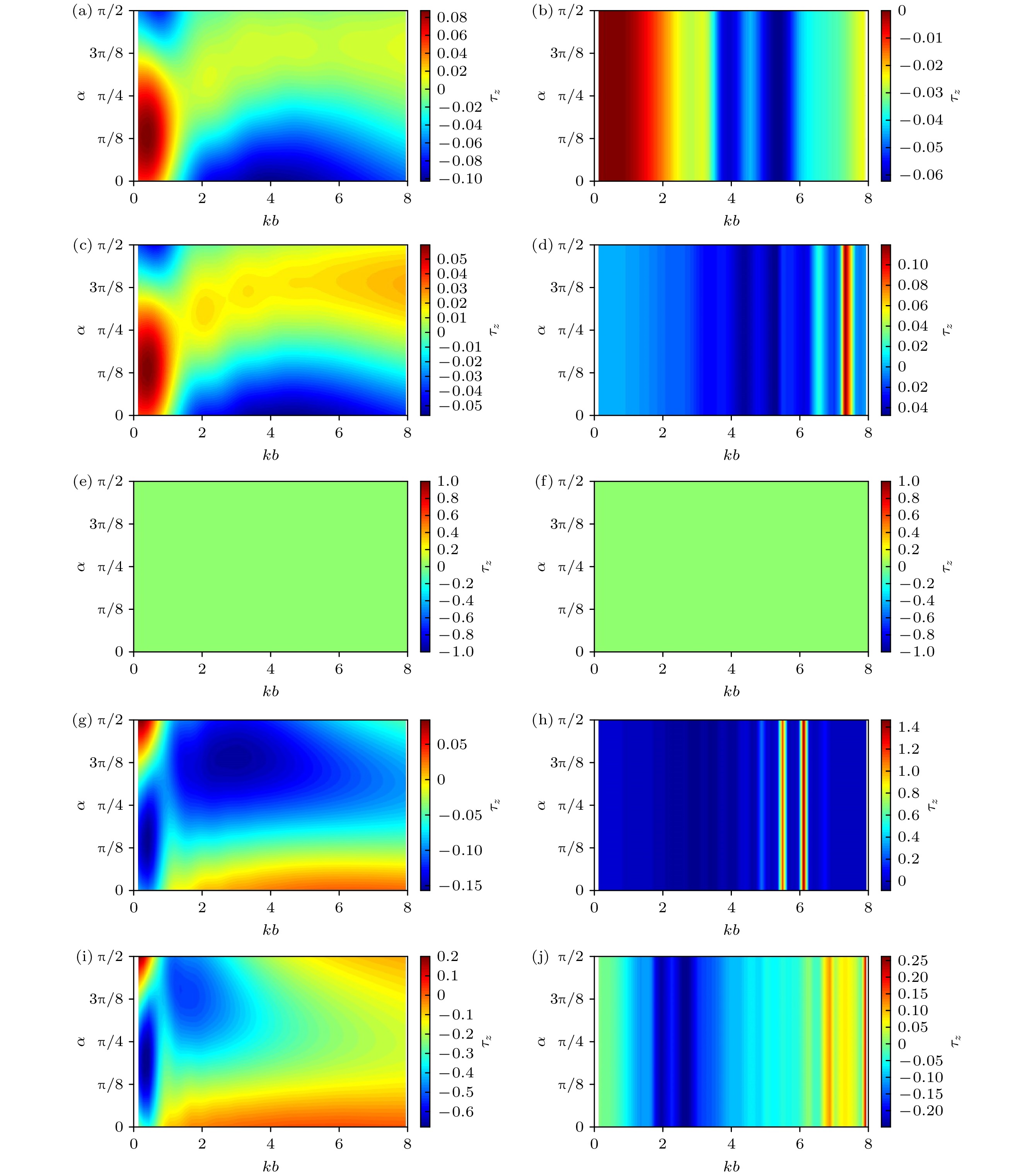
图 5 椭圆柱的声辐射力矩函数随kb和α的变化关系(kx0 = –3, ky0 = –3, kW0 = 3) (a) a/b = 1/2, 刚性; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, 刚性; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, 刚性; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, 刚性; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, 刚性; (j) a/b = 2, PDMS-TBE
Fig. 5. The acoustic radiation torque function plots for an elliptical cylinder versus kb and α (kx0 = –3, ky0 = –3, kW0 = 3): (a) a/b = 1/2, rigid; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, rigid; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, rigid; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, rigid; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, rigid; (j) a/b = 2, PDMS-TBE.
图 6 椭圆柱的声辐射力矩函数随kb和kW0的变化关系(kx0 = –3, ky0 = –3, α = π/4) (a) a/b = 1/2, 刚性; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, 刚性; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, 刚性; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, 刚性; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, 刚性; (j) a/b = 2, PDMS-TBE
Fig. 6. The acoustic radiation torque function plots for an elliptical cylinder versus kb and kW0 (kx0 = –3, ky0 = –3, α = π/4): (a) a/b = 1/2, rigid; (b) a/b = 1/2, PDMS-TBE; (c) a/b = 2/3, rigid; (d) a/b = 2/3, PDMS-TBE; (e) a/b = 1, rigid; (f) a/b = 1, PDMS-TBE; (g) a/b = 3/2, rigid; (h) a/b = 3/2, PDMS-TBE; (i) a/b = 2, rigid; (j) a/b = 2, PDMS-TBE.
图 7 PDMS-TBE椭圆柱的声辐射力矩函数随kb的变化关系(kx0 = 0, ky0 = 6, kW0 = 3, α = π/4)
Fig. 7. The acoustic radiation torque function plots for a PDMS-TBE elliptical cylinder versus kb (kx0 = 0, ky0 = 6, kW0 = 3, α = π/4).
-
[1] Wu J R 1991 J. Acoust. Soc. Am. 89 2140
Google Scholar
[2] 黄先玉, 蔡飞燕, 李文成, 郑海荣, 何兆剑, 邓科, 赵鹤平 2017 66 044301
Google Scholar
Huang X Y, Cai F Y, Li W C, Zheng H R, He Z J, Deng K, Zhao H P 2017 Acta Phys. Sin. 66 044301
Google Scholar
[3] Ozcelik A, Rufo J, Guo F, Gu Y Y, Li P, Lata J 2018 Nat. Methods 15 1021
Google Scholar
[4] Baudoin M, Thomas J L 2020 Annu. Rev. Fluid Mech. 52 205
Google Scholar
[5] Lierke E G 1996 Acustica 82 220
[6] Yarin A L, Pfaffenlehner M, Tropea C 1998 J. Fluid Mech. 356 65
Google Scholar
[7] Chung S K, Trinh E H 1998 J. Cryst. Growth 194 384
Google Scholar
[8] Mitri F G, Garzon F H, Sinha D N 2011 Rev. Sci. Instrum. 82 034903
Google Scholar
[9] Maidanik G 1958 J. Acoust. Soc. Am. 30 620
Google Scholar
[10] Fan Z W, Mei D Q, Yang K J, Chen Z C 2008 J. Acoust. Soc. Am. 124 2727
Google Scholar
[11] Zhang L K, Marston P L 2011 J. Acoust. Soc. Am. 129 1679
Google Scholar
[12] Zhang L K, Marston P L 2011 Phys. Rev. E 84 065601
Google Scholar
[13] Silva G T, Lobo T P, Mitri F G 2012 EPL 97 54003
Google Scholar
[14] Mitri F G 2012 Phys. Rev. E 85 026602
Google Scholar
[15] Mitri F G, Lobo T P, Silva G T 2012 Phys. Rev. E 86 059902
Google Scholar
[16] Zhang L K 2018 Phys. Rev. Appl. 10 034039
Google Scholar
[17] Gong Z X, Marston P L 2019 Phys. Rev. Appl. 11 064022
Google Scholar
[18] Zeng Q, Li L L, Ma H L, Xu J H, Fan Y S, Wang H 2013 Appl. Phys. Lett. 102 213106
Google Scholar
[19] Yamahira S, Hatanaka S, Kuwabara M 2000 Jpn. J. Appl. Phys. 39 3683
Google Scholar
[20] Shilton R, Tan M K, Yeo L Y, Friend J R 2008 J. Appl. Phys. 104 014910
Google Scholar
[21] Hasheminejad S M, Sanaei R 2007 J. Comput. Acoust. 15 377
Google Scholar
[22] Wang J T, Dual J 2011 J. Acoust. Soc. Am. 129 3490
Google Scholar
[23] Mitri F G 2016 Phys. Fluids 28 077104
Google Scholar
[24] Mitri F G 2016 Wave Motion 66 31
Google Scholar
[25] Mitri F G 2017 J. Appl. Phys. 121 144901
Google Scholar
[26] Wang H B, Gao S, Qiao Y P, Liu J H, Liu X Z 2019 Phys. Fluids 31 047103
Google Scholar
[27] Mitri F G 2018 Appl. Phys. 124 054902
Google Scholar
[28] Mitri F G, Fellah Z E A, Silva G T 2014 J. Sound Vib. 333 7326
Google Scholar
[29] Qiao Y P, Zhang X F, Zhang G B 2017 J. Acoust. Soc. Am. 141 4633
Google Scholar
[30] Flax L, Dragonette L R, Uberall H 1978 J. Acoust. Soc. Am. 63 723
Google Scholar
[31] Werby M F, Uberall H, Nagl A, Brown S H, Dickey J W 1988 J. Acoust. Soc. Am. 84 1425
Google Scholar
[32] Wiegel F W 1979 Phys. Lett. A 70 112
Google Scholar
下载:
计量
- 文章访问数: 4949
- PDF下载量: 58
- 被引次数: 0
