-
运用分子动力学方法模拟研究了旋转的黑磷纳米管对管内水流的轴向驱动特性, 研究结果表明: 手性黑磷纳米管在旋转时会驱动管内水分子沿轴向运动, 运动方向由纳米管转向决定; 管内水流的流速和驱动力会随着黑磷管转速的提高而增大. 采用黑磷双壁Couette模型计算分析了水-黑磷界面的摩擦系数及滑移特性, 阐明了黑磷表面天然的各向异性微结构是旋转黑磷管轴向驱动水流的本质原因. 构建了在双层黑磷纳米管间填充水分子的模型, 发现内外黑磷管同时旋转时, 管间水分子的轴向运动会增强. 纳米管半径也会对水分子的定向运动产生影响, 具体表现为在相同转速下, 随着纳米管半径的增大, 管内水分子在轴向上的运动速度会减小, 而受力则会增大; 双壁黑磷纳米管在旋转时管内水分子的轴向运动情况和单壁黑磷纳米管模型差异很小, 证明黑磷管层数对水流驱动效果的影响不明显; 温度对水流驱动效果的影响规律取决于管内压强和温度对流固界面摩擦系数的耦合作用, 当温度低于常温时水分子在轴向上的速度和受力会随着温度的升高而增大, 当温度达到常温时则趋于平稳. 研究结果可为基于黑磷纳米管的流体传动器件的设计和应用提供理论基础.Since the advent of two-dimensional materials, the micro/nano technology has been greatly developed, and the design of micro/nano fluid devices has become an important research area. As a new two-dimensional material, the black phosphorus (BP) has attracted wide attention because of its excellent properties such as anisotropy, and it has been applied to many areas. In this paper, the axial motion properties of water molecules in the rotating black phosphorus nanotube (BPNT) are studied by the molecular dynamics method. The results show that water molecules in the rotating chiral BPNT can move along the axis, and the moving direction of water molecules is determined by the rotating direction of the nanotube. The velocity of water molecules and the resultant force of water molecules received from the nanotube in the axial direction increase with the angular velocity increasing. The friction coefficient and slip characteristics of the water-BP interface are calculated by using the Couette flow model, and it is clarified that the natural anisotropic microstructure on the surface of BP is the essential reason for the axial motion of water molecules in the rotating BPNT. Besides, we construct a model of filling water molecules between two BPNTs. It is found that the axial movement of water molecules between two nanotubes will be enhanced when the internal and external tube rotate simultaneously. The radius of the nanotubes will also affect the directional motion of the water molecules. Specifically, at the same angular velocity of BPNTs, with the increase of the radius, the axial motion velocity of water molecules in the BPNT will decrease, while the force received from the BPNT will increase. The axial motion of water molecules in the double-walled BPNT is little different from that in the single-walled BPNT, which proves that the number of layers has no significant influence on the driving effect of water molecules. The influence of temperature on the motion properties of water molecules depends on the coupling effect of pressure and temperature in the tube on the convection-solid interface friction coefficient. When the temperature is lower than the normal temperature, the axial velocity of water molecules and the force exerted by the BPNT will increase with the increase of temperature, and when the temperature reaches the normal temperature, it will become stable. The results will provide a theoretical basis for the study of the flow characteristics of the fluid in BPNTs and the application of the fluid drive devices based on BPNTs.
-
Keywords:
- black phosphorus nanotubes /
- rotation /
- directional motion /
- molecular dynamics
[1] Novoselov K S, Fal'ko V I, Colombo L, Gellert P R, Schwab M G, Kim K 2012 Nature 490 192
Google Scholar
[2] Naumis G G, Barraza-Lopez S, Oliva-Leyva M, Terrones H 2017 Rep. Prog. Phys. 80 096501
Google Scholar
[3] Stampfer C, Jungen A, Linderman R, Obergfell D, Roth S, Hierold C 2006 Nano Lett. 6 1449
Google Scholar
[4] So H M, Sim J W, Kwon J, Yun J, Baik S, Chang W S 2013 Mater. Res. Bull. 48 5036
Google Scholar
[5] Cagatay E, Kohler P, Lugli P, Abdellah A 2015 IEEE Sens. J. 15 3225
Google Scholar
[6] Turlo V, Politano O, Baras F 2015 Acta Materialia. 99 363
Google Scholar
[7] Thomas J A, McGaughey A J H 2008 Nano Lett. 8 2788
Google Scholar
[8] Longhurst M J, Quirke N 2007 Nano Lett. 7 3324
Google Scholar
[9] Yang X P, Yang X N, Liu S Y 2015 Chinese. J. Chem. Eng. 23 1587
Google Scholar
[10] Zhang Z Q, Ye H F, Liu Z, Ding J N, Cheng G G, Ling Z Y, Zheng Y G, Wang L, Wang J B 2012 J. Appl. Phys. 111 114304
Google Scholar
[11] Wang L Y, Wu H A, Wang F C 2017 Sci. Rep. 7 41717
Google Scholar
[12] Lu W L, Nan H Y, Hong J H, Chen Y M, Zhu C, Liang Z, Ma X Y, Ni Z H, Jin C H, Zhang, Z 2014 Nano Res. 7 853
Google Scholar
[13] Pang J B, Bachmatiuk A, Yin Y, Trzebicka B, Zhao L, Fu L, Mendes R G, Gemming T, Liu Z F, Rummeli M H 2018 Adv. Energy Mater. 8 1702093
Google Scholar
[14] Qiao J S, Kong X H, Hu Z X, Yang F, Ji W 2014 Nat. Commun. 5 4475
Google Scholar
[15] Hu T, Han Y, Dong J M 2014 Nanotechnology 25 455703
Google Scholar
[16] Yang Z Y, Zhao J H, Wei N 2015 Appl. Phys. Lett. 107 023107
Google Scholar
[17] Zhao J L, Zhu J J, Cao R, Wang H D, Guo Z N, Sang D K, Tang J N, Fan D Y, Li J Q, Zhang H 2019 Nat. Commun. 10 4062
Google Scholar
[18] Hyun C, Kin J H, Lee J Y, Lee G H, Kim K S 2020 RSC Adv. 10 350
Google Scholar
[19] Zhang Z Q, Liu H L, Liu Z, Zhang Z, Cheng G G, Wang X D, Ding J N 2019 Appl. Surf. Sci. 475 857
Google Scholar
[20] 张忠强, 刘汉伦, 范晋伟, 丁建宁, 程广贵 2019 68 170202
Google Scholar
Zhang Z Q, Liu H L, Fan J W, Ding J N, Cheng G G 2019 Acta Phys. Sin. 68 170202
Google Scholar
[21] Cai K, Wan J, Wei N, Qin Q H 2016 Nanotechnology 27 275701
Google Scholar
[22] Hao F, Liao X B, Xiao H, Chen X 2016 Nanotechnology 27 155703
Google Scholar
[23] Fernández-Escamilla H N, Quijano-Briones J J, Tlahuice-Flores A 2016 Phys. Chem. Chem. Phys. 18 12414
Google Scholar
[24] Horn H W, Swope W C, Pitera J W, Madura J D, Dick T J, Hura G L, Head-Gordon T 2004 J. Chem. Phys. 120 9665
Google Scholar
[25] Zhang H W, Ye H F, Zheng Y G, Zhang Z Q 2011 Microfluid. Nanofluid. 10 403
Google Scholar
[26] Cai K, Liu L, Shi J, Qin Q H 2017 Mater. Des. 121 406
Google Scholar
[27] Ryckaert J P, Ciccotti G, Berendsen H J C 1977 J. Comput. Phys. 23 327
Google Scholar
[28] Hou Q W, Cao B Y, Guo Z Y 2009 Nanotechnology 20 495503
Google Scholar
-
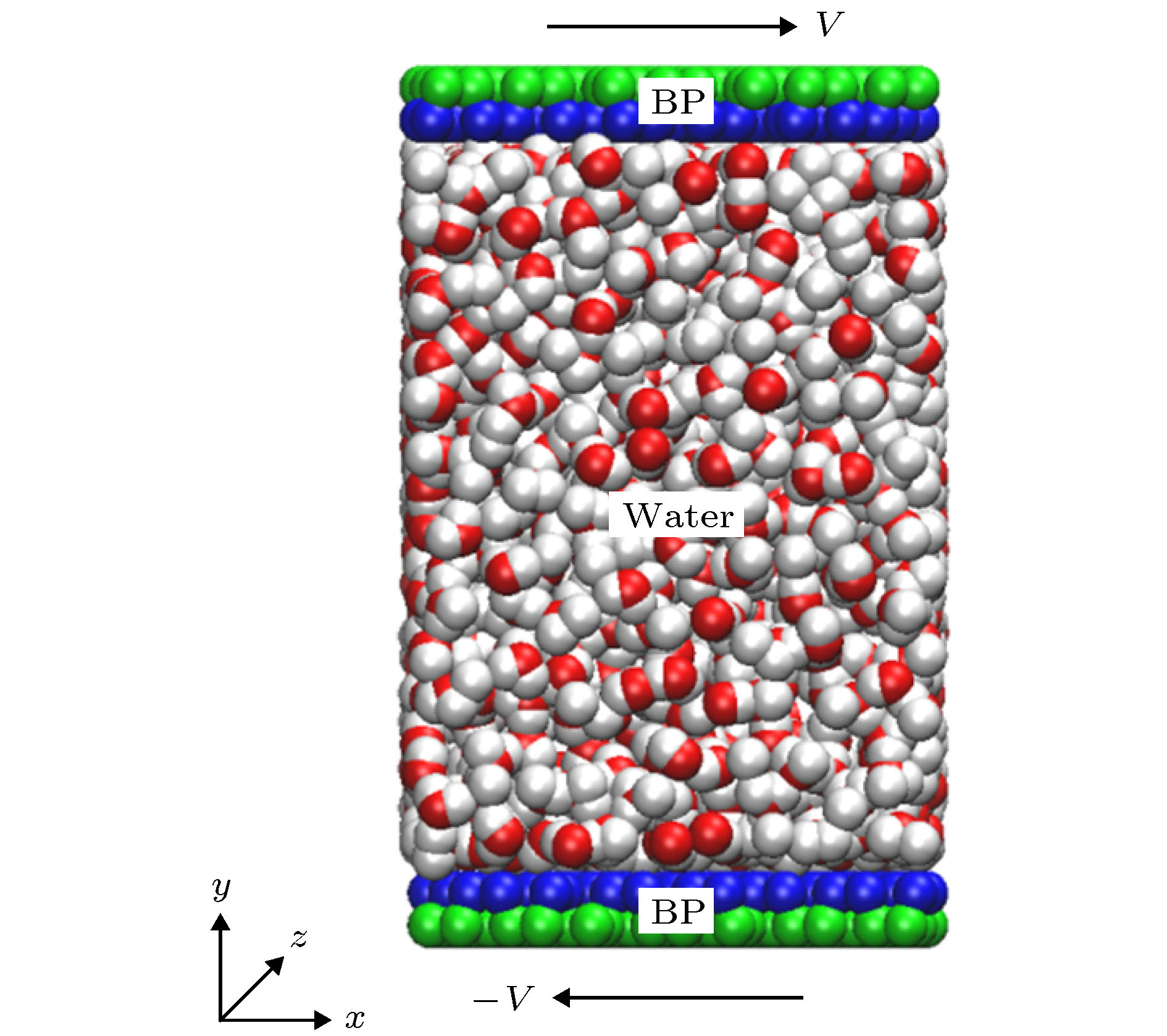
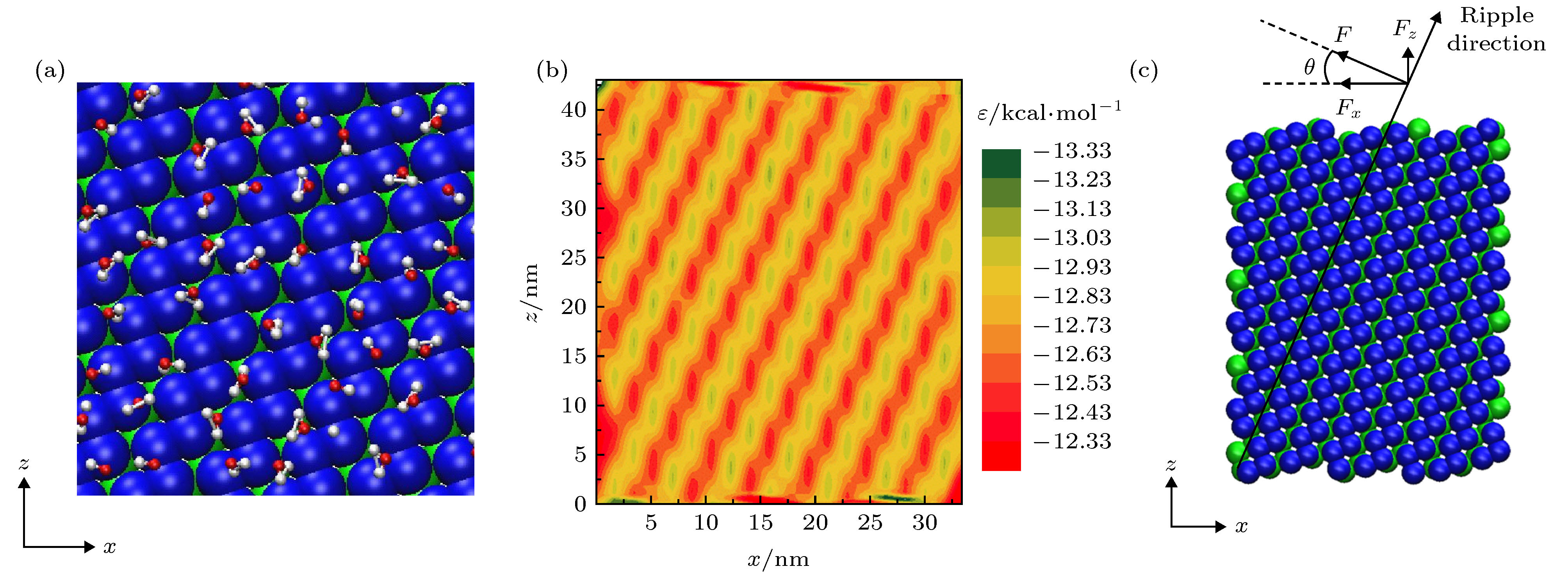
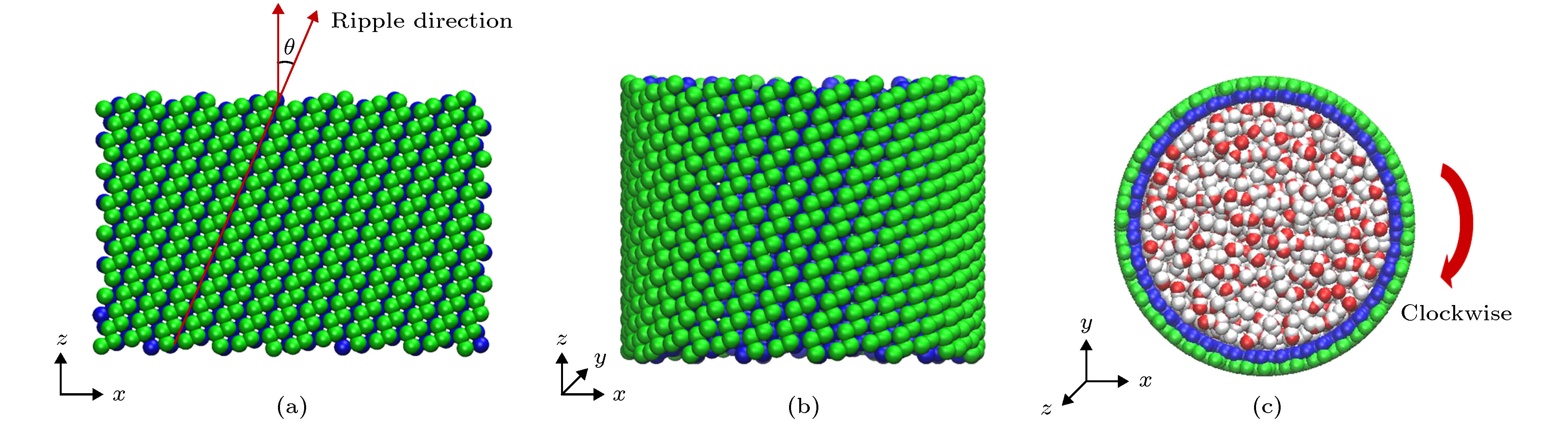
图 1 (a)单层黑磷模型, 其中手性角度θ指黑磷褶皱方向与z轴方向(纳米管轴向)的夹角; (b)手性角度为23.4°的黑磷纳米管; (c)填充水分子的黑磷纳米管旋转模型图
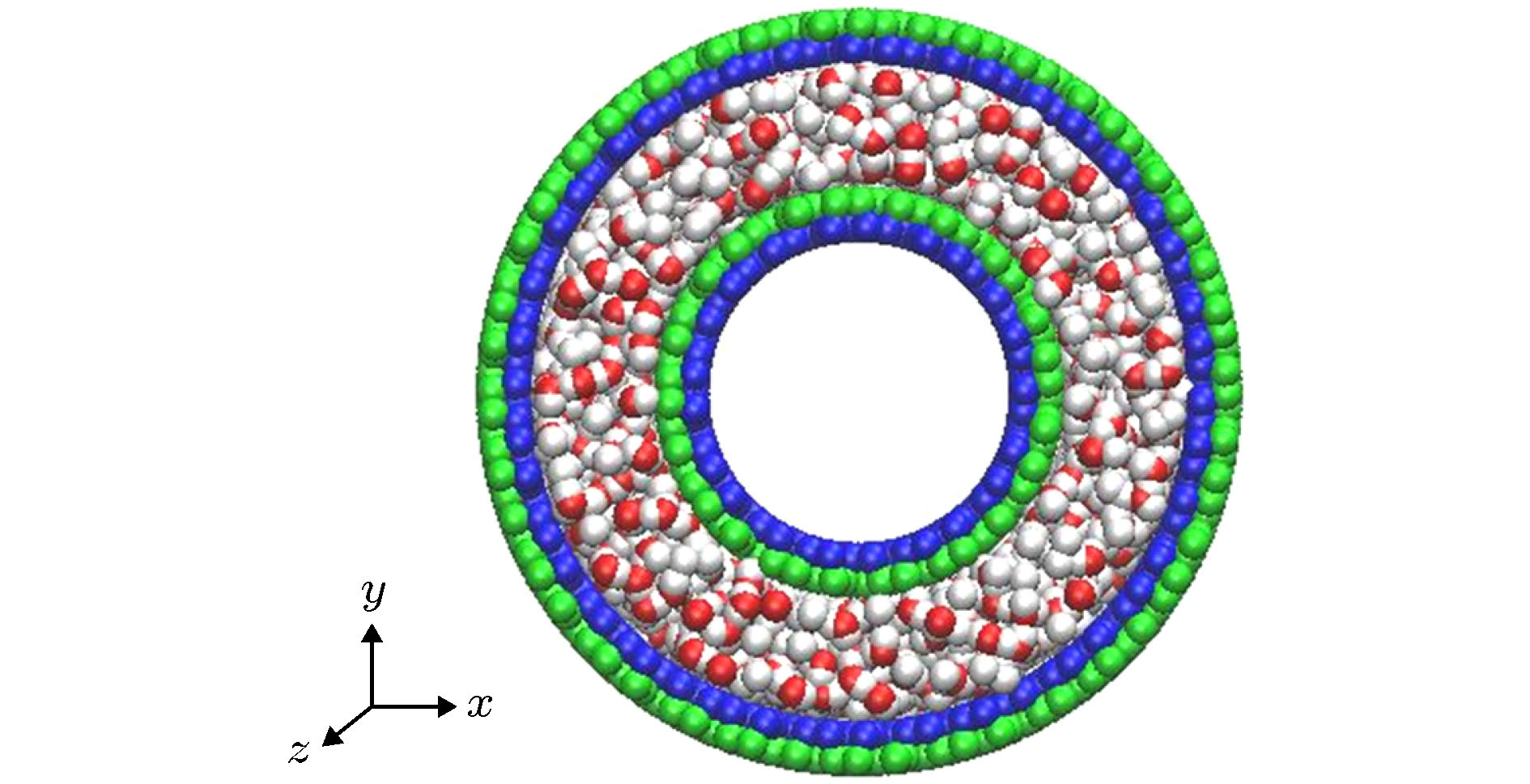
Fig. 1. (a) Monolayer black phosphorus model, chiral angle θ is the intersection angle between the ripple direction of BP monolayer and z direction (the axial direction of the BPNT); (b) BPNT with a chiral angle of 23.4°; (c) model of the rotating BPNT filled with water molecules.
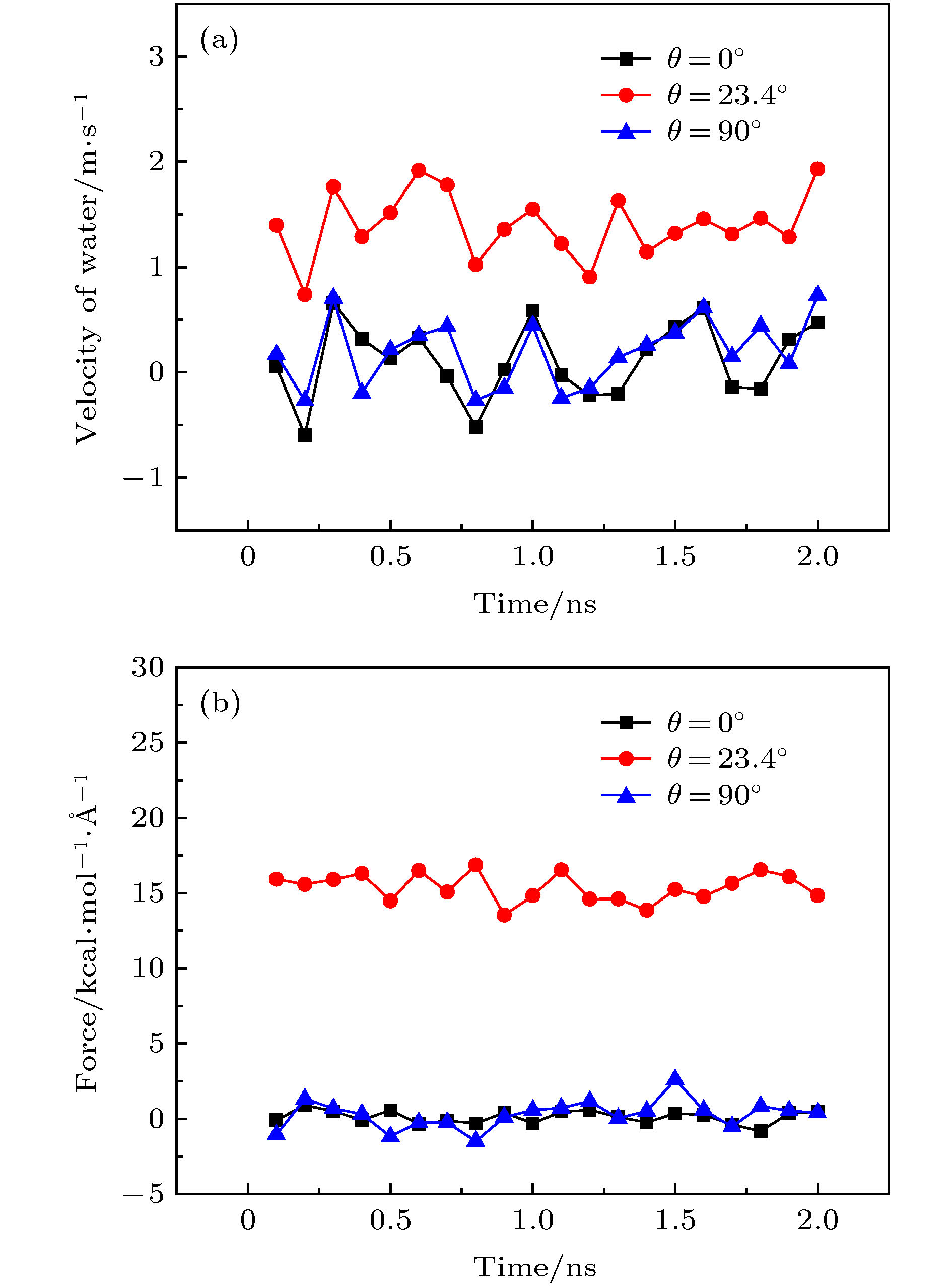
图 2 不同手性角度的黑磷纳米管以50 rad/ns的转速顺时针旋转时管内水分子沿轴线方向的(a)速度和(b)受力随时间的变化关系
Fig. 2. For the angular velocity of the BPNT being 50 rad/ns, (a) the velocity in the axial direction of water molecules in BPNTs and (b) the resultant force in the axial direction of water molecules received from BPNTs with different chiral angles as a function of time.
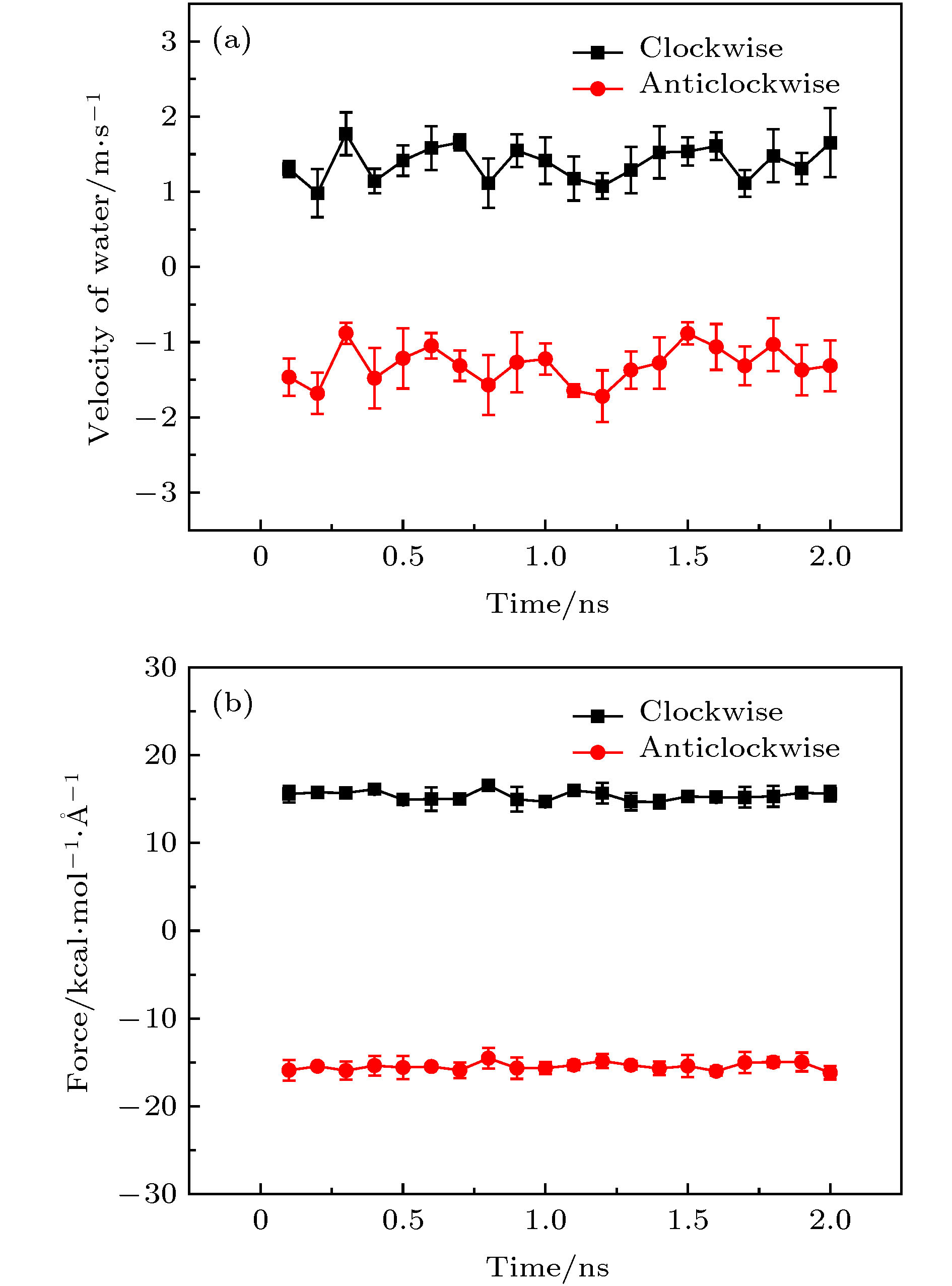
图 3 手性角度为23.4°的黑磷纳米管以50 rad/ns的转速沿不同方向旋转时管内水分子沿轴向的(a)速度和(b)受力随时间的变化关系
Fig. 3. For the angular velocity of the BPNT being 50 rad/ns in different directions of rotation, (a) the velocity in the axial direction of water molecules in the BPNT and (b) the resultant force in the axial direction of water molecules received from the BPNT as a function of time when the chiral angle is 23.4°.
图 4 手性角度为23.4°时, 黑磷纳米管内水分子的轴向速度与受力随纳米管转动速度的变化关系
Fig. 4. The velocity in the axial direction of water molecules in the BPNT and the resultant force in the axial dire-ction of water molecules received from the BPNT as a function of the angular velocity of the BPNT when the chiral angle is 23.4°.
图 5 黑磷纳米通道内水分子的Couette流模型图
Fig. 5. Couette flow model diagram of water molecules flowing in BP nanochannel.
图 6 黑磷纳米通道宽度方向上水分子的速度分布
Fig. 6. Velocity distribution of water molecules along the width of the BP nanochannel.
图 7 (a)水分子的边界滑移速度和(b)剪切应力随剪切应变率的变化关系
Fig. 7. (a) The boundary slip velocity of water molecules and (b) the shear stress as a function of the shear strain rate.
图 8 (a)水分子边界处的微观构型图; (b)黑磷-水交互界面势能分布图; (c)黑磷对水分子的作用力示意图
Fig. 8. (a) Microstructure of the boundary of water molecules; (b) potential energy distribution cloud diagram of BP-water; (c) schematic diagram of the force of BP on water molecules.
图 10 3种情形下黑磷纳米管间水分子沿轴线方向的(a)速度和(b)受力随转速的变化关系
Fig. 10. (a) The velocity in the axial direction of water molecules between BPNTs and (b) the resultant force in the axial direction of water molecules received from BPNTs as a function of the angular velocity of BPNTs in three cases.
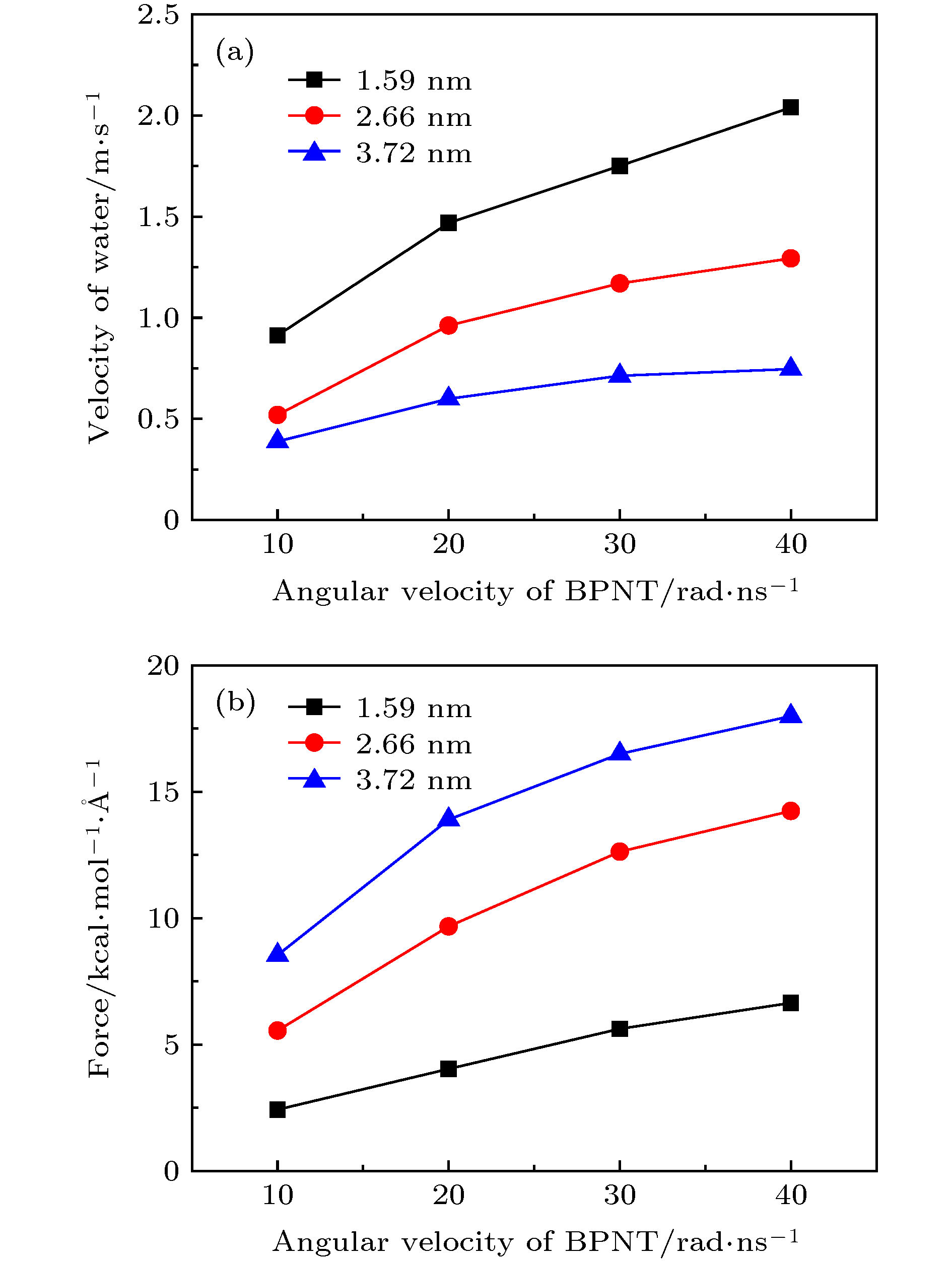
图 11 手性角度为23.4°时, 不同半径的黑磷纳米管内水分子沿轴线方向的(a)速度和(b)受力随黑磷纳米管转速的变化关系
Fig. 11. For different radius, (a) the velocity in the axial direction of water molecules in BPNTs and (b) the resultant force in the axial direction of water molecules received from BPNTs as a function of the angular velocity of BPNTs when the chiral angle is 23.4°.
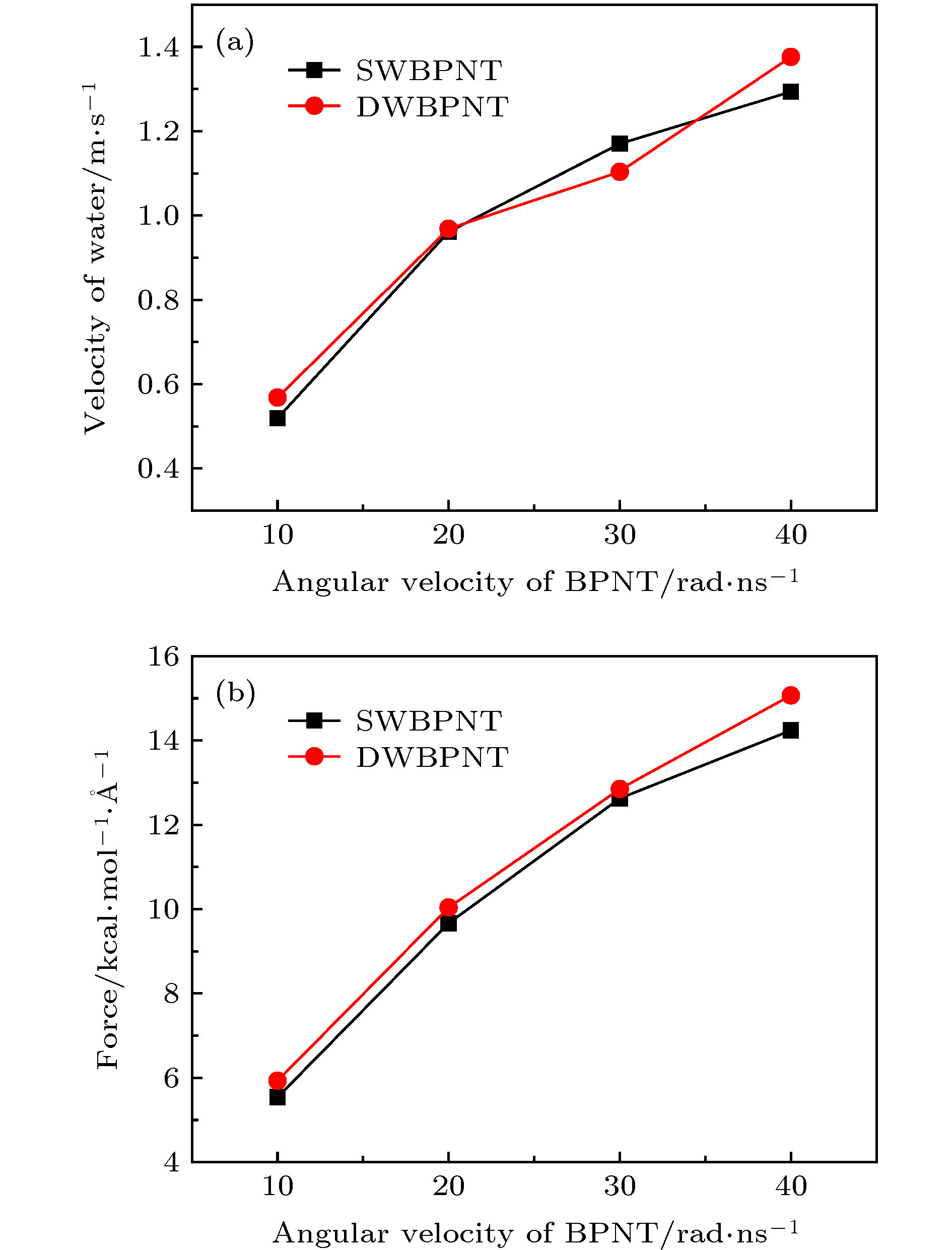
图 12 手性角度为23.4°时, 不同层数黑磷纳米管内水分子沿轴线方向的(a)速度和(b)受力随黑磷纳米管转速的变化关系
Fig. 12. For different layers, (a) the velocity in the axial direction of water molecules in BPNTs and (b) the resultant force in the axial direction of water molecules received from BPNTs as a function of the angular velocity of BPNTs when the chiral angle is 23.4°.
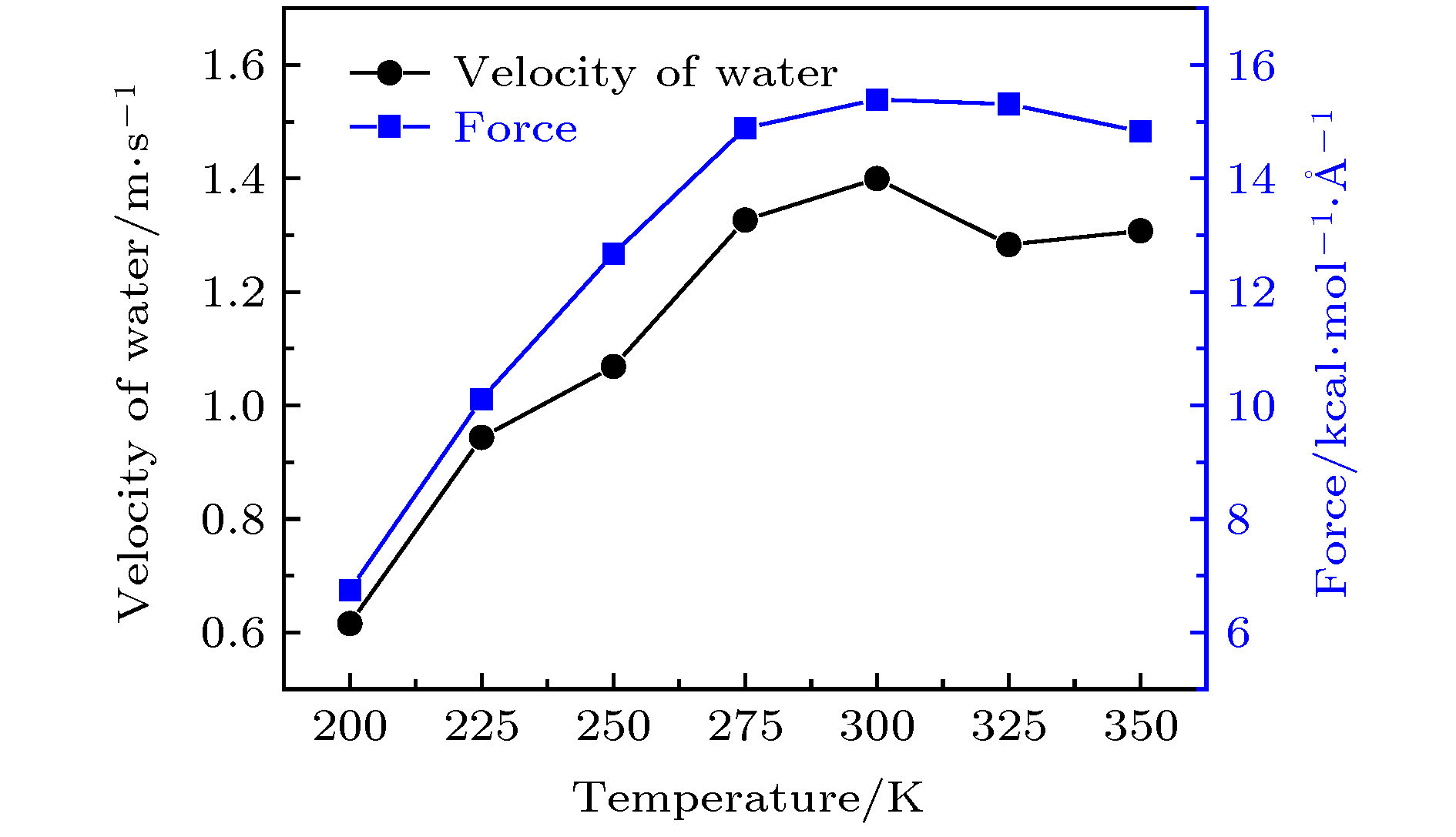
图 13 转速为50 rad/ns时, 手性角度为23.4°的黑磷纳米管内水分子的轴向速度与受力随温度的变化关系
Fig. 13. For the angular velocity of the BPNT being 50 rad/ns, the velocity in the axial direction of water molecules in the BPNT and the resultant force in the axial direction of water molecules received from the BPNT as a function of the temperature when the chiral angle is 23.4°.
表 1 LJ势能函数的参数值
Table 1. Parameter values of LJ potential function
Atoms ε/kcal·mol–1 σ/Å P—P 0.36760 3.4380 O—O 0.16275 3.16435 P—O 0.24460 3.30120 
下载: 导出CSV
-
[1] Novoselov K S, Fal'ko V I, Colombo L, Gellert P R, Schwab M G, Kim K 2012 Nature 490 192
Google Scholar
[2] Naumis G G, Barraza-Lopez S, Oliva-Leyva M, Terrones H 2017 Rep. Prog. Phys. 80 096501
Google Scholar
[3] Stampfer C, Jungen A, Linderman R, Obergfell D, Roth S, Hierold C 2006 Nano Lett. 6 1449
Google Scholar
[4] So H M, Sim J W, Kwon J, Yun J, Baik S, Chang W S 2013 Mater. Res. Bull. 48 5036
Google Scholar
[5] Cagatay E, Kohler P, Lugli P, Abdellah A 2015 IEEE Sens. J. 15 3225
Google Scholar
[6] Turlo V, Politano O, Baras F 2015 Acta Materialia. 99 363
Google Scholar
[7] Thomas J A, McGaughey A J H 2008 Nano Lett. 8 2788
Google Scholar
[8] Longhurst M J, Quirke N 2007 Nano Lett. 7 3324
Google Scholar
[9] Yang X P, Yang X N, Liu S Y 2015 Chinese. J. Chem. Eng. 23 1587
Google Scholar
[10] Zhang Z Q, Ye H F, Liu Z, Ding J N, Cheng G G, Ling Z Y, Zheng Y G, Wang L, Wang J B 2012 J. Appl. Phys. 111 114304
Google Scholar
[11] Wang L Y, Wu H A, Wang F C 2017 Sci. Rep. 7 41717
Google Scholar
[12] Lu W L, Nan H Y, Hong J H, Chen Y M, Zhu C, Liang Z, Ma X Y, Ni Z H, Jin C H, Zhang, Z 2014 Nano Res. 7 853
Google Scholar
[13] Pang J B, Bachmatiuk A, Yin Y, Trzebicka B, Zhao L, Fu L, Mendes R G, Gemming T, Liu Z F, Rummeli M H 2018 Adv. Energy Mater. 8 1702093
Google Scholar
[14] Qiao J S, Kong X H, Hu Z X, Yang F, Ji W 2014 Nat. Commun. 5 4475
Google Scholar
[15] Hu T, Han Y, Dong J M 2014 Nanotechnology 25 455703
Google Scholar
[16] Yang Z Y, Zhao J H, Wei N 2015 Appl. Phys. Lett. 107 023107
Google Scholar
[17] Zhao J L, Zhu J J, Cao R, Wang H D, Guo Z N, Sang D K, Tang J N, Fan D Y, Li J Q, Zhang H 2019 Nat. Commun. 10 4062
Google Scholar
[18] Hyun C, Kin J H, Lee J Y, Lee G H, Kim K S 2020 RSC Adv. 10 350
Google Scholar
[19] Zhang Z Q, Liu H L, Liu Z, Zhang Z, Cheng G G, Wang X D, Ding J N 2019 Appl. Surf. Sci. 475 857
Google Scholar
[20] 张忠强, 刘汉伦, 范晋伟, 丁建宁, 程广贵 2019 68 170202
Google Scholar
Zhang Z Q, Liu H L, Fan J W, Ding J N, Cheng G G 2019 Acta Phys. Sin. 68 170202
Google Scholar
[21] Cai K, Wan J, Wei N, Qin Q H 2016 Nanotechnology 27 275701
Google Scholar
[22] Hao F, Liao X B, Xiao H, Chen X 2016 Nanotechnology 27 155703
Google Scholar
[23] Fernández-Escamilla H N, Quijano-Briones J J, Tlahuice-Flores A 2016 Phys. Chem. Chem. Phys. 18 12414
Google Scholar
[24] Horn H W, Swope W C, Pitera J W, Madura J D, Dick T J, Hura G L, Head-Gordon T 2004 J. Chem. Phys. 120 9665
Google Scholar
[25] Zhang H W, Ye H F, Zheng Y G, Zhang Z Q 2011 Microfluid. Nanofluid. 10 403
Google Scholar
[26] Cai K, Liu L, Shi J, Qin Q H 2017 Mater. Des. 121 406
Google Scholar
[27] Ryckaert J P, Ciccotti G, Berendsen H J C 1977 J. Comput. Phys. 23 327
Google Scholar
[28] Hou Q W, Cao B Y, Guo Z Y 2009 Nanotechnology 20 495503
Google Scholar
下载:
计量
- 文章访问数: 6360
- PDF下载量: 82
- 被引次数: 0
