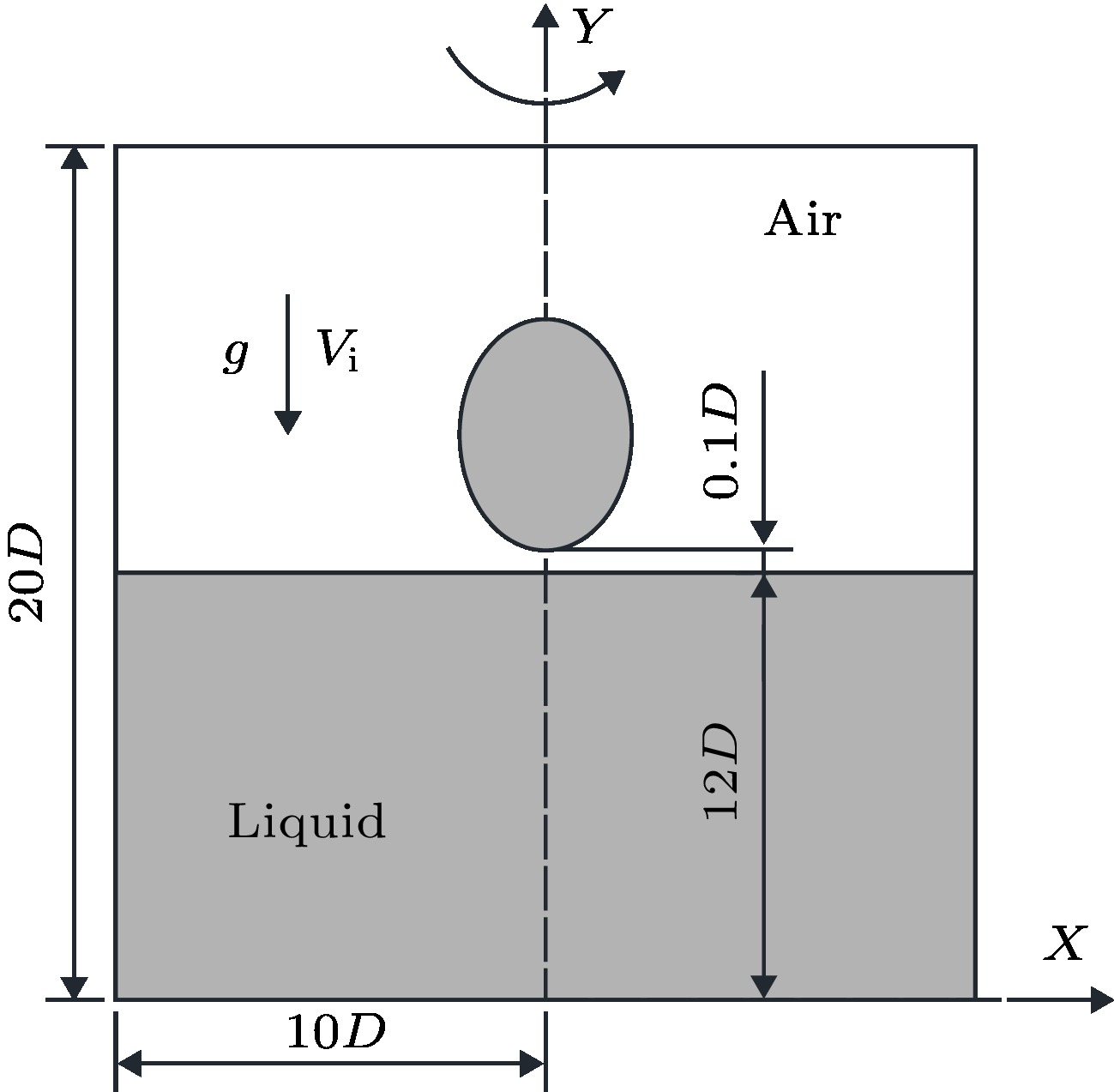
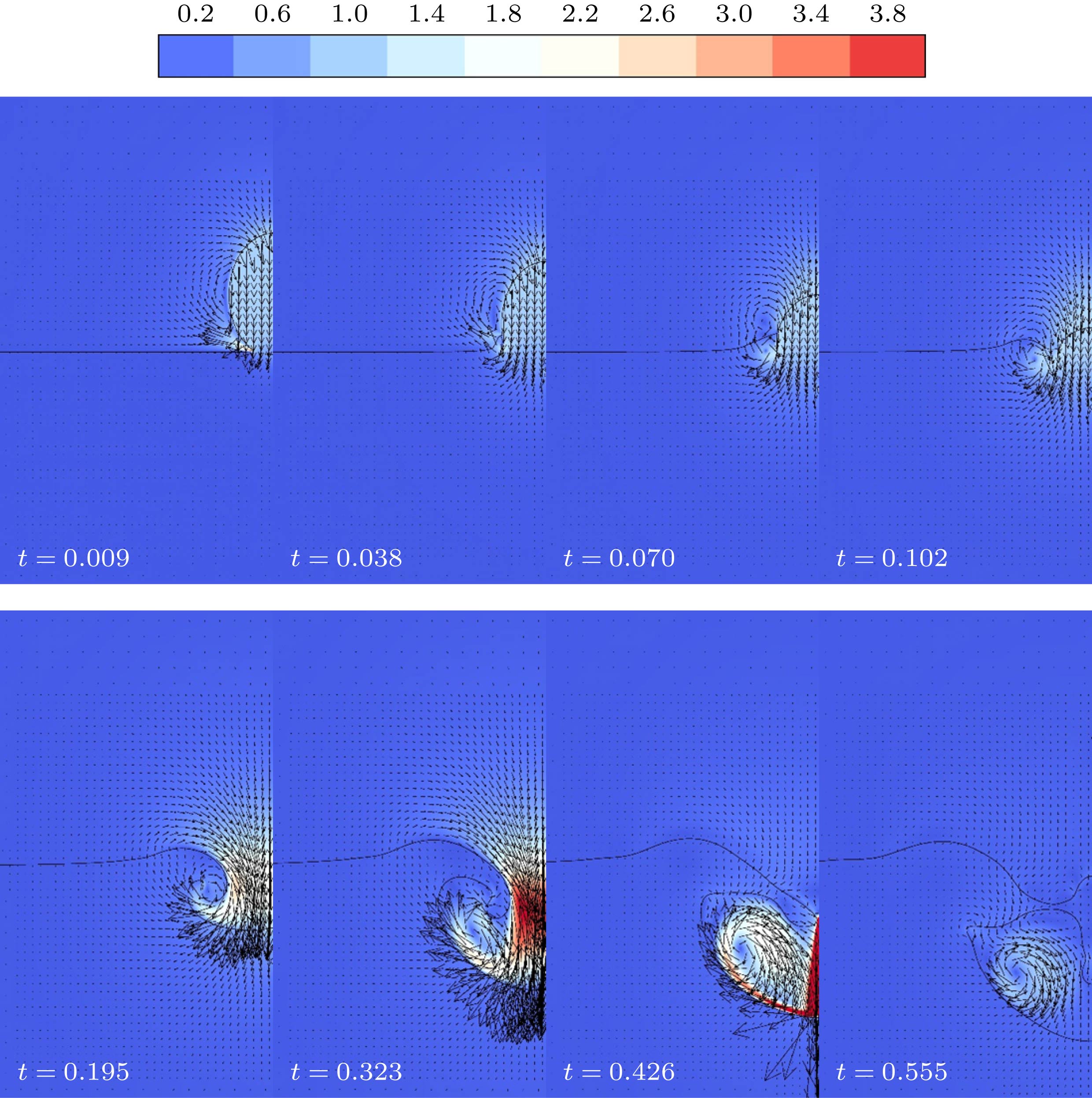
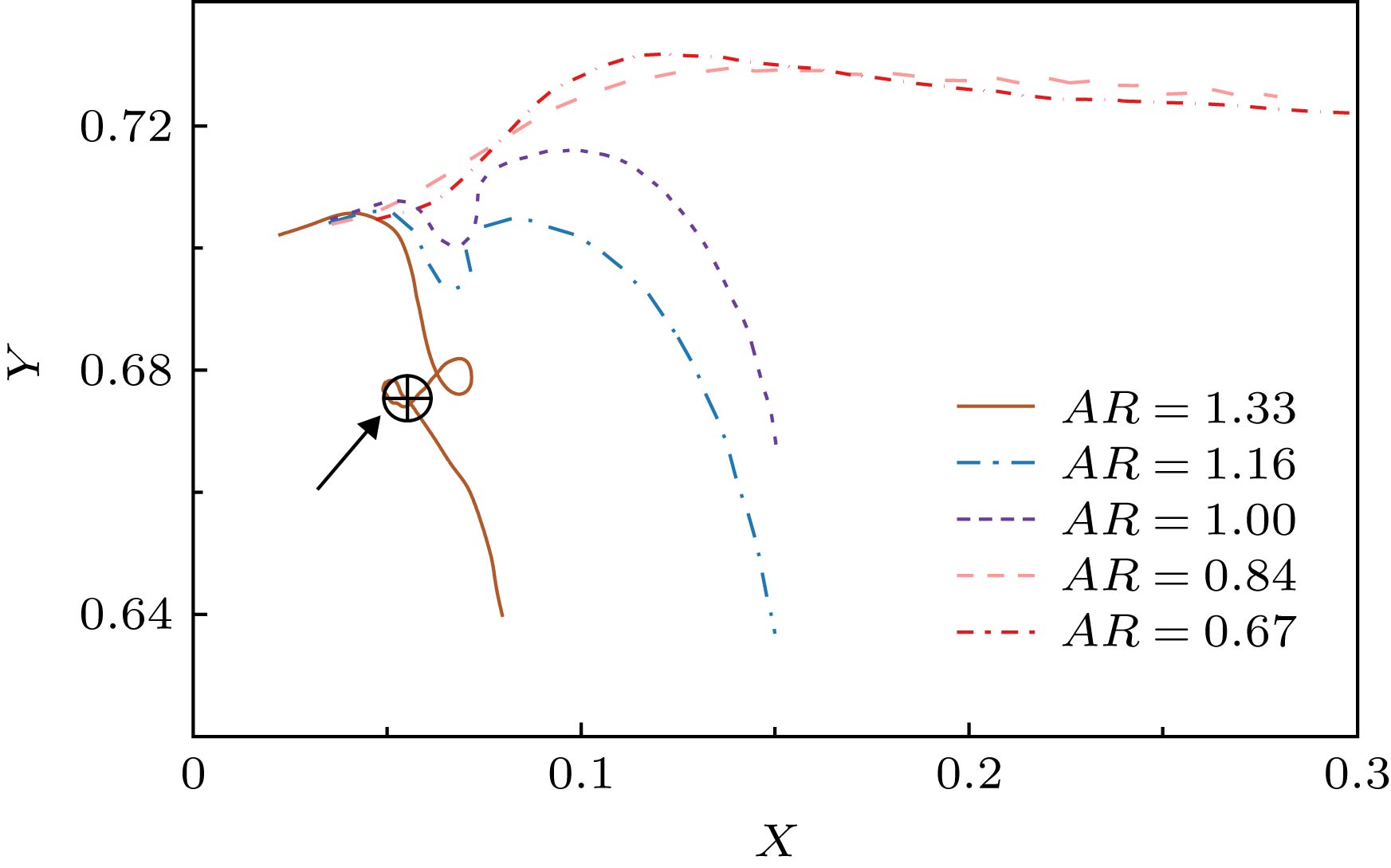
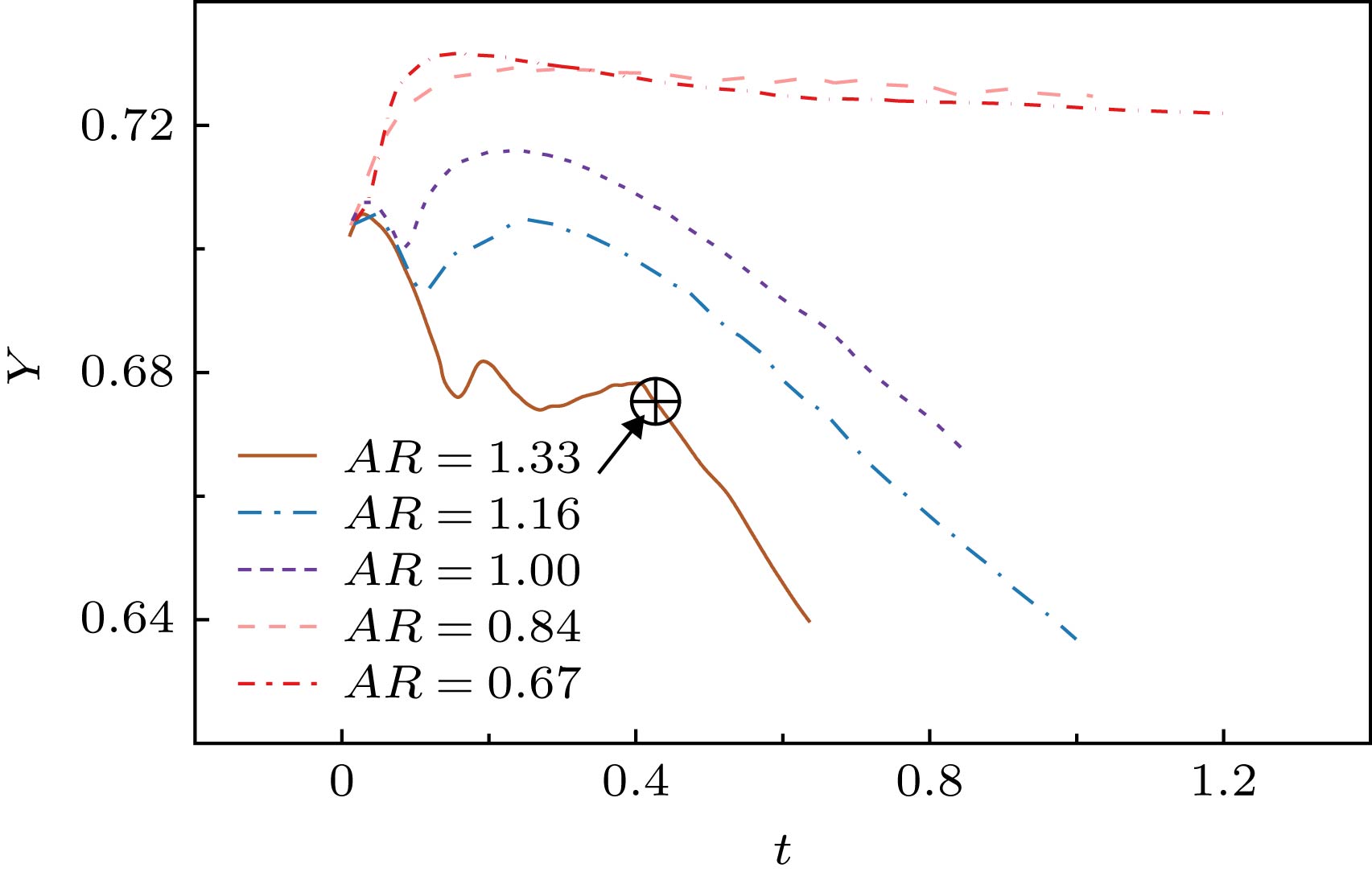
-
在微小水滴撞击深水液池运动中, 水滴在下降过程中产生的外形振荡对后续空腔产生及气泡夹带有极大影响. 因此, 本文假定5种不同宽高比(AR)的微小变形水滴, 采用自适应网格技术和体积函数方法对其运动过程进行数值模拟, 并详细探究不同撞击速度和水滴形变对撞击后空腔变形坍缩过程、涡环的发展以及气泡夹带的影响. 研究结果表明, 在较高撞击速度下(Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s), AR = 1.33下的长椭圆形变水滴与液池聚合并产生大型气泡夹带. 大型气泡截留过程主要由水滴撞击时颈部自由面下产生的涡环控制, 涡环裹挟自由界面形成滚动射流, 最终射流接触夹带大型气泡. 在气泡截留后期, 腔内气旋推动侧壁向外拓展, 有效增大了气泡体积. 在撞击初期阶段, 液滴形状越扁, 水滴与液池颈部射流曲率越大, 生成涡环强度越大. 但扁椭圆水滴生成涡环距离自由面过近, 界面的早期拉动破坏了涡环强度, 因此涡环衰减也相对较快.Water droplet impacting into a deep liquid pool is one of the most well-known flow phenomena in fluid mechanics. As a ubiquitous natural aeration process, the coalescence of water droplets in lakes and ponds and the subsequent bubble entrapment are one of the most notable ways of gas-liquid exchange in nature, and it is of great significance for underwater sound transmission, aquatic ecosystems and chemical process. The shape of an oscillating droplet in impact under different surrounding medium and initial condition is a key factor for the subsequent cavity formation and bubble entrapment. In this study, the adaptive mesh refinement technique and volume of fluid (VOF) method are applied to the study of the water droplet impact phenomena. Five kinds of deformed micron water droplets with different aspect ratios and impact velocities of 4 m/s and 6 m/s are selected to investigate the influences of drop deformation and impact velocity on the bubble entrapment, capillary wave propagation, and vortex ring evolution. The results show that at low impact velocities (Fr = 75, We = 64.4, Re = 1160, Vi = 4 m/s), the shape of water droplet does not cause the cavity formation and bubble entrapment to change significantly. However, under higher impact velocity (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s), deformed droplet with an aspect ratio of 1.33 coalesces with the pool, and large bubble entrainment occurs. The large bubble entrapment is affected mainly by the vortex ring generated under the free surface at the neck between the droplet and the pool. The vortex ring penetrates more deeply before it pulls the free surface to generate a rolling jet at the upper interface of the cavity. The rolling jets then contact the center of the cavity and collapse to entrain a large bubble. At the end of the bubble entrapment phenomenon, the cyclone inside the cavity pushes the sidewall of the cavity continuously, and effectively increases the lateral volume of the bubble, which plays a vital role in the bubble entrainment process. In the initial stage of the impact, the flatter the shape of the droplet, the greater the curvature of the jet generated on the neck between the droplet and the pool, the greater the strength of the vortex ring generated under the free surface. However, the vortex ring formed by the oblate-shaped water droplet is generated too close to the free surface, and the early free surface pulling reduces the strength of the vortex ring, thus the vorticity maximum value decays relatively fast.
-
Keywords:
- water droplet /
- deep water pool /
- bubble entrapment
[1] Yarin A L 2006 Annu. Rev. Fluid Mech. 38 159
Google Scholar
[2] Prosperetti A, Oğuz H N 1993 Annu. Rev. Fluid Mech. 25 577
Google Scholar
[3] Jayaratne O W, Mason B J 1964 Proc. R. Soc. London Ser. A-Math. Phys. Eng. Sci. 280 545
Google Scholar
[4] Michon G J, Josserand C, Séon T 2017 Phys. Rev. Fluids 2 023601
Google Scholar
[5] 董琪琪, 胡海豹, 陈少强, 何强, 鲍路瑶 2018 67 054702
Google Scholar
Dong Q Q, Hu H B, Chen S Q, He Q, Bao L Y 2018 Acta Phys. Sin. 67 054702
Google Scholar
[6] 黄虎, 洪宁, 梁宏, 施保昌, 柴振华 2016 65 084702
Google Scholar
Huang H, Hong N, Liang H, Shi B C, Chai Z H 2016 Acta Phys. Sin. 65 084702
Google Scholar
[7] Wang A B, Kuan C C, Tsai P H 2013 Phys. Fluids 25 101702
Google Scholar
[8] Blanchette F, Bigioni T P 2009 J. Fluid Mech. 620 333
Google Scholar
[9] Worthington A M 1908 A Study of Splashes (London: Longmans, Green) pp129–132
[10] Thoroddsen S T, Takehara K, Nguyen H D, Etoh T G 2018 J. Fluid Mech. 848 R3
Google Scholar
[11] Deka H, Ray B, Biswas G, Dalal A, Tsai P H, Wang A B 2017 Phys. Fluids 29 09210
[12] Bouwhuis W, Hendrix M H, van der Meer D, Snoeijer J H 2015 J. Fluid Mech. 771 503
Google Scholar
[13] Pumphrey H C, Elmore P A 1990 J. Fluid Mech. 220 539
Google Scholar
[14] Oğuz H N, Prosperetti A 1990 J. Fluid Mech. 219 143
Google Scholar
[15] Oğuz H N, Prosperetti A 1989 J. Fluid Mech. 203 149
Google Scholar
[16] Oğuz H N, Prosperetti A 1991 J. Fluid Mech. 228 417
[17] Prosperetti A 1988 J. Acoust. Soc. Am. 84 1042
Google Scholar
[18] Deng Q, Anilkumar A V, Wang T G 2007 J. Fluid Mech. 578 119
Google Scholar
[19] Chen S, Guo L 2014 Chem. Eng. Sci. 109 1
Google Scholar
[20] Volkov R S, Kuznetsov G V, Strizhak P A 2015 Int. J. Heat Mass Transf. 85 1
Google Scholar
[21] Strizhak P A 2013 J. Eng. Phys. Thermophys. 86 895
Google Scholar
[22] Thokchom A K, Gupta A, Jaijus P J, Singh A 2014 Int. J. Heat Mass Transf. 68 67
Google Scholar
[23] Varaksin A Y 2013 High Temp. 51 377
Google Scholar
[24] Volkov R S, Vysokomornaya O V, Kuznestov G V, Strizhak P A 2013 J. Eng. Phys. Thermophys. 86 1413
Google Scholar
[25] Wang C Y, Zhang C B, Huang X Y, Liu X D, Chen Y P 2016 Chin. Phys. B 25 108202
Google Scholar
[26] 丁思源, 王瑞祥, 徐荣吉, 张一灏, 蔡骥驰 2016 化工学报 67 2495
Ding C Y, Wang R X, Xu R J, Zhang Y H, Cai J C 2016 CIESC Journal 67 2495
[27] Roman B, Bico J 2010 J. Phys.Condes. Matter 22 493101
Google Scholar
[28] 裴传康, 魏炳乾 2018 67 224703
Google Scholar
Pei C K, Wei B Q 2018 Acta Phys. Sin. 67 224703
Google Scholar
[29] Popinet S 2003 J. Comput. Phys. 190 572
Google Scholar
[30] Popinet S 2009 J. Comput. Phys. 228 5838
Google Scholar
[31] Thoraval M J, Li Y, Thoroddsen S T 2016 Phys. Rev. E 93 033128
-
图 4 数值模拟自由液面随时间运动过程
Fig. 4. Numerical simulation of the free surface movement at selected times.
图 5 不同工况下自由液面随时间的运动过程(Fr = 75, We = 64.4, Re = 1160, Vi = 4 m/s) (a) AR = 1.16; (b) AR = 1.00; (c) AR = 0.84
Fig. 5. Free surface profiles with simulated at selected times (Fr = 75, We = 64.4, Re = 1160, Vi = 4 m/s): (a) AR = 1.16; (b) AR = 1.00; (c) AR = 0.84.
图 6 不同工况下自由液面随时间的运动过程 (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s) (a) AR = 1.33; (b) AR = 1.16; (c) AR = 1.00; (d) AR = 0.84; (e) AR = 0.67
Fig. 6. Free surface profiles simulated at selected times (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s): (a) AR = 1.33; (b) AR = 1.16; (c) AR = 1.00; (d) AR = 0.84; (e) AR = 0.67.
图 7 不同时间节点下长椭圆形变水滴撞击液池涡量场和压力场等值线图(Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s, AR = 1.33)
Fig. 7. Vorticity and pressure contours of a prolate water droplet impacting into a water pool at selected times (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s, AR = 1.33).
图 8 不同时间节点下长椭圆形变水滴撞击液池速度矢量场 (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s, AR = 1.33)
Fig. 8. Velocity field of a prolate water droplet impacting into a water pool at selected times (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s, AR = 1.33).
图 9 自由表面下涡环的最大涡量随时间变化 (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s)
Fig. 9. Vorticity maximum of the vortex ring generated under the free surface with time under different cases (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s).
图 10 自由表面下涡环的最大涡量位置随时间变化(Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s)
Fig. 10. Vorticity maximum location of the vortex ring generated under the free surface with time under different cases (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s).
图 11 自由表面下涡环的最大涡量横向位置随时间变化 (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s)
Fig. 11. Lateral position of the vorticity maximum in the vortex ring generated under the free surface with time under different cases (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s).
图 12 自由表面下涡环的最大涡量垂向位置随时间变化(Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s)
Fig. 12. Vertical position of the vorticity maximum in the vortex ring generated under the free surface with time under different cases (Fr = 112.5, We = 145, Re = 1740, Vi = 6 m/s).
-
[1] Yarin A L 2006 Annu. Rev. Fluid Mech. 38 159
Google Scholar
[2] Prosperetti A, Oğuz H N 1993 Annu. Rev. Fluid Mech. 25 577
Google Scholar
[3] Jayaratne O W, Mason B J 1964 Proc. R. Soc. London Ser. A-Math. Phys. Eng. Sci. 280 545
Google Scholar
[4] Michon G J, Josserand C, Séon T 2017 Phys. Rev. Fluids 2 023601
Google Scholar
[5] 董琪琪, 胡海豹, 陈少强, 何强, 鲍路瑶 2018 67 054702
Google Scholar
Dong Q Q, Hu H B, Chen S Q, He Q, Bao L Y 2018 Acta Phys. Sin. 67 054702
Google Scholar
[6] 黄虎, 洪宁, 梁宏, 施保昌, 柴振华 2016 65 084702
Google Scholar
Huang H, Hong N, Liang H, Shi B C, Chai Z H 2016 Acta Phys. Sin. 65 084702
Google Scholar
[7] Wang A B, Kuan C C, Tsai P H 2013 Phys. Fluids 25 101702
Google Scholar
[8] Blanchette F, Bigioni T P 2009 J. Fluid Mech. 620 333
Google Scholar
[9] Worthington A M 1908 A Study of Splashes (London: Longmans, Green) pp129–132
[10] Thoroddsen S T, Takehara K, Nguyen H D, Etoh T G 2018 J. Fluid Mech. 848 R3
Google Scholar
[11] Deka H, Ray B, Biswas G, Dalal A, Tsai P H, Wang A B 2017 Phys. Fluids 29 09210
[12] Bouwhuis W, Hendrix M H, van der Meer D, Snoeijer J H 2015 J. Fluid Mech. 771 503
Google Scholar
[13] Pumphrey H C, Elmore P A 1990 J. Fluid Mech. 220 539
Google Scholar
[14] Oğuz H N, Prosperetti A 1990 J. Fluid Mech. 219 143
Google Scholar
[15] Oğuz H N, Prosperetti A 1989 J. Fluid Mech. 203 149
Google Scholar
[16] Oğuz H N, Prosperetti A 1991 J. Fluid Mech. 228 417
[17] Prosperetti A 1988 J. Acoust. Soc. Am. 84 1042
Google Scholar
[18] Deng Q, Anilkumar A V, Wang T G 2007 J. Fluid Mech. 578 119
Google Scholar
[19] Chen S, Guo L 2014 Chem. Eng. Sci. 109 1
Google Scholar
[20] Volkov R S, Kuznetsov G V, Strizhak P A 2015 Int. J. Heat Mass Transf. 85 1
Google Scholar
[21] Strizhak P A 2013 J. Eng. Phys. Thermophys. 86 895
Google Scholar
[22] Thokchom A K, Gupta A, Jaijus P J, Singh A 2014 Int. J. Heat Mass Transf. 68 67
Google Scholar
[23] Varaksin A Y 2013 High Temp. 51 377
Google Scholar
[24] Volkov R S, Vysokomornaya O V, Kuznestov G V, Strizhak P A 2013 J. Eng. Phys. Thermophys. 86 1413
Google Scholar
[25] Wang C Y, Zhang C B, Huang X Y, Liu X D, Chen Y P 2016 Chin. Phys. B 25 108202
Google Scholar
[26] 丁思源, 王瑞祥, 徐荣吉, 张一灏, 蔡骥驰 2016 化工学报 67 2495
Ding C Y, Wang R X, Xu R J, Zhang Y H, Cai J C 2016 CIESC Journal 67 2495
[27] Roman B, Bico J 2010 J. Phys.Condes. Matter 22 493101
Google Scholar
[28] 裴传康, 魏炳乾 2018 67 224703
Google Scholar
Pei C K, Wei B Q 2018 Acta Phys. Sin. 67 224703
Google Scholar
[29] Popinet S 2003 J. Comput. Phys. 190 572
Google Scholar
[30] Popinet S 2009 J. Comput. Phys. 228 5838
Google Scholar
[31] Thoraval M J, Li Y, Thoroddsen S T 2016 Phys. Rev. E 93 033128
下载:
计量
- 文章访问数: 8601
- PDF下载量: 89
- 被引次数: 0
