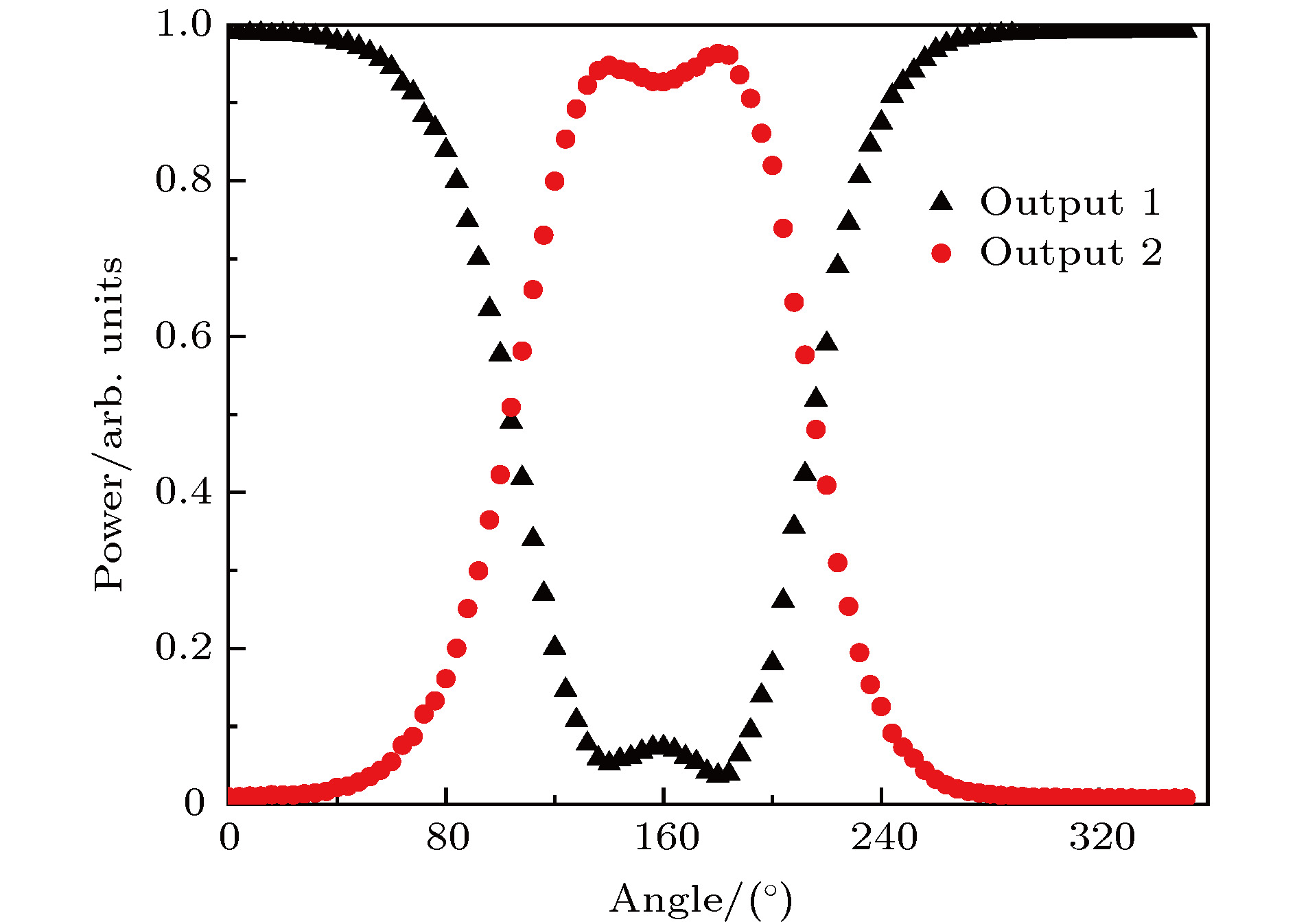
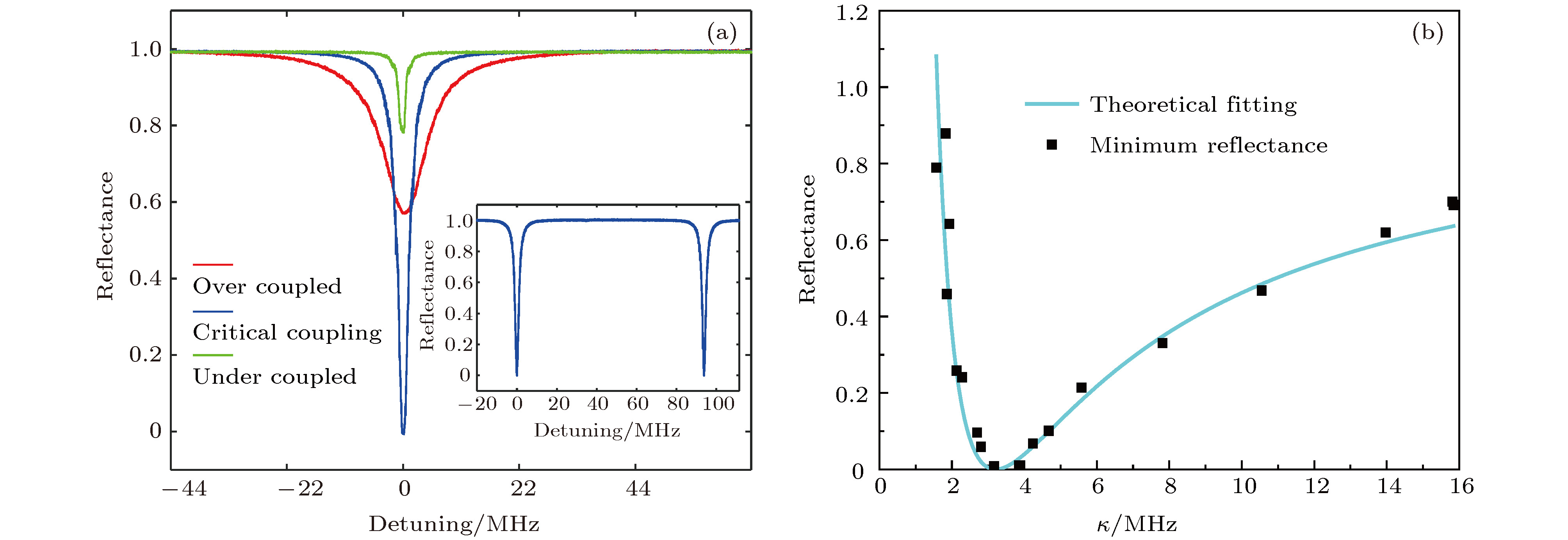
-
基于可调分束比的光纤分束器, 制作了光纤环形谐振腔并通过调节分束比实现了对光纤环形谐振腔的欠耦合、临界耦合和过耦合的状态控制. 实验测量了腔最小反射率与腔损耗之间的关系, 获得光纤环形谐振腔的腔内衰减率为
${\kappa _0}{\rm{ = }}2{\text{π}} \times \left( {1.60 \pm 0.03} \right)\;{\rm{ MHz}}$ , 品质因子为$Q = \left( {1.10 \pm 0.02} \right) \times {10.8}$ . 在此基础上, 结合了压电陶瓷拉伸光纤以控制腔长和Pound-Drever-Hall锁频两大技术优势, 克服了之前温度反馈控制等方法的反馈带宽窄、噪声大和稳定性差等问题, 实现了对光纤环形谐振腔共振频率的快速、灵敏的控制和锁定. 结果表明, 锁频过程中相位调制功率与相位调制引起腔反射光的强度调制之间的关系为线性关系, 进而通过降低相位调制信号的功率以减小相位调制对腔反射光强度调制的影响. 当调制功率设定最低为–9 dBm时, 光纤环形谐振腔仍能被稳定锁定. 该光纤环形谐振腔为其与原子、金刚石色心等发光粒子相互作用的腔量子电动力学实验研究奠定了坚实的基础.-
关键词:
- 光纤环形谐振腔 /
- 临界耦合 /
- Pound-Drever-Hall锁频
Optical resonators play an active role in fundamental research and applications in atomic fine spectra, laser generation, precision measurements, and quantum information processing because of their high-resolution spectra and strong optical field enhancement. The fiber ring resonators, as a derivative of the resonant resonators, have the advantages of simple structure, small size, stable performance and easy integration. The fiber ring resonators are widely used in fiber lasers, optical communication devices, optical fiber sensing, etc. In this paper, we demonstrate the characteristics of a fiber ring resonator based on a tunable fiber beam splitter experimentally. Control of under-coupling, critical coupling and over-coupling state of the fiber ring resonator can be achieved by adjusting the splitting ratio of the tunable fiber beam splitter. The relationship between the minimum resonator reflectance and resonator loss is given. The intrinsic decay rate of the fiber ring resonator is${\kappa _0}{\rm{ = }}2{\text{π}} \times \left( {1.60 \pm 0.03} \right)\;{\rm{ MHz}}$ , and the quality factor is$Q = \left( {1.10 \pm 0.02} \right) \times {10.8}$ . The resonance frequency of the fiber ring resonator is controlled by stretching the fiber. The fiber resonator is kept straight and fixed on a self-made U-shaped holder by gluing two points. A piezoelectric transducer is used to change the distance between the two glued points. The fiber ring resonator length is changed and controlled when the fiber is stretched. The Pound-Drever-Hall technique is used to lock the resonator to resonance with the laser. The phase of the laser beam is modulated by using an electro-optical modulator, and two sidebands of the laser frequency are generated. Due to the phase sensitivity of the fiber resonator, the reflected light of the fiber resonator with an intensity modulation is observed when the fiber ring resonator is locked. The intensity modulation is caused by the interference between the resonance frequency and the sidebands of the fiber ring resonator. The reflected spectrum of the fiber ring resonator carries the same-frequency modulation as the phase modulation. This is a disadvantage for the usage of the fiber ring resonator. Thus, we reduce the phase modulation power to reduce the intensity modulation of the resonator reflectance. The linear relationship between the phase modulation power and the intensity modulation of the resonator reflectance caused by the phase modulation is obtained. The fiber ring resonator can be locked when the phase modulation power decreases to –9 dBm. The fiber ring resonator has laid a solid experimental foundation for experimental research on the interaction between the fiber ring resonator and quantum emitters such as atoms and color centers in diamond.-
Keywords:
- fiber ring resonator /
- critical coupling /
- Pound-Drever-Hall technique
[1] Kogelnik H, Li T 1966 Proc. IEEE 54 1312
[2] 刘涛, 张天才, 王军民, 彭堃墀 2002 量子光学学报 8 30
Google Scholar
Liu T, Zhang T C, Wang J M, Peng K C 2002 J. Quantum Opt. 8 30
Google Scholar
[3] 张国威, 王兆民 2007 激光光谱学原理与技术 (北京: 北京理工大学出版社) 第87—93页
Zhang G, Wang Z M 2007 Principles and Techniques of Laser Spectroscopy (Beijing: Beijing Institute of Technology Press) pp87—93 (in Chinese)
[4] 周炳琨, 高以智, 陈倜嵘, 陈家骅, 霍力 2014 激光原理(第七版)(北京: 国防工业出版社) 第14—18页
Zhou B Z, Gao Y Z, Chen W, Chen J Y, Huo L 2014 Laser Principles (Seventh Edition) (Beijing: National Defense Industry Press) pp14—18 (in Chinese)
[5] Coddington I, Swann W C, Nenadovic L, Newbury N R 2009 Nat. Photonics 3 351
Google Scholar
[6] Li P B, Gu Y, Gong Q H, Guo G C 2009 Phys. Rev. A 79 126
[7] Stokes L F, Chodorow M, Shaw H J 1982 Opt. Lett. 7 288
Google Scholar
[8] 高磊 2016 博士学位论文 (重庆: 重庆大学)
Gao L 2016 Ph. D. Dissertation (Chongqing: University of Chongqing)
[9] Pierre-Henri M, Olivier L, Gilles C 2008 IEEE Photon. Tech. L 20 1399
Google Scholar
[10] Ma H, Zhang J, Wang L, Lu Y, Ying D, Jin Z 2015 Opt. Lett. 40 5862
Google Scholar
[11] Tong L M, Gattass R R, Ashcomv J B, He S L, Lou J Y, Shen M Y, Maxwell I, Mazur E 2003 Nature 426 816
Google Scholar
[12] 成凡, 张鹏飞, 王鑫, 张天才 2017 量子光学学报 23 74
Cheng F, Zhang P F, Wang X, Zhang T C 2017 J. Quantum Opt. 23 74
[13] Hoffman J E, Ravets S, Grover J A, Solano P, Kordell P R, Wong-Campos J D, Orozco L A, Rolston S L 2014 Aip Adv. 067124
[14] Nagai R, Aoki T 2014 Opt. Express 22 28427
Google Scholar
[15] Zhang P F, Cheng F, Wang X, Song L J, Zou C L, Li G, Zhang T C 2018 Opt. Express 26 31500
Google Scholar
[16] Jones D, Hickman G, Franson J, Pittman T 2016 Opt. Lett. 41 3683
Google Scholar
[17] Schneeweiss P, Zeiger S, Hoinkes T, Rauschenbeutel A, Volz J 2017 Opt. Lett. 42 85
Google Scholar
[18] Ruddell S K, Webb K E, Herrera I, Parkins A S, Hoogerland M D 2017 Optica 4 576
Google Scholar
[19] Wuttke C, Rauschenbeutel A 2013 Phys. Rev. Lett. 111 024301
Google Scholar
[20] Reynaud F, Boca J 1993 Pure Appl. Opt. 2 677
Google Scholar
[21] Jackson D A, Priest R G, Dandridge A, Tveten A B 1980 Appl. Opt. 19 2926
Google Scholar
[22] Reynaud F, Delaire E 1993 Electron. Lett. 29 1718
Google Scholar
[23] Coen S, Haelterman M, Emplit P, Delage L, Simohamed L M, Reynaud F 1998 J. Opt. Soc. Am. B 15 2283
Google Scholar
[24] Eric D, Black 2001 Am. J. Phys. 69 79
[25] 刘志强, 刘建丽, 翟泽辉 2018 量子光学学报 24 228
Liu Z Q, Liu J L, Yan Z H 2018 J. Quantum Opt. 24 228
[26] Spillane S M, Kippenberg T J, Painter O J, Vahala K J 2003 Phys. Rev. Lett. 91 043902
Google Scholar
-
图 1 (a) 光纤环形谐振腔及其光谱测量实验装置; (b)光纤拉伸支架
Fig. 1. (a) Fiber ring resonator and spectrum experiment device; (b) fiber tensile holder.
图 3 可调谐分束器的分束比与旋钮旋转角度关系
Fig. 3. Splitting ratio as a function of angle of knob of fiber splitter.
图 4 (a) 不同耦合态下的光纤环形谐振腔反射谱; (b) 最低反射率T随总衰减率κ的关系
Fig. 4. (a) Fiber ring resonator reflectance spectra with different coupling states; (b) minimum reflectance T as a function of κ.
图 5 相位调制功率为12 dBm (a)和–9 dBm(b)的锁定结果
Fig. 5. Locking results when modulation power of phase is 12 dBm (a) and –9 dBm(b).
图 6 (a)腔反射信号的频谱分析; (b)腔反射最大调制强度与无调制时的比值随相位调制功率的变化
Fig. 6. (a) Frequency spectra analysis of resonator reflectance; (b) power ratio of resonator reflectance modulated at maximum intensity and without modulation as a function of phase modulation power.
-
[1] Kogelnik H, Li T 1966 Proc. IEEE 54 1312
[2] 刘涛, 张天才, 王军民, 彭堃墀 2002 量子光学学报 8 30
Google Scholar
Liu T, Zhang T C, Wang J M, Peng K C 2002 J. Quantum Opt. 8 30
Google Scholar
[3] 张国威, 王兆民 2007 激光光谱学原理与技术 (北京: 北京理工大学出版社) 第87—93页
Zhang G, Wang Z M 2007 Principles and Techniques of Laser Spectroscopy (Beijing: Beijing Institute of Technology Press) pp87—93 (in Chinese)
[4] 周炳琨, 高以智, 陈倜嵘, 陈家骅, 霍力 2014 激光原理(第七版)(北京: 国防工业出版社) 第14—18页
Zhou B Z, Gao Y Z, Chen W, Chen J Y, Huo L 2014 Laser Principles (Seventh Edition) (Beijing: National Defense Industry Press) pp14—18 (in Chinese)
[5] Coddington I, Swann W C, Nenadovic L, Newbury N R 2009 Nat. Photonics 3 351
Google Scholar
[6] Li P B, Gu Y, Gong Q H, Guo G C 2009 Phys. Rev. A 79 126
[7] Stokes L F, Chodorow M, Shaw H J 1982 Opt. Lett. 7 288
Google Scholar
[8] 高磊 2016 博士学位论文 (重庆: 重庆大学)
Gao L 2016 Ph. D. Dissertation (Chongqing: University of Chongqing)
[9] Pierre-Henri M, Olivier L, Gilles C 2008 IEEE Photon. Tech. L 20 1399
Google Scholar
[10] Ma H, Zhang J, Wang L, Lu Y, Ying D, Jin Z 2015 Opt. Lett. 40 5862
Google Scholar
[11] Tong L M, Gattass R R, Ashcomv J B, He S L, Lou J Y, Shen M Y, Maxwell I, Mazur E 2003 Nature 426 816
Google Scholar
[12] 成凡, 张鹏飞, 王鑫, 张天才 2017 量子光学学报 23 74
Cheng F, Zhang P F, Wang X, Zhang T C 2017 J. Quantum Opt. 23 74
[13] Hoffman J E, Ravets S, Grover J A, Solano P, Kordell P R, Wong-Campos J D, Orozco L A, Rolston S L 2014 Aip Adv. 067124
[14] Nagai R, Aoki T 2014 Opt. Express 22 28427
Google Scholar
[15] Zhang P F, Cheng F, Wang X, Song L J, Zou C L, Li G, Zhang T C 2018 Opt. Express 26 31500
Google Scholar
[16] Jones D, Hickman G, Franson J, Pittman T 2016 Opt. Lett. 41 3683
Google Scholar
[17] Schneeweiss P, Zeiger S, Hoinkes T, Rauschenbeutel A, Volz J 2017 Opt. Lett. 42 85
Google Scholar
[18] Ruddell S K, Webb K E, Herrera I, Parkins A S, Hoogerland M D 2017 Optica 4 576
Google Scholar
[19] Wuttke C, Rauschenbeutel A 2013 Phys. Rev. Lett. 111 024301
Google Scholar
[20] Reynaud F, Boca J 1993 Pure Appl. Opt. 2 677
Google Scholar
[21] Jackson D A, Priest R G, Dandridge A, Tveten A B 1980 Appl. Opt. 19 2926
Google Scholar
[22] Reynaud F, Delaire E 1993 Electron. Lett. 29 1718
Google Scholar
[23] Coen S, Haelterman M, Emplit P, Delage L, Simohamed L M, Reynaud F 1998 J. Opt. Soc. Am. B 15 2283
Google Scholar
[24] Eric D, Black 2001 Am. J. Phys. 69 79
[25] 刘志强, 刘建丽, 翟泽辉 2018 量子光学学报 24 228
Liu Z Q, Liu J L, Yan Z H 2018 J. Quantum Opt. 24 228
[26] Spillane S M, Kippenberg T J, Painter O J, Vahala K J 2003 Phys. Rev. Lett. 91 043902
Google Scholar
下载:
计量
- 文章访问数: 12298
- PDF下载量: 299
- 被引次数: 0
