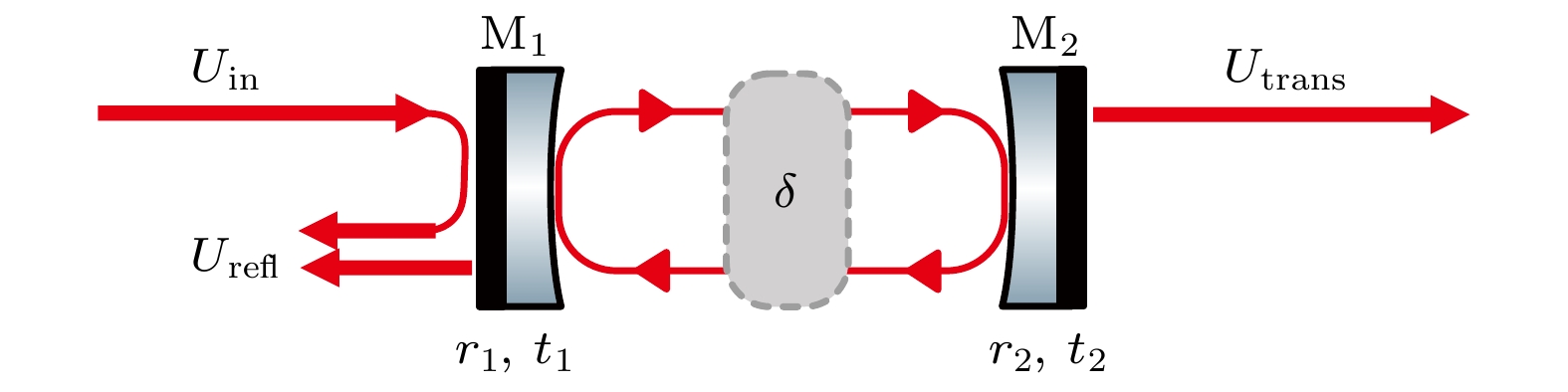
-
Quantum noise has become an important limiting factor in the application of precision measurement, and its relevant problems have become a research hotspot. As an important optical device to manipulate quantum noise, the optical resonator possesses the transmission characteristics that determine the evolution characteristics of output signal’s noise. According to their impedance matching factor a values, the resonators can be divided into three categories: over-coupled cavity for
$a \in [ - 1, 0)$ , impedance matched cavity for$a{{ = }}0$ , and under-coupled cavity for$a \in (0, 1]$ . When the resonator fully meets the resonant conditions, its output field can be regarded as a low-pass filter, the high-frequency noise is directly reflected. The high-frequency noise at the output end is greatly suppressed, and the noise at the frequency far larger than the linewidth reaches the shot noise standard. Therefore, the noise of the optical field beyond the linewidth range can be greatly suppressed by the narrow linewidth optical resonator. At the same time, from the three kinds of optical resonator phase diagrams it can be found that the over-coupled cavity is in a state of half a detuning and the sideband frequency phase rotates ± 90° relative to the carrier frequency. In this case, the phase noise of light field can be converted into amplitude noise by an over-coupled cavity, which can be used for the phase noise measurement or squeezing angle rotation of squeezed light and has important applications in analyzing the laser noise component and manipulating the quantum noise. At the same time, the energy loss of the over-coupled cavity is the largest among the three types of cavity structures. Through theoretically analysing the corresponding relation among optical resonator output intensity, phase and frequency, and by making a comparison of comparing transfer function, energy transmission, spectrum characteristics of noise transmission among over-coupled cavity, impedance matched cavity and under-coupled cavity, in this paper the power splitter, frequency filtering, and noise transformation features of the optical resonator are demonstrated. The analysis results in this paper provide a basis for applying various optical resonators to different occasions, and promote the development of using the optical resonators to control the quantum noise of light field and improving the precision of precision measurement.-
Keywords:
- optical resonator /
- transfer function /
- noise spectrum /
- quantum noise
[1] 聂丹丹, 冯晋霞, 戚蒙, 李渊骥, 张宽收 2020 69 094205
Google Scholar
Nie D D, Feng J X, Qi M, Li Y J, Zhang K S 2020 Acta Phys. Sin. 69 094205
Google Scholar
[2] 翟泽辉, 郝温静, 刘建丽, 段西亚 2020 69 184204
Google Scholar
Zhai Z H, Hao W J, Liu J L, Duan X Y 2020 Acta Phys. Sin. 69 184204
Google Scholar
[3] 刘奎, 马龙, 苏必达, 李佳明, 孙恒信, 郜江瑞 2020 69 124203
Google Scholar
Liu K, Ma L, Su B D, Li J M, Sun H X, Gao J R 2020 Acta Phys. Sin. 69 124203
Google Scholar
[4] 周瑶瑶, 田剑锋, 闫智辉, 贾晓军 2019 68 064205
Google Scholar
Zhou Y Y, Tian J F, Yan Z H, Jia X J 2019 Acta Phys. Sin. 68 064205
Google Scholar
[5] 葛瑞芳, 杨鹏飞, 韩星, 张鹏飞, 李刚, 张天才 2020 量子光学学报 26 21
Google Scholar
Ge R F, Yang P F, Han X, Zhang P F, Li G, Zhang T C 2020 Acta Sin. Quantum Opt. 26 21
Google Scholar
[6] 石柱, 郭永瑞, 徐敏志, 卢华东 2018 量子光学学报 24 237
Google Scholar
Shi Z, Guo Y R, Xu M Z, Lu H D 2018 Acta Sin. Quantum Opt. 24 237
Google Scholar
[7] Wang Y, Shen H, Jin X L, Su X L, Xie C D, Peng K C 2010 Opt. Express 18 6149
Google Scholar
[8] 王俊萍, 张文慧, 李瑞鑫, 田龙, 王雅君, 郑耀辉 2020 69 234204
Wang J P, Zhang W H, Li R X, Tian L, Wang Y Jun, Zheng Y H 2020 Acta Phys. Sin. 69 234204
[9] Villar A S 2008 Am. J. Phys. 76 922
Google Scholar
[10] Wang Y J, Zheng Y H, Shi Z, Peng K C 2012 Laser Phys. Lett. 9 506
Google Scholar
[11] Zhang W H, Wang J R, Zheng Y H, Wang Y J, Peng K C 2019 Appl. Phys. Lett. 115 171103
Google Scholar
[12] Zhao G, Hausmaninger T, Ma W G, Axner O 2017 Opt. Lett. 42 3109
Google Scholar
[13] 胡悦, 曹凤朝, 董仁婧, 郝辰悦, 刘大禾, 石锦卫 2020 69 224202
Hu Y, Cao F Z, Dong R J, Hao C Y, Liu D H, Shi J W 2020 Acta Phys. Sin. 69 224202
[14] Schreiber K U, Gebauer A, Wells J P R 2013 Opt. Lett. 38 3574
Google Scholar
[15] Leibrandt D R, Heidecker J 2015 Rev. Sci. Instrum. 86 123115
Google Scholar
[16] Liu K, Zhang F L, Li Z Y, Feng X H, Li K, Lu Z H, Schreiber K U, Luo J, Zhang J 2019 Opt. Lett. 44 2732
Google Scholar
[17] Kwee P, Willke B, Danzmann K 2011 Opt. Lett. 36 3563
Google Scholar
[18] Kaufer S, Kasprzack M, Frolov V, Willke B 2017 Classical Quantum Gravity 34 145001
Google Scholar
[19] Junker J, Oppermann P, Willke B 2017 Opt. Lett. 42 755
Google Scholar
[20] Kaufer S, Willke B 2019 Opt. Lett. 44 1916
Google Scholar
[21] Zhao Y H, Aritomi N, Capocasa E, et al. 2020 Phys. Rev. Lett. 124 171101
Google Scholar
[22] McCuller L, Whittle C, Ganapathy D, et al. 2020 Phys. Rev. Lett. 124 171102
Google Scholar
[23] Capocasa E, Barsuglia M, Degallaix J, Pinard L, Straniero N, Schnabel R, Somiya K, Aso Y, Tatsumi D, Flaminio R 2016 Phys. Rev. D 93 082004
Google Scholar
[24] Kwee P 2010 Ph. D. Dissertation (Hannover: Leibniz Universität Hannover)
[25] Kaufer S 2018 Ph. D. Dissertation (Hannover: Leibniz Universität Hannover)
[26] Brozek O S 1999 Ph. D. Dissertation (Hannover: Universität Hannover)
[27] Guo X M, Wang X Y, Li Y M, Zhang K S 2009 Appl. Opt. 48 6475
Google Scholar
-
图 2 不同腔型的特性 (a)能量传输特性; (b)循环功率特性
Fig. 2. Characteristics of different cavity types: (a) Energy transfer characteristic; (b) cyclic power characteristic.
图 3 传输函数
$G\left( f \right)$ 随频率f的变化Fig. 3. Diagram of the transfer function
$G\left( f \right)$ with respect to frequency f.
图 4 光学谐振腔光强反射率和反射位相
${\theta _{\rm{R}}}$ 与失谐量Δ的关系 (a) 过耦合腔,${R_1} = 0.99$ ,${R_2} = 0.998$ ; (b)欠耦合腔,${R_1} = 0.998$ ,${R_2} = 0.99$ ; (c)阻抗匹配腔,${R_1} = {R_2} $ $ = 0.994$ Fig. 4. Relations between optical intensity reflectivity and reflection phase
${\theta _R}$ and detuning Δ in optical resonator: (a) Over-coupled cavity,${R_1}{{ = }}0.99$ ,${R_2} = 0.998$ ; (b) under-coupled cavity,${R_1} = 0.998$ ,${R_2} = 0.99$ ; (c) impedance matched cavity,${R_1} = R_2 = 0.994$ .
图 6 腔输出场的量子噪声限制 (a)阻抗匹配腔中噪声随频率的变化; (b)非阻抗匹配腔中噪声随频率的变化; (c)反射光场噪声随阻抗匹配因子a的变化
Fig. 6. Quantum noise limitation of cavity output field: (a) Variation of noise with frequency in impedance matched cavity; (b) variation of noise with frequency in a non-impedance matched cavity; (c) variation of noise of the reflected light field with impedance matching factor a.
-
[1] 聂丹丹, 冯晋霞, 戚蒙, 李渊骥, 张宽收 2020 69 094205
Google Scholar
Nie D D, Feng J X, Qi M, Li Y J, Zhang K S 2020 Acta Phys. Sin. 69 094205
Google Scholar
[2] 翟泽辉, 郝温静, 刘建丽, 段西亚 2020 69 184204
Google Scholar
Zhai Z H, Hao W J, Liu J L, Duan X Y 2020 Acta Phys. Sin. 69 184204
Google Scholar
[3] 刘奎, 马龙, 苏必达, 李佳明, 孙恒信, 郜江瑞 2020 69 124203
Google Scholar
Liu K, Ma L, Su B D, Li J M, Sun H X, Gao J R 2020 Acta Phys. Sin. 69 124203
Google Scholar
[4] 周瑶瑶, 田剑锋, 闫智辉, 贾晓军 2019 68 064205
Google Scholar
Zhou Y Y, Tian J F, Yan Z H, Jia X J 2019 Acta Phys. Sin. 68 064205
Google Scholar
[5] 葛瑞芳, 杨鹏飞, 韩星, 张鹏飞, 李刚, 张天才 2020 量子光学学报 26 21
Google Scholar
Ge R F, Yang P F, Han X, Zhang P F, Li G, Zhang T C 2020 Acta Sin. Quantum Opt. 26 21
Google Scholar
[6] 石柱, 郭永瑞, 徐敏志, 卢华东 2018 量子光学学报 24 237
Google Scholar
Shi Z, Guo Y R, Xu M Z, Lu H D 2018 Acta Sin. Quantum Opt. 24 237
Google Scholar
[7] Wang Y, Shen H, Jin X L, Su X L, Xie C D, Peng K C 2010 Opt. Express 18 6149
Google Scholar
[8] 王俊萍, 张文慧, 李瑞鑫, 田龙, 王雅君, 郑耀辉 2020 69 234204
Wang J P, Zhang W H, Li R X, Tian L, Wang Y Jun, Zheng Y H 2020 Acta Phys. Sin. 69 234204
[9] Villar A S 2008 Am. J. Phys. 76 922
Google Scholar
[10] Wang Y J, Zheng Y H, Shi Z, Peng K C 2012 Laser Phys. Lett. 9 506
Google Scholar
[11] Zhang W H, Wang J R, Zheng Y H, Wang Y J, Peng K C 2019 Appl. Phys. Lett. 115 171103
Google Scholar
[12] Zhao G, Hausmaninger T, Ma W G, Axner O 2017 Opt. Lett. 42 3109
Google Scholar
[13] 胡悦, 曹凤朝, 董仁婧, 郝辰悦, 刘大禾, 石锦卫 2020 69 224202
Hu Y, Cao F Z, Dong R J, Hao C Y, Liu D H, Shi J W 2020 Acta Phys. Sin. 69 224202
[14] Schreiber K U, Gebauer A, Wells J P R 2013 Opt. Lett. 38 3574
Google Scholar
[15] Leibrandt D R, Heidecker J 2015 Rev. Sci. Instrum. 86 123115
Google Scholar
[16] Liu K, Zhang F L, Li Z Y, Feng X H, Li K, Lu Z H, Schreiber K U, Luo J, Zhang J 2019 Opt. Lett. 44 2732
Google Scholar
[17] Kwee P, Willke B, Danzmann K 2011 Opt. Lett. 36 3563
Google Scholar
[18] Kaufer S, Kasprzack M, Frolov V, Willke B 2017 Classical Quantum Gravity 34 145001
Google Scholar
[19] Junker J, Oppermann P, Willke B 2017 Opt. Lett. 42 755
Google Scholar
[20] Kaufer S, Willke B 2019 Opt. Lett. 44 1916
Google Scholar
[21] Zhao Y H, Aritomi N, Capocasa E, et al. 2020 Phys. Rev. Lett. 124 171101
Google Scholar
[22] McCuller L, Whittle C, Ganapathy D, et al. 2020 Phys. Rev. Lett. 124 171102
Google Scholar
[23] Capocasa E, Barsuglia M, Degallaix J, Pinard L, Straniero N, Schnabel R, Somiya K, Aso Y, Tatsumi D, Flaminio R 2016 Phys. Rev. D 93 082004
Google Scholar
[24] Kwee P 2010 Ph. D. Dissertation (Hannover: Leibniz Universität Hannover)
[25] Kaufer S 2018 Ph. D. Dissertation (Hannover: Leibniz Universität Hannover)
[26] Brozek O S 1999 Ph. D. Dissertation (Hannover: Universität Hannover)
[27] Guo X M, Wang X Y, Li Y M, Zhang K S 2009 Appl. Opt. 48 6475
Google Scholar
下载:
计量
- 文章访问数: 8833
- PDF下载量: 470
- 被引次数: 0
