-
研究了三椭圆谐振腔耦合波导中可调谐双重等离子体诱导透明效应. 三椭圆谐振腔耦合波导结构由1个亮模和2个暗模或1个暗模和2个亮模组成, 类比于原子系统的四能级结构. 为了获得较理想的双重等离子体诱导透明窗口, 利用有限元法数值分析了多种三椭圆谐振腔耦合波导结构的光学透射特性. 针对较佳的三椭圆谐振腔波导结构, 分别讨论波导结构参数(如椭圆腔长轴半径、底部椭圆腔与主波导间耦合距离、椭圆腔间耦合距离、对称破缺度, 以及椭圆腔填充材料有效折射率)对双重等离子体诱导透明效应的影响. 多重透明窗口的数值模拟结果为等离子体诱导透明在等离子体开关及传感器方面的潜在应用提供理论基础.
-
关键词:
- 表面等离极化激元 /
- 等离子体诱导透明 /
- 金属-介质-金属波导
The tunable double plasmon-induced transparency (PIT) effects are investigated in a waveguide coupled by the three ellipse-shaped resonators. By the finite element method, we study the influences of coupling modes of the three ellipse-shaped resonators, waveguide structure parameters and the refractive indices of dielectric in three ellipse-shaped resonators on double PIT effects. The waveguide structure consists of three ellipse-shaped resonators, and is similar to a four-level structure of the atomic system. The bottom ellipse-shaped resonator can be named a bright mode, the middle and top ellipse-shaped resonators each can be seen as a dark mode. In order to obtain an ideal double PIT transparency window, we also numerically analyze the optical transmission characteristics of structures of several three-ellipse-shaped resonator coupled waveguides. Furthermore, we mainly discuss the transmission spectra in the better three-ellipse-shaped resonator coupled waveguide structure as a function of the radii of the long axis in ellipse-shaped resonators, the coupling distance between the bottom ellipse-shaped resonator and the bus waveguide, the coupling distance between ellipse-shaped resonators, and the symmetry broken degree. In addition, we also consider the effect of the refractive indices of dielectric in three ellipse-shaped resonators on double PIT spectra. It is found that the transmission spectra in the three-ellipse-shaped resonator coupled waveguide have obvious red shift when the refractive indices of dielectric in the three ellipse-shaped resonators increase. All the simulation results may provide the theoretical basis for the potential application of multiple PIT in plasma switches and sensors.-
Keywords:
- surface plasmon polaritons /
- plasmon-induced transparency /
- metal-insulator-metal waveguide
[1] Ritchie R H 1957 Phys. Rev. 106 874
Google Scholar
[2] Barnes W L, Dereux A, Ebbesen T W 2003 Nature 424 824
Google Scholar
[3] Dionne J A, Sweatlock L A, Atwater H A, Polman A 2006 Phys. Rev. B 73 035407
Google Scholar
[4] Galvez F, del Valle J, Gomez A, Osorio M R, Granados D, Perez de Lara D, Garcia M A, Vicent J L 2016 Opt. Materials Express 6 3086
Google Scholar
[5] Yang X Y, Hua E, Su H, Guo J, Yan S B 2020 Sensors 20 4125
Google Scholar
[6] 陈颖, 谢进朝, 周鑫德, 张灿, 杨惠, 李少华 2019 68 237301
Google Scholar
Chen Y, Xie J C, Zhou X D, Zhang C, Yang H, Li S H 2019 Acta Phys. Sin. 68 237301
Google Scholar
[7] Han X, Wang T, Li X, Zhu Y 2016 Plasmonics 11 729
Google Scholar
[8] 杨韵茹, 关建飞 2016 65 057301
Google Scholar
Yang Y R, Guan J F 2016 Acta Phys. Sin. 65 057301
Google Scholar
[9] Liu X, Li J N, Chen J F, Rohimah S, Tian H, Wang J F 2021 Opt. Express 29 20829
Google Scholar
[10] 祁云平, 张雪伟, 周培阳, 胡兵兵, 王向贤 2018 67 197301
Google Scholar
Qi Y P, Zhang X W, Zhou P Y, Hu B B, Wang X Y 2018 Acta Phys. Sin. 67 197301
Google Scholar
[11] Hao X X, Huo Y P, He Q, Guo Y Y, Niu Q Q, Cui P F, Wang Y Y, Song M N 2021 Phys. Scripta 96 075505
Google Scholar
[12] Amrani M, Khattou S, Rezzouk Y, Mouadili A, Noual A, El Boudouti E H, Djafari-Rouhani B 2022 J. Phys. D: Appl. Phys. 55 075106
Google Scholar
[13] Zhang Z, Yang J, He X, Han Y, Zhang J, Huang J, Chen D 2018 Appl. Sci. 8 462
Google Scholar
[14] Harris S E, Field J E, Imamoğlu A 1990 Phys. Rev. Lett. 64 1107
Google Scholar
[15] 褚培新, 张玉斌, 陈俊学 2020 69 134205
Google Scholar
Chu P X, Zhang Y B, Chen J X 2020 Acta Phys. Sin. 69 134205
Google Scholar
[16] Chen M M, Xiao Z Y, Lu X J 2020 Carbon 159 273
Google Scholar
[17] Li M W, Liang C P, Zhang Y B, Yi Z, Chen X F, Zhou Z G, Yang H, Tang Y J, Yi Y G 2019 Results Phys. 15 102603
Google Scholar
[18] Wang X J, Meng H Y, Deng S Y, Lao C D, Wei Z C, Wang F H, Tan C G, Huang X 2019 Nanomaterials 9 385
Google Scholar
[19] Liu L, Xia S X, Luo X, Zhai X, Yu Y B, Wang L L 2018 Opt. Commun. 418 27
Google Scholar
[20] Waks E, Vuckovic J 2006 Phys. Rev. Lett. 96 153601
Google Scholar
[21] Marco P, Dario G, Liam O F, Claudio A L 2018 Opt. Express 26 8470
Google Scholar
[22] Li J J, Tian J P, Yang R C 2019 Eur. Phys. J. D 73 230
Google Scholar
[23] Han X, Wang T, Li X M, Liu B, He Y, Tang J 2015 J. Phys. D: Appl. Phys. 48 235102
Google Scholar
[24] Niu Y Y, Wang J C, Liu D D, Hu Z D, Sang T, Gao S M 2017 Optik 140 1038
Google Scholar
[25] Wang G X, Lu H, Liu X M 2012 Opt. Express 20 020902
Google Scholar
[26] Wen K H, Yan L S, Pan W, Luo B, Guo Z, Guo Y H, Luo X G 2014 J. Light. Technol. 32 1701
Google Scholar
[27] Cao G T, Li H J, Zhan S P, Xu H Q, Liu Z M, He Z H, Wang Y 2013 Opt. Express 21 9198
Google Scholar
[28] Wang Y Q, He Z H, Cui W, Ren X C, Li C J, Xue W W, Cao D M, Li G, Lei W L 2020 Results Phys. 16 102981
Google Scholar
[29] 李继军, 吴耀德, 宋明玉 2007 长江大学学报(自科版)理工卷 4 1673
Google Scholar
Li J J, Wu Y D, Song M Y 2007 J. Yangtze University (Nat. Sci. Edit) Sci. Eng. V. 4 1673
Google Scholar
[30] Li Z F, Wen K H, Fang Y H, Guo Z C 2020 IEEE J. Quantum Electron. 56 2982249
Google Scholar
[31] Qiong Z, Wang Z 2019 Opt. Express 27 303
Google Scholar
[32] Yin X G, Feng T H, Yip S, Liang Z X, Hui A, Ho J C, Li J S 2013 Appl. Phys. Lett. 103 021115
Google Scholar
[33] Xu H, Lu Y, Lee Y, Ham B S 2010 Opt. Express 18 17736
Google Scholar
[34] Zhu Y, Hu X Y, Yang H, Gong Q H 2014 Sci. Rep. UK 4 3752
Google Scholar
[35] 闫西成 2018 硕士学位论文(武汉:华中科技大学) (Wuhan: Huazhong University of Science & Technology)
Yan X C 2018 M. S. Thesis (Wuhan: Huazhong University of Science & Technology
[36] Ye C G, Zhang L 2008 Opt. Lett. 33 1911
Google Scholar
-
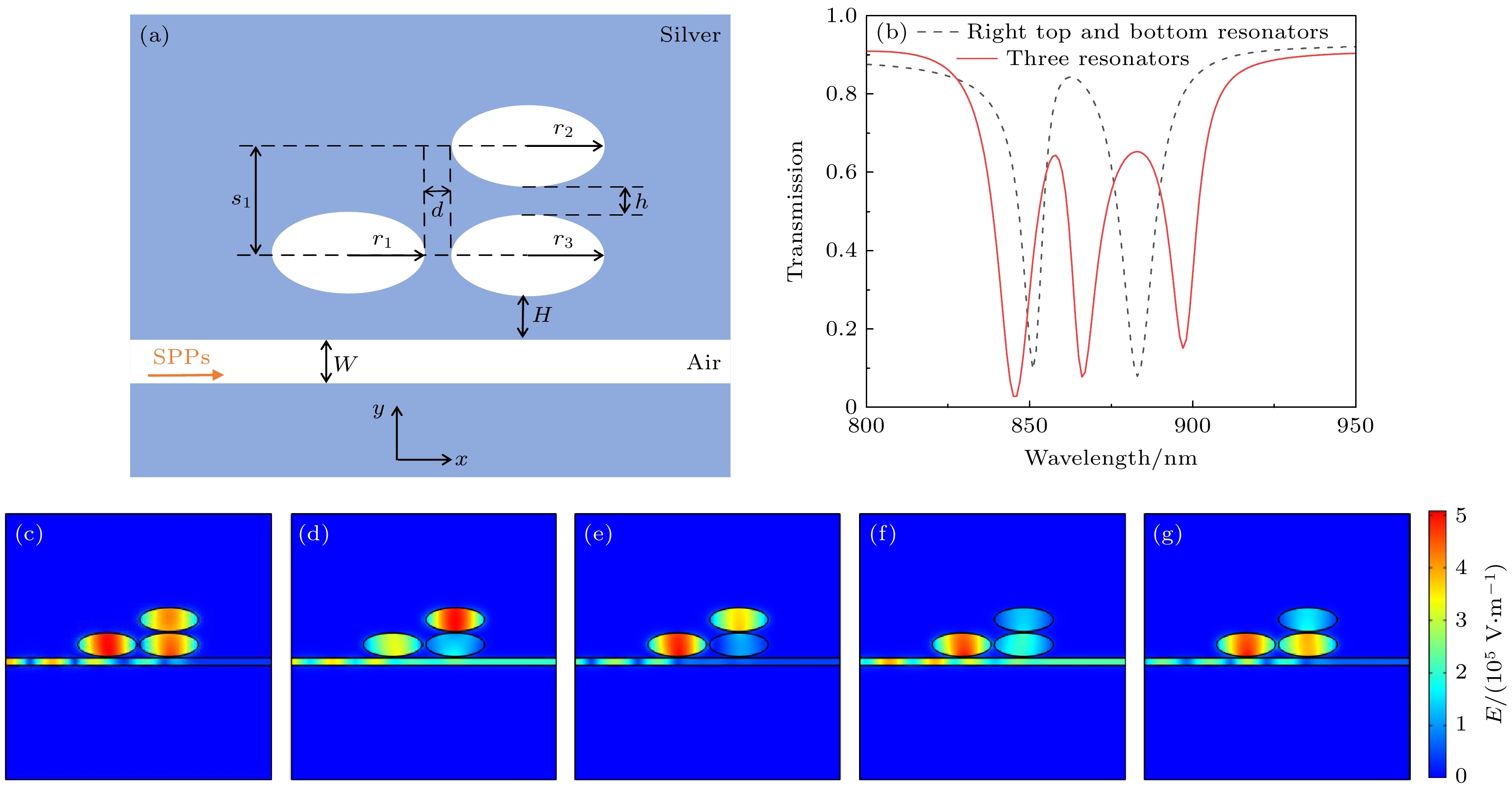
图 1 (a) 三椭圆谐振腔耦合波导结构(三椭圆腔左右放置且s1 = s2); (b) 双椭圆(黑色虚线)和三椭圆(红色实线)波导结构透射谱;(c)−(g) 三椭圆腔波导结构中波长分别为849, 855, 860, 866, 883 nm时的电场分布
Fig. 1. (a) Schematic diagram of three ellipse-shaped resonators coupled waveguide structure (three ellipse-shaped resonators are placed left and right and s1 = s2); (b) transmission spectra of the two (black dash) and three (red solid) ellipse-shaped resonators waveguide structure; (c)−(g) electric field distribution of three ellipse-shaped resonators waveguide structure at wavelength of 849, 855, 860, 866, 883 nm, respectively.
图 2 (a) 三椭圆谐振腔耦合波导结构(三椭圆腔左右放置且s1 = 0); (b) 双椭圆(黑色虚线)和三椭圆(红色实线)波导结构透射谱;(c)−(g) 三椭圆腔波导结构中波长分别为844, 851, 867, 877, 888 nm时的电场分布
Fig. 2. (a) Schematic diagram of three ellipse-shaped resonators coupled waveguide structure (three ellipse-shaped resonators are placed left and right and s1 = 0); (b) transmission spectra of two (black dash) and three (red solid) ellipse-shaped resonators waveguide structure; (c)−(g) electric field distribution of three ellipse-shaped resonators waveguide structure at wavelength of 844, 851, 867, 877, 888 nm, respectively.
图 3 (a) 三椭圆谐振腔耦合波导结构(三椭圆腔左右放置且s2 = 0); (b) 双椭圆(黑色虚线)和三椭圆(红色实线)波导结构透射谱;(c)−(g) 三椭圆腔波导结构中波长分别为846, 858, 866, 883, 897 nm时的电场分布
Fig. 3. (a) Schematic diagram of three ellipse-shaped resonators coupled waveguide structure (three ellipse-shaped resonators are placed left and right and s2 = 0); (b) transmission spectra of two (black dash) and three (red solid) ellipse-shaped resonators waveguide structure; (c)−(g) electric field distribution of three ellipse-shaped resonators waveguide structure at wavelength of 846, 858, 866, 883, 897 nm, respectively.
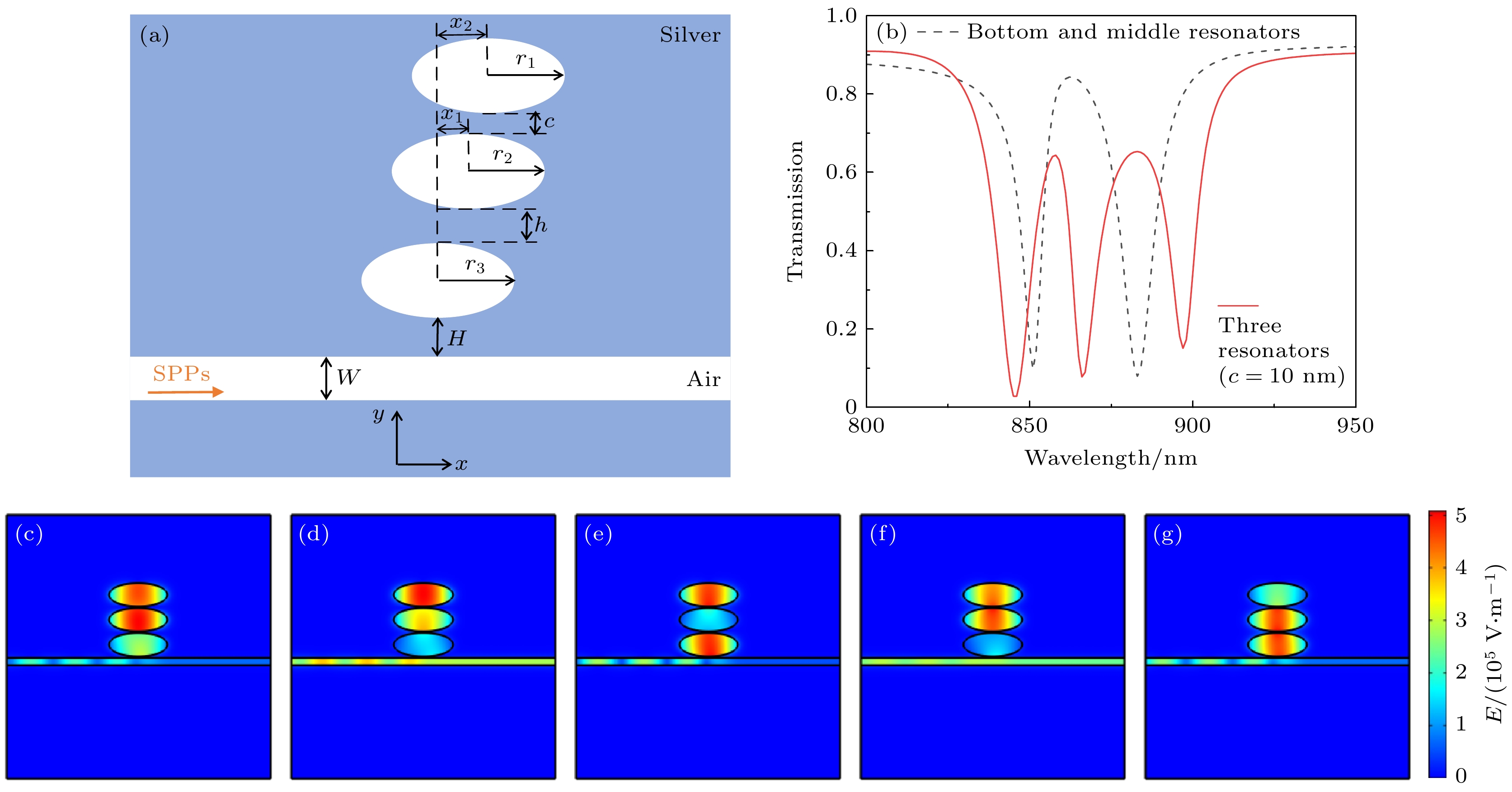
图 4 (a) 三椭圆谐振腔耦合波导结构(三椭圆腔在一条直线上竖直放置); (b) 双椭圆(黑色虚线)和三椭圆(红色实线)波导结构透射谱; (c)−(g) 三椭圆腔波导结构中波长分别为845, 851, 867, 878, 889 nm时的电场分布
Fig. 4. (a) Schematic diagram of three ellipse-shaped resonators coupled waveguide structure (three ellipse-shaped resonators are placed vertically in a straight line); (b) transmission spectra of two (black dash) and three (red solid) ellipse-shaped resonators waveguide structure; (c)−(g) electric field distribution of three ellipse-shaped resonators waveguide structure at wavelength of 845, 851, 867, 878, 889 nm, respectively.
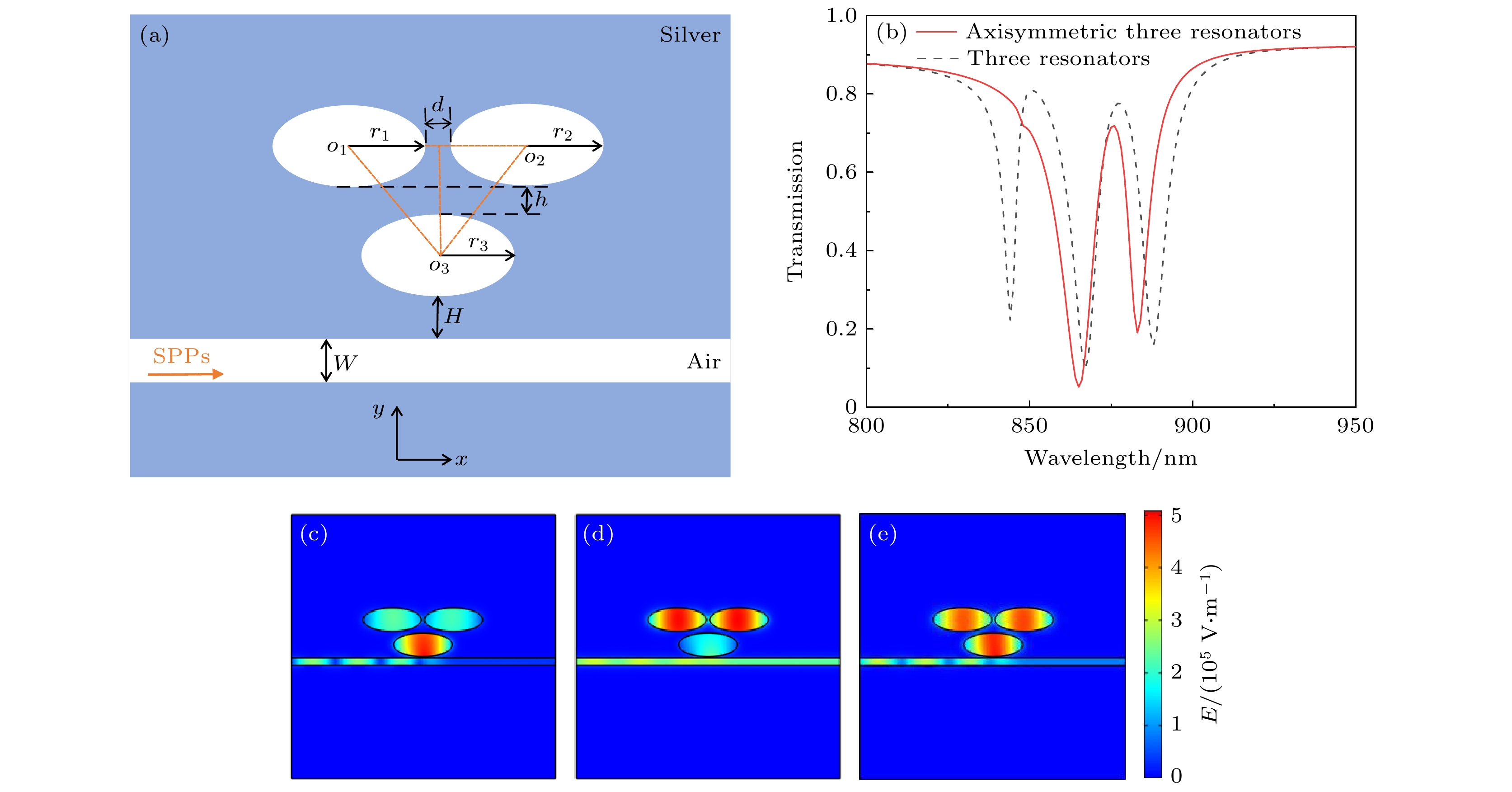
图 5 (a) 轴对称三椭圆谐振腔耦合波导结构(三椭圆腔倒等腰三角形放置且O3O1 = O3O2); (b) 非轴对称(黑色虚线)和轴对称(红色实线)三椭圆腔波导结构透射谱; (c)−(e) 轴对称波导结构中波长分别为865, 876, 883 nm时的电场分布
Fig. 5. (a) Schematic diagram of the axisymmetric three ellipse-shaped resonators coupled waveguide structure (three ellipse-shaped resonators are placed in an inverted isosceles triangle and O3O1 = O3O2); (b) transmission spectra of the non-axisymmetric (black dash) and the axisymmetric (red solid) three ellipse-shaped resonators waveguide structure; (c)−(e) electric field distribution of the axisymmetric three ellipse-shaped resonators waveguide structure at wavelength of 865, 876, 883 nm, respectively.
图 6 (a) 轴对称三椭圆谐振腔耦合波导结构(三椭圆腔正等腰三角形放置且O2O1 = O2O3); (b) 非轴对称(黑色虚线)和轴对称(红色实线)三椭圆腔波导结构透射谱; (c)−(e) 轴对称波导结构中波长分别为853, 879, 895 nm时的电场分布
Fig. 6. (a) Schematic diagram of the axisymmetric three ellipse-shaped resonators coupled waveguide structure (three ellipse-shaped resonators are placed in a positive isosceles triangle and O2O1 = O2O3); (b) transmission spectra of the non-axisymmetric (black dash) and the axisymmetric (red solid) three ellipse-shaped resonators waveguide structure; (c)−(e) electric field distribution of the axisymmetric three ellipse-shaped resonators waveguide structure at wavelength of 853, 879, 895 nm, respectively.
图 7 三椭圆腔耦合波导结构双PIT效应实验设计示意简图
Fig. 7. Schematic diagram of experimental design of double PIT effects for the three ellipse-shaped resonators coupled waveguide structure.
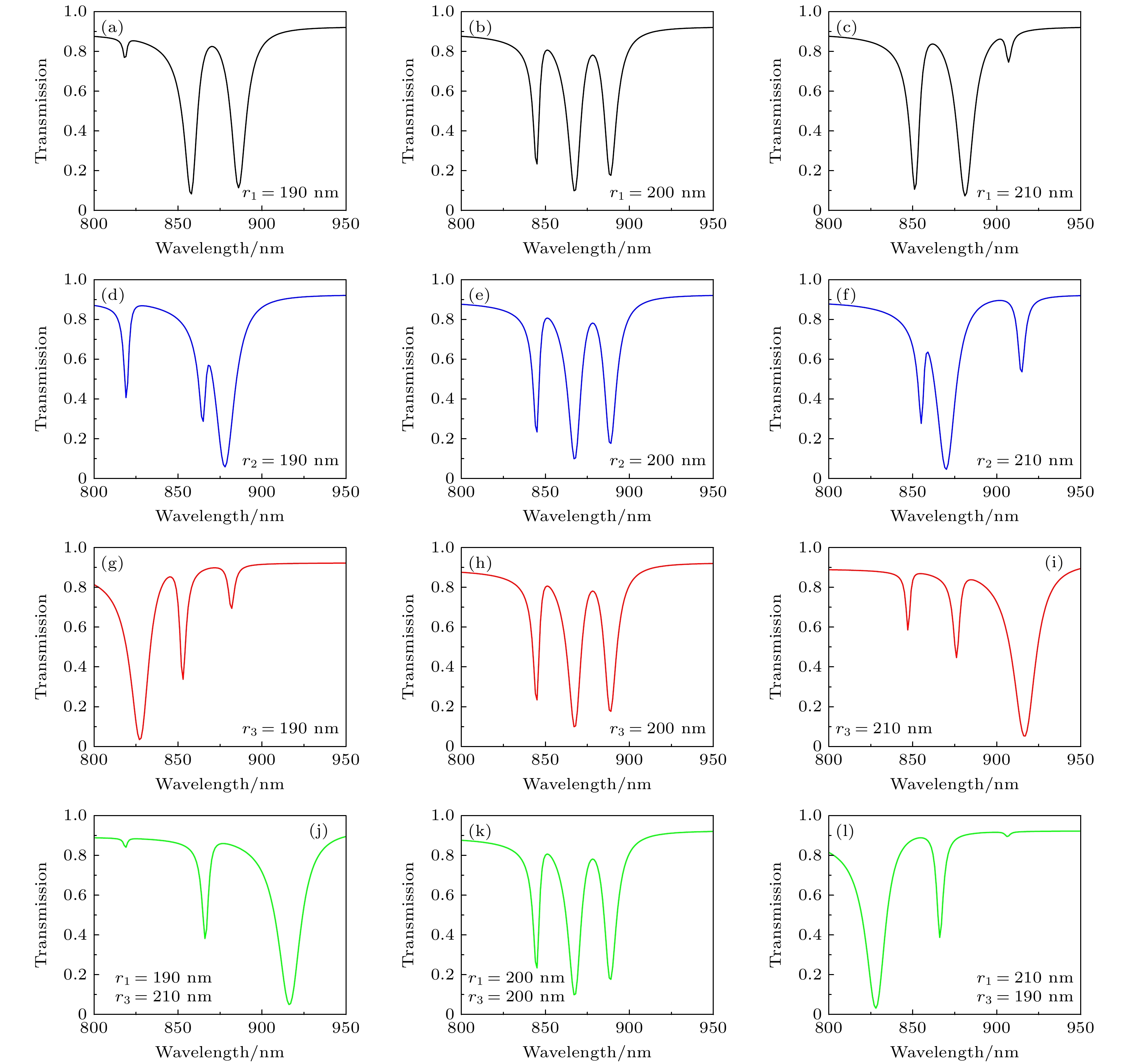
图 8 当改变椭圆腔长轴半径时, 三椭圆谐振腔波导结构的透射谱 (a)−(c) 改变顶部椭圆腔长轴半径r1; (d)−(f) 改变中部椭圆腔长轴半径r2; (g)−(i) 改变底部椭圆腔长轴半径r3; (j)—(l) 改变r1和r3
Fig. 8. Transmission spectra in the three ellipse-shaped resonators coupled waveguide structure when changing the long-axis radius of the elliptical cavity: (a)−(c) Change radius of the long axis r1 in the top ellipse-shaped resonator; (d)−(f) change r2 in the middle ellipse-shaped resonator; (g)−(i) change r3 in the bottom ellipse-shaped resonator; (j)−(l) change r1 and r3.
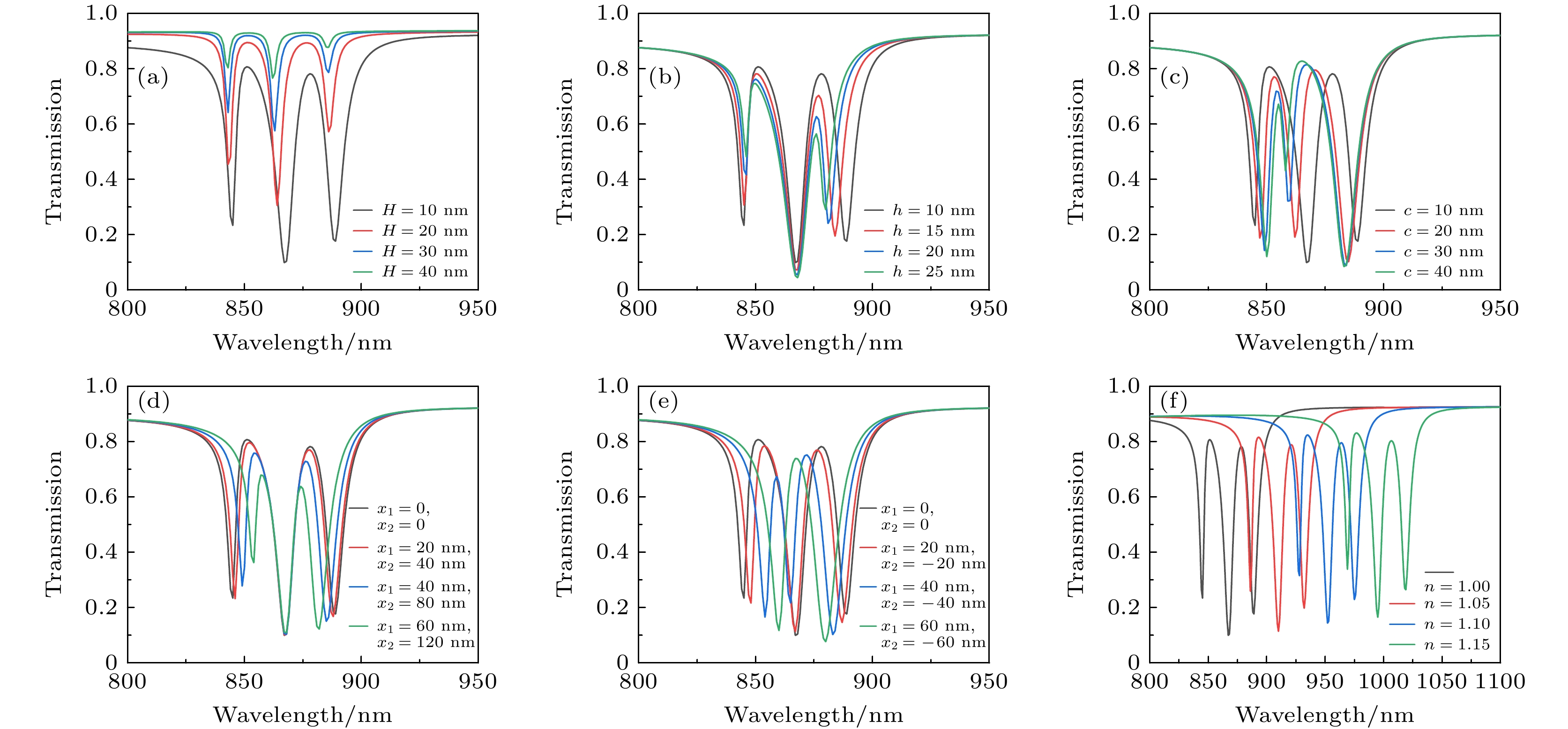
图 9 三椭圆谐振腔波导结构透射谱随结构参数的变化 (a) 当x1 = x2 = 0, h = c = 10 nm时, 透射谱随H的变化; (b) 当x1 = x2 = 0, H = c = 10 nm时, 透射谱随h的变化; (c) 当x1 = x2 = 0, H = h = 10 nm时, 透射谱随c的变化; (d), (e) 当H = h = c = 10 nm时, 透射谱随x1和x2的变化; (f) 当x1 = x2 = 0, H = h = 10 nm时, 透射谱随n的变化
Fig. 9. Transmission spectra in the three ellipse-shaped resonators coupled waveguide structure with different parameters: (a) With H when x1 = x2 = 0, h = c = 10 nm; (b) with h when x1 = x2 = 0, H = c =10 nm; (c) with c when x1 = x2 = 0, H = h = 10 nm; (d), (e) with x1 and x2 when H = h = c = 10 nm; (f) with n when x1 = x2 = 0, H = h = 10 nm.
-
[1] Ritchie R H 1957 Phys. Rev. 106 874
Google Scholar
[2] Barnes W L, Dereux A, Ebbesen T W 2003 Nature 424 824
Google Scholar
[3] Dionne J A, Sweatlock L A, Atwater H A, Polman A 2006 Phys. Rev. B 73 035407
Google Scholar
[4] Galvez F, del Valle J, Gomez A, Osorio M R, Granados D, Perez de Lara D, Garcia M A, Vicent J L 2016 Opt. Materials Express 6 3086
Google Scholar
[5] Yang X Y, Hua E, Su H, Guo J, Yan S B 2020 Sensors 20 4125
Google Scholar
[6] 陈颖, 谢进朝, 周鑫德, 张灿, 杨惠, 李少华 2019 68 237301
Google Scholar
Chen Y, Xie J C, Zhou X D, Zhang C, Yang H, Li S H 2019 Acta Phys. Sin. 68 237301
Google Scholar
[7] Han X, Wang T, Li X, Zhu Y 2016 Plasmonics 11 729
Google Scholar
[8] 杨韵茹, 关建飞 2016 65 057301
Google Scholar
Yang Y R, Guan J F 2016 Acta Phys. Sin. 65 057301
Google Scholar
[9] Liu X, Li J N, Chen J F, Rohimah S, Tian H, Wang J F 2021 Opt. Express 29 20829
Google Scholar
[10] 祁云平, 张雪伟, 周培阳, 胡兵兵, 王向贤 2018 67 197301
Google Scholar
Qi Y P, Zhang X W, Zhou P Y, Hu B B, Wang X Y 2018 Acta Phys. Sin. 67 197301
Google Scholar
[11] Hao X X, Huo Y P, He Q, Guo Y Y, Niu Q Q, Cui P F, Wang Y Y, Song M N 2021 Phys. Scripta 96 075505
Google Scholar
[12] Amrani M, Khattou S, Rezzouk Y, Mouadili A, Noual A, El Boudouti E H, Djafari-Rouhani B 2022 J. Phys. D: Appl. Phys. 55 075106
Google Scholar
[13] Zhang Z, Yang J, He X, Han Y, Zhang J, Huang J, Chen D 2018 Appl. Sci. 8 462
Google Scholar
[14] Harris S E, Field J E, Imamoğlu A 1990 Phys. Rev. Lett. 64 1107
Google Scholar
[15] 褚培新, 张玉斌, 陈俊学 2020 69 134205
Google Scholar
Chu P X, Zhang Y B, Chen J X 2020 Acta Phys. Sin. 69 134205
Google Scholar
[16] Chen M M, Xiao Z Y, Lu X J 2020 Carbon 159 273
Google Scholar
[17] Li M W, Liang C P, Zhang Y B, Yi Z, Chen X F, Zhou Z G, Yang H, Tang Y J, Yi Y G 2019 Results Phys. 15 102603
Google Scholar
[18] Wang X J, Meng H Y, Deng S Y, Lao C D, Wei Z C, Wang F H, Tan C G, Huang X 2019 Nanomaterials 9 385
Google Scholar
[19] Liu L, Xia S X, Luo X, Zhai X, Yu Y B, Wang L L 2018 Opt. Commun. 418 27
Google Scholar
[20] Waks E, Vuckovic J 2006 Phys. Rev. Lett. 96 153601
Google Scholar
[21] Marco P, Dario G, Liam O F, Claudio A L 2018 Opt. Express 26 8470
Google Scholar
[22] Li J J, Tian J P, Yang R C 2019 Eur. Phys. J. D 73 230
Google Scholar
[23] Han X, Wang T, Li X M, Liu B, He Y, Tang J 2015 J. Phys. D: Appl. Phys. 48 235102
Google Scholar
[24] Niu Y Y, Wang J C, Liu D D, Hu Z D, Sang T, Gao S M 2017 Optik 140 1038
Google Scholar
[25] Wang G X, Lu H, Liu X M 2012 Opt. Express 20 020902
Google Scholar
[26] Wen K H, Yan L S, Pan W, Luo B, Guo Z, Guo Y H, Luo X G 2014 J. Light. Technol. 32 1701
Google Scholar
[27] Cao G T, Li H J, Zhan S P, Xu H Q, Liu Z M, He Z H, Wang Y 2013 Opt. Express 21 9198
Google Scholar
[28] Wang Y Q, He Z H, Cui W, Ren X C, Li C J, Xue W W, Cao D M, Li G, Lei W L 2020 Results Phys. 16 102981
Google Scholar
[29] 李继军, 吴耀德, 宋明玉 2007 长江大学学报(自科版)理工卷 4 1673
Google Scholar
Li J J, Wu Y D, Song M Y 2007 J. Yangtze University (Nat. Sci. Edit) Sci. Eng. V. 4 1673
Google Scholar
[30] Li Z F, Wen K H, Fang Y H, Guo Z C 2020 IEEE J. Quantum Electron. 56 2982249
Google Scholar
[31] Qiong Z, Wang Z 2019 Opt. Express 27 303
Google Scholar
[32] Yin X G, Feng T H, Yip S, Liang Z X, Hui A, Ho J C, Li J S 2013 Appl. Phys. Lett. 103 021115
Google Scholar
[33] Xu H, Lu Y, Lee Y, Ham B S 2010 Opt. Express 18 17736
Google Scholar
[34] Zhu Y, Hu X Y, Yang H, Gong Q H 2014 Sci. Rep. UK 4 3752
Google Scholar
[35] 闫西成 2018 硕士学位论文(武汉:华中科技大学) (Wuhan: Huazhong University of Science & Technology)
Yan X C 2018 M. S. Thesis (Wuhan: Huazhong University of Science & Technology
[36] Ye C G, Zhang L 2008 Opt. Lett. 33 1911
Google Scholar
下载:
计量
- 文章访问数: 3823
- PDF下载量: 58
- 被引次数: 0
