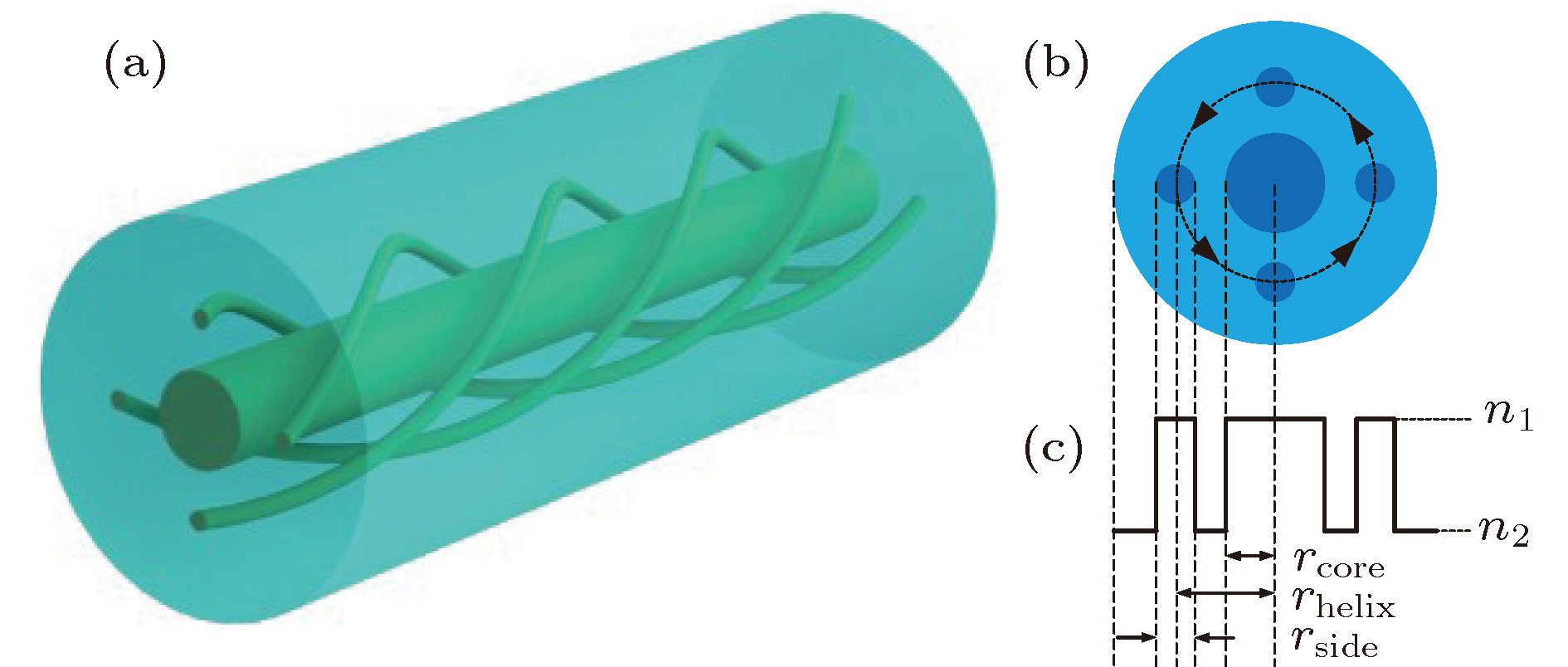
-
A type of fiber-based orbital angular momentum (OAM) modulator is designed according to transformation relation between OAM beam and optical fiber vector mode, together with mode-coupling theory, which is based on the combination of multimode fiber structure and chirally-coupled-cores structure. Instead of applying external force or grating etching to the fiber in the system, chirally-coupled-cores fiber can realize the modulation of any optical OAM by using single fiber at 1550 nm. Therefore, the test system is relatively simple. From the equation
${\rm{OAM}}_{ \pm l,n}^{ \pm \sigma } = {\rm{HE}}_{l + 1,n}^{{\rm{even}}} \pm {\rm{i}} \times {\rm{HE}}_{l + 1,n}^{{\rm{odd}}}$ , it can be seen that the OAM mode generated by long period chirally-coupled-cores fiber depends on the higher-order modes supported by the central fiber core. Therefore, the generation and modulation of any order OAM beam can be realized by changing the diameter of the central fiber core in theory. Through theoretical analysis and numerical simulation, the effects of different structure parameters on OAM modes are analyzed, including mode purity, mode transmission loss and effective refractive index. By keeping the propagation constants of the center core and side cores unchanged, the number of side cores has no effect on mode purity nor effective refractive index, but which is not for mode transmission loss. The loss of mode transmission increases with the increase of the number of side cores. However, it does not mean that the less number of side cores is a better case, in that the fiber symmetry and processing technology should also be considered. And the pitch calculated by the formula of phase matching condition can change in value within a certain numerical range without strongly affecting the mode purity and mode transmission loss. Pitch has a great influence on the effective refractive index of modes, therefore the pitch can be under control to change the difference in effective refractive index between OAM modes and reduce crosstalk between disparate modes. The distance between the center core and side cores of fiber has little effect on mode purity, great effect on mode transmission loss, but no effect on effective refractive index. Theoretically, the mode purity and mode transmission loss perform better with the distance between two kinds of cores increasing. But it will be limited by the fiber integration level.-
Keywords:
- orbital angular momentum /
- fiber /
- optical vortex /
- mode
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[2] Gong Y, Wang R, Deng Y, Zhang B, Nan W, Ning L, Pei W 2017 IEEE Trans. Antenn. Propag. 65 2940
Google Scholar
[3] Yan X, Guo L, Cheng M, Li J 2018 Opt. Express 26 12605
Google Scholar
[4] Bai X, Chen H, Ma Y, Yang H 2018 Progress in Electromagnetics Research Symposium-spring St. Petersburg, Russia, May 22−25, 2017 p3105
[5] Xing D, Liu J, Zeng X, Lu J, Yi Z 2018 Opt. Commun. 423 200
Google Scholar
[6] Jiang X, Liang B, Cheng J C, Qiu C W 2018 Adv. Mater. 30 1800257
Google Scholar
[7] Wang A, Zhu L, Wang L, Ai J, Chen S, Wang J 2018 Opt. Express 26 10038
Google Scholar
[8] Donato M G, Messina E, Foti A, Smart T J, Jones P H, Iatì M A, Saija R, Gucciardi P G, Maragò O M 2018 Nanoscale 10 1245
Google Scholar
[9] Zhou H L, Fu D Z, Dong J J, Pei Z, Chen D X, Cai X L, Li F L, Zhang X L 2017 Light-Sci. Appl. 6 e16251
Google Scholar
[10] Stefani A, Lwin R, Kuhlmey B T, Fleming S C 2018 Novel Optical Materials & Applications Zurich, Switzerland, July 2−5, 2018 NoTh1D.2
[11] Liang F, Padgett M J, Jian W 2017 Laser Photon. Rev. 11 1700183
Google Scholar
[12] Lin M, Yue G, Liu P, Liu J 2017 IEEE Trans. Antenn. Propag. 65 3510
Google Scholar
[13] Efron U 1994 Spatial Light Modulator Technology: Materials, Devices, and Applications (Vol. 47) (Florida: CRC Press) pp287−349
[14] Willner A E, Huang H, Yan Y, Ren Y, Ahmed N, Xie G, Bao C, Li L, Cao Y, Zhao Z, Wang J, Lavery M P J, Tur M, Ramachandran S, Molisch A F, Ashrafi N, Ashrafi S 2015 Adv. Opt. Photon. 7 66
Google Scholar
[15] Cheng C, Zhou G, Gai Z, Xu M, Hou Z, Xia C, Yuan J J 2016 Opt. Commun. 368 27
Google Scholar
[16] McGloin D, Simpson N B, Padgett M J 1998 Appl. Opt. 37 469
Google Scholar
[17] Ramachandran S 2010 IEEE Photinic Societys Meeting Denver, CO, USA, November 7−11, 2010 p679
[18] Alexeyev C N 2012 Appl. Opt. 51 6125
Google Scholar
[19] Swan M C, Liu C H, Guertin D, Jacobsen N, Tankala K, Galvanauskas A 2008 Conference on Optical Fiber Communication/National Fiber Optic Engineers Conference San Diego, CA, USA, February 24−28, 2008 paper OWU2
[20] Ma X, Liu C H, Chang G, Galvanauskas A 2011 Opt. Express 19 26515
Google Scholar
[21] 杜城, 陈伟, 李诗愈, 莫琦, 张涛, 柯一礼 2013 中国专利 CN103204629B
Du C, Chen W, Li S Y, Mo Q, Zhang T, Ke Y L 2013 CN Patent CN103204629B (in Chinese)
[22] Nicolet A, Zolla F, Guenneau S 2004 Eur. Phys. J. Appl. Phys. 28 153
Google Scholar
[23] 许华醒 2013 博士论文 (合肥: 中国科学技术大学)
Xu H 2013 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[24] Zheng S, Wang J 2017 Opt. Express 25 18492
Google Scholar
[25] Li S, Wang J 2014 Sci. Rep. 4 3853
Google Scholar
-
图 1 长周期手性耦合光纤结构(N = 4) (a)三维示意图; (b)横截面; (c) 折射率分布
Fig. 1. Structure of long-period chirally-coupled-core fiber (N = 4): (a) Three-dimensional diagram; (b) cross section; (c) refractive-index profile.
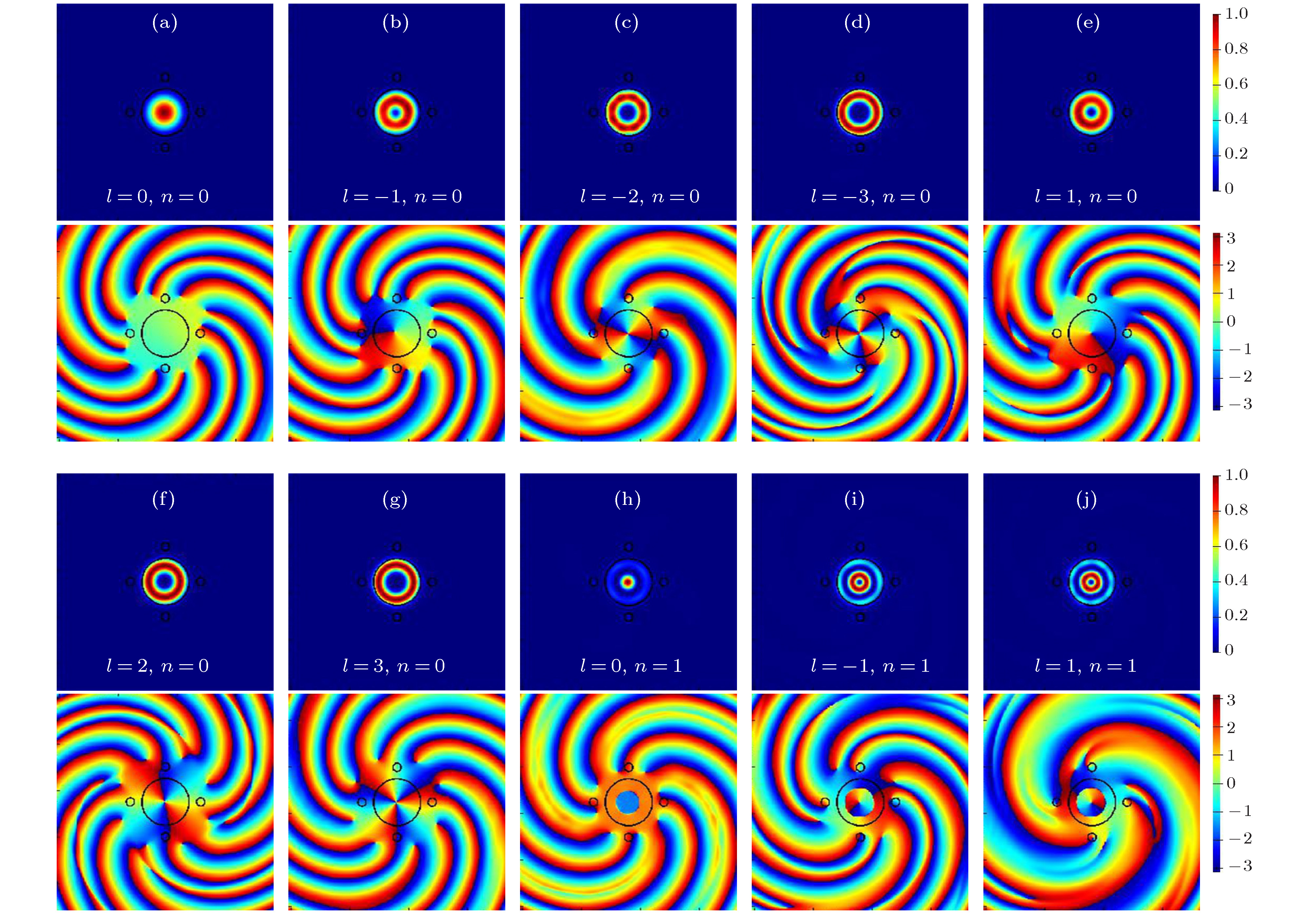
图 2 光纤OAM模式的场强和相位分布 (a)—(g)径向拓扑荷为0, 角向拓扑荷为0, –1, –2, –3, 1, 2, 3; (h)—(j)径向拓扑荷为1, 角向拓扑荷为0, –1, 1
Fig. 2. Field intensity and phase distribution of fiber OAM mode: (a)−(g) Radial topological charge of 0, angular topological charge of 0, –1, –2, –3, 1, 2, 3; (h)−(j) radial topological charge of 1, angular topological charge of 0, –1, 1.
图 3 (a)
$\varLambda = 4600\;{\text{μ}}{\rm{m}}$ 时, 多组rhelix值下N对OAM模式的影响; (b) rhelix = 40${\text{μ}}{\rm{m}}$ 时, 多组$\varLambda $ 值下N对OAM模式的影响; (c) rhelix = 45${\text{μ}}{\rm{m}}$ ,$\varLambda = 4600\;{\text{μ}}{\rm{m}}$ 下N对OAM模式的影响; 其中结构参数n1 = 1.453, n2 = 1.45, rcore = 20${\text{μ}}{\rm{m}}$ , rside = 3.5${\text{μ}}{\rm{m}}$ Fig. 3. (a) Effect of N on OAM modes under multiple values of rhelix when
$\varLambda = 4600\;{\text{μ}}{\rm{m}}$ ; (b) effect of N on OAM modes under multiple values of$\varLambda $ when rhelix = 40${\text{μ}}{\rm{m}}$ ; (c) effect of N on OAM modes when rhelix = 45${\text{μ}}{\rm{m}}$ ,$\varLambda = 4600\;{\text{μ}}{\rm{m}}$ . n1 = 1.453, n2 = 1.45, rcore = 20${\text{μ}}{\rm{m}}$ , rside = 3.5${\text{μ}}{\rm{m}}$ .
图 4 (a) rhelix = 35
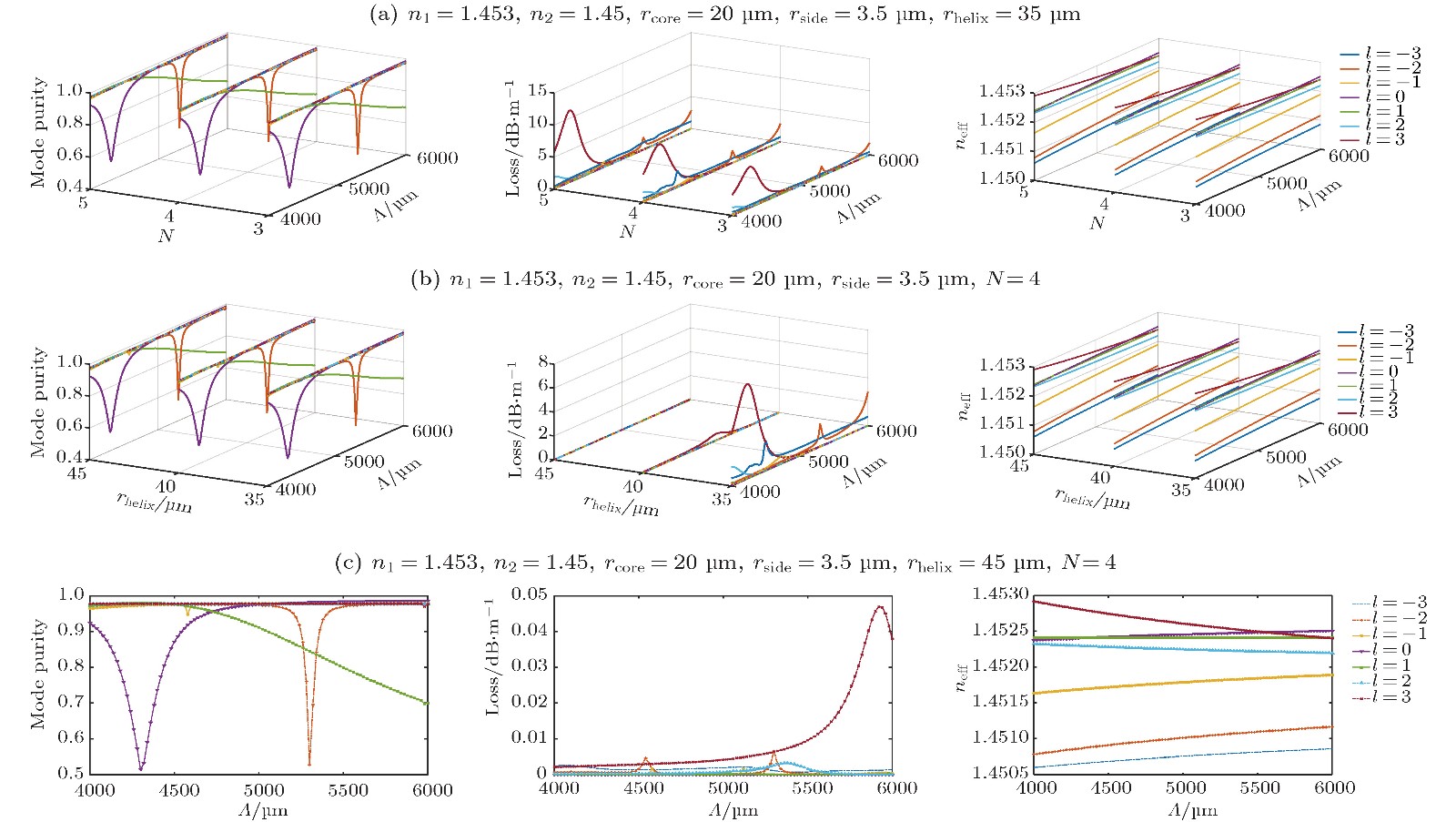
${\text{μ}}{\rm{m}}$ 时, 多组N值下$\varLambda $ 对OAM模式的影响; (b) N = 4时, 多组rhelix值下$\varLambda $ 对OAM模式的影响; (c) rhelix = 45${\text{μ}}{\rm{m}}$ , N = 4下$ \varLambda$ 对OAM模式的影响; 其中结构参数n1 = 1.453, n2 = 1.45, rcore = 20${\text{μ}}{\rm{m}}$ , rside = 3.5${\text{μ}}{\rm{m}}$ Fig. 4. (a) Effect of
$\varLambda $ on OAM modes under multiple values of N when rhelix = 35${\text{μ}}{\rm{m}}$ ; (b) effect of$\varLambda $ on OAM modes under multiple values of rhelix when N = 4; (c) effect of$\varLambda $ on OAM modes when rhelix = 45${\text{μ}}{\rm{m}}$ , N = 4. n1 = 1.453, n2 = 1.45, rcore = 20${\text{μ}}{\rm{m}}$ , rside = 3.5${\text{μ}}{\rm{m}}$ .
图 5 (a)
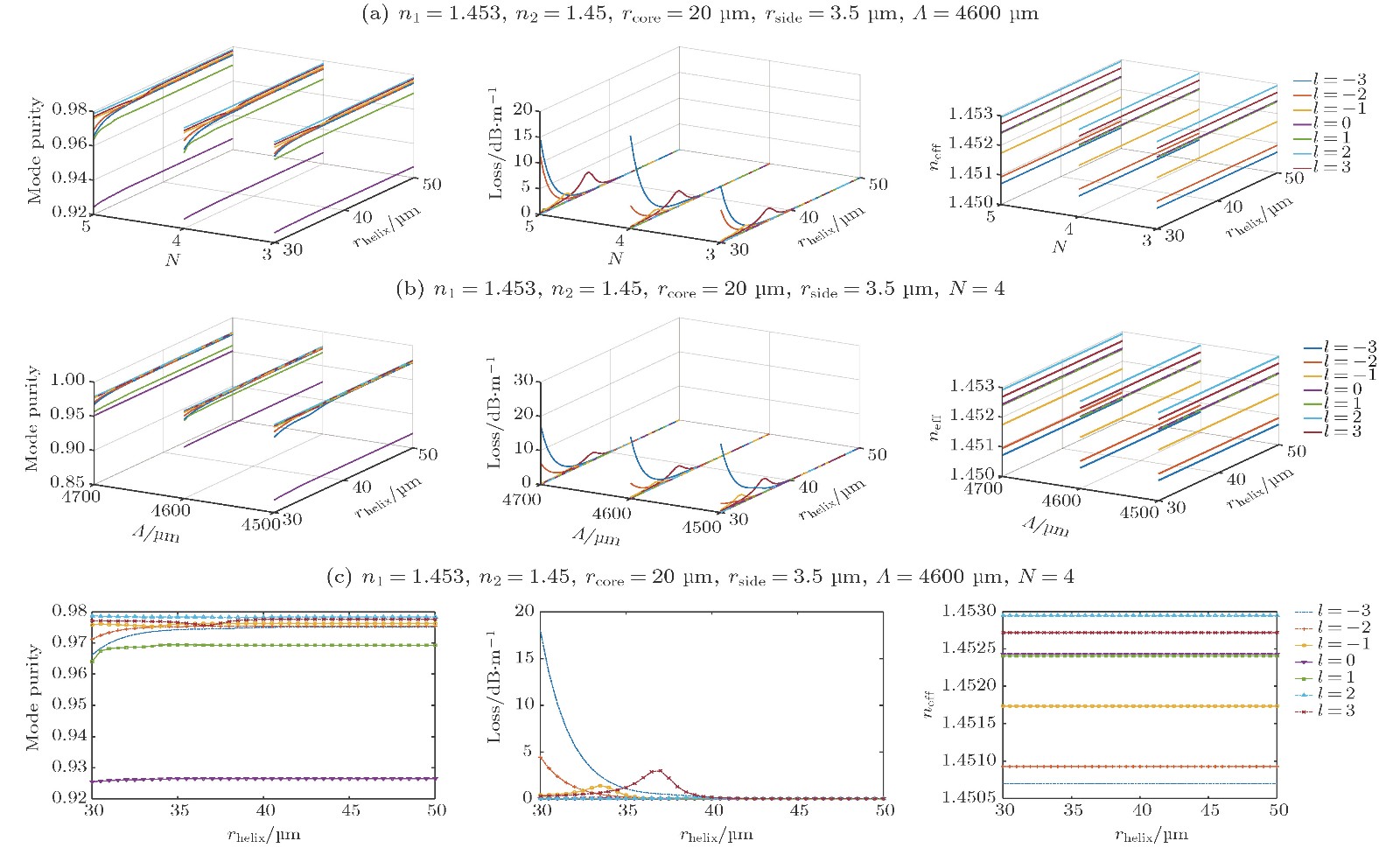
$\varLambda = 4600\; {\text{μ}}{\rm{m}}$ 时多组N值下rhelix对OAM模式的影响; (b) N = 4时, 多组$\varLambda $ 值下rhelix对OAM模式的影响; (c)$\varLambda = 4600\;{\text{μ}}{\rm{m}}$ , N = 4下$ r_{\rm helix}$ 对OAM模式的影响; 其中结构参数n1 = 1.453, n2 = 1.45, rcore = 20${\text{μ}}{\rm{m}}$ , rside = 3.5${\text{μ}}{\rm{m}}$ Fig. 5. (a) Effect of rhelix on OAM modes under multiple values of N when
$\varLambda = 4600\; {\text{μ}}{\rm{m}}$ ; (b) effect of rhelix on OAM modes under multiple values of$\varLambda $ when N = 4; (c) effect of$r_{\rm helix} $ on OAM modes when$\varLambda = 4600\;{\text{μ}}{\rm{m}}$ , N = 4. n1 = 1.453, n2 = 1.45, rcore = 20${\text{μ}}{\rm{m}}$ , rside = 3.5${\text{μ}}{\rm{m}}$ . -
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[2] Gong Y, Wang R, Deng Y, Zhang B, Nan W, Ning L, Pei W 2017 IEEE Trans. Antenn. Propag. 65 2940
Google Scholar
[3] Yan X, Guo L, Cheng M, Li J 2018 Opt. Express 26 12605
Google Scholar
[4] Bai X, Chen H, Ma Y, Yang H 2018 Progress in Electromagnetics Research Symposium-spring St. Petersburg, Russia, May 22−25, 2017 p3105
[5] Xing D, Liu J, Zeng X, Lu J, Yi Z 2018 Opt. Commun. 423 200
Google Scholar
[6] Jiang X, Liang B, Cheng J C, Qiu C W 2018 Adv. Mater. 30 1800257
Google Scholar
[7] Wang A, Zhu L, Wang L, Ai J, Chen S, Wang J 2018 Opt. Express 26 10038
Google Scholar
[8] Donato M G, Messina E, Foti A, Smart T J, Jones P H, Iatì M A, Saija R, Gucciardi P G, Maragò O M 2018 Nanoscale 10 1245
Google Scholar
[9] Zhou H L, Fu D Z, Dong J J, Pei Z, Chen D X, Cai X L, Li F L, Zhang X L 2017 Light-Sci. Appl. 6 e16251
Google Scholar
[10] Stefani A, Lwin R, Kuhlmey B T, Fleming S C 2018 Novel Optical Materials & Applications Zurich, Switzerland, July 2−5, 2018 NoTh1D.2
[11] Liang F, Padgett M J, Jian W 2017 Laser Photon. Rev. 11 1700183
Google Scholar
[12] Lin M, Yue G, Liu P, Liu J 2017 IEEE Trans. Antenn. Propag. 65 3510
Google Scholar
[13] Efron U 1994 Spatial Light Modulator Technology: Materials, Devices, and Applications (Vol. 47) (Florida: CRC Press) pp287−349
[14] Willner A E, Huang H, Yan Y, Ren Y, Ahmed N, Xie G, Bao C, Li L, Cao Y, Zhao Z, Wang J, Lavery M P J, Tur M, Ramachandran S, Molisch A F, Ashrafi N, Ashrafi S 2015 Adv. Opt. Photon. 7 66
Google Scholar
[15] Cheng C, Zhou G, Gai Z, Xu M, Hou Z, Xia C, Yuan J J 2016 Opt. Commun. 368 27
Google Scholar
[16] McGloin D, Simpson N B, Padgett M J 1998 Appl. Opt. 37 469
Google Scholar
[17] Ramachandran S 2010 IEEE Photinic Societys Meeting Denver, CO, USA, November 7−11, 2010 p679
[18] Alexeyev C N 2012 Appl. Opt. 51 6125
Google Scholar
[19] Swan M C, Liu C H, Guertin D, Jacobsen N, Tankala K, Galvanauskas A 2008 Conference on Optical Fiber Communication/National Fiber Optic Engineers Conference San Diego, CA, USA, February 24−28, 2008 paper OWU2
[20] Ma X, Liu C H, Chang G, Galvanauskas A 2011 Opt. Express 19 26515
Google Scholar
[21] 杜城, 陈伟, 李诗愈, 莫琦, 张涛, 柯一礼 2013 中国专利 CN103204629B
Du C, Chen W, Li S Y, Mo Q, Zhang T, Ke Y L 2013 CN Patent CN103204629B (in Chinese)
[22] Nicolet A, Zolla F, Guenneau S 2004 Eur. Phys. J. Appl. Phys. 28 153
Google Scholar
[23] 许华醒 2013 博士论文 (合肥: 中国科学技术大学)
Xu H 2013 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[24] Zheng S, Wang J 2017 Opt. Express 25 18492
Google Scholar
[25] Li S, Wang J 2014 Sci. Rep. 4 3853
Google Scholar
下载:
计量
- 文章访问数: 8304
- PDF下载量: 104
- 被引次数: 0
