-
在集成光学模块的一体化离子阱中, 极易出现光学焦点与囚禁离子鞍点错位的问题, 严重阻碍了该实验方法的实用性. 为解决该问题, 可利用多射频场方法对离子鞍点位置进行补偿和移动. 然而, 在实际实验过程中, 多射频方法的应用, 需要知道鞍点实际空间位置对应的应加载射频电压幅值. 这就需要建立一套数学模型, 对二者关系进行描述. 模型的精确程度决定了鞍点空间位置的控制精度、模型的简易程度决定了求解过程的速度. 因此, 本文提出一种基于数值仿真电场分布结果和多项式拟合方法而建立的多射频电场电压和鞍点位置关系的数学模型, 可以在无需考虑物理机制和模型基础上, 快速、准确地给出二者之间的数学描述. 本文利用数值方法对该模型的正确性和适用范围进行了验证和讨论, 可以在实验中快速准确地给出应加载射频电压幅值, 使鞍点移动并与光学焦点重合, 该方法极大地降低了由于求解引起的时间延迟、提高了鞍点位置移动过程中反馈环路带宽.In an integrated ion trap with integrated optical modules, the problem of misalignment between the optical focus and the trapped ion saddle point is very likely to occur, which seriously hinders the practicality of the experimental method. To solve this problem, the multi-RF field method can be used to compensate for and move the ion saddle point position. However, in the actual experimental process, the application of the multi-RF method requires the knowledge of the amplitude of the RF voltage to be loaded corresponding to the actual spatial position of the saddle point. Therefore, a set of mathematical models is established to describe the relationship. The accuracy of the model determines the control accuracy of the spatial position of the saddle point, and the simplicity of the model determines the speed of the solution process. Therefore, in this work, a mathematical model of the relationship between the multi-RF electric field voltage and the saddle point position is proposed based on the numerically simulated electric field distribution and the polynomial fitting method. It can quickly and accurately give a mathematical description between the two without considering the physical mechanism or model. Numerical method is adopted to verify and discuss the correctness and scope of application of the model, and can quickly and accurately provide the amplitude of the RF voltage to be loaded in the experiment, causing the saddle point to move and coincide with the optical focus. This method greatly reduces the time delay caused by the solution and improves the feedback loop bandwidth during the movement of the saddle point position.
-
Keywords:
- surface ion trap /
- saddle point movement /
- multiple RF fields control /
- boundary element analysis
[1] Siverns J D, Quraishi Q 2017 Quantum Inf. Process. 16 314
Google Scholar
[2] Ding X Y, Yu Q, Lu X Q, Wang X H, Huo X M, Qian X 2023 Anal. Chem. 95 2348
Google Scholar
[3] Papanastasiou D, Kounadis D, Lekkas A, et al. 2022 J. Am. Soc. Mass Spectrom. 33 1990
Google Scholar
[4] Ozawa A, Davila-Rodriguez J, Bounds J R, Schuessler H A, Hänsch T W, Udem T 2017 Nat. Commun. 8 44
Google Scholar
[5] Amitrano V, Roggero A, Luchi P, Turro F, Vespucci L, Pederiva F 2023 Phys. Rev. D 107 023007
Google Scholar
[6] Monroe C, Campbell W C, Duan L M, et al. 2021 Rev. Mod. Phys. 93 025001
Google Scholar
[7] Niroula P, Shaydulin R, Yalovetzky R, Minssen P, Herman D, Hu S, Pistoia M 2022 Sci. Rep. 12 17171
Google Scholar
[8] Pogorelov I, Feldker T, Marciniak C D, et al. 2021 PRX Quantum 2 020343
Google Scholar
[9] Wang P P, Luan C Y, Qiao M, Um M, Zhang J H, Wang Y, Yuan X, Gu M L, Zhang J N, Kim K 2021 Nat. Commun. 12 233
Google Scholar
[10] Manovitz T, Shapira Y, Gazit L, Akerman N, Ozeri R 2022 PRX Quantum 3 010347
Google Scholar
[11] Mehta K K, Bruzewicz C D, McConnell R, Ram R J, Sage J M, Chiaverini J 2016 Nature Nanotech. 11 1066
Google Scholar
[12] Ballance C J, Harty T P, Linke N M, Sepiol M A, Lucas D M 2016 Phys. Rev. Lett. 117 060504
Google Scholar
[13] Rudolph M S, Toussaint N B, Katabarwa A, Johri S, Peropadre B, Perdomo-Ortiz A 2022 Phys. Rev. X 12 031010
Google Scholar
[14] 范桁 2018 67 120301
Google Scholar
Fan H 2018 Acta Physica Sinica 67 120301
Google Scholar
[15] Murali P, Debroy D M, Brown K R, Martonosi M 2022 Commun. ACM 65 101
Google Scholar
[16] Pino J M, Dreiling J M, Figgatt C, Gaebler J P, Moses S A, Allman M, Baldwin C, Foss-Feig M, Hayes D, Mayer K 2021 Nature 592 209
Google Scholar
[17] Ivory M, Setzer W J, Karl N, McGuinness H, DeRose C, Blain M, Stick D, Gehl M, Parazzoli L P 2021 Phys. Rev. X 11 041033
Google Scholar
[18] Malinowski M, Allcock D T C, Ballance C J 2023 PRX Quantum 4 040313
Google Scholar
[19] Romaszko Z D, Hong S, Siegele M, Puddy R K, Lebrun-Gallagher F R, Weidt S, Hensinger W K 2020 Nat. Rev. Phys. 2 285
Google Scholar
[20] Wang Y H, Li Y, Yin Z Q, Zeng B 2018 npj Quantum Inf. 4 46
Google Scholar
[21] Ryan-Anderson C, Bohnet J G, Lee K, et al. 2021 Phys. Rev. X 11 041058
Google Scholar
[22] Mehta K K, Zhang C, Malinowski M, Nguyen T L, Stadler M, Home J P 2020 Nature 586 533
Google Scholar
[23] Bao X Y, Cui J M, Fang D, Chen W B, Wang J, Huang Y F, Li C F, Guo G C 2023 JUSTC 53 0705
Google Scholar
[24] Van Rynbach A, Maunz P, Kim J 2016 Appl. Phys. Lett. 109 221108
Google Scholar
[25] Wang Z, Wang B R, Ma Q L, Guo J Y, Li M S, Wang Y, Rao X X, Huang Z Q, Luo L 2020 arXiv: 2004.08845 [quant-ph]
[26] Holz P C, Auchter S, Stocker G, Valentini M, Lakhmanskiy K, Rössler C, Stampfer P, Sgouridis S, Aschauer E, Colombe Y, Blatt R 2020 Adv. Quantum Technol. 3 2000031
Google Scholar
[27] Liu Y R, Wang Z, Xiang Z X, Wang Q K, Hu T Y, Wang X 2024 Chip 3 100078
Google Scholar
[28] Hong S, Lee M, Cheon H, Kim T, Cho D I 2016 Sensors 16 616
Google Scholar
[29] Read F H, Bowring N J 2011 Nucl. Instr. and Meth. A 645 273
Google Scholar
[30] House M G 2008 Phys. Rev. A 78 033402
Google Scholar
[31] Lauprêtre T, Achi B, Groult L, Carry É, Kersalé Y, Delehaye M, Hafiz M A, Lacroûte C 2023 Appl. Phys. B 129 37
Google Scholar
[32] Zhang X, Ou B, Chen T, Xie Y, Wu W, Chen P 2020 Phys. Scr. 95 045103
Google Scholar
[33] Zhang C, Mehta K K, Home J P 2022 New J. Phys. 24 073030
Google Scholar
[34] 王晨旭, 贺冉, 李睿睿, 陈炎, 房鼎, 崔金明, 黄运锋, 李传锋, 郭光灿 2022 71 133701
Google Scholar
Wang C X, He R, Li R R, Chen Y, Fang D, Cui J M, Huang Y F, Li C F, Guo G C 2022 Acta Phys. Sin. 71 133701
Google Scholar
[35] Kumph M, Holz P, Langer K, Meraner M, Niedermayr M, Brownnutt M, Blatt R 2016 New J. Phys. 18 023047
Google Scholar
[36] Dehmelt H G 1968 Adv. At. Mol. Phys. 3 53
Google Scholar
[37] Niedermayr M, Lakhmanskiy K, Kumph M, Partel S, Edlinger J, Brownnutt M, Blatt R 2014 New J. Phys. 16 113068
Google Scholar
[38] 吴宇恺, 段路明 2023 72 230302
Google Scholar
Wu Y K, Duan L M 2023 Acta Phys. Sin. 72 230302
Google Scholar
-
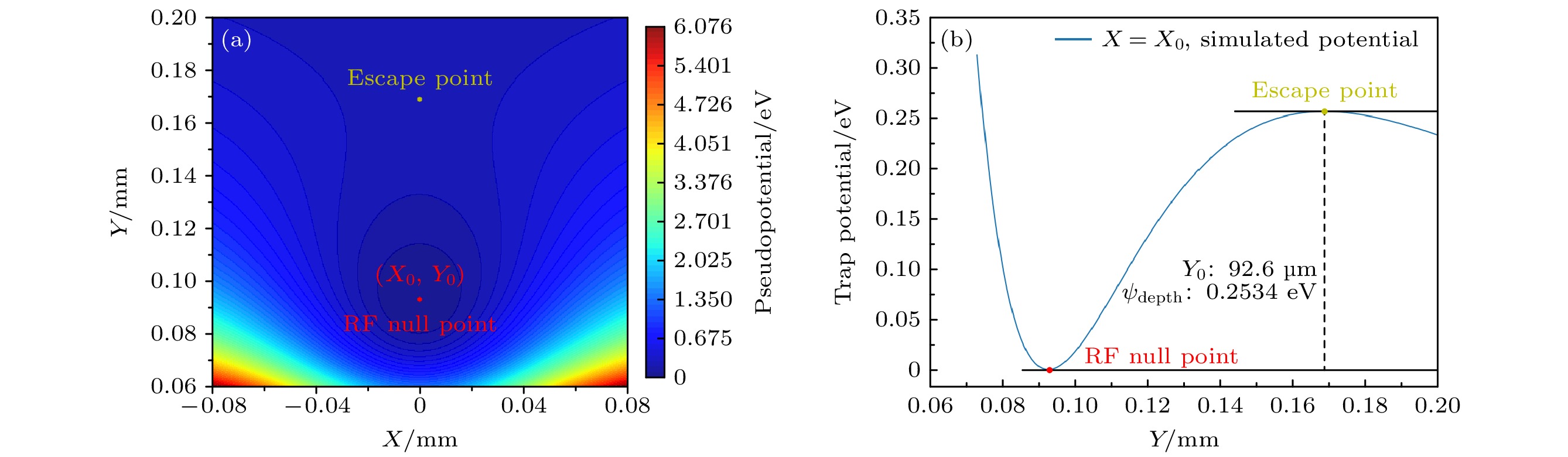
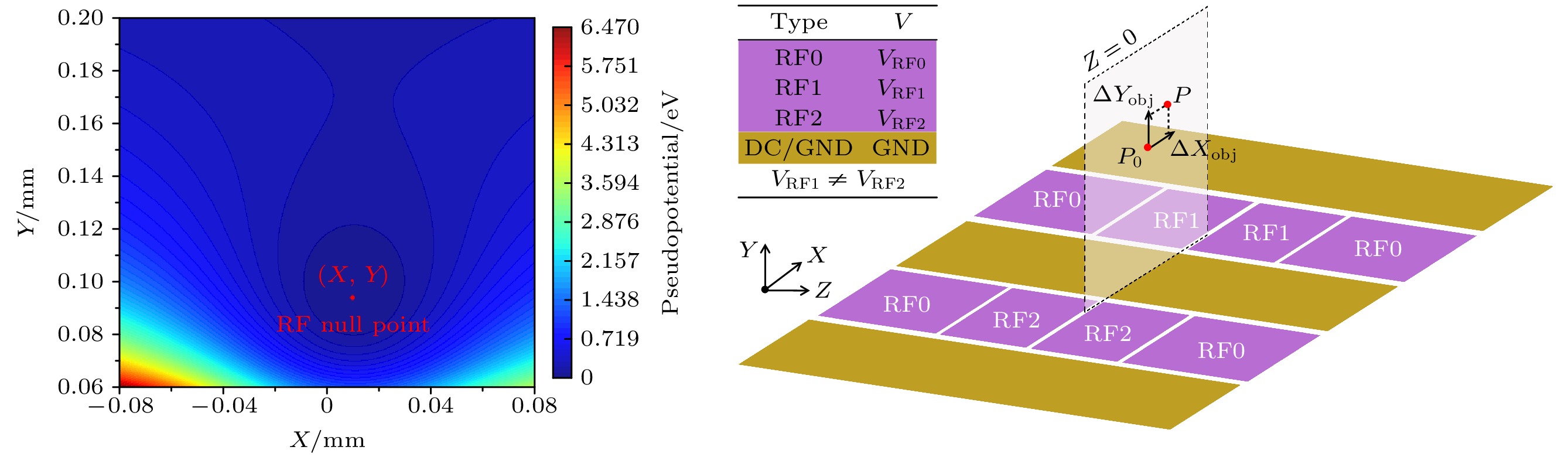
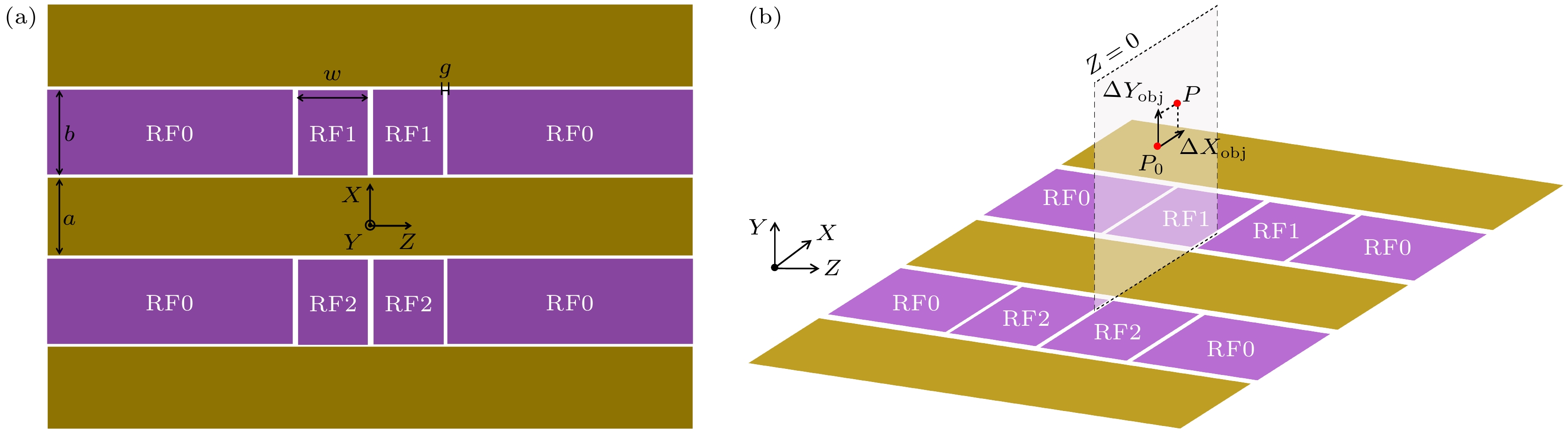
图 1 建立多射频场驱动鞍点移动的基本电极结构 (a) 射频电极经分段处理的表面离子阱结构; (b) 调节多射频电压幅值驱动鞍点径向移动
Fig. 1. Establishing the basic electrode structure for multi-RF field driven saddle point movement: (a) Surface ion trap structure after radio frequency electrode segmentation treatment; (b) adjusting the amplitude of multiple RF voltages to drive the radial movement of the saddle point.
图 2 赝势分布情况 (a) 研究对象Z = 0处初始赝势分布; (b) 径向面内赝势梯度变化最小方向上的赝势分布
Fig. 2. Pseudopotential distribution: (a) Initial pseudopotential distribution on the radial plane Z = 0; (b) pseudopotential distribution in the direction with the smallest pseudopotential gradient change in the radial plane.
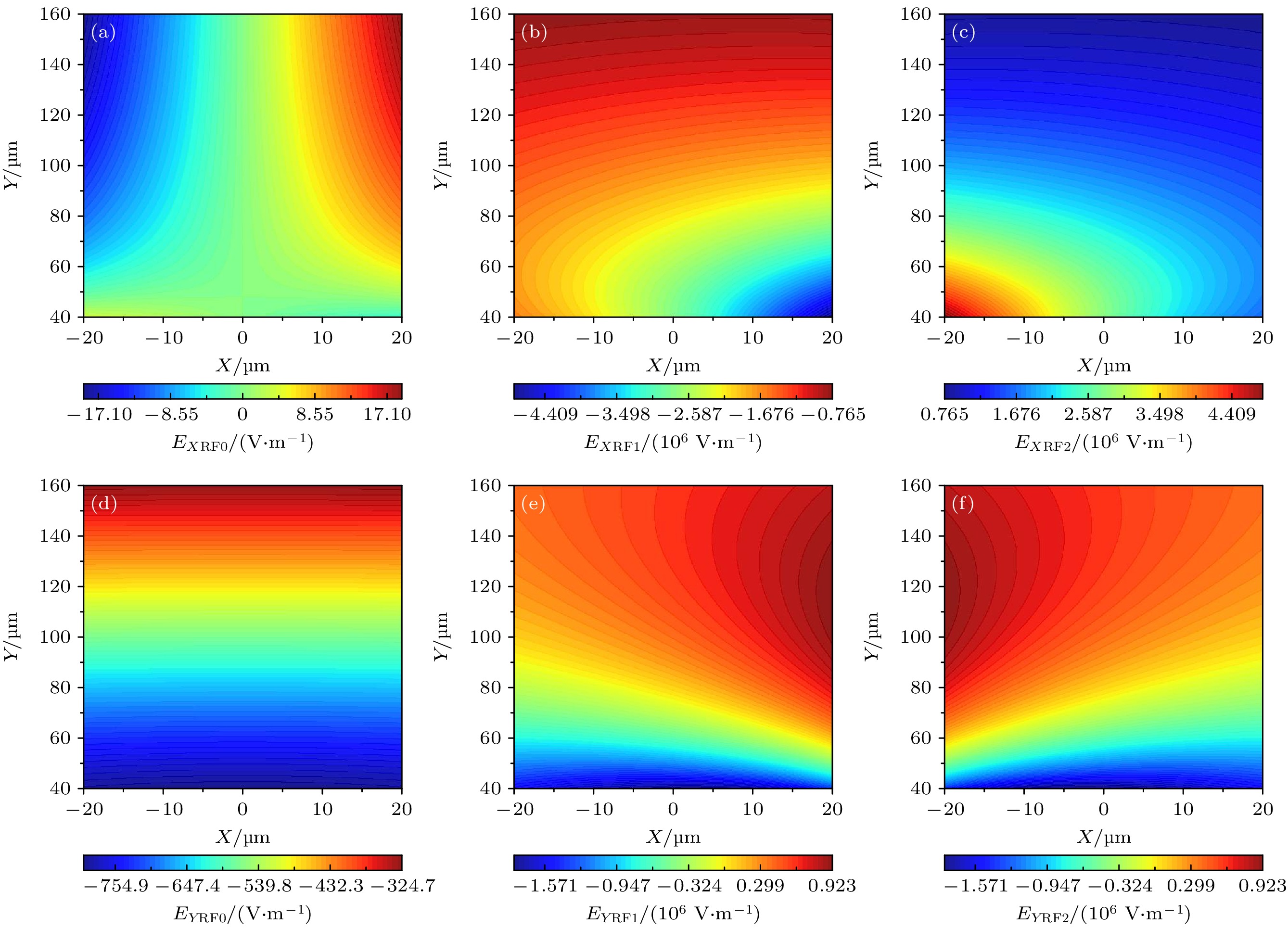
图 3 射频电极组各电极RFi (i = 0—2)依次施加单位电压时, Z = 0处电场分布情况 (a) RF0作用下X方向的电场分布EXRF0; (b) RF1作用下X方向的电场分布EXRF1; (c) RF2作用下X方向的电场分布EXRF2; (d) RF0作用下Y方向的电场分布EYRF0; (e) RF1作用下Y方向的电场分布EYRF1; (f) RF2作用下Y方向的电场分布EYRF2
Fig. 3. When unit voltage is applied to each electrode RFi (i = 0—2) of the RF electrode group in turn, the electric field distribution at Z = 0: (a) Electric field distribution EXRF0 in the X direction under the action of electrode RF0; (b) electric field distribution EXRF1 in the X direction under the action of electrode RF1; (c) electric field distribution EXRF2 in the X direction under the action of electrode RF2; (d) electric field distribution in the Y direction under the action of electrode RF0; (e) electric field distribution in the Y direction under the action of electrode RF1; (f) electric field distribution in the Y direction under the action of electrode RF2.
图 4 鞍点模拟移动量ΔXBEM, ΔYBEM与目标移动量ΔXobj, ΔYobj间的误差情况 (a) 模型1的Xerr分布; (b) 模型1的Yerr分布
Fig. 4. Error between the simulated displacements of the saddle point (ΔXBEM, ΔYBEM) and the target displacements (ΔXobj, ΔYobj): (a) Xerr distribution of model 1; (b) Yerr distribution of model 1.
图 5 鞍点模拟位移量(ΔXBEM, ΔYBEM)与目标移动量(ΔXobj, ΔYobj)间的误差情况 (a) 模型2的Xerr分布; (b) 模型2的Yerr分布
Fig. 5. Error between the simulated displacements of the saddle point (ΔXBEM, ΔYBEM) and the target displacements (ΔXobj, ΔYobj): (a) Xerr distribution of model 2; (b) Yerr distribution of model 2.
图 6 鞍点模拟位移量(ΔXBEM, ΔYBEM)与目标移动量(ΔXobj, ΔYobj)间的误差情况 (a) 模型3的Xerr分布; (b) 模型3的Yerr分布
Fig. 6. Error between the simulated displacements of the saddle point (ΔXBEM, ΔYBEM) and the target displacements (ΔXobj, ΔYobj): (a) Xerr distribution of model 3; (b) Yerr distribution of model 3.
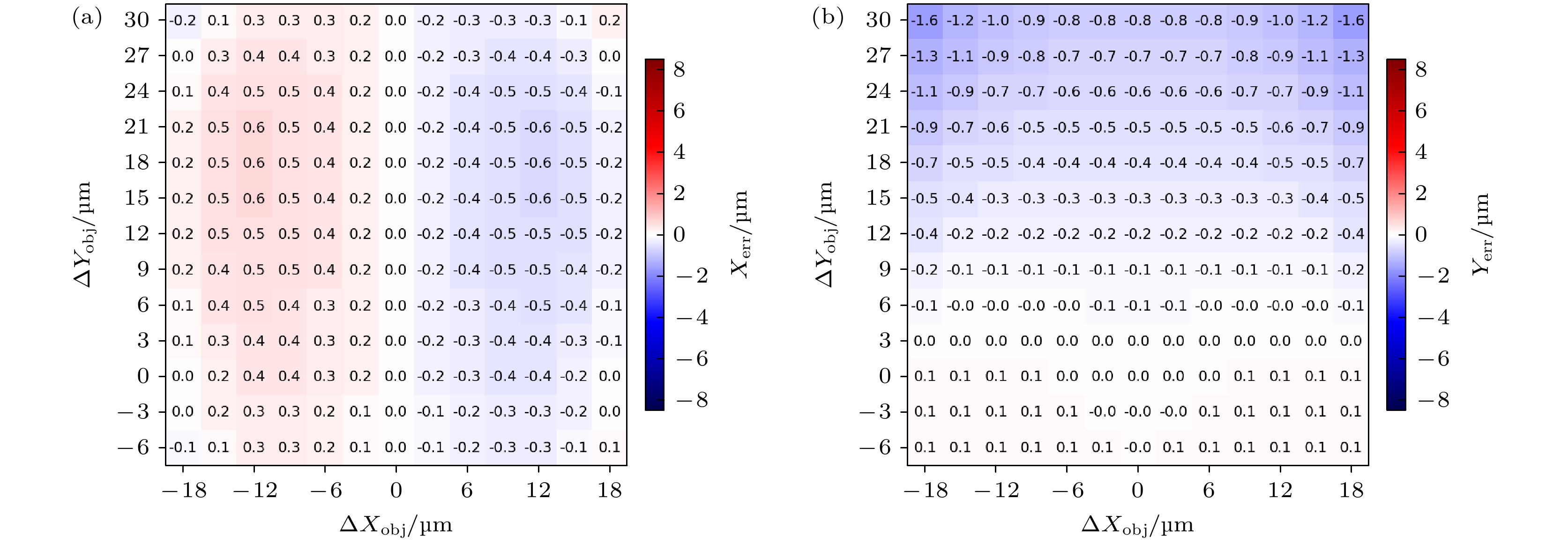
表 1 初步拟合1的拟合情况
Table 1. The fitting situation of the preliminary fit 1.
EXRF0(X, Y) EXRF1(X, Y) EXRF2(X, Y) EYRF0(X, Y) EYRF1(X, Y) EYRF2(X, Y) X阶数 1 2 2 1 2 2 Y阶数 3 3 3 1 3 3 Adj R-sq 0.9979 0.9977 0.9977 0.9992 0.9963 0.9963 
下载: 导出CSV
表 2 拟合2的拟合情况
Table 2. The fitting situation of the fit 2.
EXRF0(X, Y) EXRF1(X, Y) EXRF2(X, Y) EYRF0(X, Y) EYRF1(X, Y) EYRF2(X, Y) X阶数 1 2 2 1 2 2 Y阶数 4 4 4 1 4 4 Adj R-sq 0.9996 0.9997 0.9997 0.9992 0.9994 0.9994
下载: 导出CSV
表 3 拟合3的拟合情况
Table 3. The fitting situation of the fit 3.
EXRF0(X, Y) EXRF1(X, Y) EXRF2(X, Y) EYRF0(X, Y) EYRF1(X, Y) EYRF2(X, Y) X阶数 5 5 5 5 5 5 Y阶数 5 5 5 5 5 5 Adj R-sq 1.0000 1.0000 1.0000 1.0000 0.9999 0.9999
下载: 导出CSV
-
[1] Siverns J D, Quraishi Q 2017 Quantum Inf. Process. 16 314
Google Scholar
[2] Ding X Y, Yu Q, Lu X Q, Wang X H, Huo X M, Qian X 2023 Anal. Chem. 95 2348
Google Scholar
[3] Papanastasiou D, Kounadis D, Lekkas A, et al. 2022 J. Am. Soc. Mass Spectrom. 33 1990
Google Scholar
[4] Ozawa A, Davila-Rodriguez J, Bounds J R, Schuessler H A, Hänsch T W, Udem T 2017 Nat. Commun. 8 44
Google Scholar
[5] Amitrano V, Roggero A, Luchi P, Turro F, Vespucci L, Pederiva F 2023 Phys. Rev. D 107 023007
Google Scholar
[6] Monroe C, Campbell W C, Duan L M, et al. 2021 Rev. Mod. Phys. 93 025001
Google Scholar
[7] Niroula P, Shaydulin R, Yalovetzky R, Minssen P, Herman D, Hu S, Pistoia M 2022 Sci. Rep. 12 17171
Google Scholar
[8] Pogorelov I, Feldker T, Marciniak C D, et al. 2021 PRX Quantum 2 020343
Google Scholar
[9] Wang P P, Luan C Y, Qiao M, Um M, Zhang J H, Wang Y, Yuan X, Gu M L, Zhang J N, Kim K 2021 Nat. Commun. 12 233
Google Scholar
[10] Manovitz T, Shapira Y, Gazit L, Akerman N, Ozeri R 2022 PRX Quantum 3 010347
Google Scholar
[11] Mehta K K, Bruzewicz C D, McConnell R, Ram R J, Sage J M, Chiaverini J 2016 Nature Nanotech. 11 1066
Google Scholar
[12] Ballance C J, Harty T P, Linke N M, Sepiol M A, Lucas D M 2016 Phys. Rev. Lett. 117 060504
Google Scholar
[13] Rudolph M S, Toussaint N B, Katabarwa A, Johri S, Peropadre B, Perdomo-Ortiz A 2022 Phys. Rev. X 12 031010
Google Scholar
[14] 范桁 2018 67 120301
Google Scholar
Fan H 2018 Acta Physica Sinica 67 120301
Google Scholar
[15] Murali P, Debroy D M, Brown K R, Martonosi M 2022 Commun. ACM 65 101
Google Scholar
[16] Pino J M, Dreiling J M, Figgatt C, Gaebler J P, Moses S A, Allman M, Baldwin C, Foss-Feig M, Hayes D, Mayer K 2021 Nature 592 209
Google Scholar
[17] Ivory M, Setzer W J, Karl N, McGuinness H, DeRose C, Blain M, Stick D, Gehl M, Parazzoli L P 2021 Phys. Rev. X 11 041033
Google Scholar
[18] Malinowski M, Allcock D T C, Ballance C J 2023 PRX Quantum 4 040313
Google Scholar
[19] Romaszko Z D, Hong S, Siegele M, Puddy R K, Lebrun-Gallagher F R, Weidt S, Hensinger W K 2020 Nat. Rev. Phys. 2 285
Google Scholar
[20] Wang Y H, Li Y, Yin Z Q, Zeng B 2018 npj Quantum Inf. 4 46
Google Scholar
[21] Ryan-Anderson C, Bohnet J G, Lee K, et al. 2021 Phys. Rev. X 11 041058
Google Scholar
[22] Mehta K K, Zhang C, Malinowski M, Nguyen T L, Stadler M, Home J P 2020 Nature 586 533
Google Scholar
[23] Bao X Y, Cui J M, Fang D, Chen W B, Wang J, Huang Y F, Li C F, Guo G C 2023 JUSTC 53 0705
Google Scholar
[24] Van Rynbach A, Maunz P, Kim J 2016 Appl. Phys. Lett. 109 221108
Google Scholar
[25] Wang Z, Wang B R, Ma Q L, Guo J Y, Li M S, Wang Y, Rao X X, Huang Z Q, Luo L 2020 arXiv: 2004.08845 [quant-ph]
[26] Holz P C, Auchter S, Stocker G, Valentini M, Lakhmanskiy K, Rössler C, Stampfer P, Sgouridis S, Aschauer E, Colombe Y, Blatt R 2020 Adv. Quantum Technol. 3 2000031
Google Scholar
[27] Liu Y R, Wang Z, Xiang Z X, Wang Q K, Hu T Y, Wang X 2024 Chip 3 100078
Google Scholar
[28] Hong S, Lee M, Cheon H, Kim T, Cho D I 2016 Sensors 16 616
Google Scholar
[29] Read F H, Bowring N J 2011 Nucl. Instr. and Meth. A 645 273
Google Scholar
[30] House M G 2008 Phys. Rev. A 78 033402
Google Scholar
[31] Lauprêtre T, Achi B, Groult L, Carry É, Kersalé Y, Delehaye M, Hafiz M A, Lacroûte C 2023 Appl. Phys. B 129 37
Google Scholar
[32] Zhang X, Ou B, Chen T, Xie Y, Wu W, Chen P 2020 Phys. Scr. 95 045103
Google Scholar
[33] Zhang C, Mehta K K, Home J P 2022 New J. Phys. 24 073030
Google Scholar
[34] 王晨旭, 贺冉, 李睿睿, 陈炎, 房鼎, 崔金明, 黄运锋, 李传锋, 郭光灿 2022 71 133701
Google Scholar
Wang C X, He R, Li R R, Chen Y, Fang D, Cui J M, Huang Y F, Li C F, Guo G C 2022 Acta Phys. Sin. 71 133701
Google Scholar
[35] Kumph M, Holz P, Langer K, Meraner M, Niedermayr M, Brownnutt M, Blatt R 2016 New J. Phys. 18 023047
Google Scholar
[36] Dehmelt H G 1968 Adv. At. Mol. Phys. 3 53
Google Scholar
[37] Niedermayr M, Lakhmanskiy K, Kumph M, Partel S, Edlinger J, Brownnutt M, Blatt R 2014 New J. Phys. 16 113068
Google Scholar
[38] 吴宇恺, 段路明 2023 72 230302
Google Scholar
Wu Y K, Duan L M 2023 Acta Phys. Sin. 72 230302
Google Scholar
下载:
计量
- 文章访问数: 502
- PDF下载量: 14
- 被引次数: 0
