-
高保真度的多离子纠缠和量子逻辑门是离子阱量子计算的基础. 在现有的方案中, Mølmer-Sørensen 门是比较成熟的实现多离子纠缠和量子逻辑门的实验方案. 近年来, 还出现了通过设计超快激光脉冲序列, 在Lamb-Dicke区域以外实现超快量子纠缠和量子逻辑门的方案. 这些方案均借助离子链这一多体量子系统的声子能级来耦合离子之间的自旋状态, 并且均通过调制激光脉冲或设计合适的脉冲序列解耦多运动模式, 来提高纠缠门的保真度. 本文从理论和实验层面分析了这些多体量子纠缠和量子逻辑门操作的关键技术, 揭示了离子阱中利用激光场驱动离子链运动态, 通过非平衡过程中的非线性相互作用, 来实现量子逻辑门的基本物理过程.The high-fidelity multi-ion entangled states and quantum gates are the basis for trapped-ion quantum computing. Among the developed quantum gate schemes, Mølmer-Sørensen gate is a relatively mature experimental technique to realize multi-ion entanglement and quantum logic gates. In recent years, there have also been schemes to realize ultrafast quantum entanglement and quantum logic gates that operate outside the Lamb-Dicke regime by designing ultrafast laser pulse sequences. In such a many-body quantum system, these entanglement gates couple the spin states between ions by driving either the phonon energy level or the motional state of the ion chain. To improve the fidelity of quantum gates, the modulated laser pulses or the appropriately designed pulse sequences are applied to decouple the multi-mode motional states. In this review, we summarize and analyze the essential aspects of realizing these entanglement gates from both theoretical and experimental points of view. We also reveal that the basic physical process of realizing quantum gates is to utilize nonlinear interactions in non-equilibrium processes through driving the motional states of an ion chain with laser fields.
-
Keywords:
- trapped-ion quantum computing /
- quantum gate /
- quantum entanglement /
- spin-dependent interaction
[1] Schmidt-Kaler F, Häffner H, Riebe M, Gulde S, Lancaster G P T, Deuschle T, Becher C, Roos C F, Eschner J, Blatt R 2003 Nature 422 408
Google Scholar
[2] Sackett C A, Kielpinski D, King B E, Langer C, Meyer V, Myatt C J, Rowe M, Turchette Q A, Itano W M, Wineland D J, Monroe C 2000 Nature 404 256
Google Scholar
[3] Marquet C, Schmidt-Kaler F, James D F V 2003 Appl. Phys. B 76 199
Google Scholar
[4] James D F V 1998 Appl. Phys. B: Lasers Opt. 66 181
Google Scholar
[5] Zhu S L, Monroe C, Duan L M 2006 Phys. Rev. Lett. 97 050505
Google Scholar
[6] Leibfried D, Blatt R, Monroe C, Wineland D 2003 Rev. Mod. Phys. 75 281
Google Scholar
[7] Wineland D J, Monroe C, Itano W M, Leibfried D, King B E, Meekhof D M 1998 J. Res. Natl. Inst. Stand. Technol. 103 259
Google Scholar
[8] Meekhof D M, Leibfried D, Monroe C, King B E, Itano W M, Wineland D J 1997 Brazilian J. Phys. 27 15
[9] Diedrich F, Bergquist J C, Itano W M, Wineland D J 1989 Phys. Rev. Lett. 62 403
Google Scholar
[10] Monroe C, Meekhof D M, King B E, Jefferts S R, Itano W M, Wineland D J, Gould P 1995 Phys. Rev. Lett. 75 4011
Google Scholar
[11] King B E, Wood C S, Myatt C J, Turchette Q A, Leibfried D, Itano W M, Monroe C, Wineland D J 1998 Phys. Rev. Lett. 81 1525
Google Scholar
[12] Mølmer K, Sørensen A 1999 Phys. Rev. Lett. 82 1835
Google Scholar
[13] Sørensen A, Mølmer K 1999 Phys. Rev. Lett. 82 1971
Google Scholar
[14] Kim K, Chang M S, Islam R, Korenblit S, Duan L M, Monroe C 2009 Phys. Rev. Lett. 103 120502
Google Scholar
[15] Leung P H, Landsman K A, Figgatt C, Linke N M, Monroe C, Brown K R 2018 Phys. Rev. Lett. 120 020501
Google Scholar
[16] Landsman K A, Wu Y K, Leung P H, Zhu D W, Linke N M, Brown K R, Duan L M, Monroe C 2019 Phys. Rev. A 100 022332
Google Scholar
[17] Benhelm J, Kirchmair G, Roos C F, Blatt R 2008 Nat. Phys. 4 463
Google Scholar
[18] Roos C F 2008 New J. Phys. 10 013002
Google Scholar
[19] Klarsfeld S, Oteo J A 1989 Phys. Rev. A 39 3270
Google Scholar
[20] Cundiff S T, Ye J 2003 Rev. Mod. Phys. 75 325
Google Scholar
[21] Udem T, Holzwarth R, Hänsch T W 2002 Nature 416 233
Google Scholar
[22] Pe'er A, Shapiro E A, Stowe M C, Shapiro M, Ye J 2007 Phys. Rev. Lett. 98 113004
Google Scholar
[23] Hayes D, Matsukevich D N, Maunz P, Hucul D, Quraishi Q, Olmschenk S, Campbell W, Mizrahi J, Senko C, Monroe C 2010 Phys. Rev. Lett. 104 140501
Google Scholar
[24] Wong-Campos J D, Moses S A, Johnson K G, Monroe C 2017 Phys. Rev. Lett. 119 230501
Google Scholar
[25] Mizrahi J, Senko C, Neyenhuis B, Johnson K G, Campbell W C, Conover C W S, Monroe C 2013 Phys. Rev. Lett. 110 203001
Google Scholar
[26] Mizrahi J, Neyenhuis B, Johnson K G, Campbell W C, Senko C, Hayes D, Monroe C 2014 Appl. Phys. B 114 45
[27] Nielsen M A, Chuang I L 2010 Quantum Computation and Quantum Information, 10th Anniversary Edition (New York: Cambridge University Press) pp189–193
[28] Soderberg K A B and Monroe C 2010 Rep. Prog. Phys. 73 036401
Google Scholar
[29] Figgatt C, Ostrander A, Linke N M, Landsman K A, Zhu D W, Maslov D, Monroe C 2019 Nature 572 368
Google Scholar
[30] Lu Y, Zhang S N, Zhang K, Chen W T, Shen Y C, Zhang J L, Zhang J N, Kim K 2019 Nature 572 363
Google Scholar
[31] Choi T, Debnath S, Manning T A, Figgatt C, Gong Z X, Duan L M, Monroe C 2014 Phys. Rev. Lett. 112 190502
Google Scholar
[32] Zhu S L, Monroe C, Duan L M 2006 Europhys. Lett. 73 485
Google Scholar
[33] Green T J, Biercuk M J 2015 Phys. Rev. Lett. 114 120502
Google Scholar
[34] Schäfer V M, Ballance C J, Thirumalai K, Stephenson L J, Ballance T G, Steane A M, Lucas D M 2018 Nature 555 75
Google Scholar
[35] Hussain M I, Heinrich D, Guevara-Bertsch M, Torrontegui E, García-Ripoll J J, Roos C F, Blatt R 2021 Phys. Rev. Appl. 15 024054
Google Scholar
[36] Ratcliffe A K, Oberg L M, Hope J J 2020 Phys. Rev. A 101 052332
Google Scholar
[37] Mehdi Z, Ratcliffe A K, Hope J J 2021 Phys. Rev. Res. 3 013026
Google Scholar
[38] Campbell W C, Mizrahi J, Quraishi Q, Senko C, Hayes D, Hucul D, Matsukevich D N, Maunz P, Monroe C 2010 Phys. Rev. Lett. 105 090502
Google Scholar
[39] Debnath S, Linke N M, Figgatt C, Landsman K A, Wright K, Monroe C 2016 Nature 536 63
Google Scholar
[40] Monroe C, Campbell W C, Duan L M, Gong Z X, Gorshkov A V, Hess P W, Islam R, Kim K, Linke N M, Pagano G, Richerme P, Senko C, Yao N Y 2021 Rev. Mod. Phys. 93 025001
Google Scholar
[41] Moehring D L, Blinov B B, Gidley D W, Kohn R N, J r., Madsen M J, Sanderson T D, Vallery R S, Monroe C 2006 Phys. Rev. A 73 023413
Google Scholar
[42] Blinov B B, Kohn R N, J r., Madsen M J, Maunz P, Moehring D L, Monroe C 2006 J. Opt. Soc. Am. B 23 1170
Google Scholar
[43] Madsen M J, Moehring D L, Maunz P, Kohn R N, Jr., Duan L M, Monroe C 2006 Phys. Rev. Lett. 97 040505
Google Scholar
[44] Johnson K G, Wong-Campos J D, Neyenhuis B, Mizrahi J, Monroe C 2017 Nat. Commun. 8 1
Google Scholar
[45] Gardiner S A, Cirac J I, Zoller P 1997 Phys. Rev. Lett. 79 4790
Google Scholar
-
图 1 两离子在阱中的振动模式 (a) 质心模; (b) 呼吸模
Fig. 1. Vibration modes of two ions in trap: (a) Center of mass mode; (b) relative motion mode.
图 2 5个离子径向模式和轴向模式的本征频率 (a) 径向模式的本征频率以及本征模向量,
$b_m(m=1—5)$ 表示了5个离子远离平衡位置$x_0$ 的大小,$\delta$ 是执行受激拉曼跃迁过程中设置的失谐; (b) 模拟计算的径向模式和轴向模式的本征频率谱Fig. 2. Eigenfrequencies of the transverse and axial modes of the 5 ions in trap: (a) Eigenfrequencies of transverse modes and eigenmode vectors,
$b_m(m=1-5)$ represents the size of the 5 ions away from the equilibrium position$x_0$ ,$\delta$ is the detuning in stimulated Raman transition; (b) simulated eigenfrequency spectra of transverse and axial modes.
图 3
$^ {171}{\rm{Yb}}^{+}$ 中受激拉曼跃迁示意图 ($\omega_1$ 、$\omega_2$ 分别为两束激光的频率;$\varDelta$ 为两束激光在$^ {2}{\rm{P}}_{1/2}$ 能级处的失谐)Fig. 3. The stimulated Raman transition of
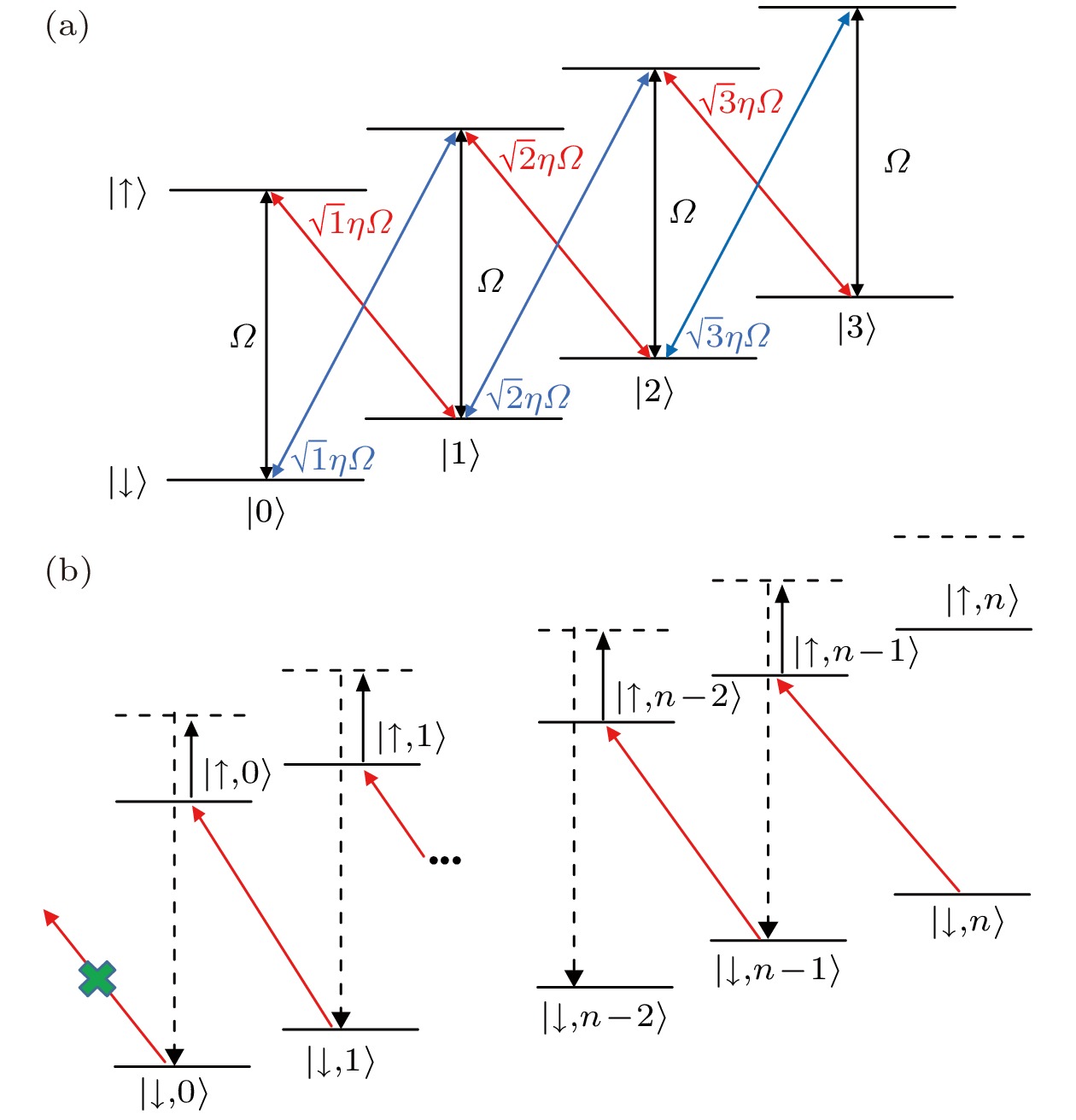
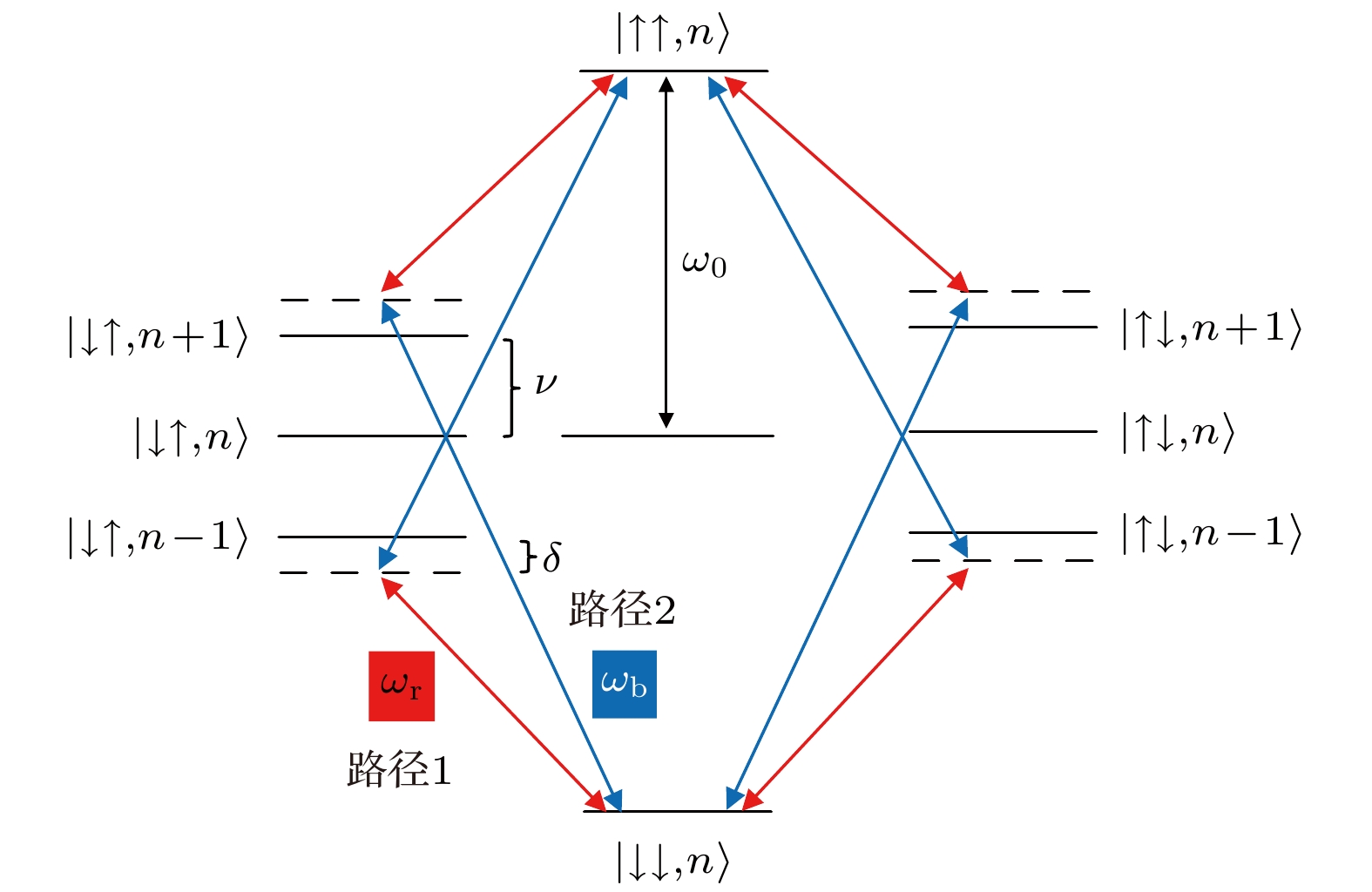
$^ {171}{\rm{Yb}}^{+}$ ($\omega_1$ and$\omega_2$ are the frequencies of the two laser beams;$\varDelta$ is the detuning of the two laser beams at the$^ {2}{\rm{P}}_{1/2}$ energy level).
图 4 边带跃迁和边带冷却示意图 (a) 载波(黑)、红边带(红)和蓝边带(蓝)跃迁; (b)
$^{171}{\rm{Yb}}^+$ 红边带冷却示意图Fig. 4. Schematic diagram of sideband transition and sideband cooling: (a) Carrier (black arrow), red sideband (red arrow) and blue sideband (blue arrow) transitions; (b) red sideband cooling of
$^{171}{\rm{Yb}}^+$ .
图 5 Mølmer-Sørensen门能级跃迁示意图, 其中
$\omega_{\rm{b}}$ ,$\omega_{\rm{r}}$ 分别为蓝边带和红边带的频率, 红色箭头和蓝色箭头分别为红边带跃迁和蓝边带跃迁.$\nu$ 为声子频率,$\delta$ 为红、蓝边带处的小失谐Fig. 5. The energy level transition in Mølmer-Sørensen gate.
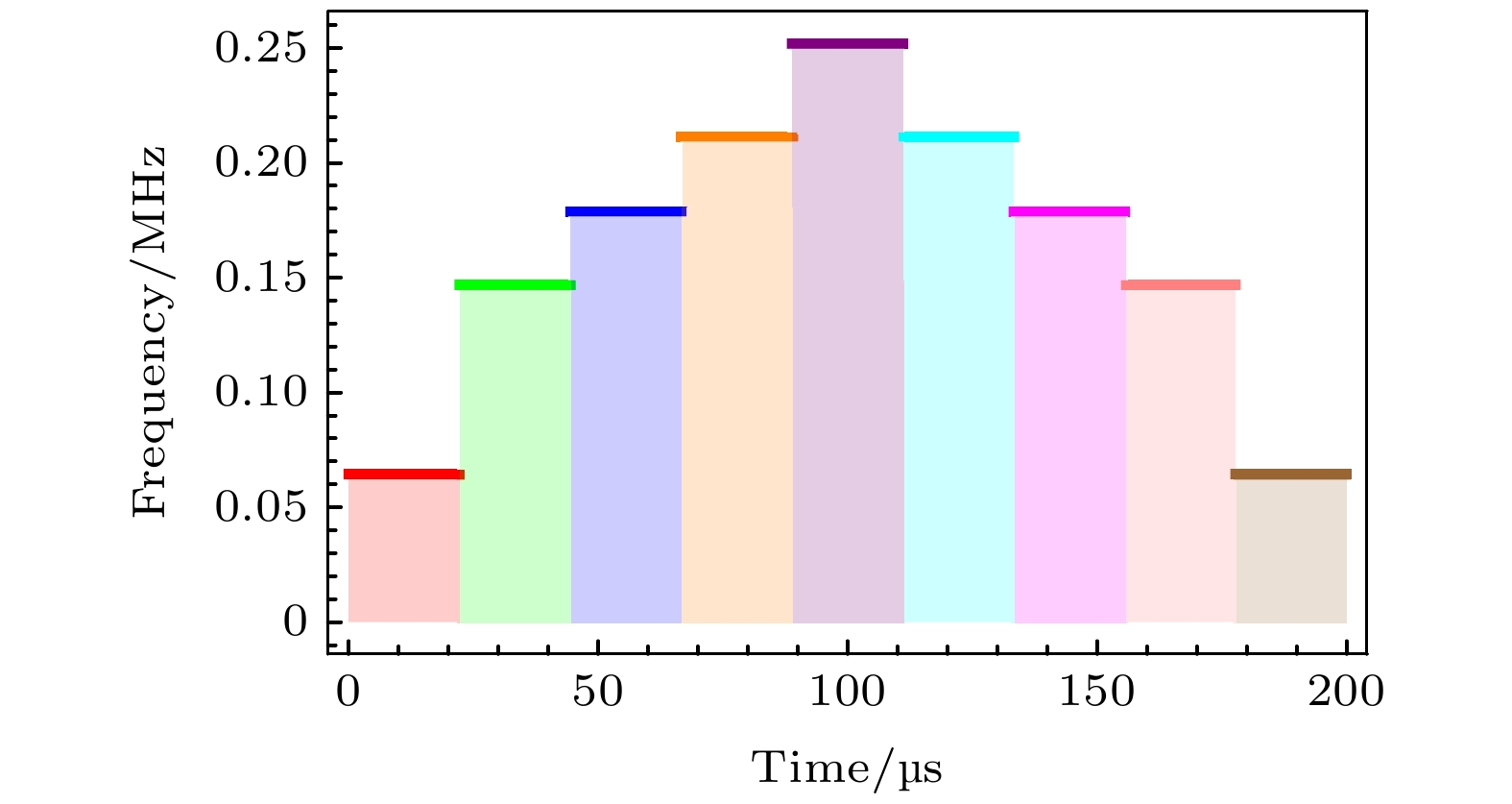
$\omega_{\rm{b}}$ and$\omega_{\rm{r}}$ are frequencies of blue sideband and red sideband respectively, and blue arrow and red arrow are blue sideband transition and red sideband transition respectively;$\nu$ is the phonon frequency, and$\delta$ is the small detuning of each sideband.
图 6 自旋依赖的动量转移 (a) 不同自旋状态在相空间被转移的方向不同, 红色圆代表
$ \left| \uparrow \right\rangle $ 态, 蓝色圆代表$ \left| \downarrow \right\rangle $ 态; (b) 超快脉冲序列照射离子产生自旋依赖的动量转移Fig. 6. Spin-dependent momentum transfer: (a) Different spin states are transferred in different directions in phase space, red circle represents
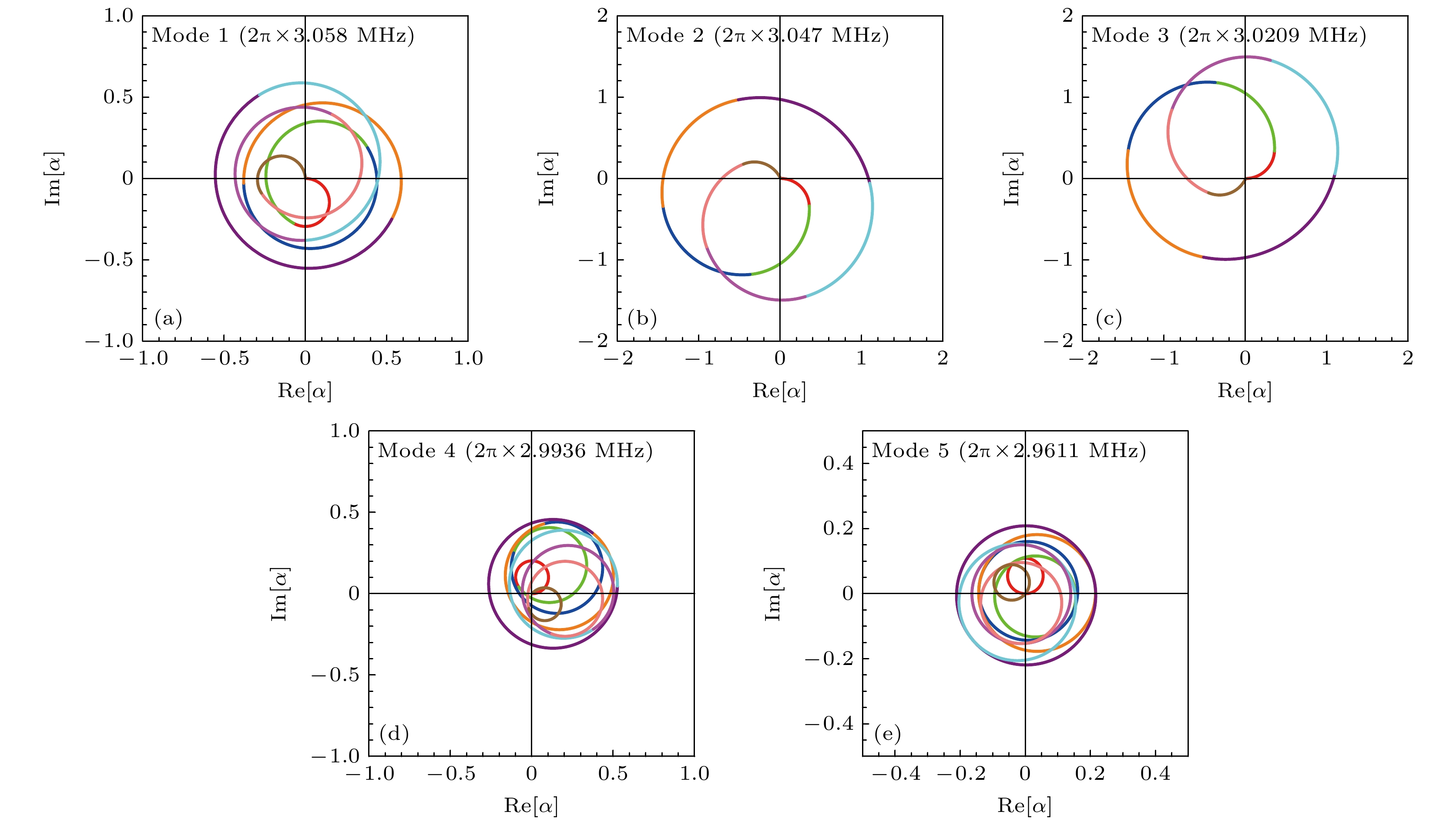
$ \left| \uparrow \right\rangle $ state and blue circle represents$ \left| \downarrow \right\rangle $ state; (b) ultrafast pulse trains irradiate ions and produce spin-dependent momentum transfer.
图 7 脉冲激光线宽覆盖若干个本征频率的示意图
Fig. 7. Schematic of laser linewidth covering several eigenfrequencies.
图 8 门时间为
$200 \ \mu{\rm{s}}$ , 失谐$\delta$ 为3.0318 MHz时脉冲幅度调制模拟结果Fig. 8. Simulation result of laser amplitude modulation when gate time is
$200 \; \mu{\rm{s}}$ and detuning$\delta$ is 3.0318 MHz.
图 9 5个振动模式的运动态在相空间内的运动轨迹 (a)线频率为3.058 MHz的模式1; (b) 线频率为3.047 MHz的模式2; (c) 线频率为3.0209 MHz的模式3; (d) 线频率为2.9936 MHz的模式4; (e) 线频率为2.9611 MHz的模式5
Fig. 9. The motional state trajectories of the five vibration modes in phase space: (a) Mode 1, line frequency is 3.058 MHz; (b) Mode 2, line frequency is 3.047 MHz; (c) Mode 3, line frequency is 3.0209 MHz; (d) Mode 4, line frequency is 2.9936 MHz; (e) Mode 5, line frequency is 2.9611 MHz.
图 10 两个运动模式在脉冲序列的作用下与自旋解耦 (a) 超快脉冲序列; (b) 两个运动模式在相空间的运动轨迹
Fig. 10. Two motional modes are decoupled from spin: (a) Ultrafast pulse train; (b) trajectories of two motion modes in phase space.
表 1 离子链上的离子位置
Table 1. Ion position on the ion chain.
离子序数 1 2 3 4 5 位置/$\mu {\rm{m}}$ –10.52 –4.96 0 4.96 10.52 
下载: 导出CSV
-
[1] Schmidt-Kaler F, Häffner H, Riebe M, Gulde S, Lancaster G P T, Deuschle T, Becher C, Roos C F, Eschner J, Blatt R 2003 Nature 422 408
Google Scholar
[2] Sackett C A, Kielpinski D, King B E, Langer C, Meyer V, Myatt C J, Rowe M, Turchette Q A, Itano W M, Wineland D J, Monroe C 2000 Nature 404 256
Google Scholar
[3] Marquet C, Schmidt-Kaler F, James D F V 2003 Appl. Phys. B 76 199
Google Scholar
[4] James D F V 1998 Appl. Phys. B: Lasers Opt. 66 181
Google Scholar
[5] Zhu S L, Monroe C, Duan L M 2006 Phys. Rev. Lett. 97 050505
Google Scholar
[6] Leibfried D, Blatt R, Monroe C, Wineland D 2003 Rev. Mod. Phys. 75 281
Google Scholar
[7] Wineland D J, Monroe C, Itano W M, Leibfried D, King B E, Meekhof D M 1998 J. Res. Natl. Inst. Stand. Technol. 103 259
Google Scholar
[8] Meekhof D M, Leibfried D, Monroe C, King B E, Itano W M, Wineland D J 1997 Brazilian J. Phys. 27 15
[9] Diedrich F, Bergquist J C, Itano W M, Wineland D J 1989 Phys. Rev. Lett. 62 403
Google Scholar
[10] Monroe C, Meekhof D M, King B E, Jefferts S R, Itano W M, Wineland D J, Gould P 1995 Phys. Rev. Lett. 75 4011
Google Scholar
[11] King B E, Wood C S, Myatt C J, Turchette Q A, Leibfried D, Itano W M, Monroe C, Wineland D J 1998 Phys. Rev. Lett. 81 1525
Google Scholar
[12] Mølmer K, Sørensen A 1999 Phys. Rev. Lett. 82 1835
Google Scholar
[13] Sørensen A, Mølmer K 1999 Phys. Rev. Lett. 82 1971
Google Scholar
[14] Kim K, Chang M S, Islam R, Korenblit S, Duan L M, Monroe C 2009 Phys. Rev. Lett. 103 120502
Google Scholar
[15] Leung P H, Landsman K A, Figgatt C, Linke N M, Monroe C, Brown K R 2018 Phys. Rev. Lett. 120 020501
Google Scholar
[16] Landsman K A, Wu Y K, Leung P H, Zhu D W, Linke N M, Brown K R, Duan L M, Monroe C 2019 Phys. Rev. A 100 022332
Google Scholar
[17] Benhelm J, Kirchmair G, Roos C F, Blatt R 2008 Nat. Phys. 4 463
Google Scholar
[18] Roos C F 2008 New J. Phys. 10 013002
Google Scholar
[19] Klarsfeld S, Oteo J A 1989 Phys. Rev. A 39 3270
Google Scholar
[20] Cundiff S T, Ye J 2003 Rev. Mod. Phys. 75 325
Google Scholar
[21] Udem T, Holzwarth R, Hänsch T W 2002 Nature 416 233
Google Scholar
[22] Pe'er A, Shapiro E A, Stowe M C, Shapiro M, Ye J 2007 Phys. Rev. Lett. 98 113004
Google Scholar
[23] Hayes D, Matsukevich D N, Maunz P, Hucul D, Quraishi Q, Olmschenk S, Campbell W, Mizrahi J, Senko C, Monroe C 2010 Phys. Rev. Lett. 104 140501
Google Scholar
[24] Wong-Campos J D, Moses S A, Johnson K G, Monroe C 2017 Phys. Rev. Lett. 119 230501
Google Scholar
[25] Mizrahi J, Senko C, Neyenhuis B, Johnson K G, Campbell W C, Conover C W S, Monroe C 2013 Phys. Rev. Lett. 110 203001
Google Scholar
[26] Mizrahi J, Neyenhuis B, Johnson K G, Campbell W C, Senko C, Hayes D, Monroe C 2014 Appl. Phys. B 114 45
[27] Nielsen M A, Chuang I L 2010 Quantum Computation and Quantum Information, 10th Anniversary Edition (New York: Cambridge University Press) pp189–193
[28] Soderberg K A B and Monroe C 2010 Rep. Prog. Phys. 73 036401
Google Scholar
[29] Figgatt C, Ostrander A, Linke N M, Landsman K A, Zhu D W, Maslov D, Monroe C 2019 Nature 572 368
Google Scholar
[30] Lu Y, Zhang S N, Zhang K, Chen W T, Shen Y C, Zhang J L, Zhang J N, Kim K 2019 Nature 572 363
Google Scholar
[31] Choi T, Debnath S, Manning T A, Figgatt C, Gong Z X, Duan L M, Monroe C 2014 Phys. Rev. Lett. 112 190502
Google Scholar
[32] Zhu S L, Monroe C, Duan L M 2006 Europhys. Lett. 73 485
Google Scholar
[33] Green T J, Biercuk M J 2015 Phys. Rev. Lett. 114 120502
Google Scholar
[34] Schäfer V M, Ballance C J, Thirumalai K, Stephenson L J, Ballance T G, Steane A M, Lucas D M 2018 Nature 555 75
Google Scholar
[35] Hussain M I, Heinrich D, Guevara-Bertsch M, Torrontegui E, García-Ripoll J J, Roos C F, Blatt R 2021 Phys. Rev. Appl. 15 024054
Google Scholar
[36] Ratcliffe A K, Oberg L M, Hope J J 2020 Phys. Rev. A 101 052332
Google Scholar
[37] Mehdi Z, Ratcliffe A K, Hope J J 2021 Phys. Rev. Res. 3 013026
Google Scholar
[38] Campbell W C, Mizrahi J, Quraishi Q, Senko C, Hayes D, Hucul D, Matsukevich D N, Maunz P, Monroe C 2010 Phys. Rev. Lett. 105 090502
Google Scholar
[39] Debnath S, Linke N M, Figgatt C, Landsman K A, Wright K, Monroe C 2016 Nature 536 63
Google Scholar
[40] Monroe C, Campbell W C, Duan L M, Gong Z X, Gorshkov A V, Hess P W, Islam R, Kim K, Linke N M, Pagano G, Richerme P, Senko C, Yao N Y 2021 Rev. Mod. Phys. 93 025001
Google Scholar
[41] Moehring D L, Blinov B B, Gidley D W, Kohn R N, J r., Madsen M J, Sanderson T D, Vallery R S, Monroe C 2006 Phys. Rev. A 73 023413
Google Scholar
[42] Blinov B B, Kohn R N, J r., Madsen M J, Maunz P, Moehring D L, Monroe C 2006 J. Opt. Soc. Am. B 23 1170
Google Scholar
[43] Madsen M J, Moehring D L, Maunz P, Kohn R N, Jr., Duan L M, Monroe C 2006 Phys. Rev. Lett. 97 040505
Google Scholar
[44] Johnson K G, Wong-Campos J D, Neyenhuis B, Mizrahi J, Monroe C 2017 Nat. Commun. 8 1
Google Scholar
[45] Gardiner S A, Cirac J I, Zoller P 1997 Phys. Rev. Lett. 79 4790
Google Scholar
下载:
计量
- 文章访问数: 8146
- PDF下载量: 351
- 被引次数: 0
