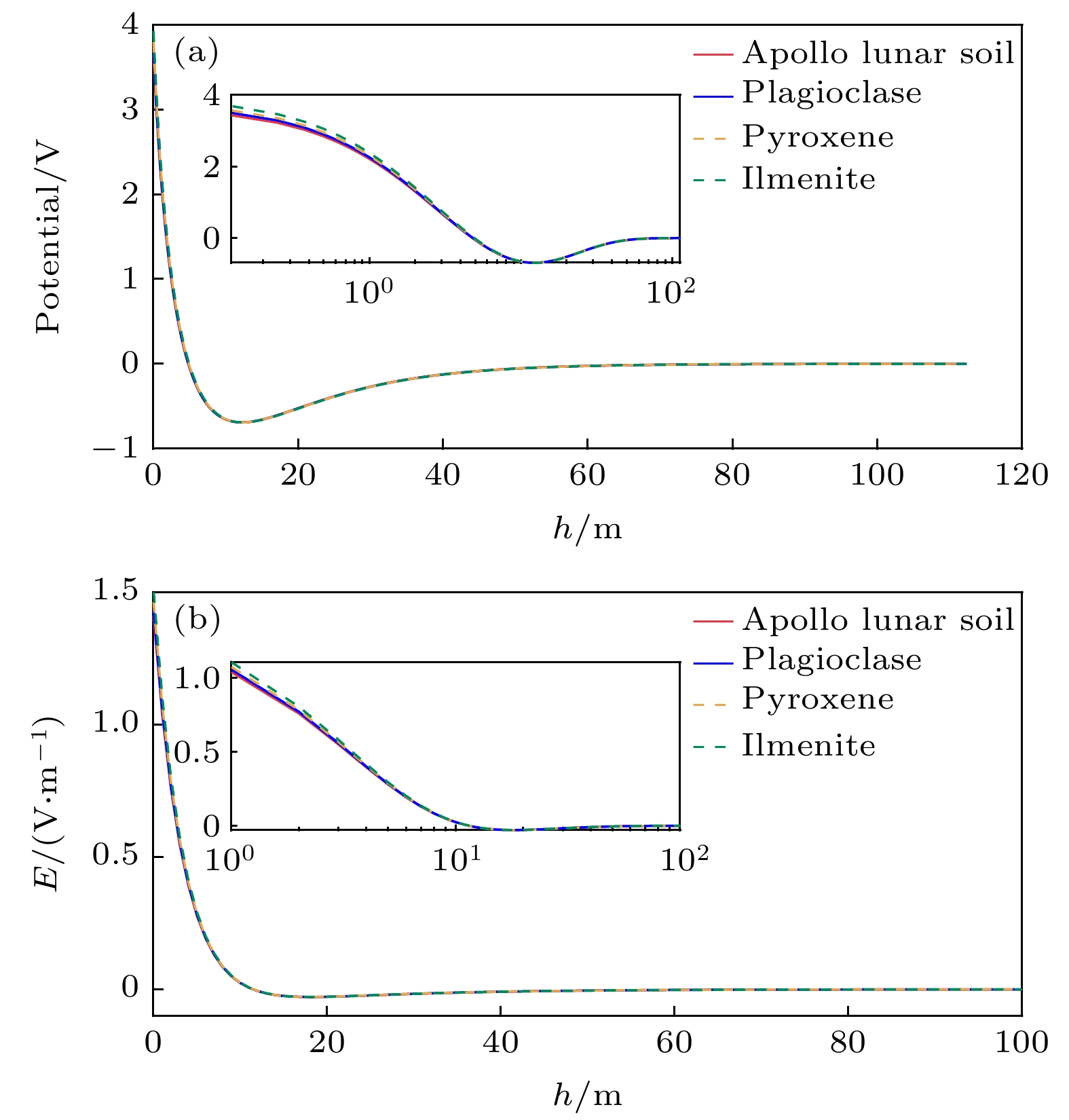
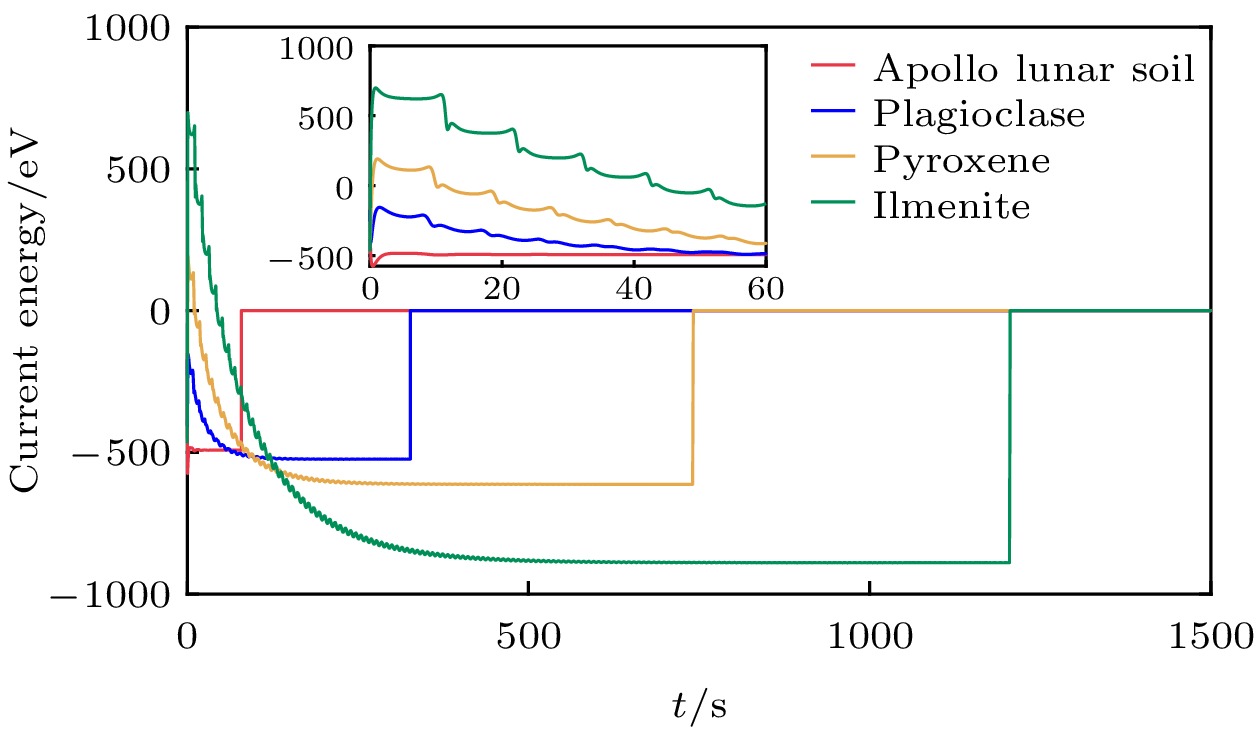
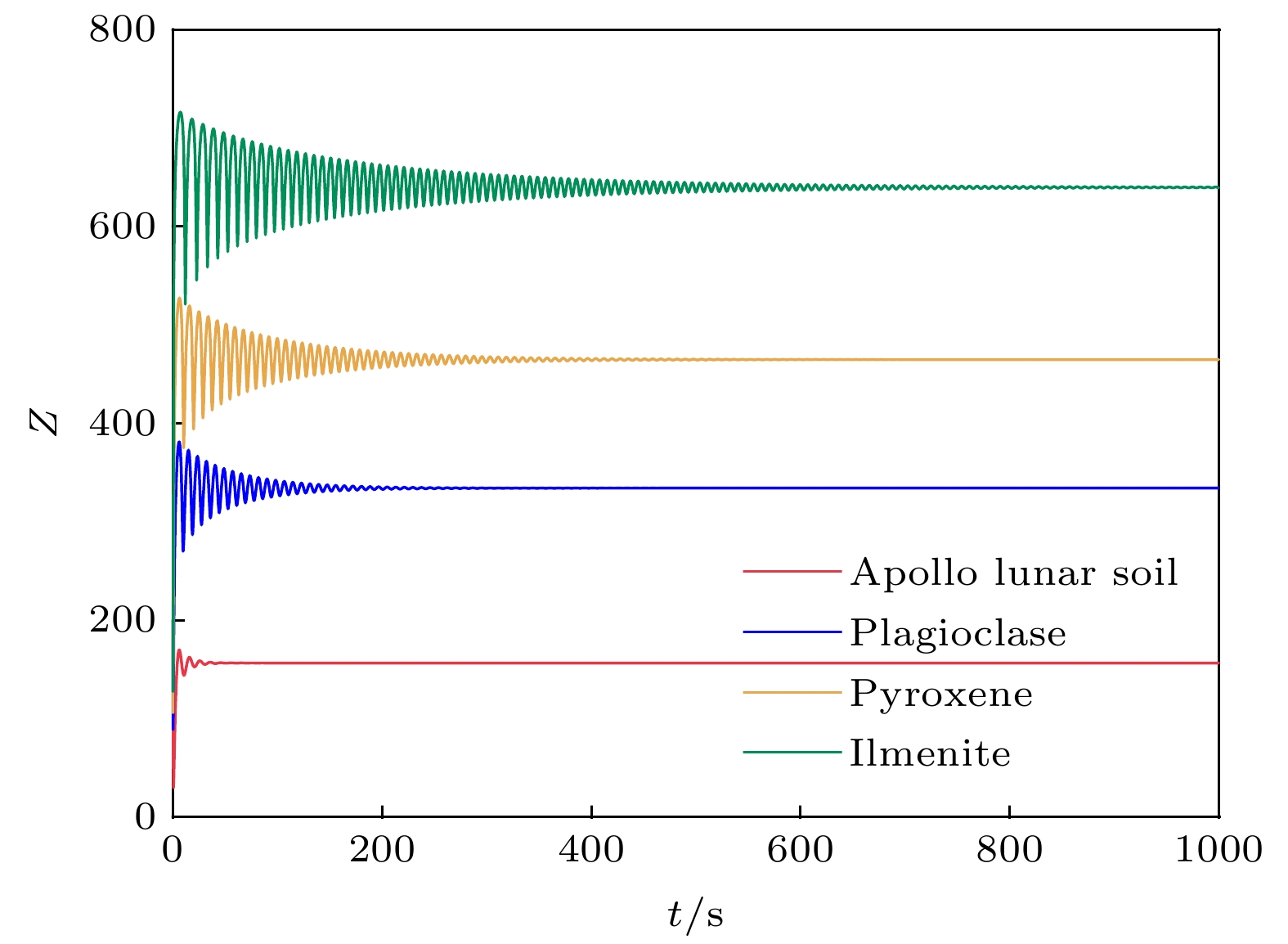
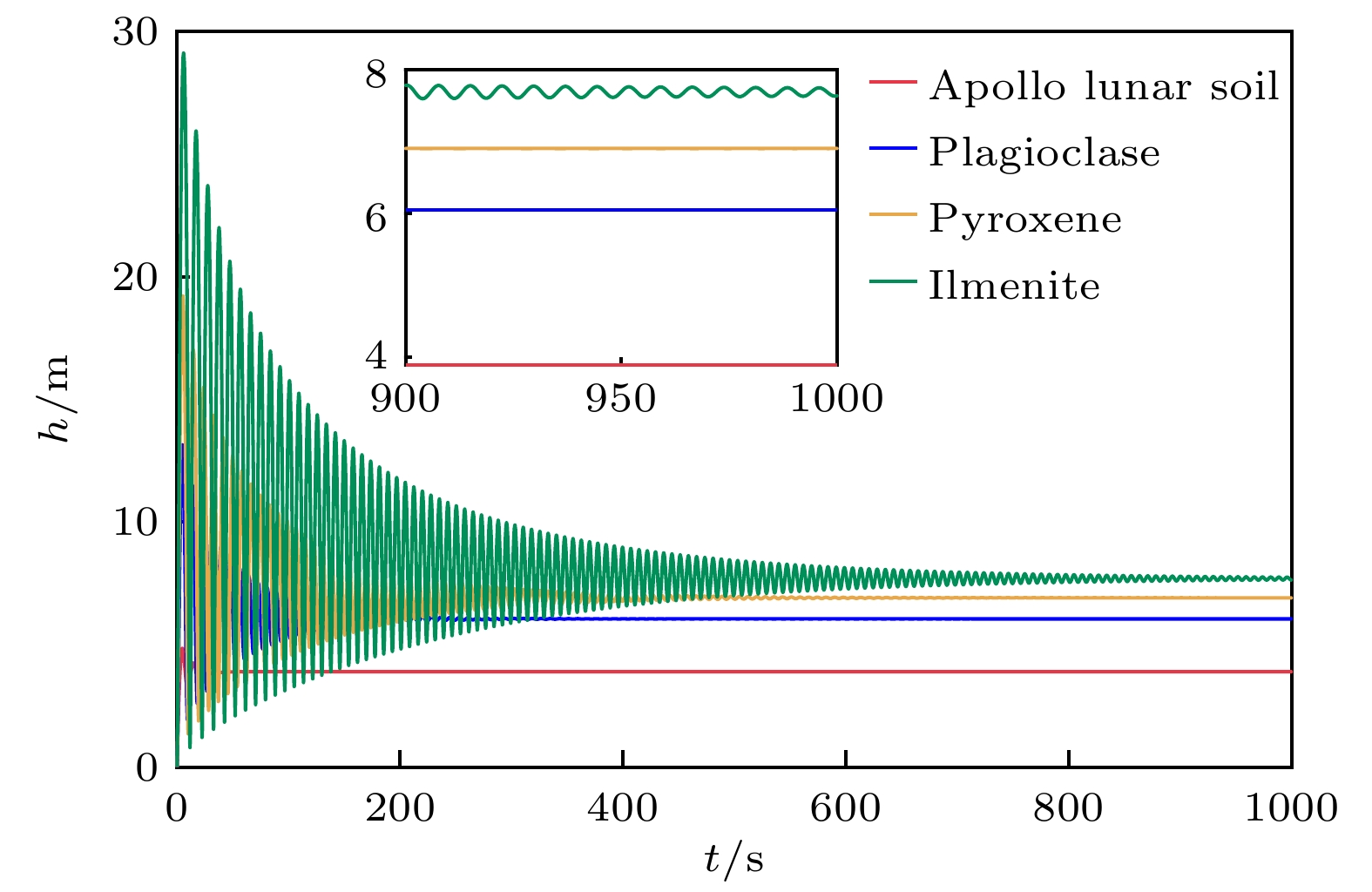
-
月球表面的带电尘埃对太空任务的顺利实施构成严重威胁, 对尘埃的充电和动力学的进一步研究有助于月球探测任务的顺利实施. 本文研究了具有不同功函数的尘埃颗粒在月球表面的充电和动力学. 本文重新计算了与四种尘埃颗粒功函数相关的表面充电电流, 并得到了它们在不同太阳天顶角下的充电和动力学结果, 揭示了尘埃颗粒充电和动力学结果对功函数的依赖性. 结果显示具有较小功函数的尘埃颗粒能够达到较大的平衡态, 且需要更长时间才能达到这些平衡态, 其中包括尘埃颗粒能够稳定悬浮的平衡高度, 能够携带的表面电荷量以及流经尘埃颗粒表面的充电电流. 结果表明, 当太阳天顶角在0°到90°范围内变化时, 平衡态与功函数之间都呈现明显的反比关系. 尘埃颗粒在临界太阳天顶角下不能发生稳定悬浮, 且该角度的大小与功函数也呈反比关系.Charged dust on the lunar surface poses a threat to space missions. Research into charged dust is essential for the safety of future space missions. The conventional lunar dust charging theory assumes a single constant work function when calculating the charging currents related to photoelectrons. However, the components of lunar regolith exhibit considerable diversity, including plagioclase, pyroxene, and ilmenite. Because the ability of the lunar surface or lunar dust to emit photoelectrons strongly depends on their work function, it is necessary to analyze the effect of work function on dust charging and dynamics near the lunar surface. In this work, we used a novel method that can predict the photoelectric yield of materials with different work functions to recalculate the surface charging currents of four types of dust particles and derived their subsequent charging and dynamic results at different solar zenith angles (SZAs). When SZA varies from 0°to 90°, the work function of dust decreases incrementally through four values: 6 eV (Apollo lunar soil), 5.58 eV (Plagioclase), 5.14 eV (Pyroxene), and 4.29 eV (Ilmenite). With each decrement in work function, the equilibrium charging currents of dust particles increase by approximately 0.5 times, the equilibrium charge numbers increase by approximately 120-170 elemental charges, and the equilibrium heights increase by approximately 0.3-2 m. We found that dust particles could not levitate stably at a critical SZA, and the critical SZAs for the four types of dust particles are 28°, 76°, 85.8°, and 89.6°, respectively (arranged in order of decreasing work function). These results indicated that the equilibrium heights, equilibrium currents, and critical SZAs all have an inverse relationship with the work functions of dust particles as the SZA varies from 0°to 90°. In addition, a higher photoelectron density in areas with lower work functions results in smaller energy losses, causing dust particles to take longer to reach equilibrium, which means the equilibrium time follows the same pattern as that of the work function.
-
Keywords:
- Moon /
- Dust levitation /
- Work function
-
图 1 阿波罗任务带回的样品的光电子产率
Fig. 1. Photoelectric yield measured on samples returned by Apollo missions.
图 2 最小太阳活动周期下的太阳辐射光谱
Fig. 2. Wavelength dependence of solar radiation spectrum under minimum solar activity conditions.
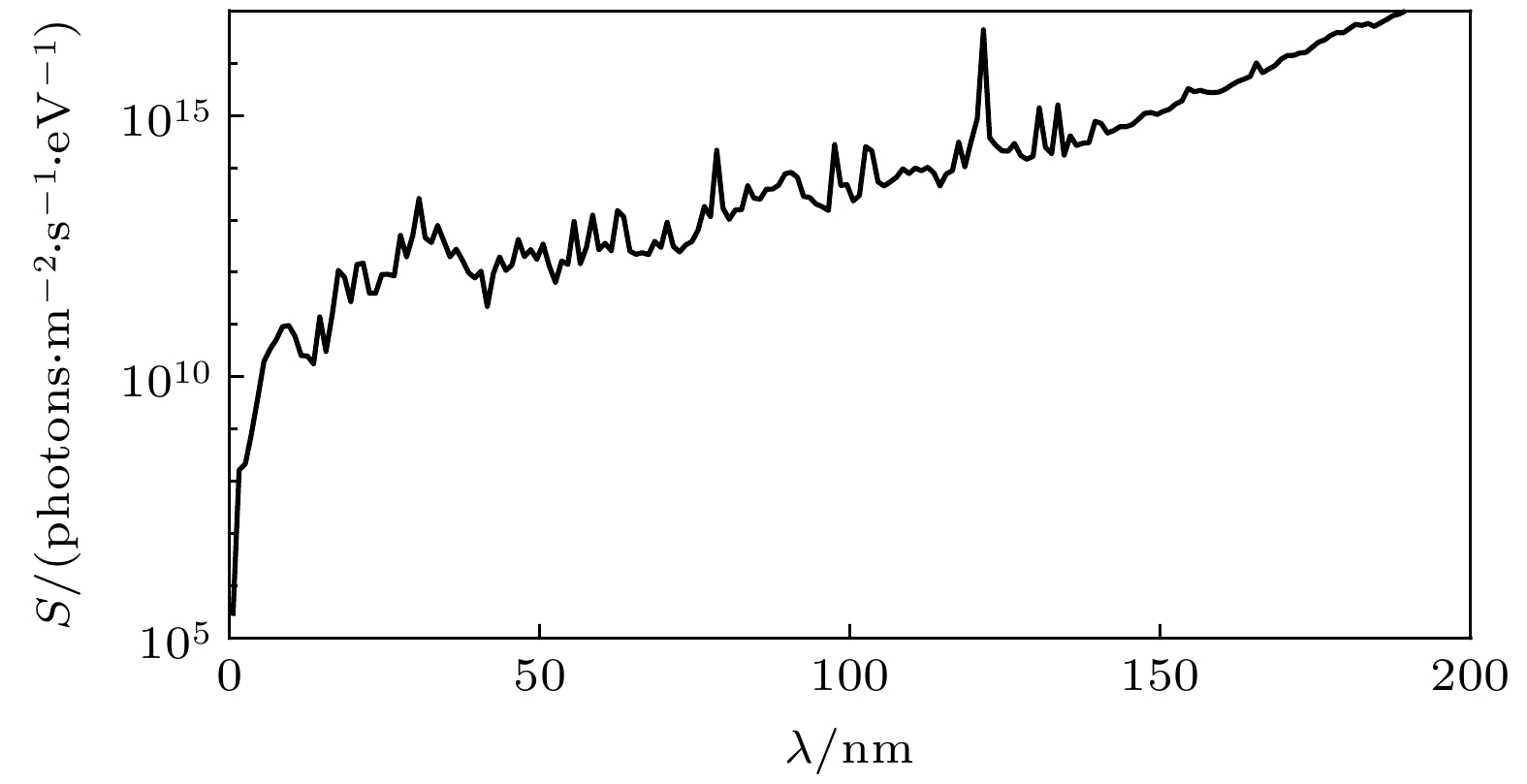
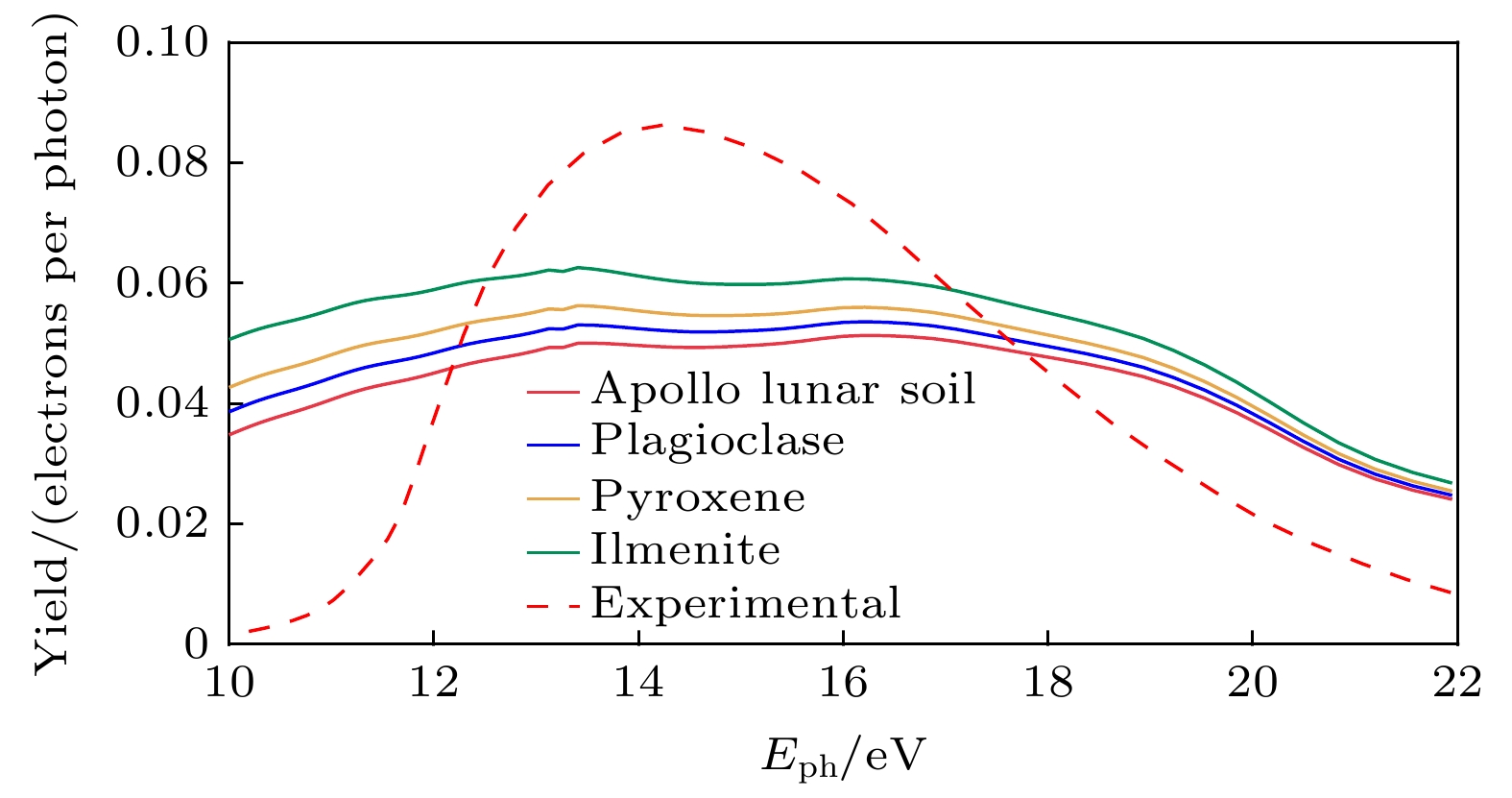
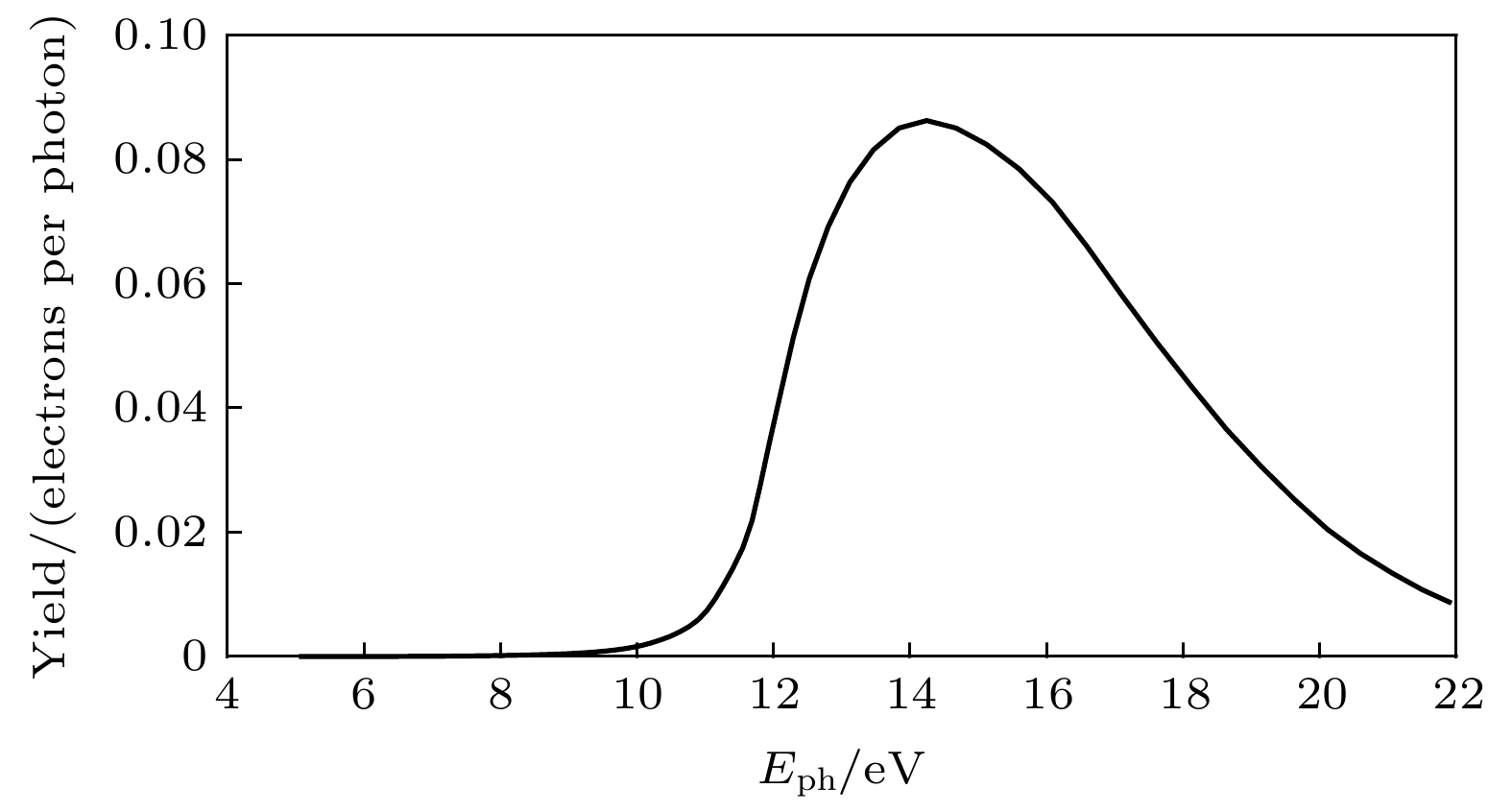
图 3 四种不同功函数尘埃的光电子产率. 实线表示使用Kimura方法计算的产率, 红色实线代表阿波罗月球土壤, 蓝色实线代表斜长石, 黄色实线代表辉石, 绿色实线代表钛铁矿. 红色虚线表示阿波罗月球土壤的实验产率
Fig. 3. Photoelectric yield for four different types of dust particles. Solid lines represent yield calculated by using Kimura's method. Red line represents Apollo lunar soil, blue line represents plagioclase, yellow line represents pyroxene, and green line represents ilmenite. Red dash line represents experimental yield of Apollo lunar soil.
图 4 四个区域内正午时分的电势、电场与高度的函数关系, 为方便比较, 绘制了半对数横坐标形式的内插图 (a) 电势与高度的函数关系; (b) 电场与高度的函数关系
Fig. 4. Height dependence of surface potential and electric field at noon. For clarity, the semilogx form has been plotted: (a) potential; (b) electric field.
图 5 正午时分悬浮尘埃颗粒的充电电流 (a) 光电子发射电流; (b) 光电子收集电流; (c) 太阳风电子电流
Fig. 5. Charging currents of suspended dust particles at noon: (a) photoemission current; (b) photoelectron collection current; (c) solar wind electron current.
图 6 正午时分四种尘埃颗粒的电流能, 0—60 s内的结果已被绘制为内插图
Fig. 6. Energy created by charging currents at noon. Current energy from 0–60 s has been magnified.
图 8 正午时分悬浮尘埃颗粒的高度, 900—1000 s的结果已被绘制为内插图
Fig. 8. Vertical height of suspended dust particles at noon, results at 900–1000 s have been magnified.
图 9 悬浮尘埃颗粒的平衡态 (a) 平衡高度; (b) 平衡电荷数
Fig. 9. Equilibria of suspended dust particles: (a) equilibrium height; (b) equilibrium charge numbers.
表 1 四个区域中的材料参数及正午时分的光电子浓度
Table 1. Material parameters and photoelectron density of four areas at noon.
尘埃类型 密度/(g$ \cdot $cm$ ^{-3} $) 功函数/(eV) 浓度/(m$ ^{-3} $) 阿波罗月壤 1.5 6.00 $ 6.6943\times 10^7 $ 斜长石 2.7 5.58 $ 6.9190\times 10^7 $ 辉石 3.2 5.14 $ 7.1508\times 10^7 $ 钛铁矿 4.4 4.29 $ 7.5901\times 10^7 $ 
下载: 导出CSV
表 2 初始参数
Table 2. Initial Parameters.
参数 参数值 日心距$ \mathrm{d} $ 1 AU 重力加速度$ g_{\mathrm{a}} $ 1.63 $ \mathrm{m\cdot s^{-2}} $ 尘埃质量$ m_{\mathrm{d}} $ 6.28318$ \times 10^{-18} $ $ \mathrm{kg} $ 初始电荷$ Q_0 $ 3.20424$ \times 10^{-17} $ C 初始速度$ v_{\mathrm{d0}} $ 2 $ \mathrm{m\cdot s^{-1}} $
下载: 导出CSV
-
[1] Zakharov A V, Popel S I, Kuznetsov I A, Borisov N D, Rosenfeld E V, Skorov Y, Zelenyi L M 2022 Phys. Plasmas 29 110501
Google Scholar
[2] Xia Q, Cai M H, Xu L L, Han R L, Yang T, Han J W 2022 Chin. Phys. B 31 045201
Google Scholar
[3] Grard R, Tunaley J 1971 J. Geophys. Res. 76 2498
Google Scholar
[4] Nitter T, Havnes O 1992 Earth Moon and Planets 56 7
Google Scholar
[5] Nitter T, Havnes O, Melands F 1998 J. Geophys. Res.: Space Phys. 103 6605
Google Scholar
[6] Colwell J, Batiste S, Horányi M, Robertson S, Sture S 2007 Rev. Geophys. 45
[7] Lee P 1996 Icarus 124 181
Google Scholar
[8] Walbridge E 1973 J. Geophys. Res. 78 3668
Google Scholar
[9] Whipple E C 1981 Rep. Prog. Phys. 44 1197
Google Scholar
[10] Wang X, Horányi M, Robertson S 2009 J.Geophys.Res.:SpacePhys. 114 A05103
[11] Wang X, HoráNyi M, Robertson S 2010 J.Geophys.Res.:SpacePhys. 115 A11102
[12] Wang X, Horányi M, Robertson S 2011 Planet. Space Sci. 59 1791
Google Scholar
[13] Wang X, Schwan J, Hsu H W, Grün E, Horányi M 2016 Geophys. Res. Lett. 43 6103
Google Scholar
[14] Wang X, Pilewskie J, Hsu H W, Horányi M 2016 Geophys.Res.Lett. 43 525
Google Scholar
[15] Schwan J, Wang X, Hsu H W, Grün E, Horányi M 2017 Geophys. Res. Lett. 44 3059
Google Scholar
[16] Zimmerman M I, Farrell W M, Hartzell C M, Wang X, Horanyi M, Hurley D M, Hibbitts K 2016 J. Geophys. Res.: Planets 121 2150
Google Scholar
[17] Hartzell C, Zimmerman M, Hergenrother C 2022 Planet. Sci. J. 3 85
Google Scholar
[18] Golub’ A P, Dol’nikov G G, Zakharov A V, Zelenyi L M, Izvekova Y N, Kopnin S I, Popel S I 2012 Jetp. Lett. 95 182
Google Scholar
[19] Popel S I, Kopnin S I, Golub’ A P, Dol’nikov G G, Zakharov A V, Zelenyi L M, Izvekova Y N 2013 Sol. Syst. Res. 47 419
Google Scholar
[20] Popel S I, Golub’ A P, Zakharov A V, Zelenyi L M 2019 In J. Phys.: Conf. Ser., vol. 1147 of Journal of Physics Conference Series (IOP), p 012110
[21] Zelenyi L M, Popel S I, Zakharov A V 2020 Plasma Phys. Rep. 46 527
Google Scholar
[22] Hess S L G, Sarrailh P, Mateo-Velez J C, Jeanty-Ruard B, Cipriani F, Forest J, Hilgers A, Honary F, Thiebault B, Marple S R, Rodgers D 2015 IEEE Trans. Plasma Sci. 43 2799
Google Scholar
[23] Kuznetsov I A, Hess S L G, Zakharov A V, Cipriani F, Seran E, Popel S I, Lisin E A, Petrov O F, Dolnikov G G, Lyash A N, Kopnin S I 2018 Planet.SpaceSci. 156 62
Google Scholar
[24] Davari H, Farokhi B, Ali Asgarian M 2023 Sci. Rep. 13 1111
Google Scholar
[25] Piquette M, Horányi M 2017 Icarus 291 65
Google Scholar
[26] Li M Y, Xia Q, Cai M H, Yang T, Xu L L, Jia X Y, Han J W 2024 Acta Phys. Sin. 73 155201
Google Scholar
[27] Zhao C, Gan H, Xie L, Wang Y, Wang Y, Hong J 2023 Sci. China: Earth Sci. 66 2278
Google Scholar
[28] Gan H, Wei G F, Zhang W W, Li X Y, Jiang S Y, Wang C, Ma J N, Zhang X P 2023 Sci. China: Phys., Mech. Astron. 53 127
[29] Li L, Zhang Y T, Zhou B, Feng Y Y 2016 Sci. China: Earth Sci. 59 2053
Google Scholar
[30] Popel S I, Golub’ A P, Izvekova Y N, Afonin V V, Dol’nikov G G, Zakharov A V, Zelenyi L M, Lisin E A, Petrov O F 2014 Jetp. Lett. 99 115
Google Scholar
[31] Mishra S K 2020 Phys. Plasmas 27 082906
Google Scholar
[32] Feuerbacher B, Anderegg M, Fitton B, Laude L D, Willis R F, Grard R J L 1972 Lunar and Planetary Science Conference Proceedings 3 2655
[33] Sternovsky Z, Robertson S, Sickafoose A, Colwell J, Horányi M 2002 J. Geophys. Res.: Planets 107 5105
[34] Sternovsky Z, Chamberlin P, Horanyi M, Robertson S, Wang X 2008 J. Geophys. Res.: Space Phys. 113 A10104
[35] Kimura H 2016 Mon. Not. R. Astron. Soc. 459 2751
Google Scholar
[36] Seah M P, Dench W 1979 Surf. Interface Anal. 1 2
Google Scholar
[37] Senshu H, Kimura H, Yamamoto T, Wada K, Kobayashi M, Namiki N, Matsui T 2015 Planet. Space Sci. 116 18
Google Scholar
[38] Chamberlin P C, Woods T N, Eparvier F G 2007 Space Weather 5 S07005
[39] Rakesh Chandran S B, Veenas C L, Asitha L R, Parvathy B, Rakhimol K R, Abraham A, Rajesh S R, Sunitha A P, Renuka G 2022 Adv. Space Res. 70 546
Google Scholar
[40] Stubbs T J, Farrell W M, Halekas J S, Burchill J K, Collier M R, Zimmerman M I, Vondrak R R, Delory G T, Pfaff R F 2014 Planet. Space Sci. 90 10
Google Scholar
[41] Colwell J E, Gulbis A A, Horányi M, Robertson S 2005 Icarus 175 159
Google Scholar
[42] Gan H, Li X, Wei G, Wang S 2015 Adv. Space Res. 56 2432
Google Scholar
[43] Willis R F, Anderegg M, Feuerbacher B, Fitton B 1973 In Grard R J L, editor, Photon and Particle Interactions with Surfaces in Space, vol. 37 of Astrophys. Space Sci. Libr. p 389
[44] Zhao J, Wei X, Du X, He X, Han D 2021 IEEE Trans. Plasma Sci. 49 3036
Google Scholar
[45] Nitter T, Aslaksen T K, Melandso F, Havnes O 1994 IEEE Trans. Plasma Sci. 22 159
Google Scholar
[46] QIAN X Y, ZHANG Y Y, FANG Z, YANG J F, FANG Y W, LI S Q 2024 J. Astronaut. 45 613
[47] Poppe A, Horányi M 2010 J. Geophys. Res.: Space Phys. 115 A08106
[48] Hartzell C M 2019 Icarus 333 234
Google Scholar
[49] Popel S I, Golub’ A P, Kassem A I, Zelenyi L M 2022 Phys. Plasmas 29 013701
Google Scholar
下载:
计量
- 文章访问数: 155
- PDF下载量: 1
- 被引次数: 0
