基于监控标记单光子源的量子密钥分发协议

罗一振; 马洛嘉; 孙铭烁; 吴思睿; 邱丽华; 王禾; 王琴

doi:10.7498/aps.73.20241269

摘要

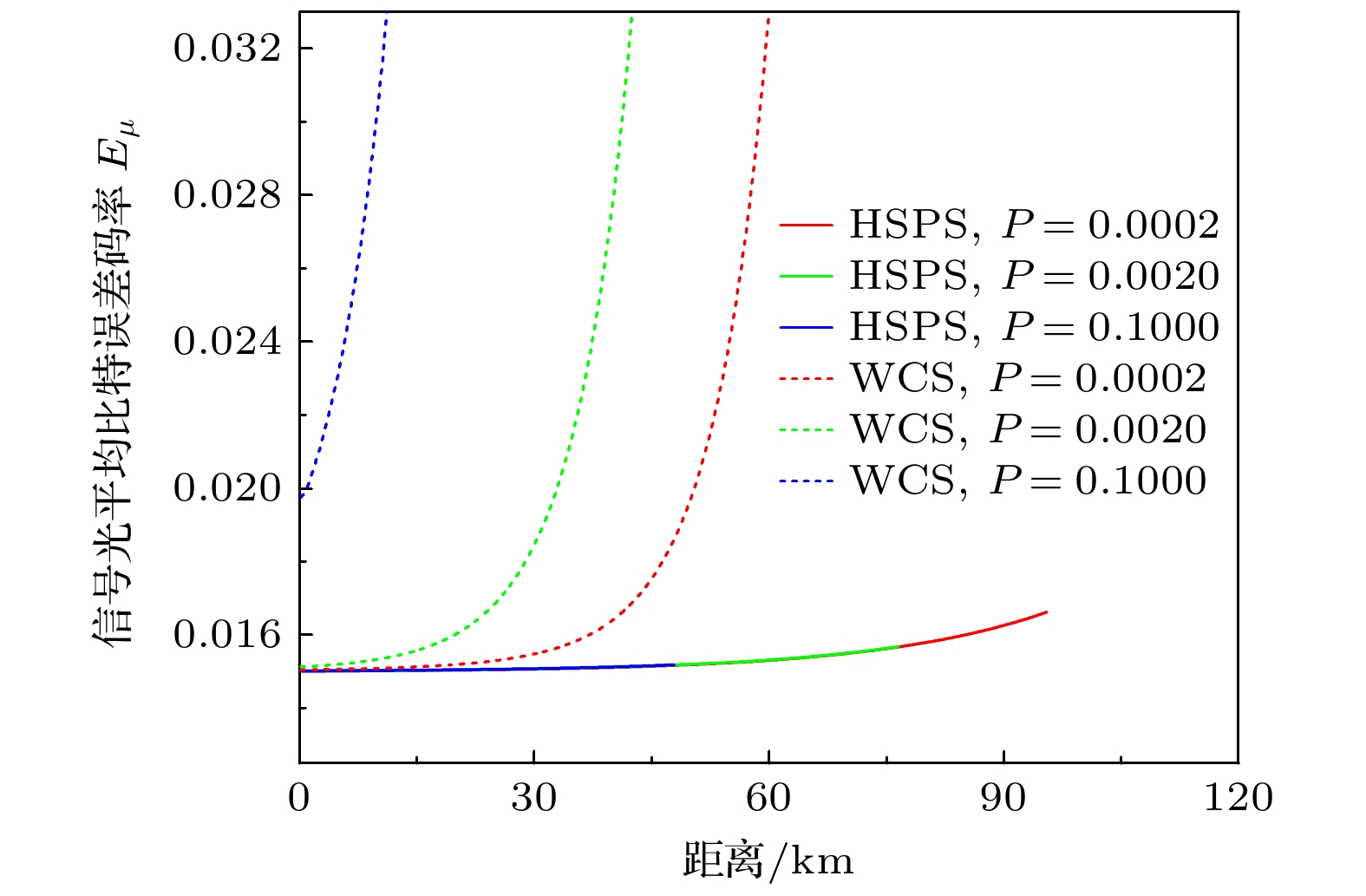
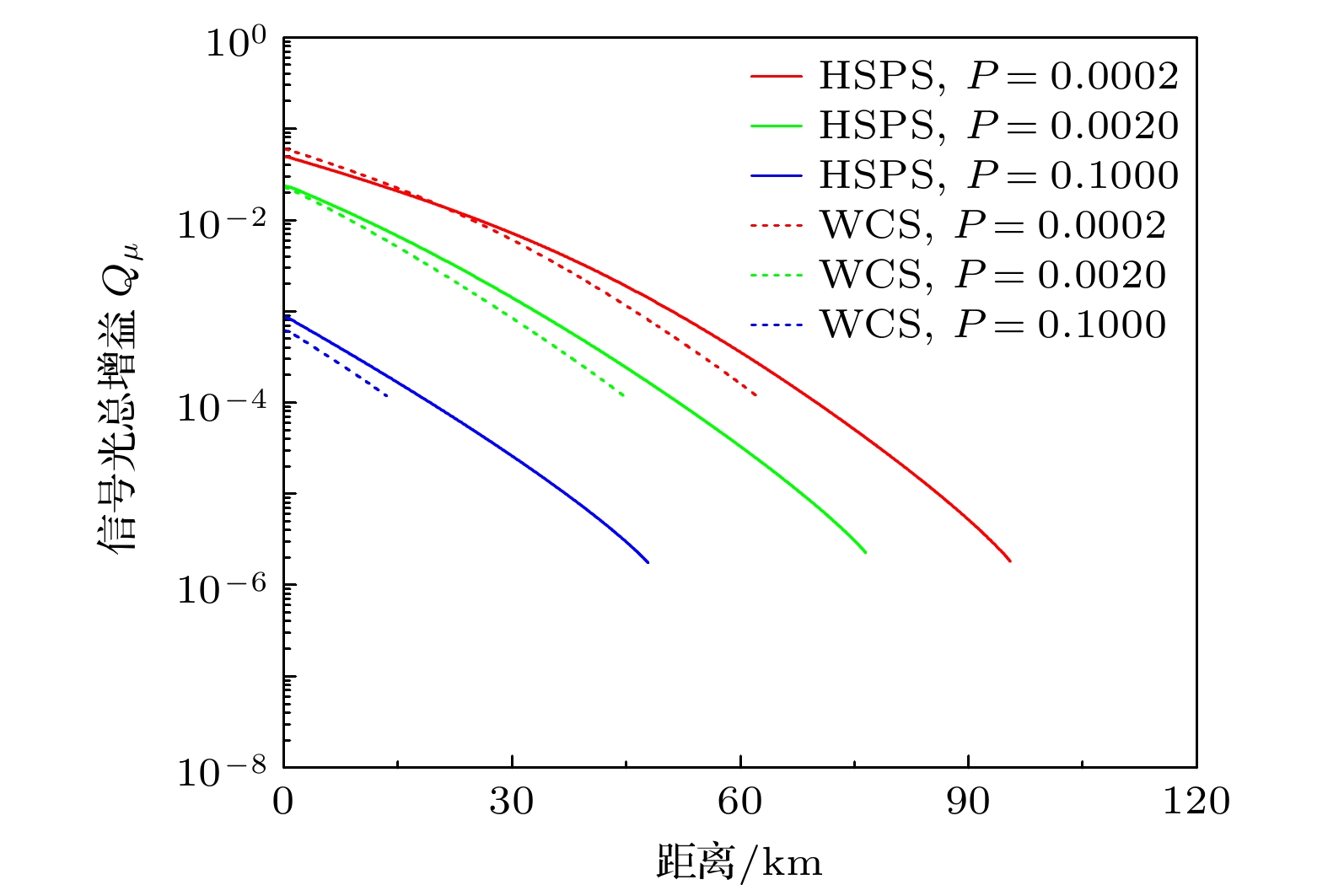
现有量子密钥分发系统的光源主要是弱相干态光源, 但是由于该类光源中含有大量的真空态脉冲, 并且在光源调制过程中可能存在一定信息泄漏, 从而限制了量子密钥分发系统的最远安全传输距离. 为克服这一局限, 本文提出了一种基于监控标记单光子源的量子密钥分发协议. 一方面, 通过借助标记单光子源中极低的真空态概率, 提升了系统的极限传输距离; 另一方面, 在系统发射端添加了Hong-Ou-Mandel (HOM)光源监控模块, 通过测量HOM干涉可见度的大小来精确刻画出源端可能泄漏信息量的大小, 从而更加准确地估算出系统可提取密钥率的大小. 此外, 将本工作与其他同类协议进行数值仿真对比, 仿真结果显示, 本协议在传输距离和密钥率等方面具有更加优越的性能. 因此, 本工作为未来发展更安全可靠的量子通信网络提供了重要的参考价值.

关键词:

Abstract

The security of quantum key distribution (QKD) is based on the basic principles of quantum mechanics, and therefore has unconditional security in theory. In existing quantum key distribution systems, weakly coherent sources (WCSs) are often used as light sources due to a high probability of vacuum pulses in these sources, resulting in limited transmission distances. Besides, there inevitably exist equipment defects in actual QKD systems, such as certain defects in phase modulators and intensity modulators, which lead to distinguishability of quantum states in higher dimensions and result in side-channel vulnerabilities. An eavesdropper can carry out corresponding attacks, thereby threatening the actual security of QKD systems. To overcome the above limitations, we propose an improved protocol on quantum key distribution based on monitoring heralded single-photon sources. Due to the simultaneity of parametric down-conversion photon pairs, we can accurately predict the arrival of one photon by measuring the arrival time of another photon. Through this way, we can greatly reduce the probability of vacuum states in the signal light, and increase the longest transmission distance of the QKD system. Moreover, a light source monitoring module is inserted into the sender’s side. By randomly selecting a certain period of time through the source monitoring module to measure the Hong-Ou-Mandel interference between the signal light and the idle light , we can estimate the side-channel information leakage of the source and then obtain the key generation rate.Compared with the QKD protocol based on monitoring weak coherent sources, our present protocol can give a better performance in either the transmission distance or the key generation rate, especially when the interference error is large. In addition, in principle, our present protocol can also be extended to other quantum key distribution protocols, such as the measurement-device-independent protocols, to further improve the security and practicability of QKD systems. Therefore, our present work can provide valuable references for realizing the large-scale application of quantum communication networks in the near future.

Keywords:

作者及机构信息

1.
南京邮电大学, 量子信息技术研究所, 南京　210003

2.
南京邮电大学, 宽带无线通信与传感网教育部重点实验室, 南京　210003

通信作者: 王琴, qinw@njupt.edu.cn

基金项目: 江苏省重点研发计划产业前瞻与关键核心技术项目 (批准号: BE2022071)、江苏省自然科学基金前沿技术项目 (批准号: BK20192001)、国家自然科学基金 (批准号: 12074194) 和江苏省研究生科研创新计划项目(批准号: KYCX22_0954)资助的课题.

Authors and contacts

1.
Institute of Quantum Information and Technology, Nanjing University of Posts and Telecommunications, Nanjing 210003, China

2.
Key Laboratory of Broadband Wireless Communication and Sensor Network of Ministry of Education, Nanjing University of Posts and Telecommunications, Nanjing 210003, China

Corresponding author: Wang Qin, qinw@njupt.edu.cn

Funds: Project supported by the Industrial Prospect and Key Core Technology Projects of Key R & D Program of Jiangsu Province, China (Grant No. BE2022071), the Leading-edge Technology Program of the Natural Science Foundation of Jiangsu Province, China (Grant No. BK20192001), the National Natural Science Foundation of China (Grant No. 12074194), and the Postgraduate Research & Practice Innovation Program of Jiangsu Province, China (Grant No. KYCX22_0954).

文章全文

参考文献

[1]	Bennett C H, Brassard G 1984 Proceedings of IEEE International Conference on Computers, System and Signal Processing (Vol. 1 of 3) (Bangalore: IEEE) p175
[2]	Shannon C E 1949 Bell Syst. Tech. J. 28 656 Google Scholar
[3]	Bennett C H, Brassard G, Mermin N D 1992 Phys. Rev. Lett. 68 557 Google Scholar
[4]	Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503 Google Scholar
[5]	Lucamarini M, Yuan Z L, Dynes J F, Shields A J 2018 Nature 557 400 Google Scholar
[6]	Zeng P, Zhou H Y, Wu W J, Ma X F 2022 Nat. Commun. 13 3903 Google Scholar
[7]	Xie Y M, Lu Y S, Weng C X, Cao X Y, Jia Z Y, Bao Y, Wang Y, Fu Yao, Yin H L, Chen Z B 2022 PRX Quantum 3 020315 Google Scholar
[8]	Tamaki K, Curty M, Lucamarini M 2016 New J. Phys. 18 065008 Google Scholar
[9]	Xu F H, Wei K J, Sajeed S, Kaiser S, Sun S, Tang Z Y, Qian L, Makarov V, Lo H K 2015 Phys. Rev. A 92 032305 Google Scholar
[10]	Sun S H, Gao M, Jiang M S, Li C Y, Liang L M 2012 Phys. Rev. A 85 032304 Google Scholar
[11]	Nauerth S, Fürst M, Schmitt-Manderbach T, Weier H, Weinfurter H 2009 New J. Phys. 11 065001 Google Scholar
[12]	Comandar L, Lucamarini M, Fröhlich B, Dynes J F, Yuan Z L, Shields A J 2016 Opt. Express 24 17849 Google Scholar
[13]	Mauerer W, Avenhaus, Helwig W, Silberhorn C 2009 Phys. Rev. A 80 053815 Google Scholar
[14]	Faruque I I, Sinclair G F, Bonneau D, Ono T, Silberhorn C, Thompson M G, Rarity J G 2019 Phys. Rev. Appl. 12 054029 Google Scholar
[15]	Wang J, Zhang C H, Liu J Y, Qian X R, Li J, Wang Q 2021 Chin. Phys. B 30 070304 Google Scholar
[16]	Zhou X Y, Zhang C H, Zhang C M, Wang Q 2017 Phys. Rev. A 96 052337 Google Scholar
[17]	Zhang C H, Zhang C M, Wang Q 2019 Phys. Rev. A 99 052325 Google Scholar
[18]	Alexander D, Denis S 2021 Phys. Rev. A 104 012601 Google Scholar
[19]	Wang Q, Wang X B, Guo G C 2007 Phys. Rev. A 75 012312 Google Scholar
[20]	Ma Z, Zhang F L, Chen J L 2009 Phys. Lett. A 373 3407 Google Scholar
[21]	Wang X B 2005 Phys. Rev. Lett. 94 230503 Google Scholar
[22]	Lo H K, Ma X F, Chen K 2005 Phys. Rev. Lett. 94 230504 Google Scholar
[23]	Tomamichel M, Lim C C W, Gisin N, Renner R 2012 Nat. Commun. 3 634 Google Scholar
[24]	Lucamarini M, Choi I, Ward M B, Dynes J F, Yuan Z L, Shields A J 2015 Phys. Rev. X 5 031030 Google Scholar
[25]	Sun M S, Wang W L, Zhou X Y, Zhang C H, Wang Q 2023 Phys. Rev. Res. 5 043179 Google Scholar
[26]	Zhan X H, Zhong Z Q, Wang S, Yin Z Q, Chen W, He D Y, Guo G C, Han Z F 2023 Phys. Rev. Appl. 20 034069 Google Scholar

施引文献

图 1 基于监控标记单光子源的QKD实验装置结构示意图

Fig. 1. Schematic diagram of QKD experimental device structure based on monitoring marker single photon source.

下载: 全尺寸图片幻灯片

图 2 不同干涉误差下基于监控HSPS协议和基于监控WCS协议的密钥率对比

Fig. 2. Comparison of the key rates based on monitoring HSPS protocol and monitoring WCS protocol under different interference errors.

下载: 全尺寸图片幻灯片

图 3 不同干涉误差下基于监控HSPS协议和基于监控WCS协议的平均误码率对比

Fig. 3. Comparison of the average bit error rates between monitoring HSPS protocol and monitoring WCS protocol under different interference errors.

下载: 全尺寸图片幻灯片

图 4 不同干涉误差下基于监控HSPS协议和基于监控WCS协议的信号光平均增益对比

Fig. 4. Comparison of the average gain of signal light between monitoring HSPS protocol and monitoring WCS protocol under different interference errors.

下载: 全尺寸图片幻灯片

表 1 基于监控标记单光子源的量子密钥分发协议仿真使用的参数列表

Table 1. List of the parameters used in the source monitoring quantum key distribution protocol based on heralded single photon source.

N α/(dB·km^–1) d_A η_A Y₀ e_d η_B

10¹⁰ 0.2 10^–6 0.75 6.02×10^–6 0.015 0.145

下载: 导出CSV

map

[1]	Bennett C H, Brassard G 1984 Proceedings of IEEE International Conference on Computers, System and Signal Processing (Vol. 1 of 3) (Bangalore: IEEE) p175
[2]	Shannon C E 1949 Bell Syst. Tech. J. 28 656 Google Scholar
[3]	Bennett C H, Brassard G, Mermin N D 1992 Phys. Rev. Lett. 68 557 Google Scholar
[4]	Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503 Google Scholar
[5]	Lucamarini M, Yuan Z L, Dynes J F, Shields A J 2018 Nature 557 400 Google Scholar
[6]	Zeng P, Zhou H Y, Wu W J, Ma X F 2022 Nat. Commun. 13 3903 Google Scholar
[7]	Xie Y M, Lu Y S, Weng C X, Cao X Y, Jia Z Y, Bao Y, Wang Y, Fu Yao, Yin H L, Chen Z B 2022 PRX Quantum 3 020315 Google Scholar
[8]	Tamaki K, Curty M, Lucamarini M 2016 New J. Phys. 18 065008 Google Scholar
[9]	Xu F H, Wei K J, Sajeed S, Kaiser S, Sun S, Tang Z Y, Qian L, Makarov V, Lo H K 2015 Phys. Rev. A 92 032305 Google Scholar
[10]	Sun S H, Gao M, Jiang M S, Li C Y, Liang L M 2012 Phys. Rev. A 85 032304 Google Scholar
[11]	Nauerth S, Fürst M, Schmitt-Manderbach T, Weier H, Weinfurter H 2009 New J. Phys. 11 065001 Google Scholar
[12]	Comandar L, Lucamarini M, Fröhlich B, Dynes J F, Yuan Z L, Shields A J 2016 Opt. Express 24 17849 Google Scholar
[13]	Mauerer W, Avenhaus, Helwig W, Silberhorn C 2009 Phys. Rev. A 80 053815 Google Scholar
[14]	Faruque I I, Sinclair G F, Bonneau D, Ono T, Silberhorn C, Thompson M G, Rarity J G 2019 Phys. Rev. Appl. 12 054029 Google Scholar
[15]	Wang J, Zhang C H, Liu J Y, Qian X R, Li J, Wang Q 2021 Chin. Phys. B 30 070304 Google Scholar
[16]	Zhou X Y, Zhang C H, Zhang C M, Wang Q 2017 Phys. Rev. A 96 052337 Google Scholar
[17]	Zhang C H, Zhang C M, Wang Q 2019 Phys. Rev. A 99 052325 Google Scholar
[18]	Alexander D, Denis S 2021 Phys. Rev. A 104 012601 Google Scholar
[19]	Wang Q, Wang X B, Guo G C 2007 Phys. Rev. A 75 012312 Google Scholar
[20]	Ma Z, Zhang F L, Chen J L 2009 Phys. Lett. A 373 3407 Google Scholar
[21]	Wang X B 2005 Phys. Rev. Lett. 94 230503 Google Scholar
[22]	Lo H K, Ma X F, Chen K 2005 Phys. Rev. Lett. 94 230504 Google Scholar
[23]	Tomamichel M, Lim C C W, Gisin N, Renner R 2012 Nat. Commun. 3 634 Google Scholar
[24]	Lucamarini M, Choi I, Ward M B, Dynes J F, Yuan Z L, Shields A J 2015 Phys. Rev. X 5 031030 Google Scholar
[25]	Sun M S, Wang W L, Zhou X Y, Zhang C H, Wang Q 2023 Phys. Rev. Res. 5 043179 Google Scholar
[26]	Zhan X H, Zhong Z Q, Wang S, Yin Z Q, Chen W, He D Y, Guo G C, Han Z F 2023 Phys. Rev. Appl. 20 034069 Google Scholar

计量

文章访问数: 2423
PDF下载量: 76
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于监控标记单光子源的量子密钥分发协议