基于相干反馈的相敏放大器强度差压缩增强研究

刘婷婷; 杨晓华; 韩亚帅; 王军民

doi:10.7498/aps.73.20240407

摘要

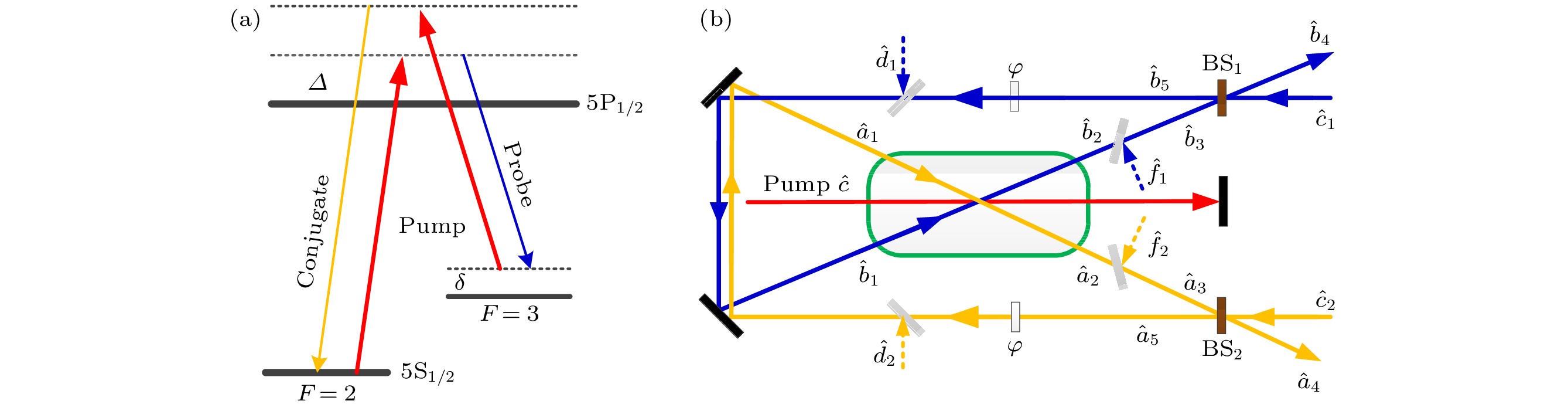
基于原子四波混频的光参量放大器是实现强度差压缩态光场的最有效手段之一. 然而, 受限于原子蒸气对光场的吸收损耗, 其输出的压缩度仍有待提升. 光参量放大器输出的非经典光场部分反馈回输入端口, 可实现其输出光场量子特性的增强. 本文对相干反馈的相敏放大器开展理论分析, 研究了其输出光场的强度差压缩与反馈强度、强度增益和损耗的依赖关系. 研究结果表明, 在无损耗的理想情况下, 通过调控反馈强度和位相可实现无穷大的压缩输出. 在考虑实际的实验参数条件下, 此方案也可在一定的反馈强度范围内和特定的位相条件下, 实现显著的压缩增强. 本研究结果可为实验实现高质量非经典光场提供有益的参考.

关键词:

Abstract

The intensity-difference squeezed state is an important concept in quantum optics, which is not only of great significance for fundamental research in quantum physics, but also an important quantum resource in the fields of quantum communication, quantum computing, and quantum precision measurement. The optical parametric amplifier based on atomic four-wave mixing is one of the most effective means to achieve intensity-difference squeezed light. However, due to the absorption loss of atomic vapor in the light field, the output squeezing still needs improving. By feeding the non-classical optical field from the optical parametric amplifier back to the input port, the quantum characteristics of its output optical field can be enhanced. However, the intensity-difference squeezing enhancement from a phase-insensitive amplifier is experimentally realized based on coherent feedback control. The intensity-difference squeezing enhancement of the phase-sensitive amplifier has not been discussed. In this work, a two-port coherent feedback-controlled phase-sensitive amplifier is analyzed theoretically. The dependence of the intensity-difference squeezing, respectively, on the feedback intensity, the intensity gain of the optical parametric amplifier, and the losses of the system are investigated. For the ideal case in which the losses of the system are ignored, infinite squeezing can be achieved by adjusting the strength and phase of feedback. Considering the actual atomic absorption losses, squeezing enhancement can also be achieved over a wide range of intensity gains within a certain feedback intensity range. In addition, the squeezing enhancement is quite efficient for the medium intensity gain range. The intensity-difference squeezing enhancement strongly depends on the absorption loss of atomic vapor. The smaller the absorption loss, the more significant the squeezing enhancement effect is. Furthermore, the experimental feasibility of this scheme is also considered in detail. Our research can provide useful references for achieving high-quality non classical light fields in experiment, which may find applications in quantum information processing and quantum precise measurement.

Keywords:

作者及机构信息

1.
山西科技学院通识教育学院, 晋城　048000

2.
安徽师范大学物理与电子信息学院, 芜湖　241000

3.
安徽师范大学, 光电信息材料功能调控与应用安徽省重点实验室, 芜湖　241000

4.
山西大学光电研究所, 量子光学与光量子器件国家重点实验室, 太原　030006

5.
山西大学, 极端光学协同创新中心, 太原　030006

通信作者: 韩亚帅, hanyashuai@ahnu.edu.cn ; 王军民, wwjjmm@sxu.edu.cn

基金项目: 国家自然科学基金(批准号: 12104013, 11974226)资助的课题.

Authors and contacts

1.
College of General Education, Shanxi Institute of Science and Technology, Jincheng 048000, China

2.
College of Physics and Electronic Information, Anhui Normal University, Wuhu 241000, China

3.
Anhui Province Key Laboratory for Control and Applications of Optoelectronic Information Materials, Anhui Normal University, Wuhu 241000, China

4.
State Key Laboratory of Quantum Optics and Quantum Optics Devices, Institute of Opto-Electronics, Shanxi University, Taiyuan 030006, China

5.
Collaborative Innovation Center of Extreme Optics, Shanxi University, Taiyuan 030006, China

Corresponding author: Han Ya-Shuai, hanyashuai@ahnu.edu.cn ; Wang Jun-Min, wwjjmm@sxu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12104013, 11974226).

文章全文 : translate this paragraph

参考文献

[1]	Slusher R E, Hollberg L W, Yurke B, Mertz J C, Valley J F 1985 Phys. Rev. Lett. 55 2409 Google Scholar
[2]	Wu L A, Kimble H J, Hall J L, Wu H F 1986 Phys. Rev. Lett. 57 2520 Google Scholar
[3]	Marino M, Pooser R C, Boyer V, Lett P D 2009 Nature 457 859 Google Scholar
[4]	Wu S H, Bao G Z, Guo J X, Chen J, Du W, Shi M W, Yang P Y, Chen L Q, Zhang W P 2023 Sci. Adv. 9 1760 Google Scholar
[5]	Liu S S, Lou Y B, Chen Y X, Jing J T 2022 Phys. Rev. Lett. 128 060503 Google Scholar
[6]	Wang D, Zhang Y, Xiao M 2013 Phys. Rev. A 87 023834 Google Scholar
[7]	韩亚帅, 张啸, 张昭, 屈军, 王军民 2022 71 074202 Google Scholar Han Y S, Zhang X, Zhang Z, Qu J, Wang J M 2022 Acta Phys. Sin. 71 074202 Google Scholar
[8]	杨荣国, 张超霞, 李妮, 张静, 郜江瑞 2019 68 094205 Google Scholar Yang R G, Zhang C X, Li N, Zhang J, Gao J R 2019 Acta Phys. Sin. 68 094205 Google Scholar
[9]	Shang Y N, Jia X J, Shen Y M, Xie C D, Peng K C 2010 Opt. Lett. 35 853 Google Scholar
[10]	Xin J, Qi J, Jing J T 2017 Opt. Lett. 42 366 Google Scholar
[11]	Lou Y B, Chen Y X, Wang J B, Liu S S, Jing J T 2023 Sci. China Phys. Mech. 66 250311 Google Scholar
[12]	Gough J E, Wildfeuer S 2009 Phys. Rev. A 80 042107 Google Scholar
[13]	Iida S, Yukawa M, Yonezawa H, Yamamoto N, Furusawa A 2012 IEEE Trans. Autom. Control 57 2045 Google Scholar
[14]	Yan Z H, Jia X J, Su X L, Duan Z Y, Xie C D, Peng K C 2012 Phys. Rev. A 85 040305 Google Scholar
[15]	Pan X C, Chen H, Wei T X, Zhang J, Marino A M, Treps N, Glasser R T, Jing J T 2018 Phys. Rev. B 97 161115 Google Scholar
[16]	Zhong Y Y, Jing J T 2020 Phys. Rev. A 101 023813 Google Scholar
[17]	Fang Y M, Jing J T 2015 New J. Phys. 17 023027 Google Scholar
[18]	Liu S S, Lou Y B, Jing J T 2019 Phys. Rev. Lett. 123 113602 Google Scholar

施引文献

图 1 (a)基于⁸⁵Rb原子D1谱线的双-Λ能级结构; (b)相干反馈的相敏放大器理论模型

Fig. 1. (a) Double-Λ energy level diagram of ⁸⁵Rb D1 transition line; (b) schematic of the coherent feedback-controlled phase-sensitive amplifier.

下载: 全尺寸图片幻灯片

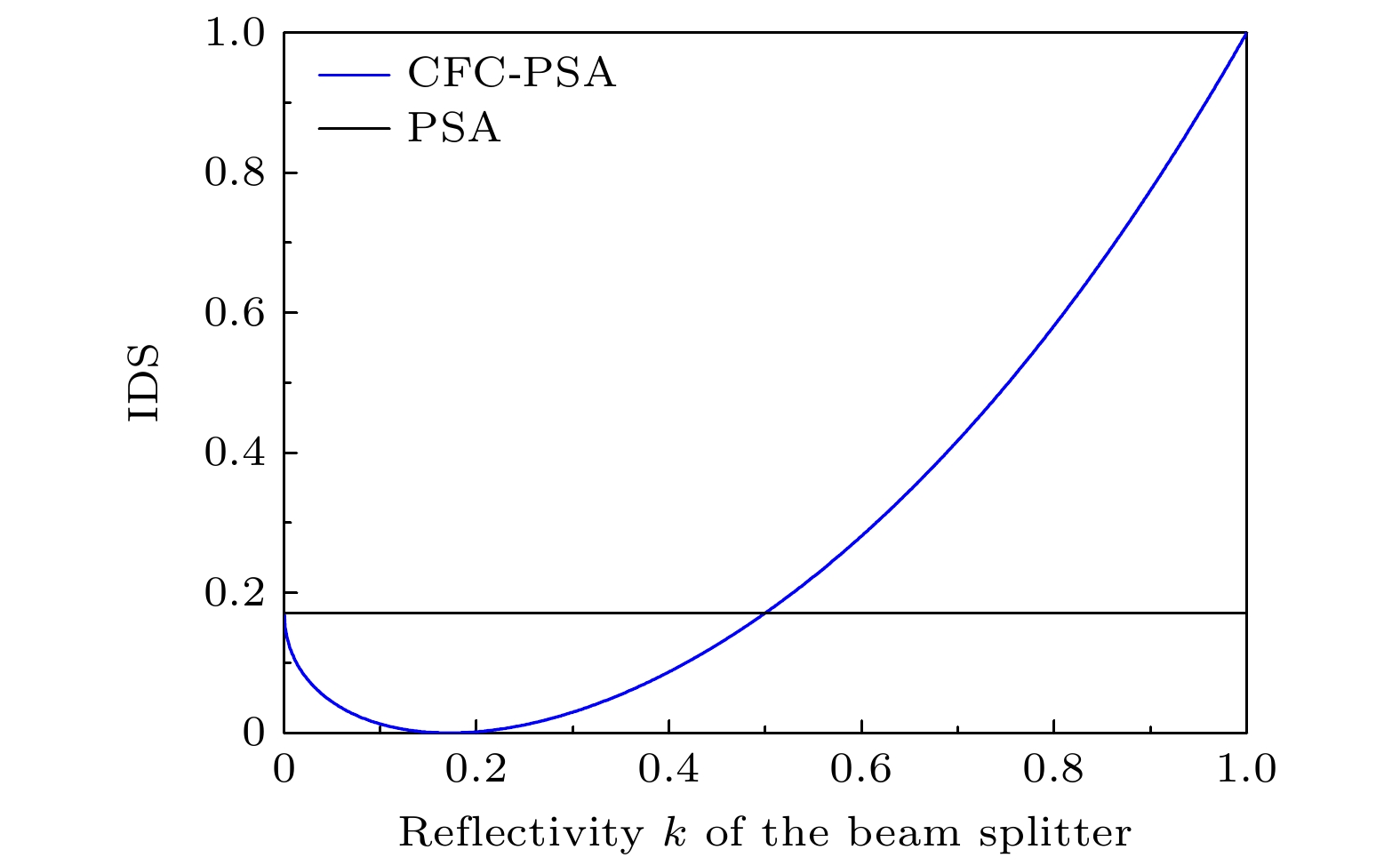
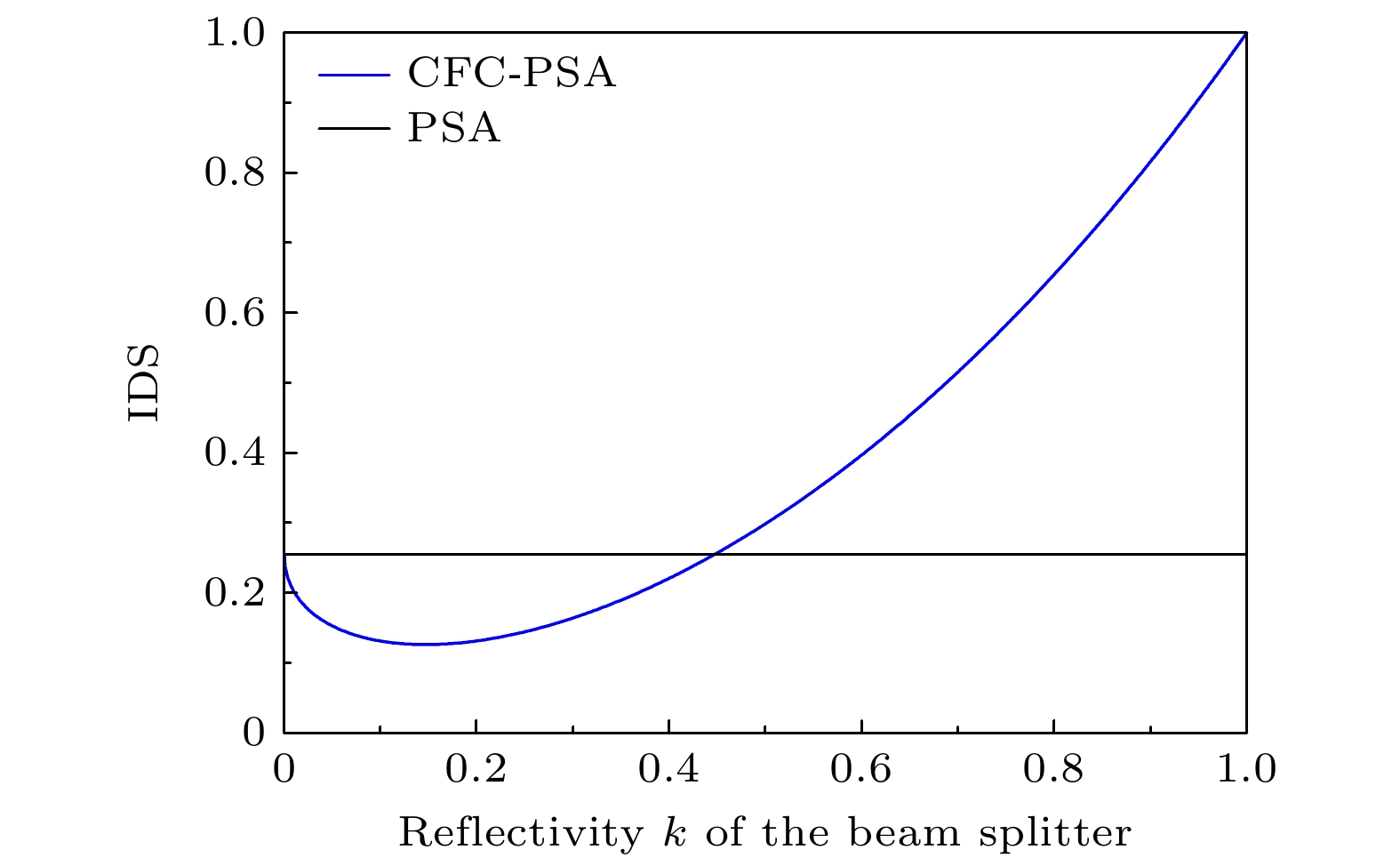
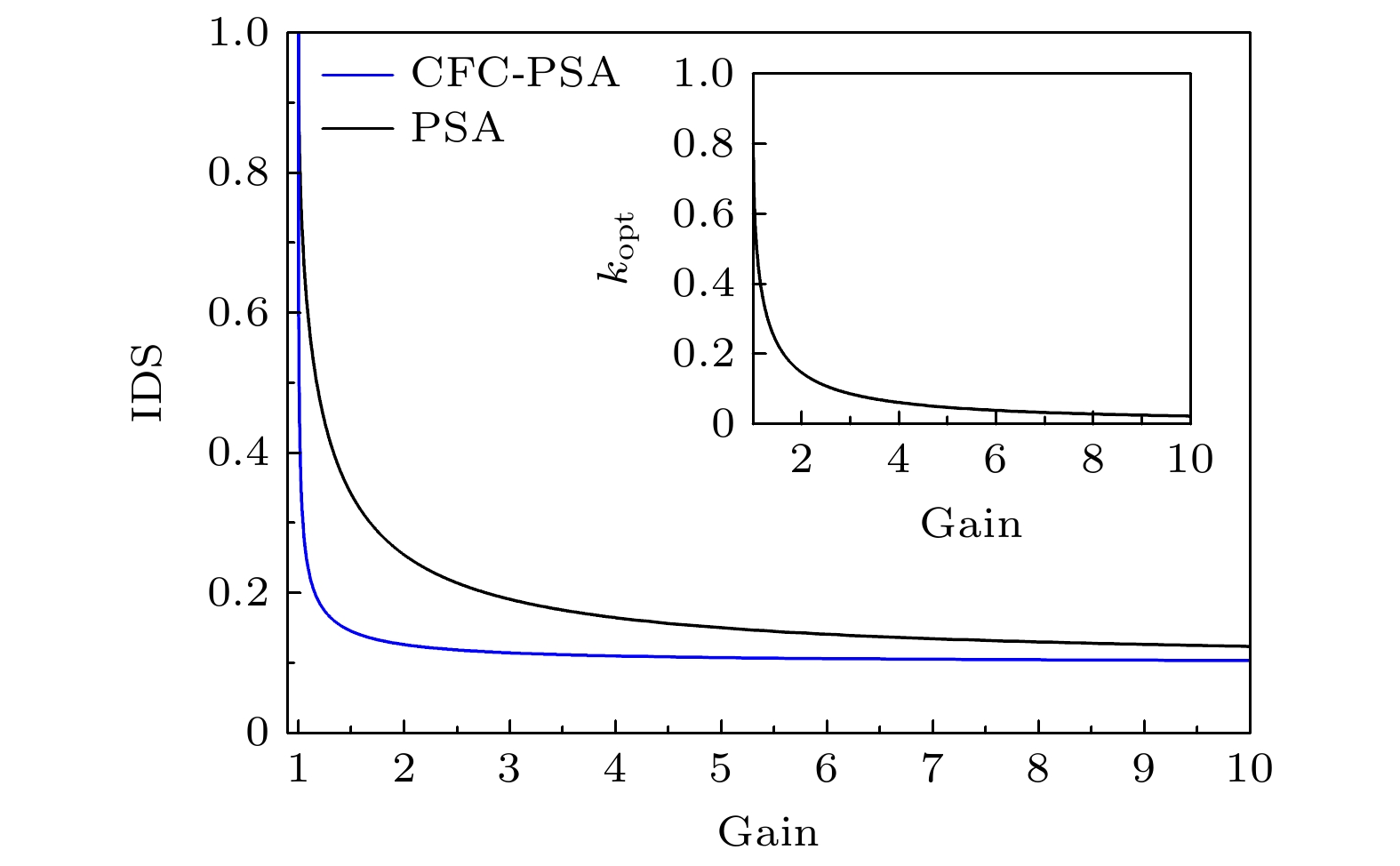
图 2 理想情况下强度差压缩随分束器反射率k的变化曲线. IDS为强度差压缩; PSA为相敏放大器; CFC-PSA为相干反馈控制的相位灵敏放大器

Fig. 2. Curve of intensity-difference squeezing versus reflectivity k of the beam splitter under the ideal condition. IDS represents intensity-difference squeezing; PSA represents phase-sensitive amplifier; CFC-PSA represents coherent feedback-controlled phase-sensitive amplifier.

下载: 全尺寸图片幻灯片

图 3 实际实验参数情况下强度差压缩随分束器反射率k的变化曲线

Fig. 3. Curve of intensity-difference squeezing versus reflectivity k of the beam splitter under the practical experimental parameters.

下载: 全尺寸图片幻灯片

图 4 实际实验参数情况下强度差压缩随强度增益G的变化曲线

Fig. 4. Curve of intensity-difference squeezing versus intensity gain G under the practical experimental parameters.

下载: 全尺寸图片幻灯片

图 5 实际实验参数情况下强度差压缩随铷池内部传输效率η₁的变化曲线

Fig. 5. Curve of intensity-difference squeezing versus the internal transmission efficiency of rubidium cells under the practical experimental parameters.

下载: 全尺寸图片幻灯片

图 6 相干反馈的相敏放大器实验系统设计

Fig. 6. Experimental system design on coherent feedback controlled PSA.

下载: 全尺寸图片幻灯片

map

[1]	Slusher R E, Hollberg L W, Yurke B, Mertz J C, Valley J F 1985 Phys. Rev. Lett. 55 2409 Google Scholar
[2]	Wu L A, Kimble H J, Hall J L, Wu H F 1986 Phys. Rev. Lett. 57 2520 Google Scholar
[3]	Marino M, Pooser R C, Boyer V, Lett P D 2009 Nature 457 859 Google Scholar
[4]	Wu S H, Bao G Z, Guo J X, Chen J, Du W, Shi M W, Yang P Y, Chen L Q, Zhang W P 2023 Sci. Adv. 9 1760 Google Scholar
[5]	Liu S S, Lou Y B, Chen Y X, Jing J T 2022 Phys. Rev. Lett. 128 060503 Google Scholar
[6]	Wang D, Zhang Y, Xiao M 2013 Phys. Rev. A 87 023834 Google Scholar
[7]	韩亚帅, 张啸, 张昭, 屈军, 王军民 2022 71 074202 Google Scholar Han Y S, Zhang X, Zhang Z, Qu J, Wang J M 2022 Acta Phys. Sin. 71 074202 Google Scholar
[8]	杨荣国, 张超霞, 李妮, 张静, 郜江瑞 2019 68 094205 Google Scholar Yang R G, Zhang C X, Li N, Zhang J, Gao J R 2019 Acta Phys. Sin. 68 094205 Google Scholar
[9]	Shang Y N, Jia X J, Shen Y M, Xie C D, Peng K C 2010 Opt. Lett. 35 853 Google Scholar
[10]	Xin J, Qi J, Jing J T 2017 Opt. Lett. 42 366 Google Scholar
[11]	Lou Y B, Chen Y X, Wang J B, Liu S S, Jing J T 2023 Sci. China Phys. Mech. 66 250311 Google Scholar
[12]	Gough J E, Wildfeuer S 2009 Phys. Rev. A 80 042107 Google Scholar
[13]	Iida S, Yukawa M, Yonezawa H, Yamamoto N, Furusawa A 2012 IEEE Trans. Autom. Control 57 2045 Google Scholar
[14]	Yan Z H, Jia X J, Su X L, Duan Z Y, Xie C D, Peng K C 2012 Phys. Rev. A 85 040305 Google Scholar
[15]	Pan X C, Chen H, Wei T X, Zhang J, Marino A M, Treps N, Glasser R T, Jing J T 2018 Phys. Rev. B 97 161115 Google Scholar
[16]	Zhong Y Y, Jing J T 2020 Phys. Rev. A 101 023813 Google Scholar
[17]	Fang Y M, Jing J T 2015 New J. Phys. 17 023027 Google Scholar
[18]	Liu S S, Lou Y B, Jing J T 2019 Phys. Rev. Lett. 123 113602 Google Scholar

[1]	孙小聪, 李卫, 王雅君, 郑耀辉. 基于压缩态光场的量子增强型光学相位追踪. , 2024, 73(5): 054203. doi: 10.7498/aps.73.20231835
[2]	韩亚帅, 张啸, 张昭, 屈军, 王军民. 基于级联光参量放大器的碱金属原子跃迁线波段压缩光源分析. , 2022, 71(7): 074202. doi: 10.7498/aps.71.20212131
[3]	李庆回, 姚文秀, 李番, 田龙, 王雅君, 郑耀辉. 明亮压缩态光场的操控及量子层析. , 2021, 70(15): 154203. doi: 10.7498/aps.70.20210318
[4]	刘奎, 马龙, 苏必达, 李佳明, 孙恒信, 郜江瑞. 基于非简并光学参量放大器产生光学频率梳纠缠态. , 2020, 69(12): 124203. doi: 10.7498/aps.69.20200107
[5]	左冠华, 杨晨, 赵俊祥, 田壮壮, 朱诗尧, 张玉驰, 张天才. 基于参量放大器的铯原子D₂线明亮偏振压缩光源的产生. , 2020, 69(1): 014207. doi: 10.7498/aps.69.20191009
[6]	仲银银, 潘晓州, 荆杰泰. 级联四波混频相干反馈控制系统量子纠缠特性. , 2020, 69(13): 130301. doi: 10.7498/aps.69.20200042
[7]	王俊萍, 张文慧, 李瑞鑫, 田龙, 王雅君, 郑耀辉. 宽频带压缩态光场光学参量腔的设计. , 2020, 69(23): 234204. doi: 10.7498/aps.69.20200890
[8]	冯晋霞, 杜京师, 靳晓丽, 李渊骥, 张宽收. 音频段1.34 μm压缩态光场的实验制备. , 2018, 67(17): 174203. doi: 10.7498/aps.67.20180301
[9]	刘增俊, 翟泽辉, 孙恒信, 郜江瑞. 低频压缩态光场的制备. , 2016, 65(6): 060401. doi: 10.7498/aps.65.060401
[10]	尤良芳, 令维军, 李可, 张明霞, 左银燕, 王屹山. 基于单个BBO晶体载波包络相位稳定的高效率光参量放大器. , 2014, 63(21): 214203. doi: 10.7498/aps.63.214203
[11]	严晓波, 杨柳, 田雪冬, 刘一谋, 张岩. 参量放大器腔中光力诱导透明与本征模劈裂性质. , 2014, 63(20): 204201. doi: 10.7498/aps.63.204201
[12]	俞立先, 梁奇锋, 汪丽蓉, 朱士群. Rabi模型的光场压缩. , 2013, 62(16): 160301. doi: 10.7498/aps.62.160301
[13]	张岩, 于旭东, 邸克, 李卫, 张靖. 压缩态光场平衡零拍探测的位相锁定. , 2013, 62(8): 084204. doi: 10.7498/aps.62.084204
[14]	孙涛, 黄锦圣, 张伟力, 王清月. 输出13.5fs激光脉冲的非共线式光参量放大器. , 2002, 51(10): 2281-2285. doi: 10.7498/aps.51.2281
[15]	姚春梅, 郭光灿. 压缩相干态腔场的类自旋GHZ态的制备. , 2001, 50(1): 59-62. doi: 10.7498/aps.50.59
[16]	冯勋立, 徐至展, 夏宇兴. 压缩真空态光场抽运的双光子激光. , 2000, 49(2): 235-240. doi: 10.7498/aps.49.235
[17]	张俊香, 贺凌翔, 张天才, 谢常德, 彭昆墀. 压缩态光场的四阶量子干涉. , 1999, 48(7): 1230-1235. doi: 10.7498/aps.48.1230
[18]	何林生, 江海河. 组合光场增强压缩真空场中原子冷却作用. , 1995, 44(12): 1904-1913. doi: 10.7498/aps.44.1904
[19]	刘甲壬, 赵波, 王育竹. 负反馈提高高阻恒流源驱动的LED的输出光场噪声压缩量. , 1994, 43(10): 1598-1604. doi: 10.7498/aps.43.1598
[20]	彭堃墀, 黄茂全, 刘晶, 廉毅敏, 张天才, 于辰, 谢常德, 郭光灿. 双模光场压缩态的实验研究. , 1993, 42(7): 1079-1085. doi: 10.7498/aps.42.1079

计量

文章访问数: 1478
PDF下载量: 45
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于相干反馈的相敏放大器强度差压缩增强研究