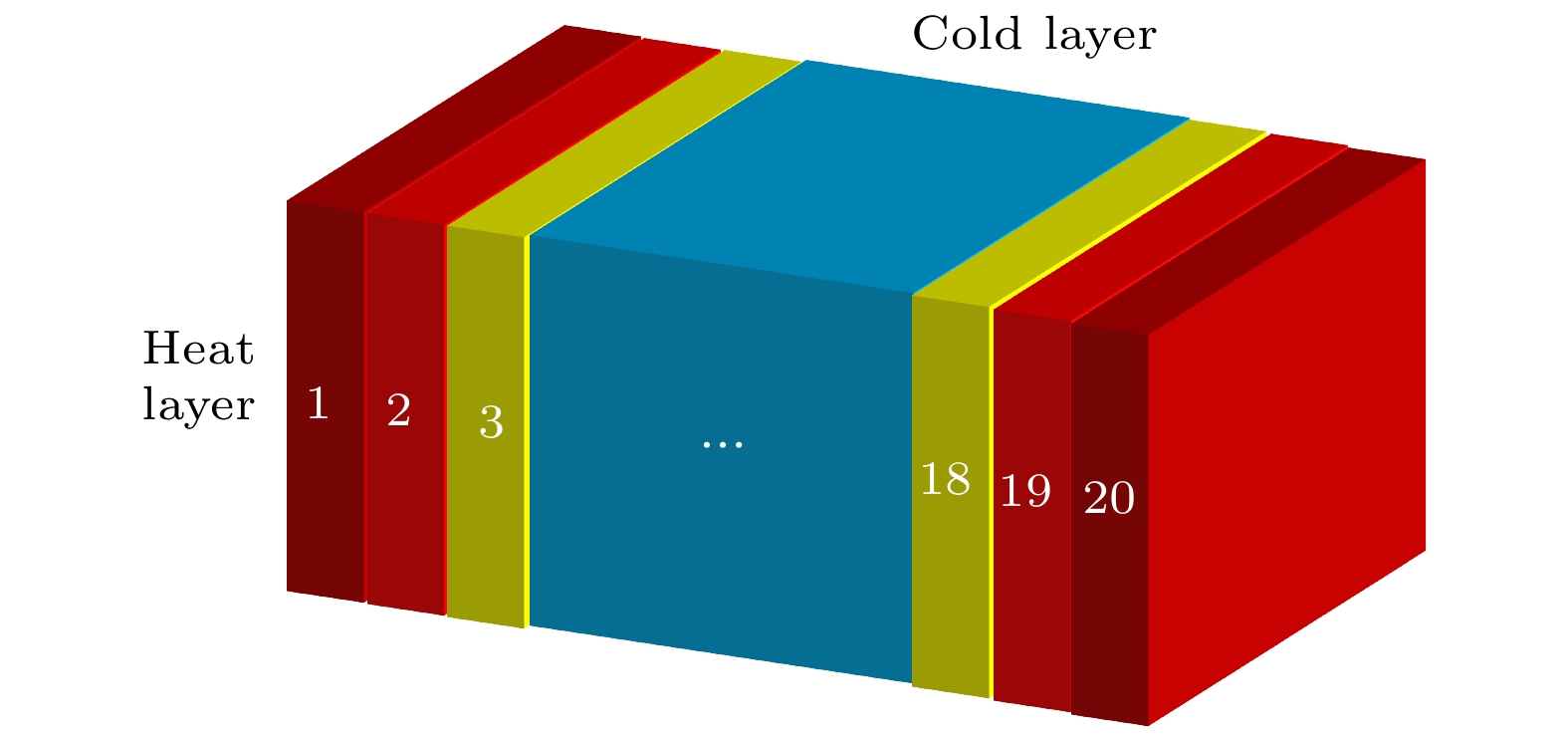
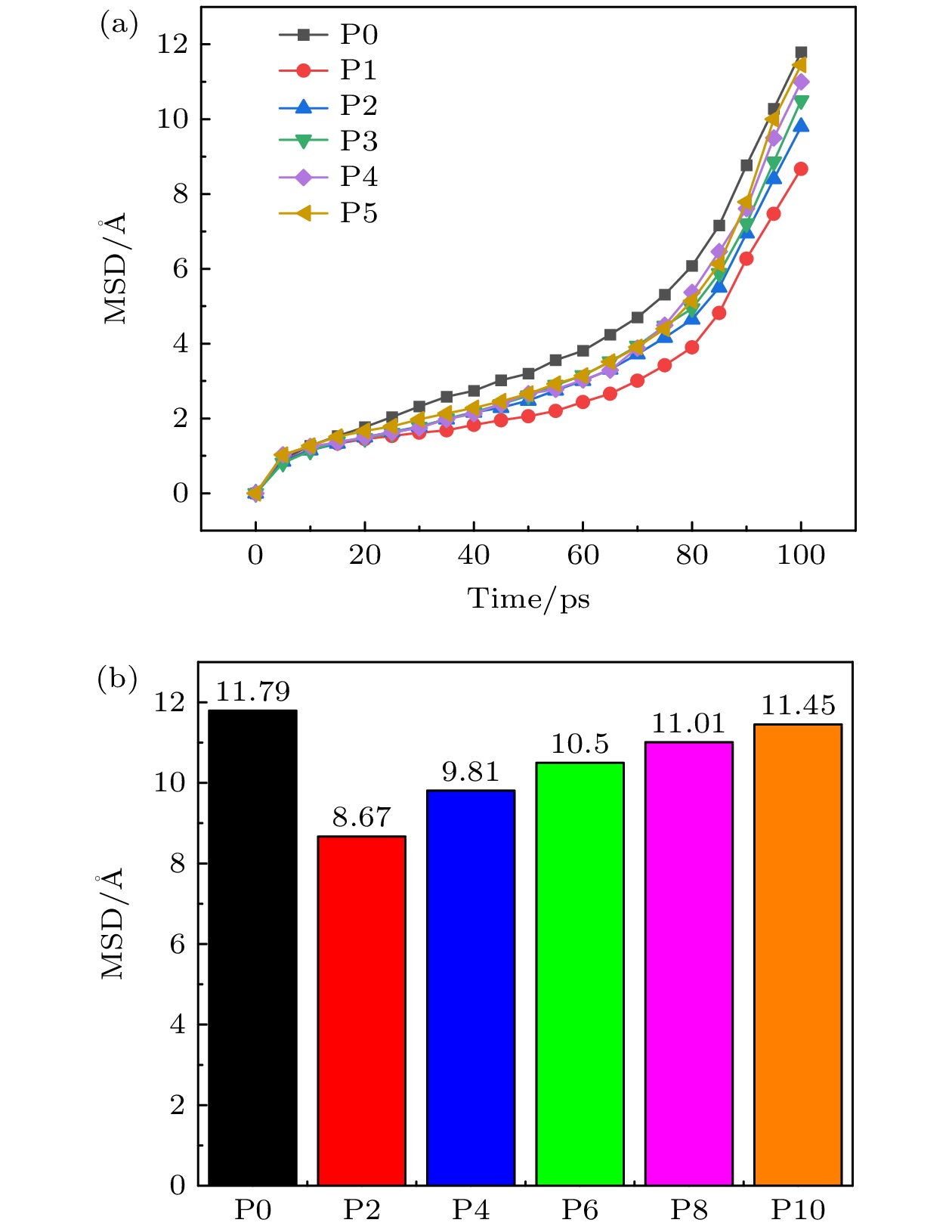
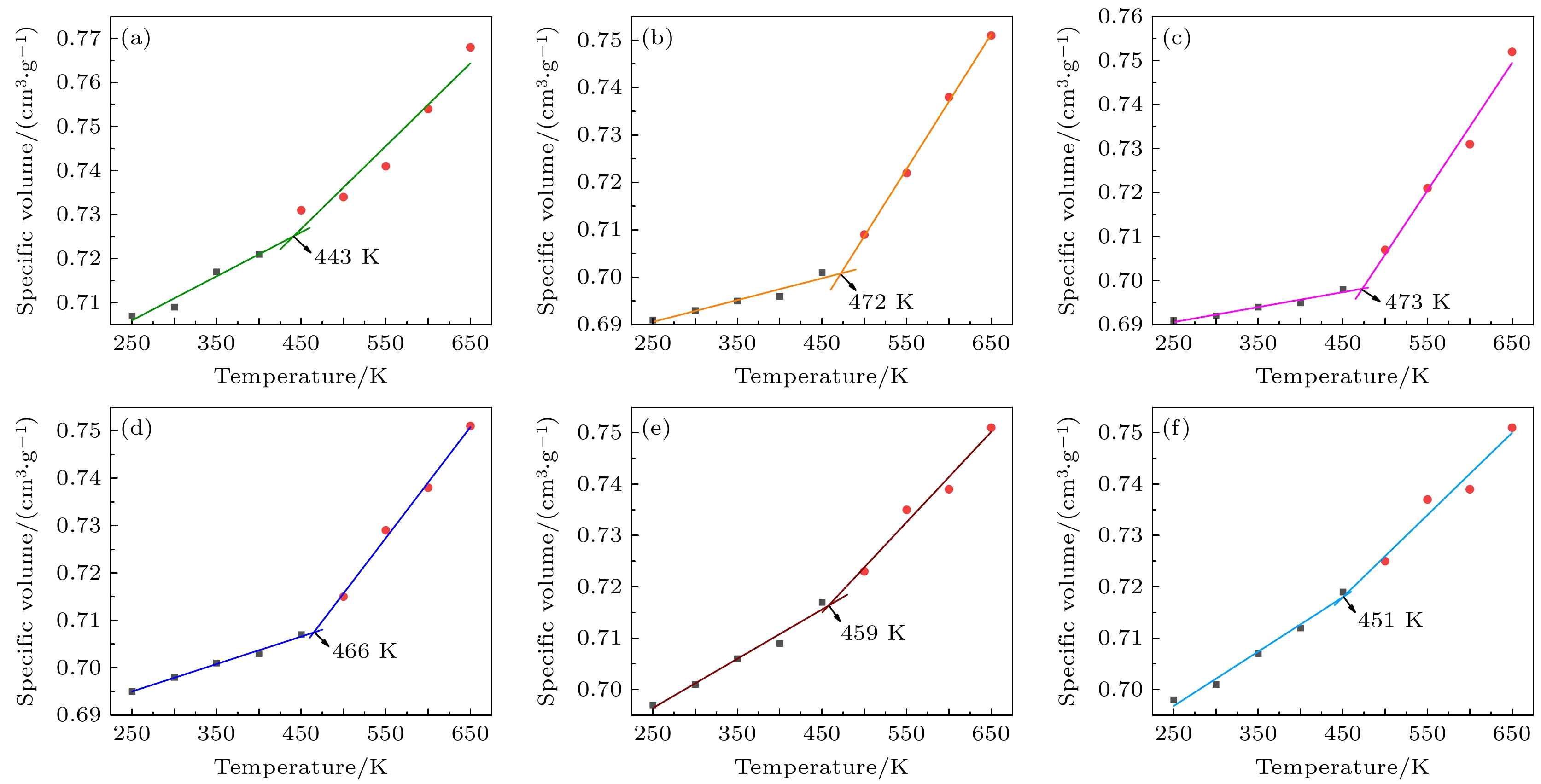
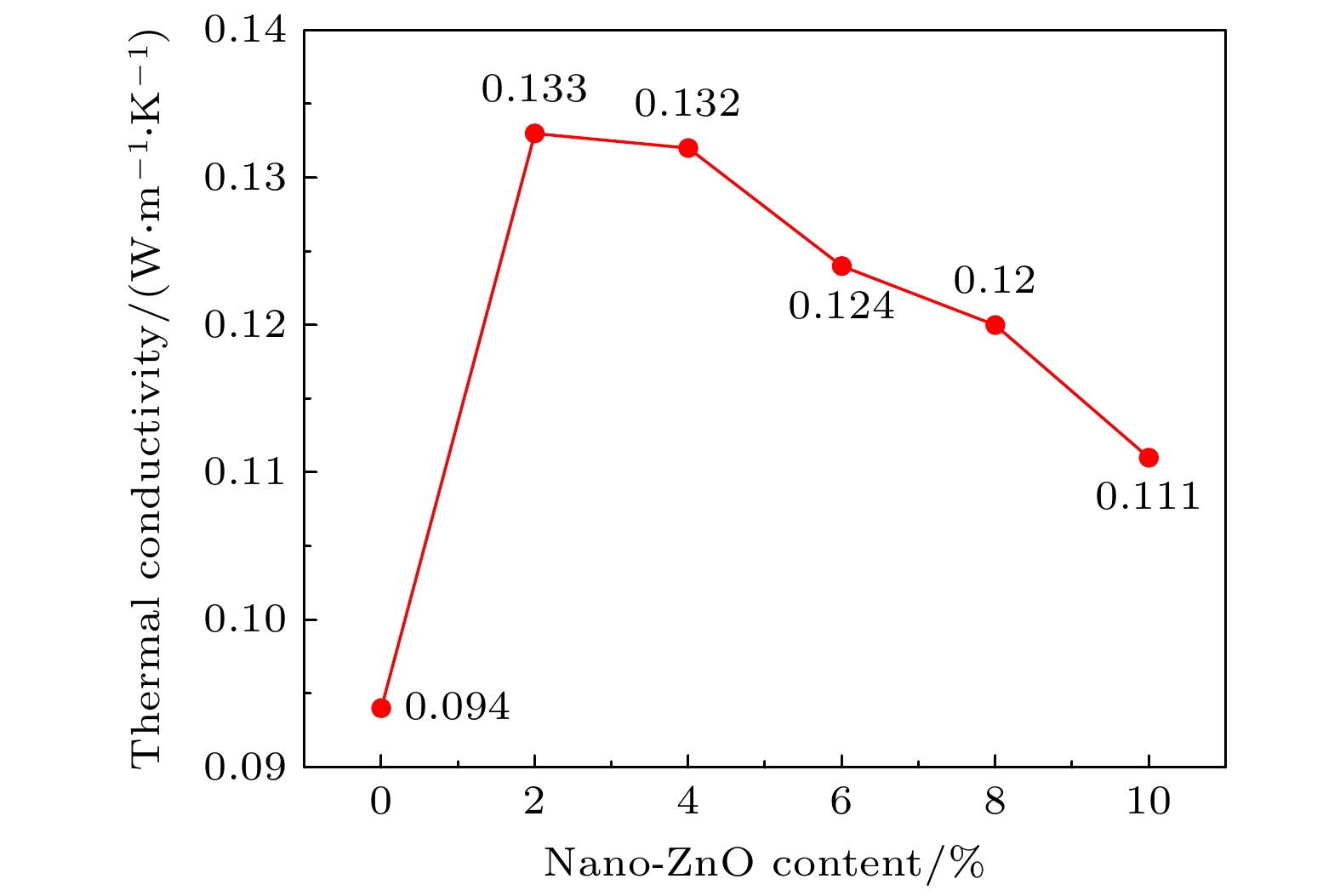
-
随着电力负荷激增和电压水平不断提高, 绝缘纸的力学性能和热稳定性面临着严峻挑战. 然而, 由于缺乏直接的科学理论或模拟指导, 传统低效的“试错性”试验难以快速高效地研发新型纤维素复合绝缘纸. 针对这一问题, 本文提出通过分子动力学模拟, 研究纳米氧化锌(nano-ZnO)对纤维素的力学和热学性能的提升效果. 首先设计了nano-ZnO/纤维素复合材料模型, 然后从微观角度分析了不同nano-ZnO含量的改性纤维素的力学性能和热稳定性, 从而确定nano-ZnO和纤维素的最佳配比. 结果表明, 相比于未改性模型, nano-ZnO改性纤维素模型的力学性能、内聚能密度、玻璃化转变温度和导热系数均有提升, 弹性模量最高提升了45.31%, 导热系数最高提升了41.49%. 因为nano-ZnO的加入能够有效填充纤维网络中的空隙, 并增强纤维素链之间的作用力和导热通道, 从而提升纤维素的热力学性能. 本工作为可快速制备出具有优良热力学性能的改性纤维素绝缘纸提供有价值的理论参考.With the surge in electrical loads and increasing voltage levels, the mechanical performance and thermal stability of insulating paper are facing severe challenges. However, due to the lack of direct scientific theories or simulation guidance, traditional inefficient “trial-and-error” experiments are difficult to effectively develop new types of cellulose composite insulating papers. For solving this problem, in this work we are to enhance the effects of nanoscale zinc oxide (nano-ZnO) on the mechanical and thermal properties of cellulose through molecular dynamics simulations. Initially, we model the nano-ZnO/cellulose composite material , then carry out a microscopic analysis of the mechanical performance and thermal stability of modified cellulose with varying nano-ZnO content, thus determining the optimal ratio of nano-ZnO to cellulose. The results indicate that compared with the outcomes from the unmodified model, the mechanical performance, cohesive energy density, glass transition temperature, and thermal conductivity of the nano-ZnO-modified cellulose model are all improved, with the highest increase in elastic modulus reaching 45.31% and the highest increase in thermal conductivity attaining 41.49%. The addition of nano-ZnO effectively fills the gaps in the fiber network and enhances the interactions between cellulose chains and thermal conduction channels, thereby improving the thermodynamic performance of cellulose. This work provides valuable theoretical references for rapidly preparing modified cellulose insulating papers with excellent thermodynamic performance.
-
Keywords:
- insulating paper /
- nano-ZnO /
- mechanical properties /
- thermal stability
[1] Shadfar H, Pashakolaei M G, Foroud A A 2021 Int. T. Electr. Energy 31 24
Google Scholar
[2] Chen Q G, Li C P, Cheng S, Sun W, Chi M H, Zhang H 2022 IEEE T. Dielect. El. In. 29 591
Google Scholar
[3] Tang C, Chen R, Zhang J Z, Peng X, Chen B H, Zhang L S 2022 IET Nanodielectrics 5 63
Google Scholar
[4] Yang L, Gao J, Peng X, Qin J S, Tang C 2021 Cellulose 28 6023
Google Scholar
[5] Tang C, Zhang S, Wang X B, Hao J 2018 Cellulose 25 3619
Google Scholar
[6] Chen Q J, Kang M C, Xie Q H, Wang J H 2020 Cellulose 27 7621
Google Scholar
[7] Ji D Y, Li T, Hu W P, Fuchs H 2019 Adv. Mater. 31 19
Google Scholar
[8] Sun X, Chi M H, Weng L, Shi J H, Zhang X R 2021 J. Mater. SCI-Mater. El. 32 26548
Google Scholar
[9] Gao F, Zhang X R, Weng L, Cheng Y J, Shi J H 2022 Pigm. Resin Technol. 51 441
Google Scholar
[10] Han Z Q, Qi S L, Liu W, Han L, Wu Z P, Wu D Z 2013 Ind. Eng. Chem. Res. 52 3042
Google Scholar
[11] Wei W C, Chen H Q, Zha J W, Zhang Y Y 2023 Front. Chem. Sci. Eeg. 17 991
Google Scholar
[12] 吕程 2014 博士学位论文 (重庆: 重庆大学)
Lv C 2014 Ph. D. Dissertation (Chongqing: Chongqing University
[13] 张松, 唐超, Chen G, 周渠, 吕程, 李旭 2015 中国科学: 技术科学 45 1167
Google Scholar
Zhang S, Tang C, Chen G, Zhou Q, Lv C, Li X 2015 Sci. Sin. Tech. 45 1167
Google Scholar
[14] Huang M, Han Q B, Lv Y Z, Wang L, Shan B L, Ge Y, Qi B, Li C R 2018 IEEE T. Dielect. El. In. 25 1135
Google Scholar
[15] Zhang Y Y, Xu C Q, WEI W C, Deng Y K, Nie S X, Zha J W 2023 High Volt. 8 599
Google Scholar
[16] Wei W C, Zhang Y Y, Chen H Q, Xu C Q, Zha J W, Nie S X 2023 Mater. Design 233 11
Google Scholar
[17] 王久亮, 刘宽, 秦秀娟, 邵光杰 2004 哈尔滨工业大学学报 02 226
Google Scholar
Wang J L, Liu K, Qin X J, Shao G J 2004 J. Harbin Instit. Tech. 02 226
Google Scholar
[18] 李酽, 李娇, 陈丽丽, 连晓雪, 朱俊武 2018 67 140701
Google Scholar
Li Y, Li J, Chen L L, Lian X X, Zhu J W 2018 Acta Phys. Sin. 67 140701
Google Scholar
[19] 李酽, 张琳彬, 李娇, 连晓雪, 朱俊武 2019 68 070701
Google Scholar
Li Y, Zhang L B, Li J, Lian X X, Zhu J W 2019 Acta Phys. Sin. 68 070701
Google Scholar
[20] Banerjee A, Bose N, Lahiri A 2023 IEEE T. Ind. Appl. 59 479
Google Scholar
[21] Lu J Y, Jiang Q S, Zhang J 2009 21st Chinese Control and Decision Conference Guilin, China, June 17–19, 2009 p4823
[22] Naskar M, Dharmendra H M, Meena K P 2021 5th International Conference on Condition Assessment Techniques in Electrical Systems Kozhikode, India, December 3–5, 2021 p186
[23] Kong Y, Li L B, Fu S Y 2022 J. Mater. Chem. A 10 14451
Google Scholar
[24] Du D Y, Tang C, Tang Y J, Yang L, Hao J 2021 Compos. Struct. 261 6
Google Scholar
[25] Zhang Z X, Zhou H B, Li W T, Tang C 2021 Processes 9 9
Google Scholar
[26] Zhang Y Y, Li Y, Zheng H B, Zhu M Z, Liu J F, Yang T, Zhang C H, Li Y 2020 Cellulose 27 2455
Google Scholar
[27] Mo Y, Yang L J, Yin F, Gao Y Y 2022 Polym. Composite 43 1698
Google Scholar
[28] Inagak T, Siesler H W, Mitsui K, Tsuchikawa S 2010 Biomacromolecules 11 2300
Google Scholar
[29] Mazeau K, Heux L 2003 J. Phys. Chem. B 107 2394
Google Scholar
[30] Wang X B, Tang C, Wang Q, Li X P, Hao J 2017 Energies 10 11
Google Scholar
[31] Bond S D, Leimkuhler B J, Laird B B 1999 J. Comput. Phys. 151 114
Google Scholar
[32] 吕健, 詹怀宇, 晋华春 2008 中国造纸 5 54
Google Scholar
Lv J, Zhan H Y, Jin H C 2008 China Pulp Paper 5 54
Google Scholar
[33] Tanaka F, Okamura K 2005 Cellulose 12 243
Google Scholar
[34] Chang K S, Chung Y C, Yang T H, Lue S J, Tung K L, Lin Y F 2012 J. Membrane Sci. 417 119
Google Scholar
[35] 王有元, 杨涛, 廖瑞金, 张大伟, 刘强, 田苗 2012 高电压技术 38 1199
Wang Y Y, Yang T, Liao R J, Zhang D W, Liu Q, Tian M 2012 High Volt. Eng. 38 1199
[36] Liu X J, Rao Z H 2019 Int. J. Heat. Mass Tran. 132 362
Google Scholar
[37] 王振华, 卢咏来, 张立群 2009 橡胶工业 56 581
Google Scholar
Wang Z H, Lu Y L, Zhang L Q 2009 China Rubber Ind. 56 581
Google Scholar
-
图 1 nano-ZnO和纤维素的复合模型 (a) P0; (b) P2; (c) P4; (d) P6; (e) P8; (f) P10
Fig. 1. Composite model of nano-ZnO and cellulose: (a) P0; (b) P2; (c) P4; (d) P6; (e) P8; (f) P10.
图 2 不同nano-ZnO含量的nano-ZnO/纤维素模型的体积模量、剪切模量和弹性模量
Fig. 2. Volume modulus, shear modulus, and elastic modulus of nano-ZnO/cellulose model with different nano-ZnO content.
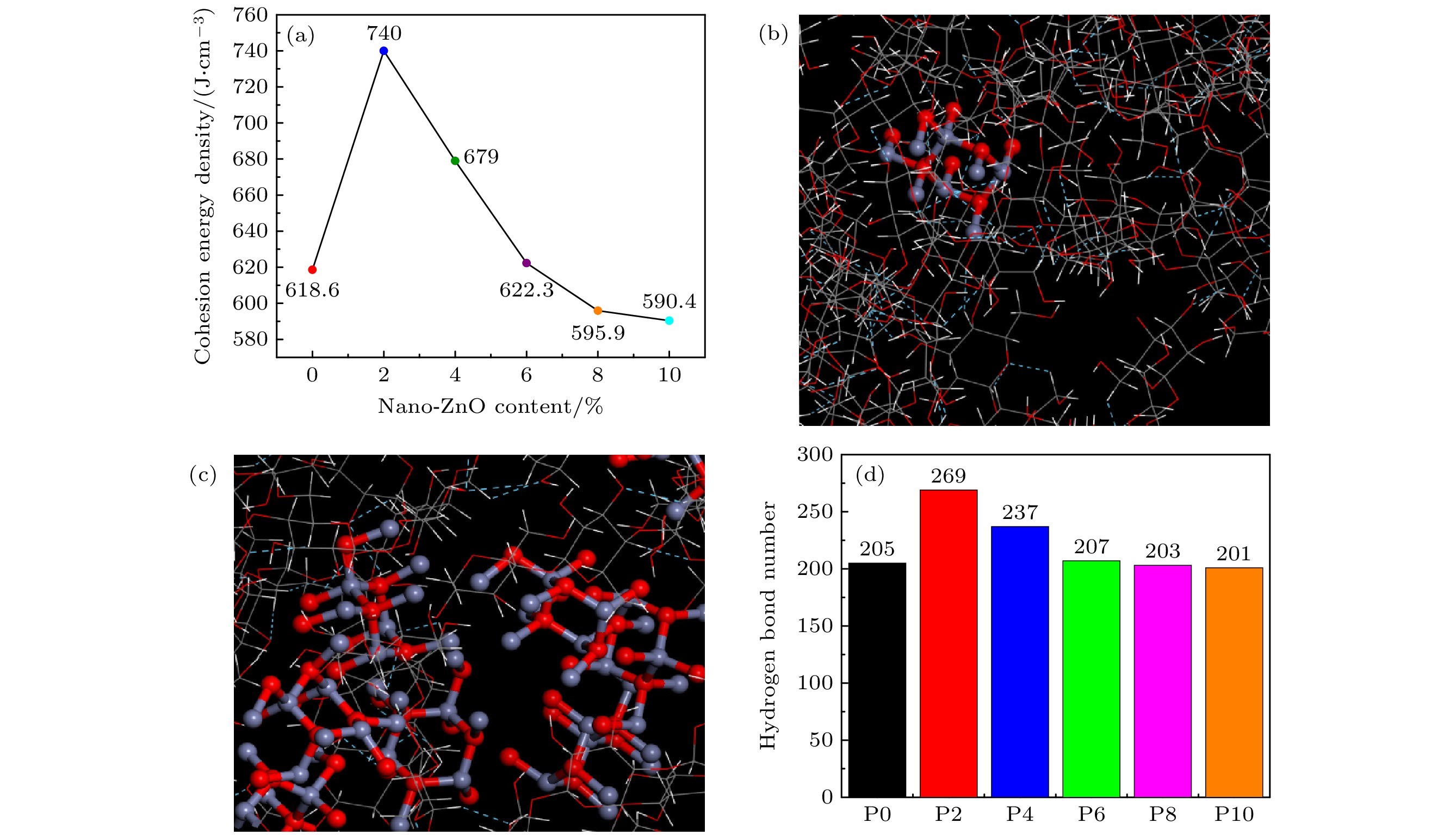
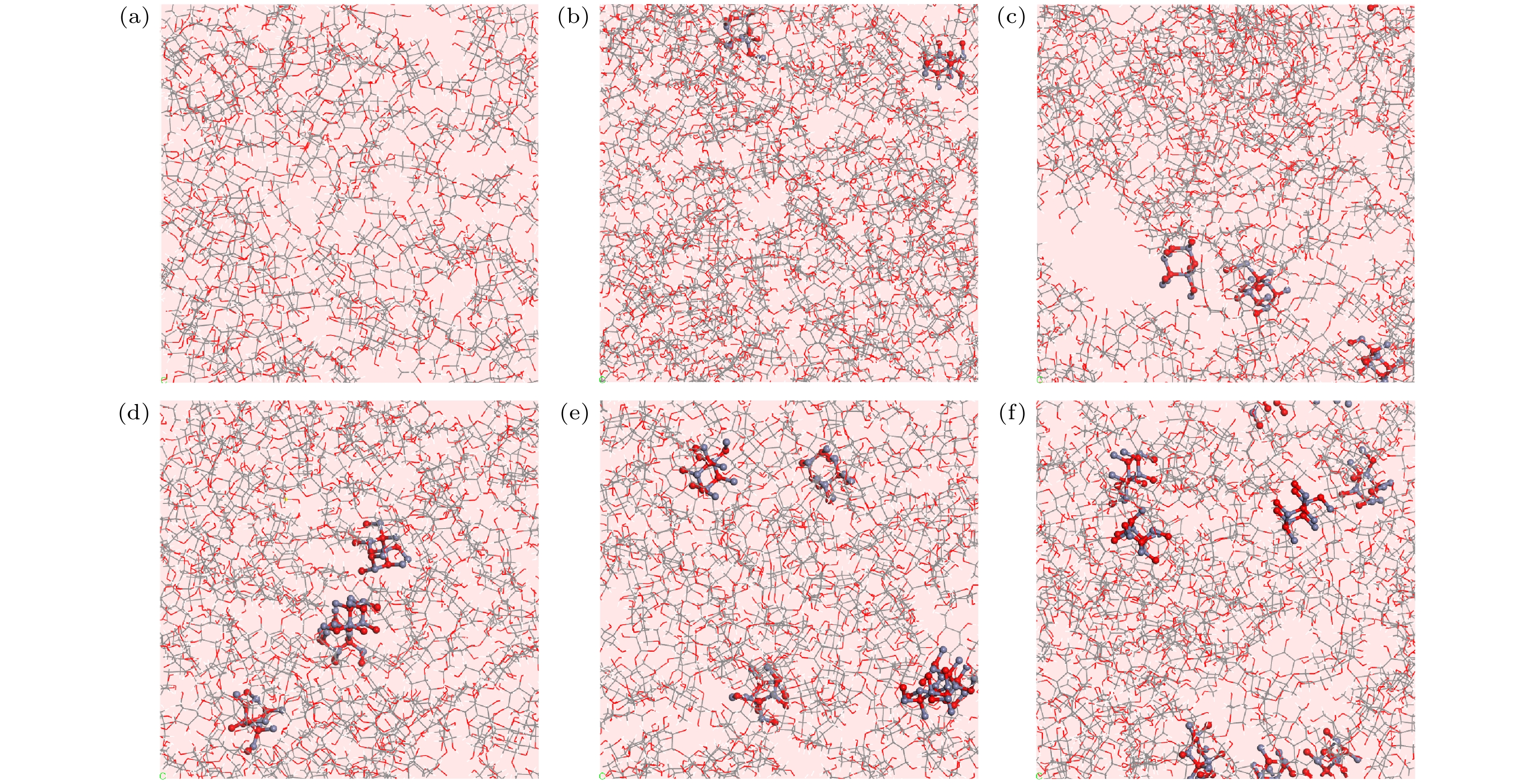
图 3 (a)不同nano-ZnO含量的nano-ZnO/纤维素模型的内聚能密度; (b) Nano-ZnO填充无定形区域空隙时产生的氢键; (c) Nano-ZnO出现团聚现象时产生的氢键; (d) 6种模型的氢键数量
Fig. 3. (a) Cohesion energy density of nano-ZnO/cellulose models with different nano-ZnO contents; (b) hydrogen bonding when Nano-ZnO fills voids in amorphous regions; (c) hydrogen bonds generated when Nano-ZnO is agglomerated; (d) the number of hydrogen bonds in the six models.
图 4 (a) 6种模型的MSD曲线; (b)各模型样品的最大MSD值
Fig. 4. (a) MSD curves for six models; (b) the maximum MSD value of each model sample.
图 5 nano-ZnO/纤维素复合模型的玻璃化转变温度 (a) P0; (b) P2; (c) P4; (d) P6; (e) P8; (f) P10
Fig. 5. Glass transition temperature of nano-ZnO/cellulose models: (a) P0; (b) P2; (c) P4; (d) P6; (e) P8; (f) P10.
图 6 反向非平衡分子动力学(RNEMD)方法模型图
Fig. 6. Model diagram of reverse non-equilibrium molecular dynamics (RNEMD) method.
图 7 不同添加量下的nano-ZnO/纤维素模型的导热系数模拟结果
Fig. 7. Thermal conductivity simulation results of nano-ZnO/cellulose models with different doping ratios.
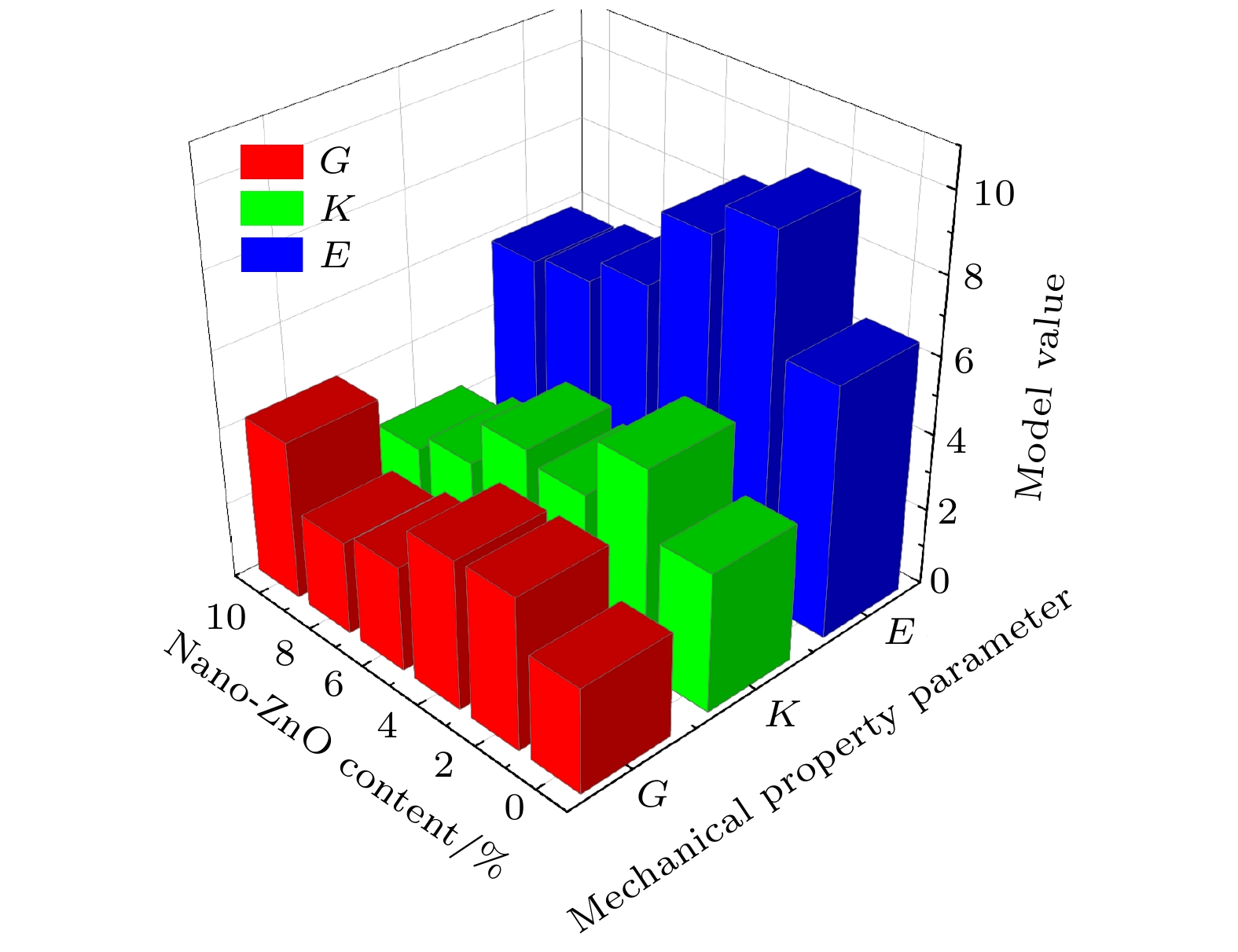
表 1 分子动力学计算纯纤维素绝缘纸和改性纤维素绝缘纸的力学参数
Table 1. Mechanical parameters of pure cellulose insulating paper and modified cellulose insulating paper calculated by molecular dynamics.
模型 λ μ E/GPa G/GPa K/GPa K/G P0 1.7942 2.7210 6.5232 2.7210 3.6082 1.3261 P1 2.6659 3.9442 9.4791 3.9442 5.2954 1.3426 P2 1.1556 3.8840 8.6586 3.8840 3.7449 0.9642 P3 2.2157 2.7362 6.6967 2.7362 4.0398 1.4764 P4 0.5645 2.7983 6.0663 2.4300 2.7983 0.8684 P5 2.6101 2.3187 5.8653 4.1559 2.3187 1.7923 
下载: 导出CSV
-
[1] Shadfar H, Pashakolaei M G, Foroud A A 2021 Int. T. Electr. Energy 31 24
Google Scholar
[2] Chen Q G, Li C P, Cheng S, Sun W, Chi M H, Zhang H 2022 IEEE T. Dielect. El. In. 29 591
Google Scholar
[3] Tang C, Chen R, Zhang J Z, Peng X, Chen B H, Zhang L S 2022 IET Nanodielectrics 5 63
Google Scholar
[4] Yang L, Gao J, Peng X, Qin J S, Tang C 2021 Cellulose 28 6023
Google Scholar
[5] Tang C, Zhang S, Wang X B, Hao J 2018 Cellulose 25 3619
Google Scholar
[6] Chen Q J, Kang M C, Xie Q H, Wang J H 2020 Cellulose 27 7621
Google Scholar
[7] Ji D Y, Li T, Hu W P, Fuchs H 2019 Adv. Mater. 31 19
Google Scholar
[8] Sun X, Chi M H, Weng L, Shi J H, Zhang X R 2021 J. Mater. SCI-Mater. El. 32 26548
Google Scholar
[9] Gao F, Zhang X R, Weng L, Cheng Y J, Shi J H 2022 Pigm. Resin Technol. 51 441
Google Scholar
[10] Han Z Q, Qi S L, Liu W, Han L, Wu Z P, Wu D Z 2013 Ind. Eng. Chem. Res. 52 3042
Google Scholar
[11] Wei W C, Chen H Q, Zha J W, Zhang Y Y 2023 Front. Chem. Sci. Eeg. 17 991
Google Scholar
[12] 吕程 2014 博士学位论文 (重庆: 重庆大学)
Lv C 2014 Ph. D. Dissertation (Chongqing: Chongqing University
[13] 张松, 唐超, Chen G, 周渠, 吕程, 李旭 2015 中国科学: 技术科学 45 1167
Google Scholar
Zhang S, Tang C, Chen G, Zhou Q, Lv C, Li X 2015 Sci. Sin. Tech. 45 1167
Google Scholar
[14] Huang M, Han Q B, Lv Y Z, Wang L, Shan B L, Ge Y, Qi B, Li C R 2018 IEEE T. Dielect. El. In. 25 1135
Google Scholar
[15] Zhang Y Y, Xu C Q, WEI W C, Deng Y K, Nie S X, Zha J W 2023 High Volt. 8 599
Google Scholar
[16] Wei W C, Zhang Y Y, Chen H Q, Xu C Q, Zha J W, Nie S X 2023 Mater. Design 233 11
Google Scholar
[17] 王久亮, 刘宽, 秦秀娟, 邵光杰 2004 哈尔滨工业大学学报 02 226
Google Scholar
Wang J L, Liu K, Qin X J, Shao G J 2004 J. Harbin Instit. Tech. 02 226
Google Scholar
[18] 李酽, 李娇, 陈丽丽, 连晓雪, 朱俊武 2018 67 140701
Google Scholar
Li Y, Li J, Chen L L, Lian X X, Zhu J W 2018 Acta Phys. Sin. 67 140701
Google Scholar
[19] 李酽, 张琳彬, 李娇, 连晓雪, 朱俊武 2019 68 070701
Google Scholar
Li Y, Zhang L B, Li J, Lian X X, Zhu J W 2019 Acta Phys. Sin. 68 070701
Google Scholar
[20] Banerjee A, Bose N, Lahiri A 2023 IEEE T. Ind. Appl. 59 479
Google Scholar
[21] Lu J Y, Jiang Q S, Zhang J 2009 21st Chinese Control and Decision Conference Guilin, China, June 17–19, 2009 p4823
[22] Naskar M, Dharmendra H M, Meena K P 2021 5th International Conference on Condition Assessment Techniques in Electrical Systems Kozhikode, India, December 3–5, 2021 p186
[23] Kong Y, Li L B, Fu S Y 2022 J. Mater. Chem. A 10 14451
Google Scholar
[24] Du D Y, Tang C, Tang Y J, Yang L, Hao J 2021 Compos. Struct. 261 6
Google Scholar
[25] Zhang Z X, Zhou H B, Li W T, Tang C 2021 Processes 9 9
Google Scholar
[26] Zhang Y Y, Li Y, Zheng H B, Zhu M Z, Liu J F, Yang T, Zhang C H, Li Y 2020 Cellulose 27 2455
Google Scholar
[27] Mo Y, Yang L J, Yin F, Gao Y Y 2022 Polym. Composite 43 1698
Google Scholar
[28] Inagak T, Siesler H W, Mitsui K, Tsuchikawa S 2010 Biomacromolecules 11 2300
Google Scholar
[29] Mazeau K, Heux L 2003 J. Phys. Chem. B 107 2394
Google Scholar
[30] Wang X B, Tang C, Wang Q, Li X P, Hao J 2017 Energies 10 11
Google Scholar
[31] Bond S D, Leimkuhler B J, Laird B B 1999 J. Comput. Phys. 151 114
Google Scholar
[32] 吕健, 詹怀宇, 晋华春 2008 中国造纸 5 54
Google Scholar
Lv J, Zhan H Y, Jin H C 2008 China Pulp Paper 5 54
Google Scholar
[33] Tanaka F, Okamura K 2005 Cellulose 12 243
Google Scholar
[34] Chang K S, Chung Y C, Yang T H, Lue S J, Tung K L, Lin Y F 2012 J. Membrane Sci. 417 119
Google Scholar
[35] 王有元, 杨涛, 廖瑞金, 张大伟, 刘强, 田苗 2012 高电压技术 38 1199
Wang Y Y, Yang T, Liao R J, Zhang D W, Liu Q, Tian M 2012 High Volt. Eng. 38 1199
[36] Liu X J, Rao Z H 2019 Int. J. Heat. Mass Tran. 132 362
Google Scholar
[37] 王振华, 卢咏来, 张立群 2009 橡胶工业 56 581
Google Scholar
Wang Z H, Lu Y L, Zhang L Q 2009 China Rubber Ind. 56 581
Google Scholar
下载:
计量
- 文章访问数: 1667
- PDF下载量: 82
- 被引次数: 0
