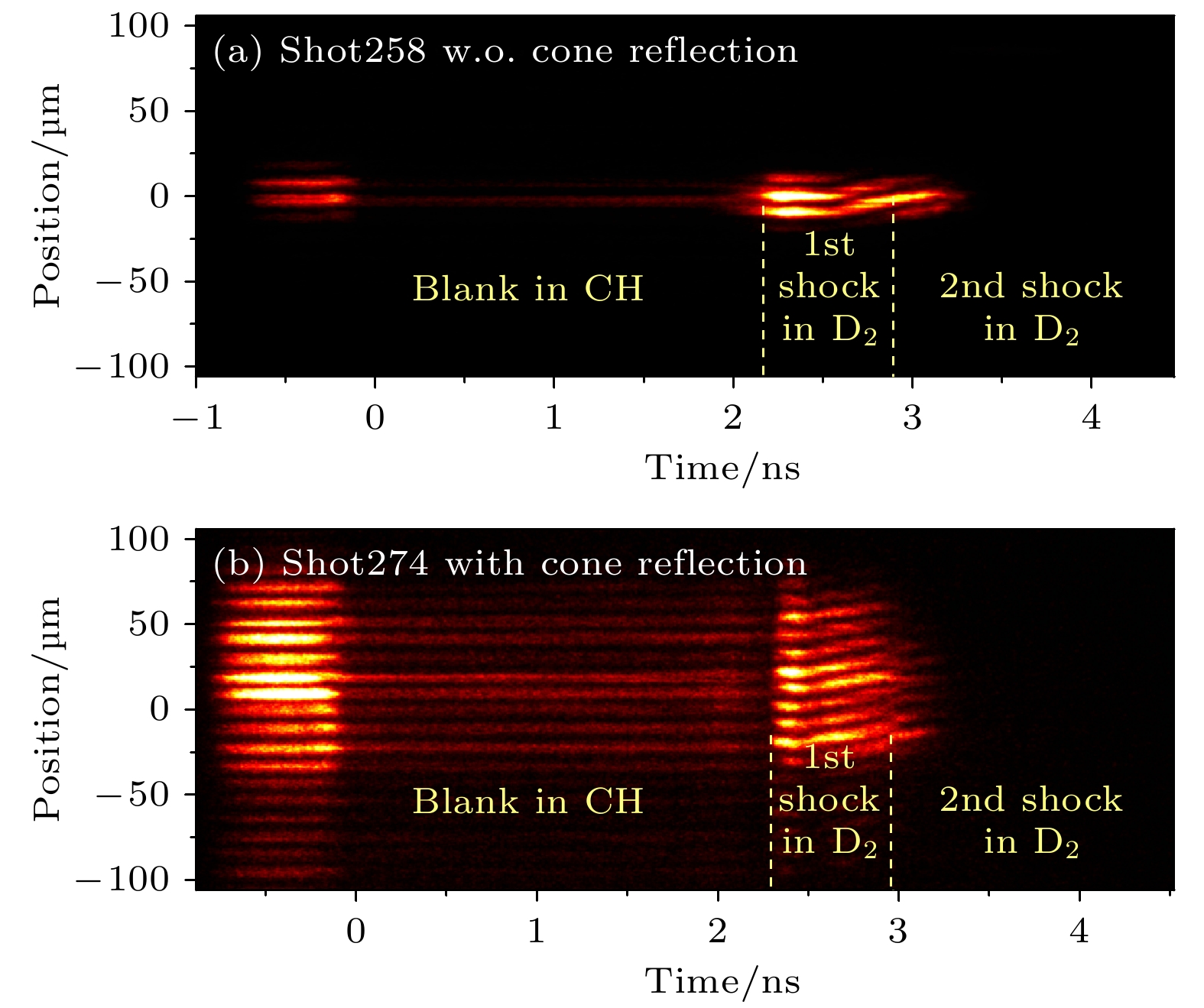
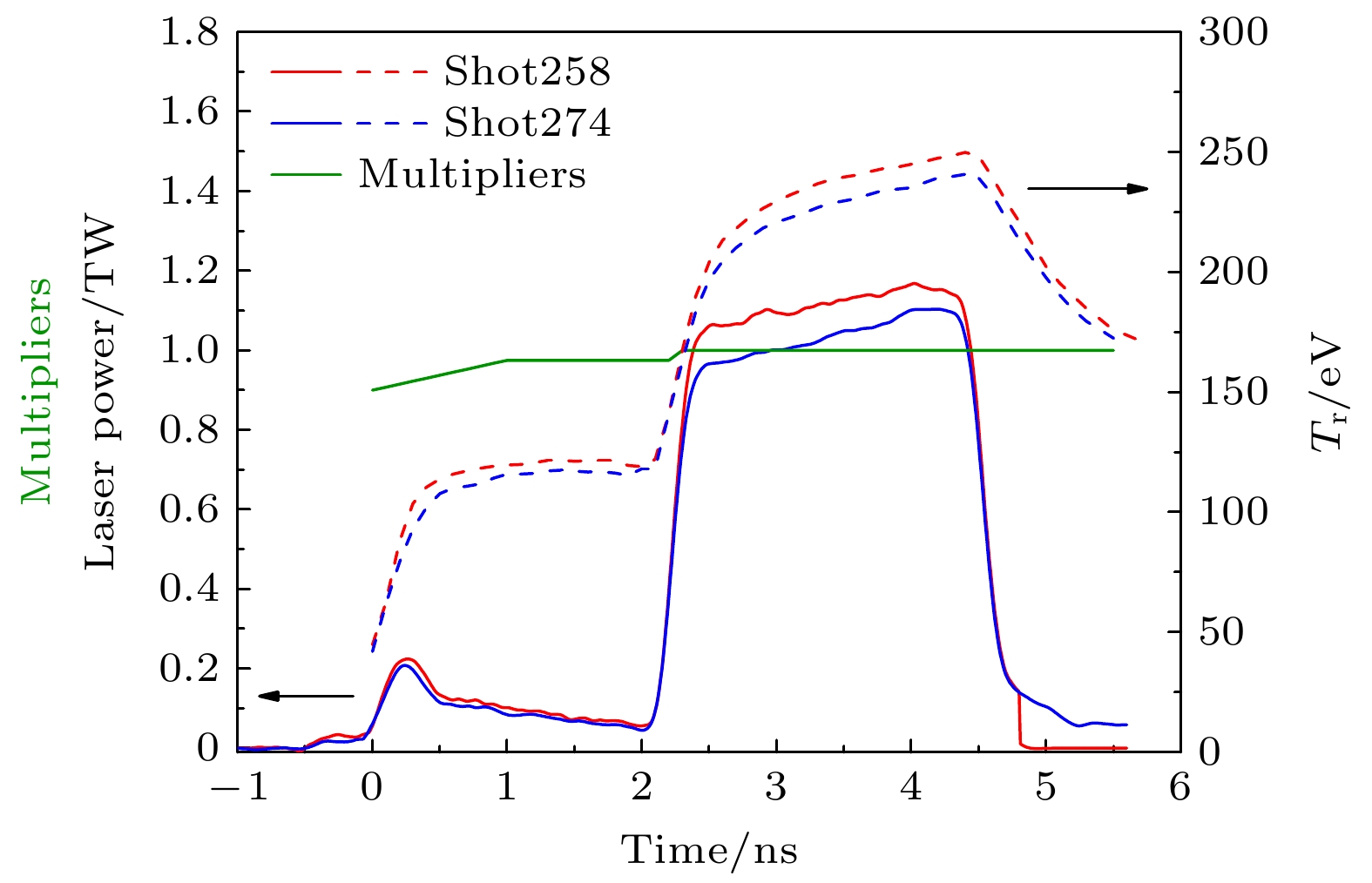
-
激光聚变研究中, 冲击波调控技术是实现靶丸压缩过程的熵增调谐, 保证高性能内爆的关键实验技术. 本文在十万焦耳激光装置上首次实现了0.375 mm半径小尺度内爆靶丸下双台阶辐射驱动的高精度冲击波调控实验测量. 针对小靶丸下任意反射面速度干涉仪(VISAR)诊断有效反射区域不足的问题, 通过建立的球形反射面VISAR图像光强的理论计算方法, 提出了利用锁孔(keyhole)锥反射效应提升VISAR诊断空间区域的实验技术路线, 使得小靶丸尺度下有效 VISAR 数据区域提升了近3倍. 在实验中首次获得了整形内爆实验条件下低温液氘靶的冲击波测量实验数据, 实现了高精度冲击波调控实验测量. 实验发现, 小时空尺度内爆设计条件下, 由于反射冲击波的作用, 激光参数的较小偏差都会对冲击波追赶后的传输行为产生显著影响, 揭示了我国当前小靶丸尺度下高性能整形内爆物理过程中冲击波传输的多因素敏感性, 以及冲击波调控实验对于内爆设计验证的重要性. 本文提出的小靶丸冲击波调控实验技术, 不仅为我国十万焦耳激光装置上整形脉冲下熵增调谐实验的开展提供了技术基础, 也对基于球汇聚压缩的超高压物理研究具有重要意义.
-
关键词:
- 惯性约束聚变 /
- 冲击波调控 /
- 整形激光 /
- 任意反射面速度干涉仪
In laser fusion research, the precision of shock-timing technology is pivotal for attaining optimal adiabatic tuning during the compression phase of fusion capsules, which is crucial for ensuring the high-performance implosion. The current main technological approach for shock-timing experiments is to use keyhole targets and VISAR (velocity interferometer system for any reflector) diagnostics to measure the shock velocity history. Nonetheless, this approach encounters limitations when scaling down to smaller capsules, primarily due to the reduced effective reflection area available for VISAR diagnostics. In this work, a novel high-precision shock-timing experimental methodology is used to realize a double-step radiation-driven implosion of a 0.375 mm radius capsule on a 100 kJ laser facility. By calculating the intensity of VISAR images with spherical reflective surfaces, a new experimental technical route is proposed, i.e. using the keyhole cone reflection effect to enhance the VISAR diagnostic spatial area, which can effectively increase the effective data collection region by nearly threefold for small-scale capsules. The technique has been adeptly used to measure shock waves in cryogenic liquid-deuterium-filled capsules under shaped implosion experimental conditions, thus obtaining high-precision shock-timing experimental data. The experimental data reveal that the application of this technology can markedly enhance both the image quality and the precision of data analysis for shock wave velocity measurements in small-scale capsules. Furthermore, it is discovered that under similar laser conditions, there exist considerable variations in the shock velocity profiles. Simulation analysis shows that the difference in chasing behavior of the “N+1” reflected shock wave caused by small changes in laser intensity is the main reason for the significant difference in merging speed. It is demonstrated that small changes in laser parameters can significantly affect the transmission behavior of the shock wave. This experiment highlights the complex sensitivity of shock wave transmission in high-performance forming implosion physics process on a current small capsule scale, making it essential to conduct shock-timing experiments to accurately adjust actual shock wave behavior. This research not only lays a robust technical foundation for promoting adiabatic tuning experiments ofour 100 kJ laser facility but also has profound significance for the ultra-high pressure physics research based on the spherical convergence effect.-
Keywords:
- inertial confinement fusion /
- shock-timing /
- shaping laser /
- velocity interferometer system for any reflector
[1] Lindl J 1995 Phys. Plasmas 2 3933
Google Scholar
[2] Lindl J D, Amendt P, Berger R L, Gail Glendinning S, Glenzer S H, Haan S W, Kauffman R L, Landen O L, Suter L J 2004 Phys. Plasmas 11 339
Google Scholar
[3] Atzeni S, Meyer-ter-vehn J 著 (沈百飞 译) 2008 惯性聚变物理 (北京: 科学出版社) 第41页
Atzeni S, Meyer-ter-vehn J (translated by Sheng B F) 2008 The Physics of Inertial Fusion (Beijing: Science Press) p41
[4] Robey H F, MacGowan B J, Landen O L, LaFortune K N, Widmayer C, Celliers P M, Moody J D, Ross J S, Ralph J, LePape S, Berzak Hopkins L F, Spears B K, Haan S W, Clark D, Lindl J D, Edwards M J 2013 Phys. Plasmas 20 052707
Google Scholar
[5] Dewald E L, Rosen M, Glenzer S H, Suter L J, Girard F, Jadaud J P, Schein J, Constantin C, Wagon F, Huser G, Neumayer P, Landen O L 2008 Phys. Plasmas 15 072706
Google Scholar
[6] Haan S W, Pollaine S M, Lindl J D, Suter L J, Berger R L, Powers L V, Alley W E, Amendt P A, Futterman J A, Levedahl W K, Rosen M D, Rowley D P, Sacks R A, Shestakov A I, Strobel G L, Tabak M, Weber S V, Zimmerman G B 1995 Phys. Plasmas 2 2480
Google Scholar
[7] Hu S X, Goncharov V N, Boehly T R, McCrory R L, Skupsky S, Collins L A, Kress J D, Militzer B 2015 Phys. Plasmas 22 056304
Google Scholar
[8] Boehly T R, Munro D, Celliers P M, Olson R E, Hicks D G, Goncharov V N, Collins G W, Robey H F, Hu S X, Marozas J A, Sangster J C, Landen O L, Meyerhofer D D 2009 Phys. Plasmas 16 056302
Google Scholar
[9] Celliers P M, Bradley D K, Collins G W, Hicks D G, Boehly T R, Armstrong W J 2004 Rev. Sci. Instrum. 75 4916
Google Scholar
[10] Boehly T R, Goncharov V N, Seka W, Barrios M A, Celliers P M, Hicks D G, Collins G W, Hu S X, Marozas J A, Meyerhofer D D 2011 Phys. Rev. Lett. 106 195005
Google Scholar
[11] Robey H F, Boehly T R, Celliers P M, et al. 2012 Phys. Plasmas 19 042706
Google Scholar
[12] Robey H F, Muncro D H, Spears B K, Marinak M M, Jones O S, Patel M V, Haan S W, Salmonson J D, Landen O L, Boehly T R, Nikroo A 2008 J. Phys. Conf. Ser. 112 022078
Google Scholar
[13] Robey H F, Celliers P M, Moody J D, Sater J, Parham T, Kozioziemski B, Dylla-Spears R, Ross J S, LePape S, Ralph J E, Hohenberger M, Dewald E L, Berzak Hopkins L, Kroll J J, Yoxall B E, Hamza A V, Boehly T R, Nikroo A, Landen O L, Edwards M J 2014 Phys. Plasmas 21 022703
Google Scholar
[14] Robey H F, Celliers P M, Kline J L, et al. 2012 Phys. Rev. Lett. 108 215004
Google Scholar
[15] Zheng W G, Wei X F, Zhu Q H, Jing F, Hu D X, Yuan X D, Dai W J, Zhou W, Wang F, Xu D P, Xie X D, Feng B, Peng Z T, Guo L F, Chen Y B, Zhang X J, Liu L Q, Lin D H, Dang Z, Xiang Y, Zhang R, Wang F, Jia H T, Deng X W 2017 Matter Radiat. Extremes 2 243
Google Scholar
[16] 晏骥, 张兴, 郑建华, 袁永腾, 康洞国, 葛峰骏, 陈黎, 宋仔峰, 袁铮, 蒋炜, 余波, 陈伯伦, 蒲昱东, 黄天晅 2015 64 125203
Google Scholar
Yan J, Zhang X, Zheng J H, Yuan Y T, Kang D G, Ge F J, Li C, Song Z F, Yuan Z, Jiang W, Yu B, Chen B L, Pu Y D, Huang T X 2015 Acta Phys. Sin. 64 125203
Google Scholar
[17] 蒲昱东, 康洞国, 黄天晅, 高耀明, 陈家斌, 唐琦, 宋仔峰, 彭晓世, 陈伯伦, 蒋炜, 余波, 晏骥, 江少恩, 刘慎业, 杨家敏, 丁永坤 2014 63 125211
Google Scholar
Pu Y D, Kang D G, Huang T X, Gao Y M, Chen J B, Tang Q, Song Z F, Peng X S, Chen B L, Jiang W, Yu B, Yan J, Jiang S E, Liu S Y, Yang J M, Ding Y K 2014 Acta Phys. Sin. 63 125211
Google Scholar
[18] 黄天晅, 吴畅书, 陈忠靖, 晏骥, 李欣, 葛峰峻, 张兴, 蒋炜, 邓博, 侯立飞, 蒲昱东, 董云松, 王立锋 2023 72 025201
Google Scholar
Huang T X, Wu C S, Chen Z J, Yan J, Li X, Ge F J, Zhang X, Jiang W, Deng B, Hou L F, Pu Y D, Dong Y S, Wang L F 2023 Acta Phys. Sin. 72 025201
Google Scholar
[19] Ge F J, Pu Y D, Wang K, Huang T X, Sun C K, Qi X B, Wu C S, Gu J F, Chen Z J, Yan J, Jiang W, Yang D, Dong Y S, Wang F, Zhou S Y, Ding Y K 2023 Nucl. Fusion 63 086033
Google Scholar
[20] Philpott M K, George A, Whiteman G, De’Ath J, Millett J C F 2015 Meas. Sci. Technol. 26 125204
Google Scholar
[21] Barker L M 1998 AIP Conf. Proc. 429 833
Google Scholar
[22] Li Z C, Jiang X H, Liu S Y, Huang T X, Zheng J, Yang J M, Li S W, Guo L, Zhao X F, Du H B, Song T M, Yi R Q, Liu Y G, Jiang S E, Ding Y K 2010 Rev. Sci. Instrum. 81 073504
Google Scholar
[23] Li Z C, Zhu X L, Jiang X H, Liu S Y, Zheng J, Li S W, Wang Z B, Yang D, Zhang H, Guo L, Xin J, Song T M, Ding Y K 2011 Rev. Sci. Instrum. 82 106106
Google Scholar
[24] Theobald W, Miller J E, Boehly T R, Vianello E, MeyerhoferD D, Sangster T C 2006 Phys. Plasmas 13 122702
Google Scholar
[25] Celliers P M, Collins G W, Da Silva L B, Cauble R, Gold D M, Foord M E, Holmes N C, Hammel B A, Wallace R J, Ng A 2000 Phys. Rev. Lett. 84 5564
Google Scholar
[26] Zaghoo M, Boehly T R, Rygg J R, Celliers P M, Hu S X, Collins G W 2019 Phys. Rev. Lett. 122 085001
Google Scholar
[27] Erskine D, Eggert J, Celliers P, Hicks D 2017 AIP Conf. Proc. 1793 160016
Google Scholar
[28] Ramis R, Schmalz R and Meyer-Ter-Vehn J 1988 Comput. Phys. Commun. 49 475
Google Scholar
[29] Eidmann K 1994 Laser Part. Beams 12 223
Google Scholar
[30] Landen O L, Caseya D T, DiNicola J M, et al. 2020 High Energy Density Phys. 36 100755
Google Scholar
-
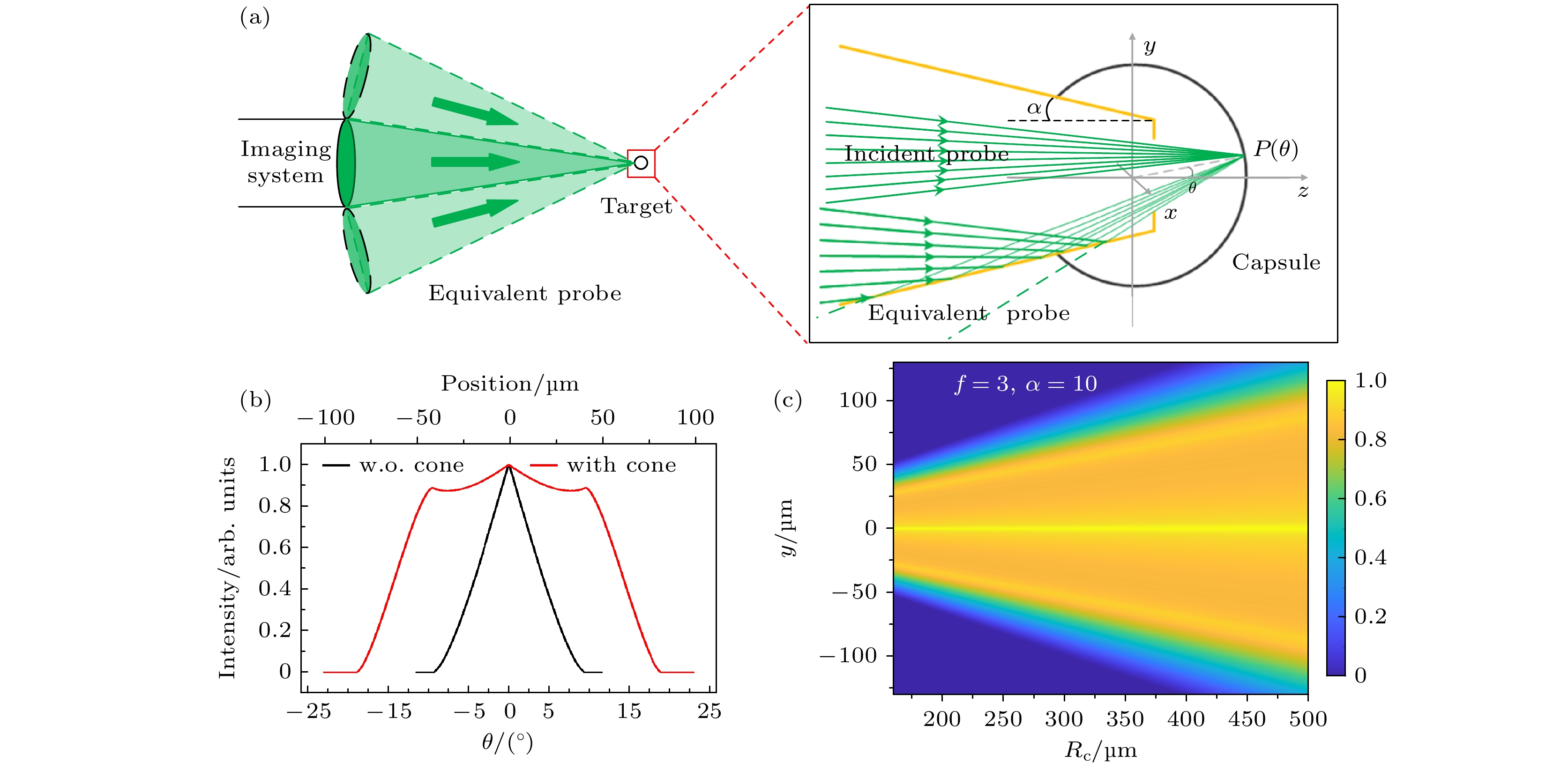
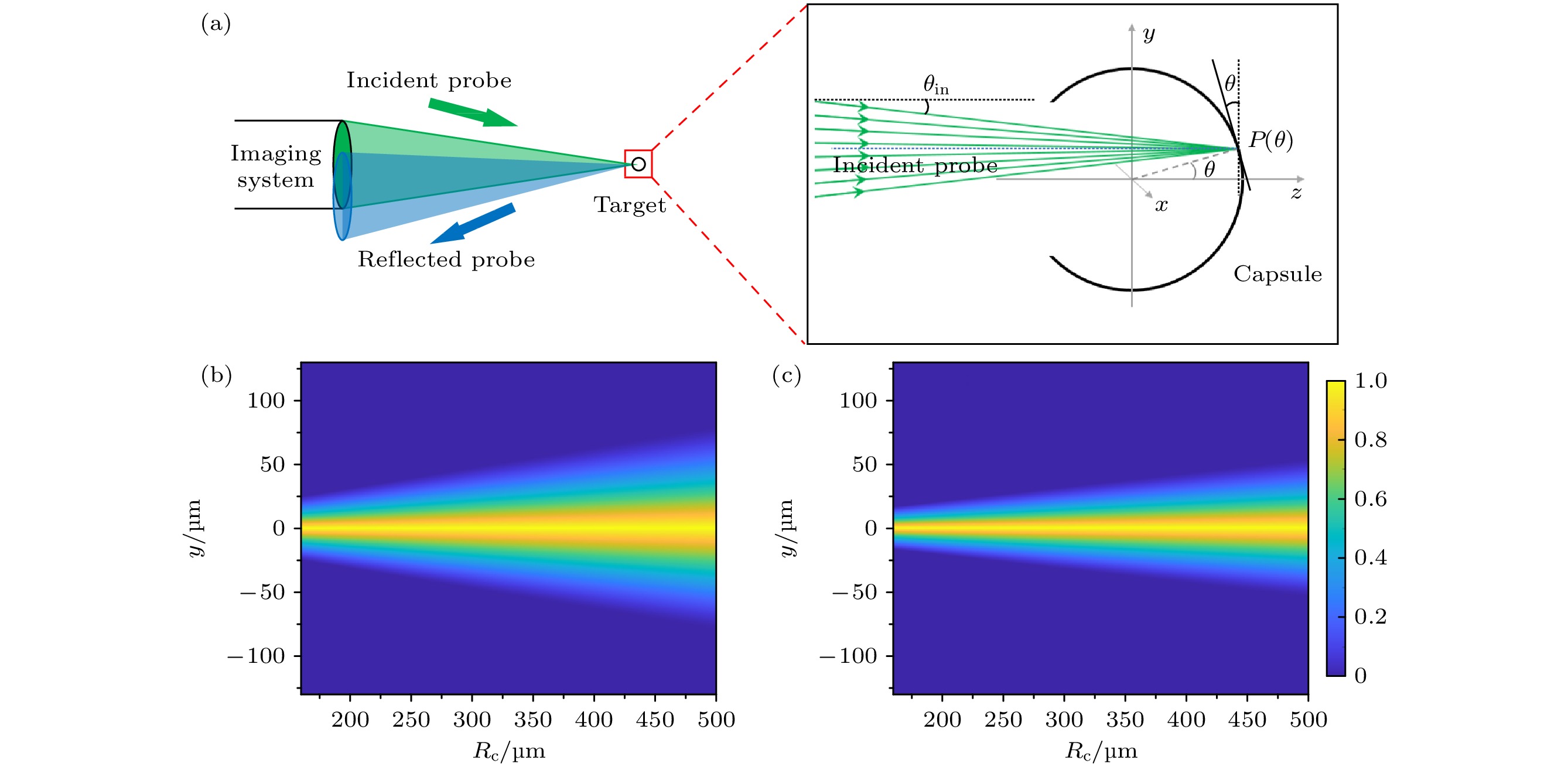
图 1 (a) 球形反射面下VISAR诊断光路入射和收光示意图; (b) 成像系统F数为3时不同半径球形反射面的VISAR数据测量空间方向的光强分布; (c) 成像系统F数为4.5时不同半径球形反射面的VISAR数据测量空间方向的光强分布
Fig. 1. (a) Diagnostic diagram of VISAR for spherical reflector; (b) spatial intensity distribution of VISAR data for spherical reflectors with different radii under the f/3 of imaging system; (c) spatial intensity distribution of VISAR data for spherical reflectors with different radii under the f/4.5 of imaging system.
图 2 (a) 利用keyhole锥壁反射效应时球形反射面的VISAR诊断示意图; (b) 有10°锥角/无锥角时VISAR光强随倾角的变化; (c) 成像系统F数为3、半锥角为10°下不同半径球形反射面的VISAR数据测量空间方向的光强分布
Fig. 2. (a) Diagnostic diagram of VISAR for spherical reflector with cone reflection; (b) comparison of the relationship between VISAR data intensity and inclination angle with 10° cone angle or without cone angle; (c) spatial intensity distribution of VISAR data for spherical reflectors with different radii under the f/3 of imaging system and 10° cone angle.
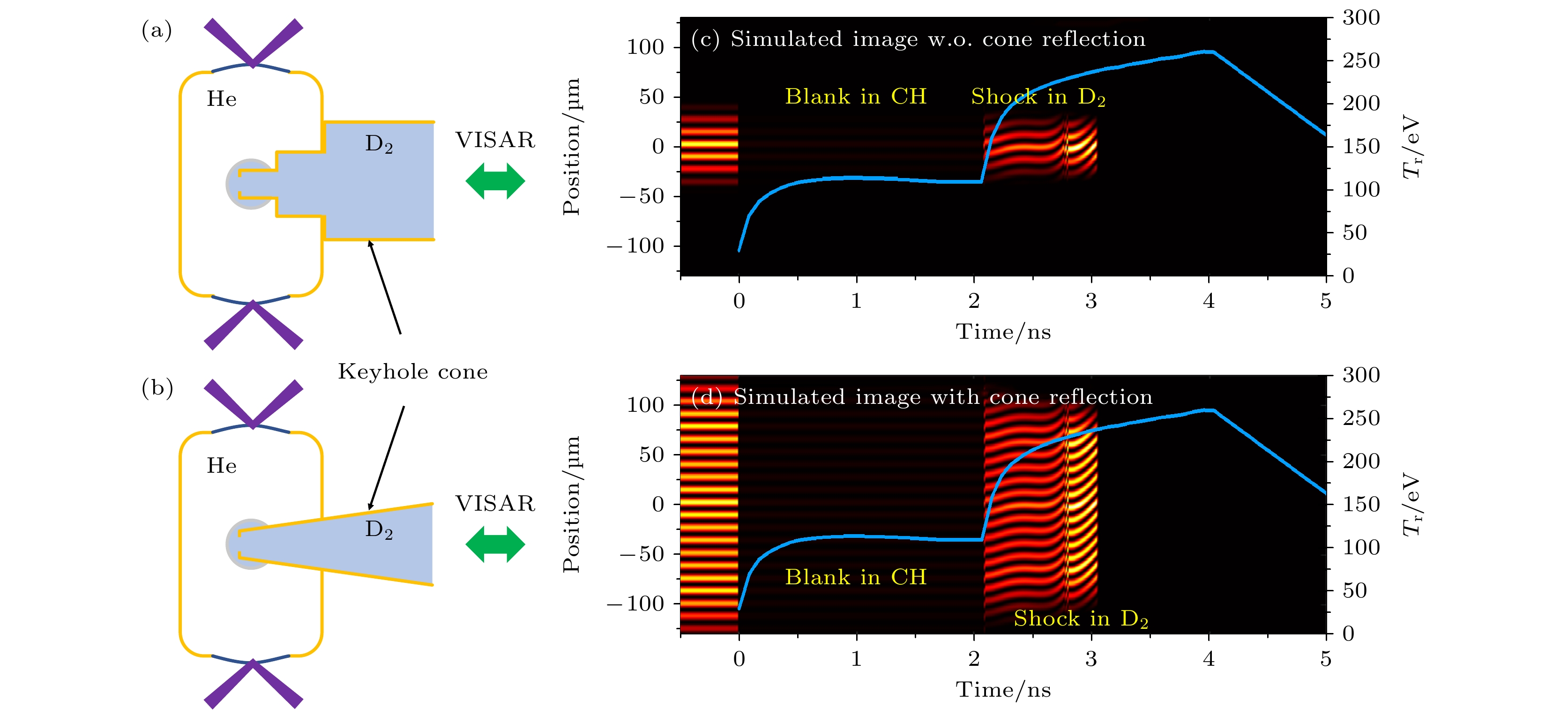
图 3 (a) 无锥角和(b) 10°锥角keyhole靶冲击波调控实验原理示意图; (c) 无锥角和(d) 10°锥角keyhole靶冲击波调控实验设计辐射温度波形(蓝色实线)、VISAR仿真图像
Fig. 3. Schematic diagram of shock-timing experiment under (a) non-cone angle and (b) 10° cone angle keyhole target; design of laser waveform (blue solid line) and VISAR simulation image of shock-timing experiment under (c) non-cone angle and (d) 10° cone angle keyhole target.
图 4 不同keyhole锥角的冲击波调控实验获得的VISAR原始图像
Fig. 4. VISAR raw images obtained from shock-timing experiments with different cone angles of keyhole target.
图 6 实验实际注入黑腔的单束激光平均波形及测量的辐射温度
Fig. 6. Average waveform of single laser beam injected into hohlraum and measured radiation temperature.
图 7 258发次冲击波传输轨迹的实验后模拟
Fig. 7. Simulation of shock propagation trajectory of shot 258.
图 8 274发次冲击波传输轨迹的实验后模拟
Fig. 8. Simulation of shock propagation trajectory of shot 274.
-
[1] Lindl J 1995 Phys. Plasmas 2 3933
Google Scholar
[2] Lindl J D, Amendt P, Berger R L, Gail Glendinning S, Glenzer S H, Haan S W, Kauffman R L, Landen O L, Suter L J 2004 Phys. Plasmas 11 339
Google Scholar
[3] Atzeni S, Meyer-ter-vehn J 著 (沈百飞 译) 2008 惯性聚变物理 (北京: 科学出版社) 第41页
Atzeni S, Meyer-ter-vehn J (translated by Sheng B F) 2008 The Physics of Inertial Fusion (Beijing: Science Press) p41
[4] Robey H F, MacGowan B J, Landen O L, LaFortune K N, Widmayer C, Celliers P M, Moody J D, Ross J S, Ralph J, LePape S, Berzak Hopkins L F, Spears B K, Haan S W, Clark D, Lindl J D, Edwards M J 2013 Phys. Plasmas 20 052707
Google Scholar
[5] Dewald E L, Rosen M, Glenzer S H, Suter L J, Girard F, Jadaud J P, Schein J, Constantin C, Wagon F, Huser G, Neumayer P, Landen O L 2008 Phys. Plasmas 15 072706
Google Scholar
[6] Haan S W, Pollaine S M, Lindl J D, Suter L J, Berger R L, Powers L V, Alley W E, Amendt P A, Futterman J A, Levedahl W K, Rosen M D, Rowley D P, Sacks R A, Shestakov A I, Strobel G L, Tabak M, Weber S V, Zimmerman G B 1995 Phys. Plasmas 2 2480
Google Scholar
[7] Hu S X, Goncharov V N, Boehly T R, McCrory R L, Skupsky S, Collins L A, Kress J D, Militzer B 2015 Phys. Plasmas 22 056304
Google Scholar
[8] Boehly T R, Munro D, Celliers P M, Olson R E, Hicks D G, Goncharov V N, Collins G W, Robey H F, Hu S X, Marozas J A, Sangster J C, Landen O L, Meyerhofer D D 2009 Phys. Plasmas 16 056302
Google Scholar
[9] Celliers P M, Bradley D K, Collins G W, Hicks D G, Boehly T R, Armstrong W J 2004 Rev. Sci. Instrum. 75 4916
Google Scholar
[10] Boehly T R, Goncharov V N, Seka W, Barrios M A, Celliers P M, Hicks D G, Collins G W, Hu S X, Marozas J A, Meyerhofer D D 2011 Phys. Rev. Lett. 106 195005
Google Scholar
[11] Robey H F, Boehly T R, Celliers P M, et al. 2012 Phys. Plasmas 19 042706
Google Scholar
[12] Robey H F, Muncro D H, Spears B K, Marinak M M, Jones O S, Patel M V, Haan S W, Salmonson J D, Landen O L, Boehly T R, Nikroo A 2008 J. Phys. Conf. Ser. 112 022078
Google Scholar
[13] Robey H F, Celliers P M, Moody J D, Sater J, Parham T, Kozioziemski B, Dylla-Spears R, Ross J S, LePape S, Ralph J E, Hohenberger M, Dewald E L, Berzak Hopkins L, Kroll J J, Yoxall B E, Hamza A V, Boehly T R, Nikroo A, Landen O L, Edwards M J 2014 Phys. Plasmas 21 022703
Google Scholar
[14] Robey H F, Celliers P M, Kline J L, et al. 2012 Phys. Rev. Lett. 108 215004
Google Scholar
[15] Zheng W G, Wei X F, Zhu Q H, Jing F, Hu D X, Yuan X D, Dai W J, Zhou W, Wang F, Xu D P, Xie X D, Feng B, Peng Z T, Guo L F, Chen Y B, Zhang X J, Liu L Q, Lin D H, Dang Z, Xiang Y, Zhang R, Wang F, Jia H T, Deng X W 2017 Matter Radiat. Extremes 2 243
Google Scholar
[16] 晏骥, 张兴, 郑建华, 袁永腾, 康洞国, 葛峰骏, 陈黎, 宋仔峰, 袁铮, 蒋炜, 余波, 陈伯伦, 蒲昱东, 黄天晅 2015 64 125203
Google Scholar
Yan J, Zhang X, Zheng J H, Yuan Y T, Kang D G, Ge F J, Li C, Song Z F, Yuan Z, Jiang W, Yu B, Chen B L, Pu Y D, Huang T X 2015 Acta Phys. Sin. 64 125203
Google Scholar
[17] 蒲昱东, 康洞国, 黄天晅, 高耀明, 陈家斌, 唐琦, 宋仔峰, 彭晓世, 陈伯伦, 蒋炜, 余波, 晏骥, 江少恩, 刘慎业, 杨家敏, 丁永坤 2014 63 125211
Google Scholar
Pu Y D, Kang D G, Huang T X, Gao Y M, Chen J B, Tang Q, Song Z F, Peng X S, Chen B L, Jiang W, Yu B, Yan J, Jiang S E, Liu S Y, Yang J M, Ding Y K 2014 Acta Phys. Sin. 63 125211
Google Scholar
[18] 黄天晅, 吴畅书, 陈忠靖, 晏骥, 李欣, 葛峰峻, 张兴, 蒋炜, 邓博, 侯立飞, 蒲昱东, 董云松, 王立锋 2023 72 025201
Google Scholar
Huang T X, Wu C S, Chen Z J, Yan J, Li X, Ge F J, Zhang X, Jiang W, Deng B, Hou L F, Pu Y D, Dong Y S, Wang L F 2023 Acta Phys. Sin. 72 025201
Google Scholar
[19] Ge F J, Pu Y D, Wang K, Huang T X, Sun C K, Qi X B, Wu C S, Gu J F, Chen Z J, Yan J, Jiang W, Yang D, Dong Y S, Wang F, Zhou S Y, Ding Y K 2023 Nucl. Fusion 63 086033
Google Scholar
[20] Philpott M K, George A, Whiteman G, De’Ath J, Millett J C F 2015 Meas. Sci. Technol. 26 125204
Google Scholar
[21] Barker L M 1998 AIP Conf. Proc. 429 833
Google Scholar
[22] Li Z C, Jiang X H, Liu S Y, Huang T X, Zheng J, Yang J M, Li S W, Guo L, Zhao X F, Du H B, Song T M, Yi R Q, Liu Y G, Jiang S E, Ding Y K 2010 Rev. Sci. Instrum. 81 073504
Google Scholar
[23] Li Z C, Zhu X L, Jiang X H, Liu S Y, Zheng J, Li S W, Wang Z B, Yang D, Zhang H, Guo L, Xin J, Song T M, Ding Y K 2011 Rev. Sci. Instrum. 82 106106
Google Scholar
[24] Theobald W, Miller J E, Boehly T R, Vianello E, MeyerhoferD D, Sangster T C 2006 Phys. Plasmas 13 122702
Google Scholar
[25] Celliers P M, Collins G W, Da Silva L B, Cauble R, Gold D M, Foord M E, Holmes N C, Hammel B A, Wallace R J, Ng A 2000 Phys. Rev. Lett. 84 5564
Google Scholar
[26] Zaghoo M, Boehly T R, Rygg J R, Celliers P M, Hu S X, Collins G W 2019 Phys. Rev. Lett. 122 085001
Google Scholar
[27] Erskine D, Eggert J, Celliers P, Hicks D 2017 AIP Conf. Proc. 1793 160016
Google Scholar
[28] Ramis R, Schmalz R and Meyer-Ter-Vehn J 1988 Comput. Phys. Commun. 49 475
Google Scholar
[29] Eidmann K 1994 Laser Part. Beams 12 223
Google Scholar
[30] Landen O L, Caseya D T, DiNicola J M, et al. 2020 High Energy Density Phys. 36 100755
Google Scholar
下载:
计量
- 文章访问数: 1611
- PDF下载量: 47
- 被引次数: 0
