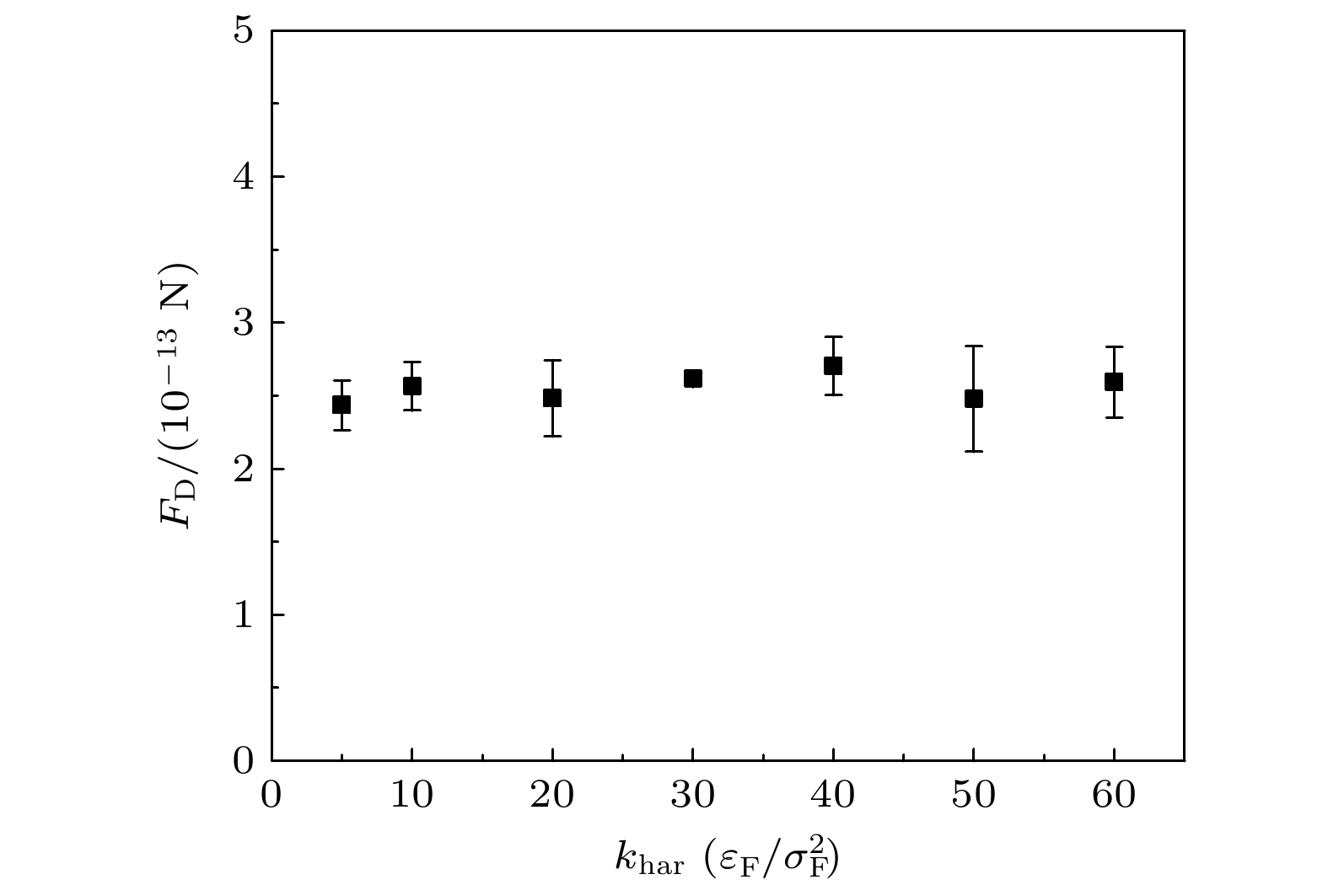
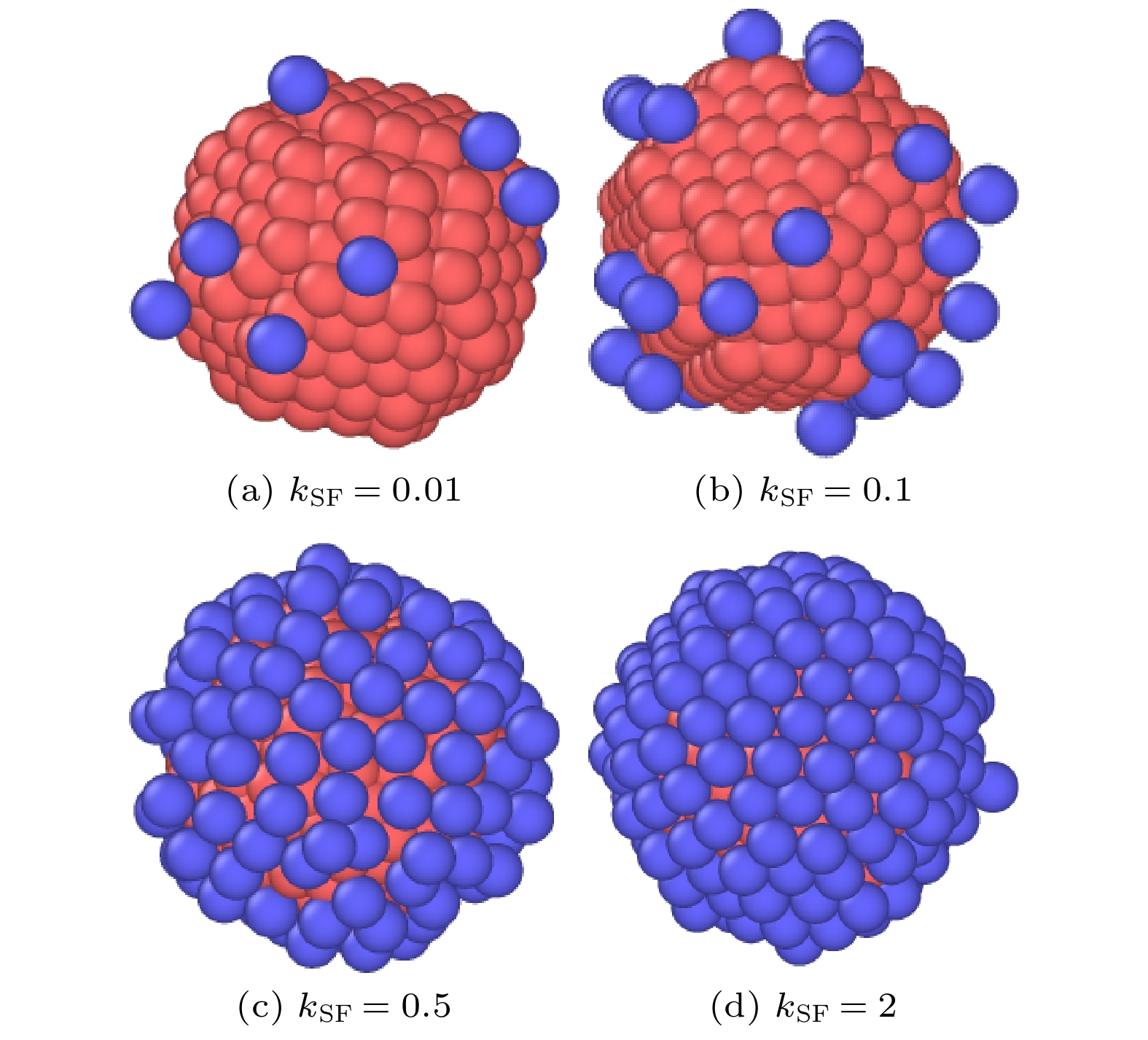
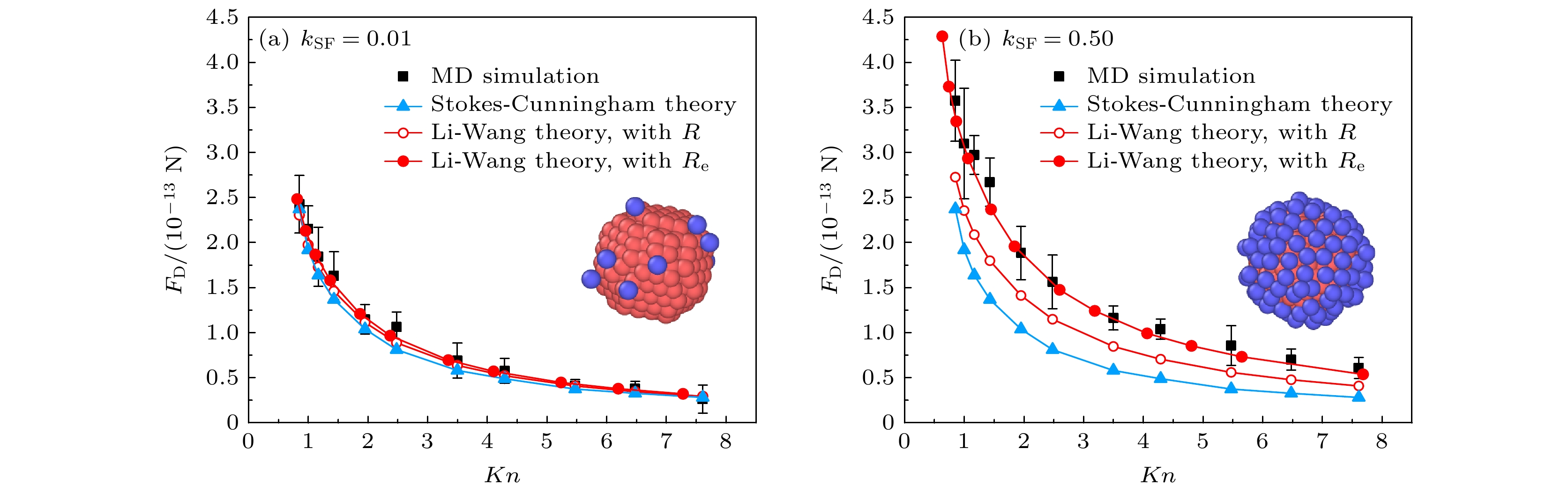
-
在过渡区内, 关于纳米颗粒曳力计算及输运特性的研究较为困难, 通常会采用一些近似方法, 将自由分子区或者连续介质区的理论计算式进行修正, 以适用于过渡区, 但是其准确性值得商榷. 本文基于分子动力学模拟方法, 研究了过渡区内纳米颗粒的曳力特性, 并与相关理论进行对比. 结果表明, 气-固分子间相互作用对纳米颗粒的曳力具有显著影响. 当气固结合强度较弱时, 理论计算结果与分子动力学模拟值吻合较好; 当气固结合强度较强时, 分子动力学模拟结果明显大于理论值, 这是由于气体分子在纳米颗粒表面的吸附所导致. 基于气体分子在颗粒表面的吸附特性, 提出引入有效颗粒半径修正, 其过渡区内曳力的理论计算结果与分子动力学模拟结果吻合较好.Transport properties of nanoparticles in gases have many practical applications, such as aerosol science, combustion, and micro- and nano-scale fabrication. A nanoparticle moving in a fluid is expected to experience a drag force, which determines the transport property of the particle. According to the Einstein relationship, the diffusion coefficient of a particle is inversely proportional to the drag force coefficient. However, in the transition regime, it is usually difficult to evaluate the drag force of suspended particles. A typical method is to extend the asymptotic solution of the free molecular or continuum limit to the transition regime. According to the gas kinetic theory, Li and Wang proposed a theoretical expression for drag force on nanoparticles in the free molecular regime, which is then extended to the entire range of Knudsen number following a semi-empirical approach [Li Z G, Wang H 2003 Phys. Rev. E 68 061207]. For nanoparticles, it is necessary to verify the theoretical predictions since the gas-particle non-rigid-body interactions must be taken into account. In this work, the drag force on nanoparticle in the transition regime is investigated by using molecular dynamics (MD) simulation. To evaluate the drag force, a harmonic potential is used to the nanoparticle to constrain its Brownian motion in our MD simulation. In the steady state, the drag force can be obtained by the balance between the drag force and harmonic force. It is found that the gas-particle non-rigid-body interaction has a significant influence on the drag force of nanoparticle. For weak gas-solid coupling, the MD simulation results can be in good agreement with the prediction of Li-Wang theory. However, for strong coupling, there exists significant discrepancy between the MD simulation results and the theoretical results. Due to the gas-solid intermolecular interactions, gas molecules can be adsorbed on the nanoparticle surface, and after a time period, they may be re-emitted from the surface when they gain sufficient kinetic energy. Therefore, an adsorption-desorption equilibrium and an adsorption layer can be established on the particle surface. The adsorption layer enlarges the collision cross-sectional area and enhances the momentum transfer between gas molecules and the particle, and thus the drag force increases. This can explain the inconsistencies between the theoretical results and MD simulations. In this work, we introduce an adsorption ratio to evaluate the thickness of the adsorption layer. Then, the effective particle radius can be defined by the sum of particle radius and the thickness of the adsorption layer. By using the effective particle radius, the simulation values are in very good agreement with the theoretical predictions. The results of this work provide insights into the applications of nanoparticles in aerosol science.
-
Keywords:
- nanoparticle /
- transition regime /
- drag force /
- molecular dynamic simulations
[1] Li Z G 2009 Phys. Rev. E 80 061204
Google Scholar
[2] Wong R Y M, Liu C R, Wang J, Chao C Y H, Li Z G 2012 J. Nanosci. Nanotechnol. 12 2311
Google Scholar
[3] Livi C, Staso G D, Clercx H J H, Toschi F 2022 Phys. Rev. E 105 015306
Google Scholar
[4] Qiu J, Qiu T T 2015 Carbon 81 20
Google Scholar
[5] Zhang Y Y, Li S Q, Yan W, Yao Q 2012 Powder Technol. 227 24
Google Scholar
[6] Breddan M J D, Wirz R E 2023 J. Aerosol Sci. 167 106079
Google Scholar
[7] Westmeiera D, Solouk-Saranb D, Vallet C 2018 Proc. Natl. Acad. Sci. 115 7087
Google Scholar
[8] Pankhurst Q, Jones S, Dobson J 2016 J. Phys. D: Appl. Phys. 49 501002
Google Scholar
[9] Mackus A J M, Weber M J, Thissen N F W, Garcia-Alonso D, Vervuurt R H J, Assali S, Bol A A, Verheijen M A, Kessels W M M 2015 Nanotechnology 27 034001
Google Scholar
[10] Linic S, Aslam U, Boerigter C, Morabito M 2015 Nat. Mater. 14 567
Google Scholar
[11] Givehchi R, Tan Z 2015 J. Aerosol Sci. 83 12
Google Scholar
[12] Liu C R, Li Z G, Wang H 2016 Phys. Rev. E 94 023102
Google Scholar
[13] 刘东静, 周福, 陈帅阳, 胡志亮 2023 72 157901
Google Scholar
Liu D J, Zhou F, Chen S Y, Hu Z L 2023 Acta Phys. Sin. 72 157901
Google Scholar
[14] Wang J, Su J J, Xia G D 2020 Phys. Rev. E 101 013103
Google Scholar
[15] Gutiérrez-Varela O, Santamaria R 2021 J. Mol. Liq. 338 116466
Google Scholar
[16] 郭瑞雪, 艾保全 2023 72 200501
Google Scholar
Guo R X, Ai B Q 2023 Acta Phys. Sin. 72 200501
Google Scholar
[17] Liao M J, Wei M T, Xu S X, Ouyang D H, Sheng P 2019 Chin. Phys. B 28 084701
Google Scholar
[18] 刘晨昊, 刘天宇, 黄仁忠, 高天附, 舒咬根 2019 68 240501
Google Scholar
Liu C H, Liu T Y, Huang R Z, Gao T F, Shu Y G 2019 Acta Phys. Sin. 68 240501
Google Scholar
[19] Epstein P S 1924 Phys. Rev. 23 710
Google Scholar
[20] Stokes G G 1851 Proc. Cambridge Philos. Soc. 9 8
[21] Bird G A 1994 Molecular Gas Dynamics and the Direct Simulation of Gas Flows (Oxford: Clarendon Press) p55
[22] Cunningham E 1910 Proc. R. Soc. London, Ser. A 83 357
Google Scholar
[23] Allen M D, Raabe O G 1982 J. Aerosol Sci. 13 537
Google Scholar
[24] Liu C R, Wang H 2019 Phys. Rev. E 99 042127
Google Scholar
[25] Su J J, Wang J, Xia G D 2021 Chin. Phys. B 30 075101
Google Scholar
[26] Liu W, Wang J, Xia G D, Li Z G 2023 Phys. Fluids 35 083316
Google Scholar
[27] Luo S, Wang J, Xia G D, Li Z G 2016 J. Fluid Mech. 795 443
Google Scholar
[28] Wang J, Yu S, Luo S, Xia G D, Zong L 2018 Phys. Fluids 30 063302
Google Scholar
[29] Luo S, Wang J, Yu S, Xia G D, Li Z G 2018 J. Fluid Mech. 846 392
Google Scholar
[30] Li Z G, Wang H 2003 Phys. Rev. E 68 061206
Google Scholar
[31] Li Z G, Wang H 2003 Phys. Rev. E 68 061207
Google Scholar
[32] Zhou X W, Johnson R A, Wadley H N G 2004 Phys. Rev. B 69 144113
Google Scholar
[33] Kröger M 2004 Phys. Rep. 390 453
Google Scholar
[34] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[35] Galliero G, Volz S 2008 J. Chem. Phys. 128 064505
Google Scholar
[36] Liu W, Cui J, Wang J, Xia G D, Li Z G 2023 Phys. Fluids 35 032004
Google Scholar
[37] 崔杰, 苏俊杰, 王军, 夏国栋, 李志刚 2021 70 055101
Google Scholar
Cui J, Su J J, Wang J, Xia G D, Li Z G 2021 Acta Phys. Sin. 70 055101
Google Scholar
[38] Tsuji T, Iseki H, Hanasaki I, Kawano S 2016 30th International Symposium on Rarefied Gas Dynamics 1786 110003
Google Scholar
[39] Hess B 2002 J. Chem. Phys. 116 209
Google Scholar
[40] Gomez A, Rosner D E 1993 Combust. Sci. Technol. 89 335
Google Scholar
[41] 马奥杰, 陈颂佳, 李玉秀, 陈颖 2021 70 148201
Google Scholar
Ma A J, Chen S J, Li Y X, Chen Y 2021 Acta Phys. Sin. 70 148201
Google Scholar
[42] Li Z G, Wang H 2005 Phys. Rev. Lett. 95 014502
Google Scholar
[43] Liu C R, Li Z G 2010 Phys. Rev. Lett. 105 174501
Google Scholar
[44] Li Z G 2009 Phys. Rev. E 79 026312
Google Scholar
-
图 1 分子动力学模拟系统模型图(红色: 纳米颗粒; 蓝色: 气体分子)
Fig. 1. Configuration of MD simulation system (Red: nanoparticle; blue: gas molecules).
图 2 纳米颗粒的曳力随弹性系数khar的变化
Fig. 2. Drag force on nanoparticles versus elastic coefficient khar.
图 3 MD模拟值与理论公式在3种气固结合强度下的对比结果 (a) kSF = 0.01; (b) kSF = 0.1; (c) kSF = 0.5
Fig. 3. Comparison of MD simulation results of the drag force with theoretical values at various kSF: (a) kSF = 0.01; (b) kSF = 0.1; (c) kSF = 0.5.
图 4 气体分子在纳米颗粒表面吸附状况的模拟快照
Fig. 4. Snapshots of the adsorption of gas molecules (blue) on the nanoparticle (red) surface.
图 5 吸附率α随气固结合强度kSF的变化
Fig. 5. Adsorption ratio α versus gas-particle coupling parameters kSF.
σ/Å ε/K Ar-Ar 3.405 114 Cu-Cu 2.338 4753 Cu-Ar 2.871 736 
下载: 导出CSV
-
[1] Li Z G 2009 Phys. Rev. E 80 061204
Google Scholar
[2] Wong R Y M, Liu C R, Wang J, Chao C Y H, Li Z G 2012 J. Nanosci. Nanotechnol. 12 2311
Google Scholar
[3] Livi C, Staso G D, Clercx H J H, Toschi F 2022 Phys. Rev. E 105 015306
Google Scholar
[4] Qiu J, Qiu T T 2015 Carbon 81 20
Google Scholar
[5] Zhang Y Y, Li S Q, Yan W, Yao Q 2012 Powder Technol. 227 24
Google Scholar
[6] Breddan M J D, Wirz R E 2023 J. Aerosol Sci. 167 106079
Google Scholar
[7] Westmeiera D, Solouk-Saranb D, Vallet C 2018 Proc. Natl. Acad. Sci. 115 7087
Google Scholar
[8] Pankhurst Q, Jones S, Dobson J 2016 J. Phys. D: Appl. Phys. 49 501002
Google Scholar
[9] Mackus A J M, Weber M J, Thissen N F W, Garcia-Alonso D, Vervuurt R H J, Assali S, Bol A A, Verheijen M A, Kessels W M M 2015 Nanotechnology 27 034001
Google Scholar
[10] Linic S, Aslam U, Boerigter C, Morabito M 2015 Nat. Mater. 14 567
Google Scholar
[11] Givehchi R, Tan Z 2015 J. Aerosol Sci. 83 12
Google Scholar
[12] Liu C R, Li Z G, Wang H 2016 Phys. Rev. E 94 023102
Google Scholar
[13] 刘东静, 周福, 陈帅阳, 胡志亮 2023 72 157901
Google Scholar
Liu D J, Zhou F, Chen S Y, Hu Z L 2023 Acta Phys. Sin. 72 157901
Google Scholar
[14] Wang J, Su J J, Xia G D 2020 Phys. Rev. E 101 013103
Google Scholar
[15] Gutiérrez-Varela O, Santamaria R 2021 J. Mol. Liq. 338 116466
Google Scholar
[16] 郭瑞雪, 艾保全 2023 72 200501
Google Scholar
Guo R X, Ai B Q 2023 Acta Phys. Sin. 72 200501
Google Scholar
[17] Liao M J, Wei M T, Xu S X, Ouyang D H, Sheng P 2019 Chin. Phys. B 28 084701
Google Scholar
[18] 刘晨昊, 刘天宇, 黄仁忠, 高天附, 舒咬根 2019 68 240501
Google Scholar
Liu C H, Liu T Y, Huang R Z, Gao T F, Shu Y G 2019 Acta Phys. Sin. 68 240501
Google Scholar
[19] Epstein P S 1924 Phys. Rev. 23 710
Google Scholar
[20] Stokes G G 1851 Proc. Cambridge Philos. Soc. 9 8
[21] Bird G A 1994 Molecular Gas Dynamics and the Direct Simulation of Gas Flows (Oxford: Clarendon Press) p55
[22] Cunningham E 1910 Proc. R. Soc. London, Ser. A 83 357
Google Scholar
[23] Allen M D, Raabe O G 1982 J. Aerosol Sci. 13 537
Google Scholar
[24] Liu C R, Wang H 2019 Phys. Rev. E 99 042127
Google Scholar
[25] Su J J, Wang J, Xia G D 2021 Chin. Phys. B 30 075101
Google Scholar
[26] Liu W, Wang J, Xia G D, Li Z G 2023 Phys. Fluids 35 083316
Google Scholar
[27] Luo S, Wang J, Xia G D, Li Z G 2016 J. Fluid Mech. 795 443
Google Scholar
[28] Wang J, Yu S, Luo S, Xia G D, Zong L 2018 Phys. Fluids 30 063302
Google Scholar
[29] Luo S, Wang J, Yu S, Xia G D, Li Z G 2018 J. Fluid Mech. 846 392
Google Scholar
[30] Li Z G, Wang H 2003 Phys. Rev. E 68 061206
Google Scholar
[31] Li Z G, Wang H 2003 Phys. Rev. E 68 061207
Google Scholar
[32] Zhou X W, Johnson R A, Wadley H N G 2004 Phys. Rev. B 69 144113
Google Scholar
[33] Kröger M 2004 Phys. Rep. 390 453
Google Scholar
[34] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[35] Galliero G, Volz S 2008 J. Chem. Phys. 128 064505
Google Scholar
[36] Liu W, Cui J, Wang J, Xia G D, Li Z G 2023 Phys. Fluids 35 032004
Google Scholar
[37] 崔杰, 苏俊杰, 王军, 夏国栋, 李志刚 2021 70 055101
Google Scholar
Cui J, Su J J, Wang J, Xia G D, Li Z G 2021 Acta Phys. Sin. 70 055101
Google Scholar
[38] Tsuji T, Iseki H, Hanasaki I, Kawano S 2016 30th International Symposium on Rarefied Gas Dynamics 1786 110003
Google Scholar
[39] Hess B 2002 J. Chem. Phys. 116 209
Google Scholar
[40] Gomez A, Rosner D E 1993 Combust. Sci. Technol. 89 335
Google Scholar
[41] 马奥杰, 陈颂佳, 李玉秀, 陈颖 2021 70 148201
Google Scholar
Ma A J, Chen S J, Li Y X, Chen Y 2021 Acta Phys. Sin. 70 148201
Google Scholar
[42] Li Z G, Wang H 2005 Phys. Rev. Lett. 95 014502
Google Scholar
[43] Liu C R, Li Z G 2010 Phys. Rev. Lett. 105 174501
Google Scholar
[44] Li Z G 2009 Phys. Rev. E 79 026312
Google Scholar
下载:
计量
- 文章访问数: 1762
- PDF下载量: 83
- 被引次数: 0
