-
利用数值模拟和搭建的腔衰荡光谱装置, 研究了注入激光有限相干性对注入光与腔纵模耦合过程的影响. 注入光的有限相干性导致了频扫过程中耦合脉冲的随机性. 随机性主要体现在两个方面: 一是随着相干长度的减小, 耦合脉冲的随机幅值范围增大. 二是随着注入光的相干性变差, 耦合脉冲由强度随机演化的单脉冲变为连续多脉冲, 整体宽度随着扫频速率的降低而逐渐增大. 随着相干性的变差, 当使用腔体的光强来关闭注入光时, 扫频速度的降低会在一个频率耦合过程中引起不止一次的注入关断和腔衰荡事件, 特别是对于当利用腔体的长度进行频扫时. 此外, 本文提出了一种利用不同时间间隔的强度积分来估计衰荡时间的理论方法, 并进行相关的实验验证. 实验结果表明, 与传统拟合法得到的衰荡时间相比, 强度积分法对应的相对误差较小.Using numerical simulation and a constructed cavity ring-down spectroscopy device, the influence of the finite coherence of the injected laser on the coupling process between the injected light and the cavity longitudinal mode is studied. The finite coherence of the injected light leads to the randomness of the coupling pulse during frequency scanning. The randomness is mainly reflected in two aspects. One is that as the coherence length decreases, the random amplitude range of the coupling pulse increases, and the other is that as the coherence of the injected light deteriorates, the coupling pulse changes from a single pulse with intensity evolution into continuous multiple pulses, and the overall width gradually increases with the decrease of the scanning rate. Moreover, with the deterioration of the coherence, when the light intensity of the cavity is used to turn off the injected light, the decrease in the scanning rate can cause more than one injection shut-off and ring-down event in a frequency coupling process, especially when scanning with the length of the cavity. In addition, a theoretical method is proposed to estimate the ring-down time by using the strength integral of different time intervals, and the relevant experimental verification is carried out. The experimental results show that the relative error of the ring-down time obtained by the intensity integration method is smaller than that obtained by the traditional fitting method.
[1] O’Keefe A, Deacon D A 1988 Rev. Sci. Instrum. 59 2544
Google Scholar
[2] Berden G, Peeters R, Meijer G 2000 Int. Rev. Phys. Chem. 19 565
Google Scholar
[3] Brown S S 2003 Chem. Rev. 103 5219
Google Scholar
[4] Bucher C R, Lehmann K K, Plusquellic D F, Fraser G T 2000 Appl. Opt. 39 3154
Google Scholar
[5] Dudek J B, Tarsa P B, Velasquez A, Wladyslawski M, Rabinowitz P, Lehmann K K 2003 Anal. Chem. 75 4599
Google Scholar
[6] Chen H, Winderlich J, Gerbig C, Hoefer A, Rella C W, Crosson E R, Wofsy S C 2010 Atmos. Meas. Tech. 3 375
Google Scholar
[7] Guo R, Tengv J, Cao K, Dong H, Cui W, Zhang T 2019 Opt. Express 27 31850
Google Scholar
[8] Fasci E, Dinesan H, Moretti L, Merlone A, Castrillo A, Gianfrani L 2018 Metrologia. 55 662
Google Scholar
[9] 康鹏, 孙羽, 王进, 刘安雯, 胡水明 2018 67 104206
Google Scholar
Kang P, Sun Y, Wang J, Liu A W, Hu S M 2018 Acta Phys. Sin. 67 104206
Google Scholar
[10] Banik G D, Maity A, Som S, Pal M, Pradhan M 2018 Laser. Phys. 28 045701
Google Scholar
[11] Čermák P, Chomet B, Ferrieres L, Vasilchenko S, Mondelain D, Kassi S, Garnache A 2016 Rev. Sci. Instrum. 87 083109
Google Scholar
[12] Bahrini C, Bénilan Y, Jolly A, Lebert B, Landsheere X, Gazeau M C 2015 Appl. Phys. B 121 533
Google Scholar
[13] Romanini D, Kachanov A A, Sadeghi N, Stoeckel F 1997 Chem. Phys. Lett. 264 316
Google Scholar
[14] Cygan A, Lisak D, Masłowski P, Bielska K, Wójtewicz S, Domysławska J, Hodges J T 2011 Rev. Sci. Instrum. 82 063107
Google Scholar
[15] Tang Y, Yang S L, Lehmann K K 2012 Rev. Sci. Instrum. 83 043115
Google Scholar
[16] Schulz K J, Simpson W R 1998 Chem. Phys. Lett. 297 523
Google Scholar
[17] He Y, Orr B J 2004 Appl. Phys. B 79 941
Google Scholar
[18] Motto-Ros V, Morville J, Rairoux P 2007 Appl. Phys. B 87 531
Google Scholar
[19] Kassi S, Romanini D, Campargue A 2009 Chem. Phys. Lett. 477 17
Google Scholar
[20] Pan H, Cheng C F, Sun Y R, Gao B, Liu A W, Hu S M 2011 Rev. Sci. Instrum. 82 103110
Google Scholar
[21] Truong G W, Douglass K O, Maxwell S E, van Zee R D, Plusquellic D F, Hodges J T, Long D A 2013 Nat. Photonics. 7 532
Google Scholar
[22] Li Z, Bennett R G T, Stedman G E 1991 Opt. Commun. 86 51
Google Scholar
[23] An K, Yang C, Dasari R R, Feld M S 1995 Opt. Lett. 20 1068
Google Scholar
[24] Hahn J W, Yoo Y S, Lee J Y, Kim J W, Lee H W 1999 Appl. Opt. 38 1859
Google Scholar
[25] Morville J, Romanini D, Chenevier M, Kachanov A 2002 Appl. Opt. 41 6980
Google Scholar
[26] Yang Q S 2021 Adv. Photonics Res. 2 2000072
Google Scholar
[27] Lehmann K K, Romanini D 1996 J. Chem. Phys. 105 10263
Google Scholar
[28] Istratov A A, Vyvenko O F 1999 Rev. Sci. Instrum. 70 1233
Google Scholar
[29] Levenberg K 1944 Q. Appl. Math. 2 164
[30] Marquardt D W 1963 J. Soc. Indust. Appl. Math. 11 431
Google Scholar
[31] Naus H, van Stokkum I H, Hogervorst W, Ubachs W 2001 Appl. Opt. 40 4416
Google Scholar
[32] 姜亚军, 赵建林, 杨德兴 2009 光子学报 38 1740
Jang Y J, Zhao J L, Yang D X 2009 Acta. Photon. Sin. 38 1740
[33] 谭中奇, 龙兴武 2007 激光杂志 28 0020
Tan Z Q, Long X W 2007 Laser J. 28 0020
[34] Matheson I B C 1987 Instrum. Sci. Technol. 16 345
Google Scholar
[35] Halmer D, von Basum G, Hering P, Mürtz M 2004 Rev. Sci. Instrum. 75 2187
Google Scholar
-
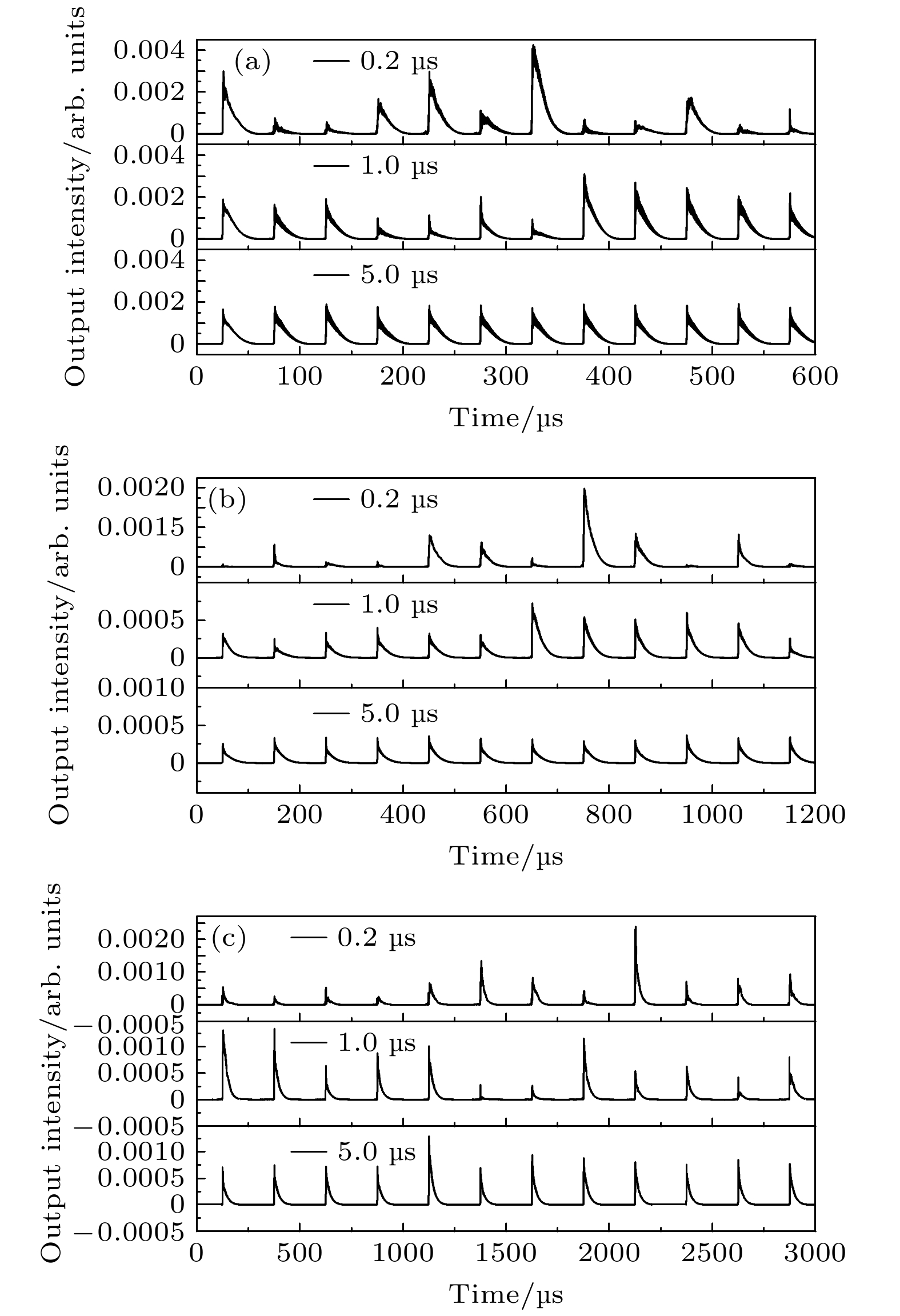
图 1 在不同扫频速率下耦合过程中输出强度的演化(其中扫频周期定义为RDC一个自由频谱范围的扫频时间) (a) TFSR = 100 μs; (b) TFSR = 200 μs; (c) TFSR = 500 μs
Fig. 1. Evolution of output intensity during coupling at different sweep rates, where the sweep period is defined as the sweep time of a free spectrum range of the decay cavity: (a) TFSR = 100 μs; (b) TFSR = 200 μs; (c) TFSR = 500 μs
图 2 腔长为600 mm时, 不同扫频速率下耦合脉冲输出强度的演变规律 (a) TFSR = 50 μs; (b) TFSR = 100 μs; (c) TFSR = 250 μs
Fig. 2. Evolution of the output intensity of the coupled pulse at different sweep rates at a cavity length of 600 mm: (a) TFSR = 50 μs; (b) TFSR = 100 μs; (c) TFSR = 250 μs.
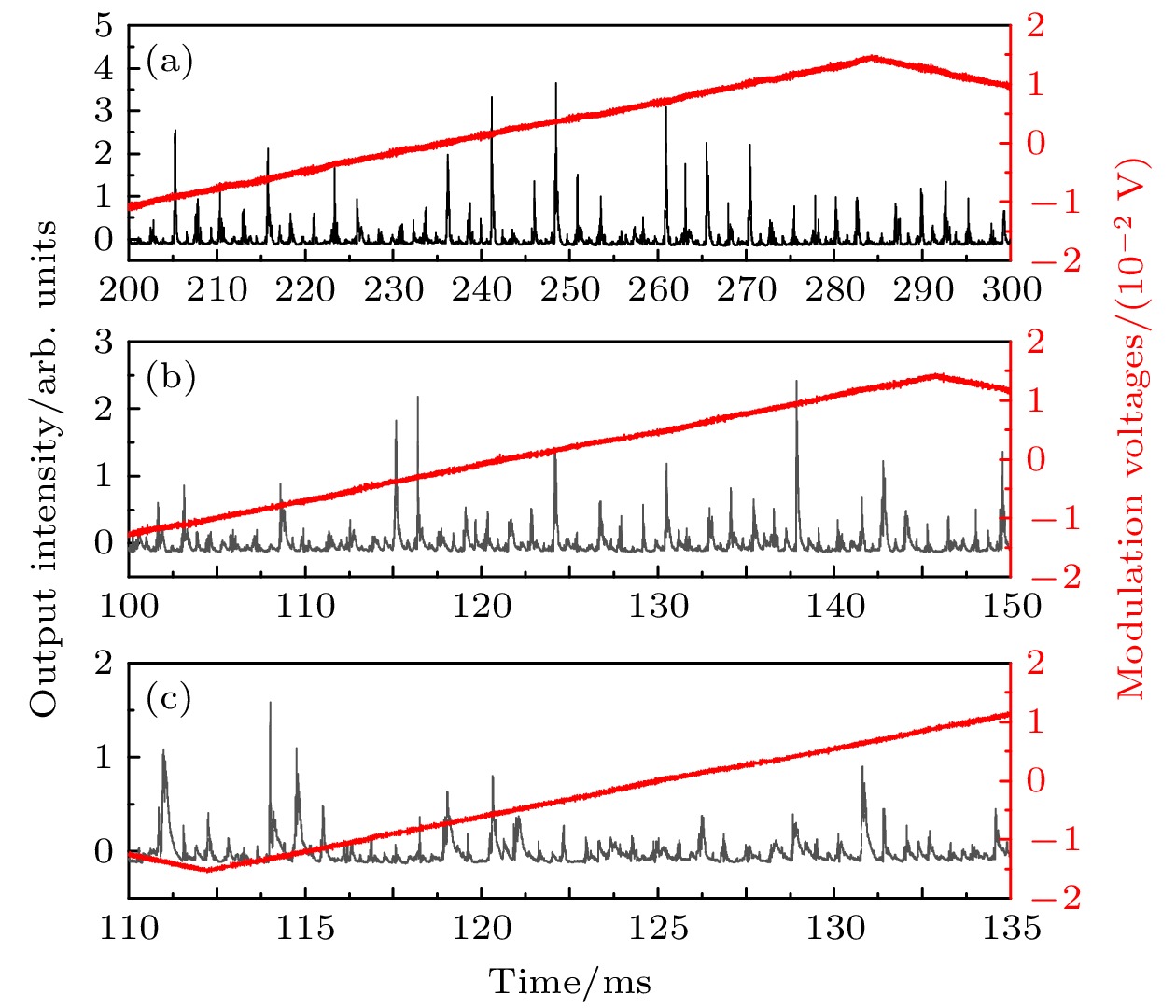
图 4 在驱动电流为81 mA和波长为1527.013 nm时, 注入光与衰荡腔纵模耦合的输出强度, 其中调制周期分别为(a) 200 ms, (b) 100 ms, (c) 50 ms; 调制电压的幅值为30 mV, 相应的频移为5.93 GHz
Fig. 4. Output intensity of longitudinal mode coupling of the injected light with the deaconation cavity with a driving current of 81 mA and a wavelength of 1527.013 nm. The modulation periods are (a) 200 ms, (b) 100 ms, and (c) 50 ms, respectively. The modulation voltage has an amplitude of 30 mV and a corresponding frequency shift of 5.93 GHz
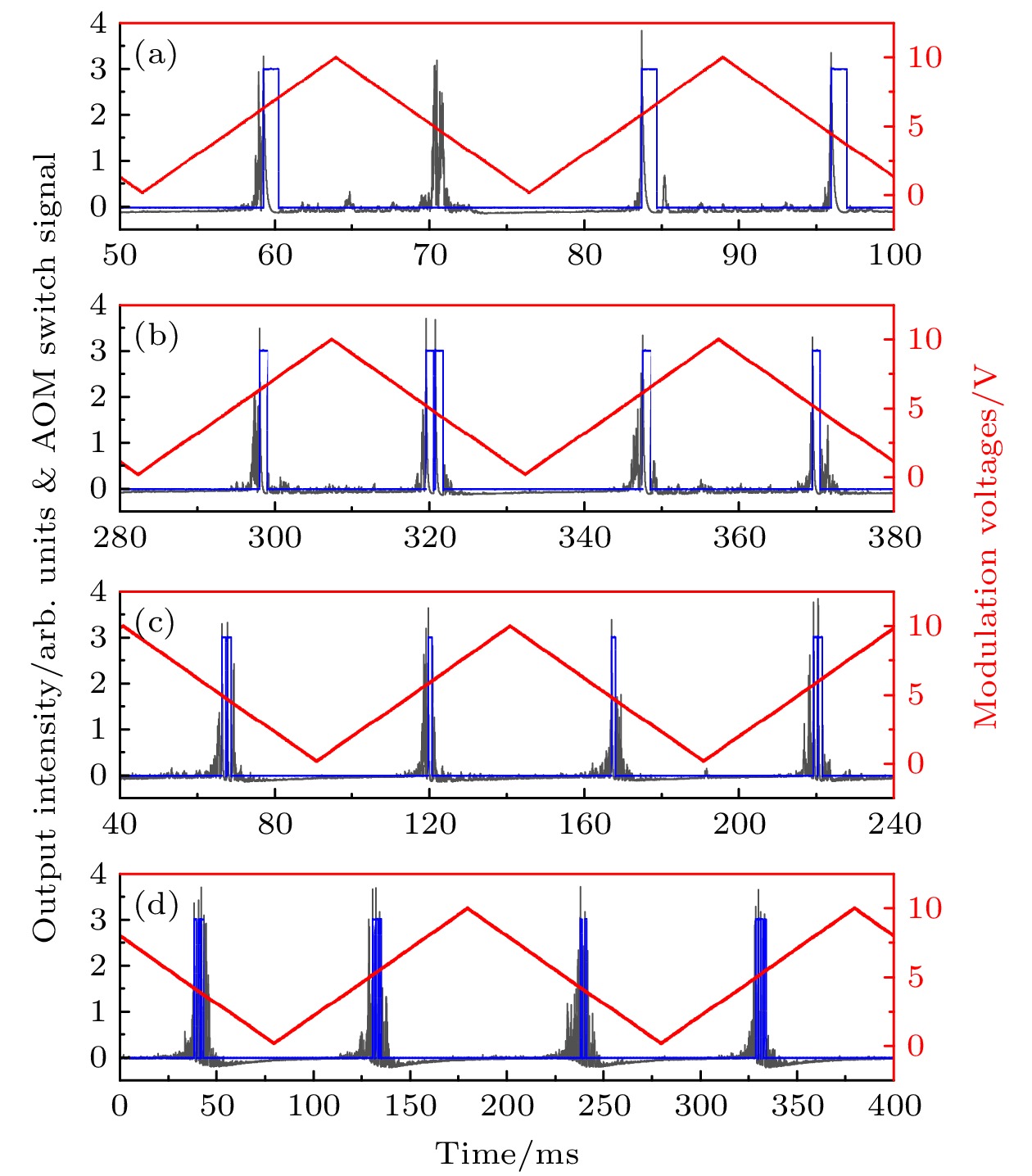
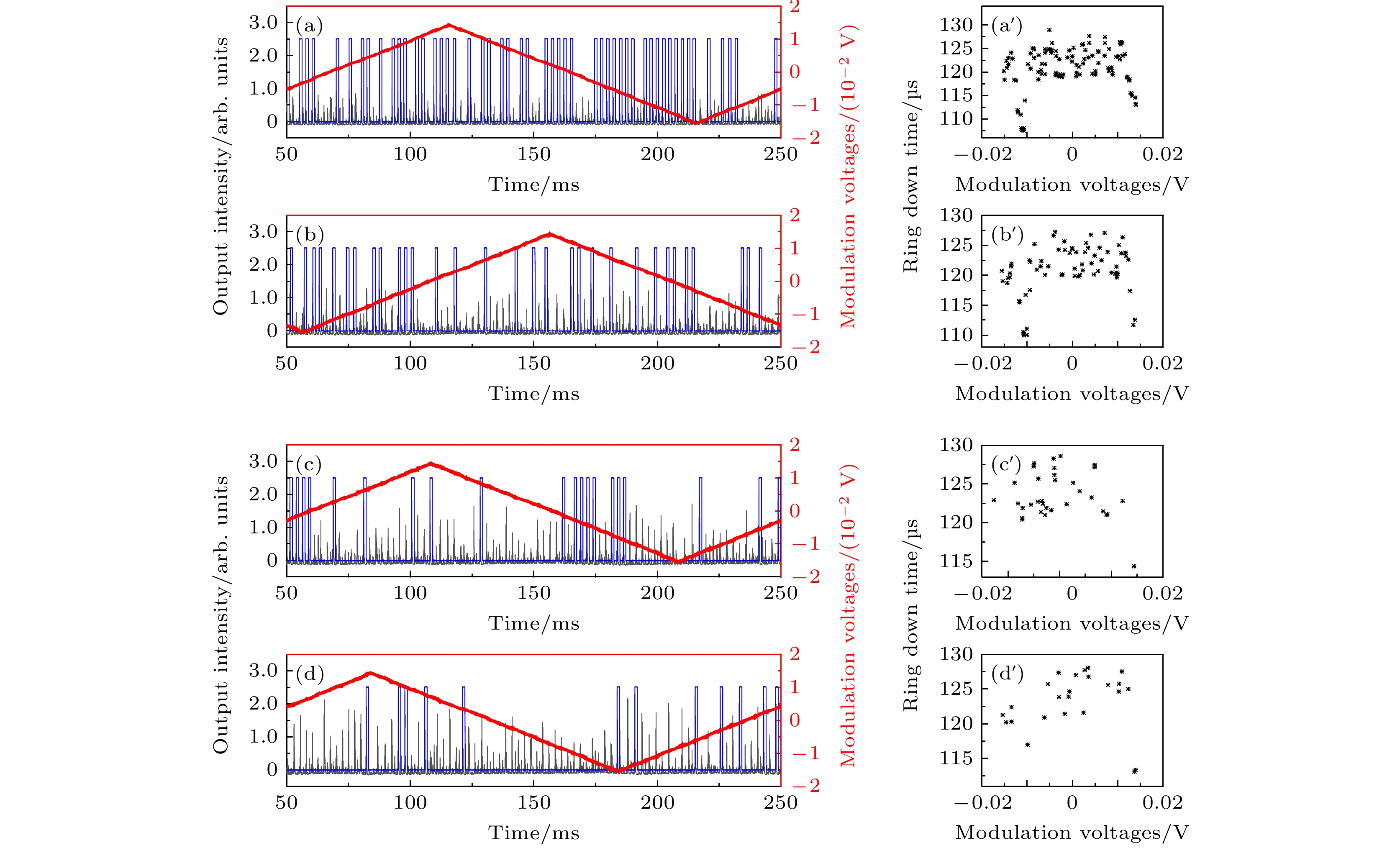
图 8 RDC的输出达到3.5 V时, 伴随注入光通过AOM快速关闭过程的注入过程和衰荡过程、三角波调制和AOM开关信号的时间曲线, 其中调制周期分别为(a) 25 ms, (b) 50 ms, (c) 100 ms, (d) 200 ms
Fig. 8. When the output of the fading cavity reaches 3.5 V, the time curve of the injection process and the ring-down process, triangular wave modulation, and AOM switching signal with the quick close of the injected light through the AOM. The modulation periods are (a) 25 ms, (b) 50 ms, (c) 100 ms, (d) 200 ms.
图 3 具有两种频扫性能的衰荡腔光谱测量装置
Fig. 3. Measurement device for the fading cavity spectrum with two types of frequency sweep performance.
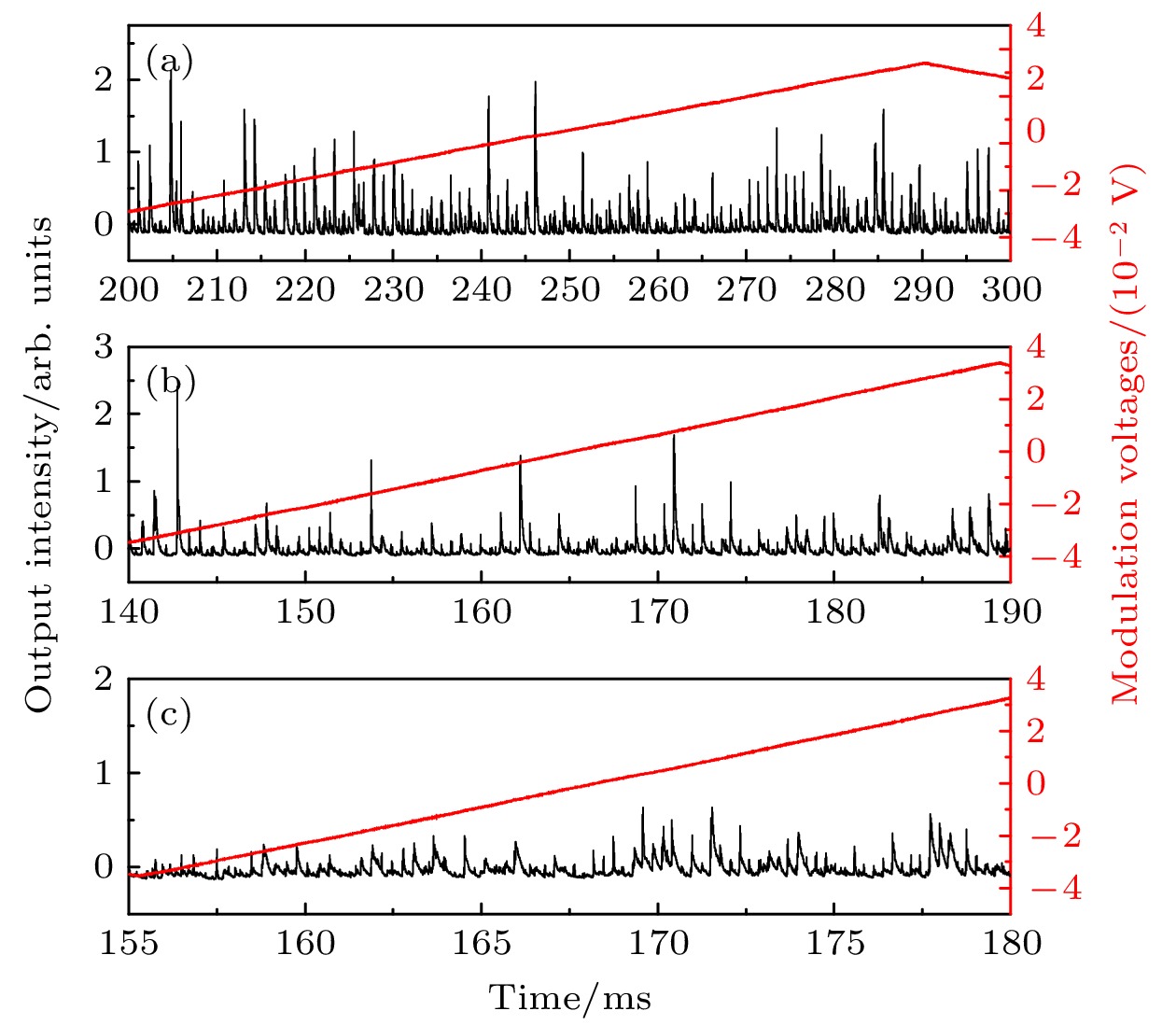
图 5 注入光与RDC纵模耦合产生的输出强度, 其中调制周期分别为(a) 200 ms, (b) 100 ms和(c) 50 ms; 驱动电流与图4相同, 调制电压为70 mV, 对应的频移为13.84 GHz
Fig. 5. Output intensity resulting from the longitudinal mode coupling of injected light and the decay cavity. The modulation periods are (a) 200 ms, (b) 100 ms, and (c) 50 ms, where the drive current is the same as Fig. 4, the modulation voltage is 70 mV, and the corresponding frequency shift is 13.84 GHz
图 6 典型的输出脉冲序列、波长调制的三角波和方波触发信号(a)—(d)及其不同触发电平的衰荡时间值(a')—(d'), 其中关闭注入光的触发电压分别为(a) 1.0 V, (b) 1.5 V, (c) 2.0 V, (d) 2.5 V; 调制幅值为30 mV, 周期为200 ms, 当方波为高压时, AOM处于关断状态
Fig. 6. Typical output pulse trains, wavelength-modulated triangle and square wave trigger signals (a)–(d) and their attenuation time values for different trigger levels (a')–(d'). The trigger voltages for closing the injected light are (a) 1.0 V, (b) 1.5 V, (c) 2.0 V, (d) 2.5 V. Here, the modulated amplitude is 30 mV with a period of 200 ms, and the AOM is off when the square wave is high voltage
图 7 当注入光具有恒定波长时, RDC的周期三角波调制导致的耦合过程输出, 这里驱动电流为81 mA, 工作温度为13.889 ℃, 调制周期分别为(a) 50 ms, (b) 100 ms, (c) 200 ms
Fig. 7. Coupling process output caused by the periodic triangulation wave modulation of RDC when the injected light has a constant wavelength. The drive current of decay cavity is 81 mA, an operating temperature is 13.889 ℃, and the modulation periods are (a) 50 ms, (b) 100 ms, and (c) 200 ms, respectively.
图 9 由衰减曲线估计RDT的阶段时间区域强度积分法原理图及计算公式
Fig. 9. Schematic diagram and calculation formula of the phase time zone intensity integration method for estimating the RDT from the ring-down curve.
图 10 (a)用拟合法和强度积分法分别计算的1000个实际衰荡事件对应的衰荡时间值; (b)用
$ 2\times |({\tau }_{{\rm{R}}{\rm{D}}, {\rm{f}}{\rm{i}}{\rm{t}}{\rm{t}}{\rm{i}}{\rm{n}}{\rm{g}}}- $ $ {\tau }_{{\rm{R}}{\rm{D}}, {\rm{i}}{\rm{n}}{\rm{t}}{\rm{e}}{\rm{g}}{\rm{r}}{\rm{a}}{\rm{l}}})/ \left({\tau }_{{\rm{R}}{\rm{D}}, {\rm{f}}{\rm{i}}{\rm{t}}{\rm{t}}{\rm{i}}{\rm{n}}{\rm{g}}}+{\tau }_{{\rm{R}}{\rm{D}}, {\rm{i}}{\rm{n}}{\rm{t}}{\rm{e}}{\rm{g}}{\rm{r}}{\rm{a}}{\rm{l}}}\right)| $ 公式计算的两种推算衰荡时间的相对误差值.Fig. 10. (a) Ring-down time values corresponding to 1000 actual ring-down events calculated by the fitting method and intensity integration method respectively; (b) the relative error values of the two estimated ring-down times calculated by the formula
$ 2\times \left|\dfrac{{\tau }_{{\rm{R}}{\rm{D}}, {\rm{f}}{\rm{i}}{\rm{t}}{\rm{t}}{\rm{i}}{\rm{n}}{\rm{g}}}- {\tau }_{{\rm{R}}{\rm{D}}, {\rm{i}}{\rm{n}}{\rm{t}}{\rm{e}}{\rm{g}}{\rm{r}}{\rm{a}}{\rm{l}}}}{{\tau }_{{\rm{R}}{\rm{D}}, {\rm{f}}{\rm{i}}{\rm{t}}{\rm{t}}{\rm{i}}{\rm{n}}{\rm{g}}}+{\tau }_{{\rm{R}}{\rm{D}}, {\rm{i}}{\rm{n}}{\rm{t}}{\rm{e}}{\rm{g}}{\rm{r}}{\rm{a}}{\rm{l}}}}\right| $ -
[1] O’Keefe A, Deacon D A 1988 Rev. Sci. Instrum. 59 2544
Google Scholar
[2] Berden G, Peeters R, Meijer G 2000 Int. Rev. Phys. Chem. 19 565
Google Scholar
[3] Brown S S 2003 Chem. Rev. 103 5219
Google Scholar
[4] Bucher C R, Lehmann K K, Plusquellic D F, Fraser G T 2000 Appl. Opt. 39 3154
Google Scholar
[5] Dudek J B, Tarsa P B, Velasquez A, Wladyslawski M, Rabinowitz P, Lehmann K K 2003 Anal. Chem. 75 4599
Google Scholar
[6] Chen H, Winderlich J, Gerbig C, Hoefer A, Rella C W, Crosson E R, Wofsy S C 2010 Atmos. Meas. Tech. 3 375
Google Scholar
[7] Guo R, Tengv J, Cao K, Dong H, Cui W, Zhang T 2019 Opt. Express 27 31850
Google Scholar
[8] Fasci E, Dinesan H, Moretti L, Merlone A, Castrillo A, Gianfrani L 2018 Metrologia. 55 662
Google Scholar
[9] 康鹏, 孙羽, 王进, 刘安雯, 胡水明 2018 67 104206
Google Scholar
Kang P, Sun Y, Wang J, Liu A W, Hu S M 2018 Acta Phys. Sin. 67 104206
Google Scholar
[10] Banik G D, Maity A, Som S, Pal M, Pradhan M 2018 Laser. Phys. 28 045701
Google Scholar
[11] Čermák P, Chomet B, Ferrieres L, Vasilchenko S, Mondelain D, Kassi S, Garnache A 2016 Rev. Sci. Instrum. 87 083109
Google Scholar
[12] Bahrini C, Bénilan Y, Jolly A, Lebert B, Landsheere X, Gazeau M C 2015 Appl. Phys. B 121 533
Google Scholar
[13] Romanini D, Kachanov A A, Sadeghi N, Stoeckel F 1997 Chem. Phys. Lett. 264 316
Google Scholar
[14] Cygan A, Lisak D, Masłowski P, Bielska K, Wójtewicz S, Domysławska J, Hodges J T 2011 Rev. Sci. Instrum. 82 063107
Google Scholar
[15] Tang Y, Yang S L, Lehmann K K 2012 Rev. Sci. Instrum. 83 043115
Google Scholar
[16] Schulz K J, Simpson W R 1998 Chem. Phys. Lett. 297 523
Google Scholar
[17] He Y, Orr B J 2004 Appl. Phys. B 79 941
Google Scholar
[18] Motto-Ros V, Morville J, Rairoux P 2007 Appl. Phys. B 87 531
Google Scholar
[19] Kassi S, Romanini D, Campargue A 2009 Chem. Phys. Lett. 477 17
Google Scholar
[20] Pan H, Cheng C F, Sun Y R, Gao B, Liu A W, Hu S M 2011 Rev. Sci. Instrum. 82 103110
Google Scholar
[21] Truong G W, Douglass K O, Maxwell S E, van Zee R D, Plusquellic D F, Hodges J T, Long D A 2013 Nat. Photonics. 7 532
Google Scholar
[22] Li Z, Bennett R G T, Stedman G E 1991 Opt. Commun. 86 51
Google Scholar
[23] An K, Yang C, Dasari R R, Feld M S 1995 Opt. Lett. 20 1068
Google Scholar
[24] Hahn J W, Yoo Y S, Lee J Y, Kim J W, Lee H W 1999 Appl. Opt. 38 1859
Google Scholar
[25] Morville J, Romanini D, Chenevier M, Kachanov A 2002 Appl. Opt. 41 6980
Google Scholar
[26] Yang Q S 2021 Adv. Photonics Res. 2 2000072
Google Scholar
[27] Lehmann K K, Romanini D 1996 J. Chem. Phys. 105 10263
Google Scholar
[28] Istratov A A, Vyvenko O F 1999 Rev. Sci. Instrum. 70 1233
Google Scholar
[29] Levenberg K 1944 Q. Appl. Math. 2 164
[30] Marquardt D W 1963 J. Soc. Indust. Appl. Math. 11 431
Google Scholar
[31] Naus H, van Stokkum I H, Hogervorst W, Ubachs W 2001 Appl. Opt. 40 4416
Google Scholar
[32] 姜亚军, 赵建林, 杨德兴 2009 光子学报 38 1740
Jang Y J, Zhao J L, Yang D X 2009 Acta. Photon. Sin. 38 1740
[33] 谭中奇, 龙兴武 2007 激光杂志 28 0020
Tan Z Q, Long X W 2007 Laser J. 28 0020
[34] Matheson I B C 1987 Instrum. Sci. Technol. 16 345
Google Scholar
[35] Halmer D, von Basum G, Hering P, Mürtz M 2004 Rev. Sci. Instrum. 75 2187
Google Scholar
下载:
计量
- 文章访问数: 2556
- PDF下载量: 61
- 被引次数: 0
