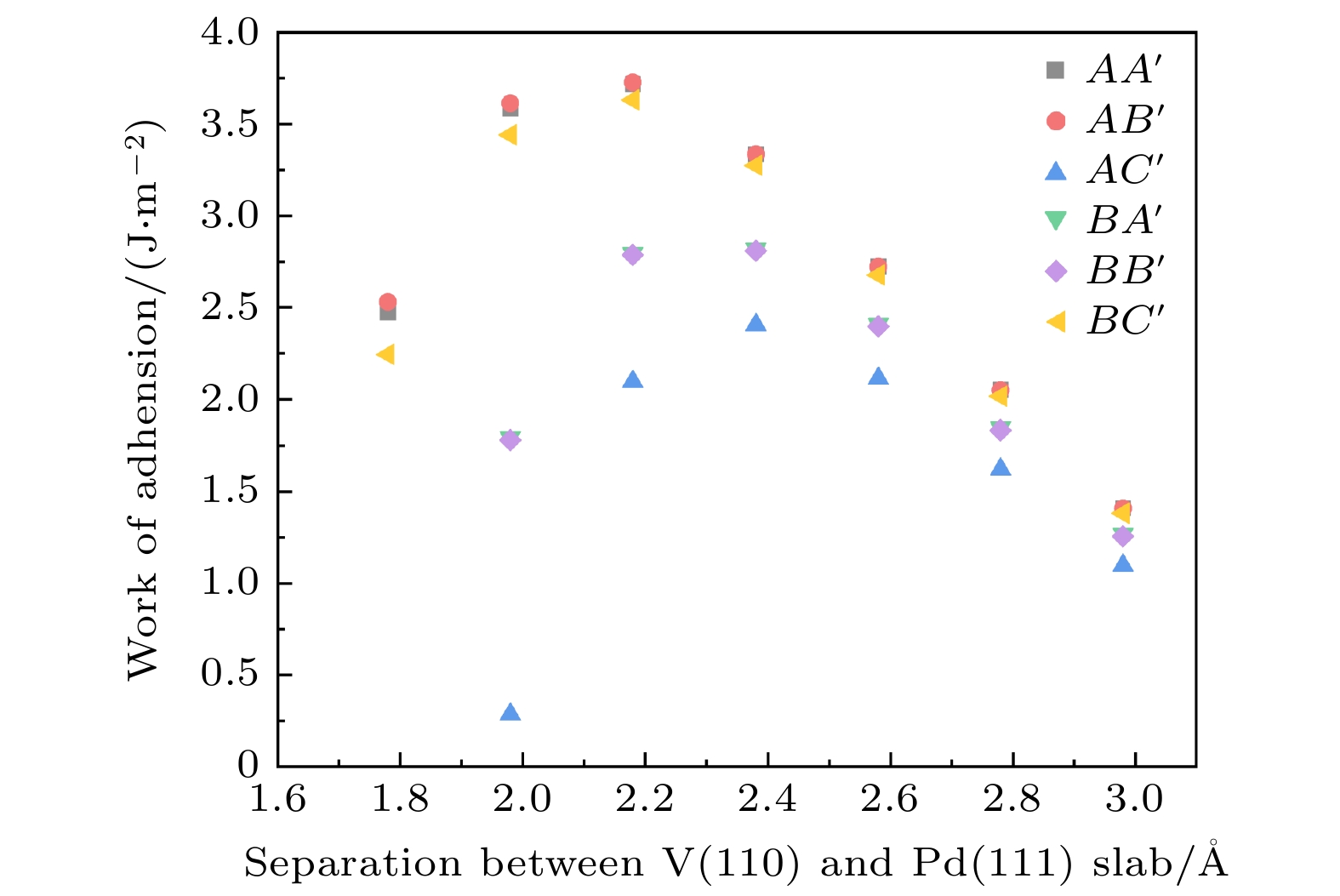
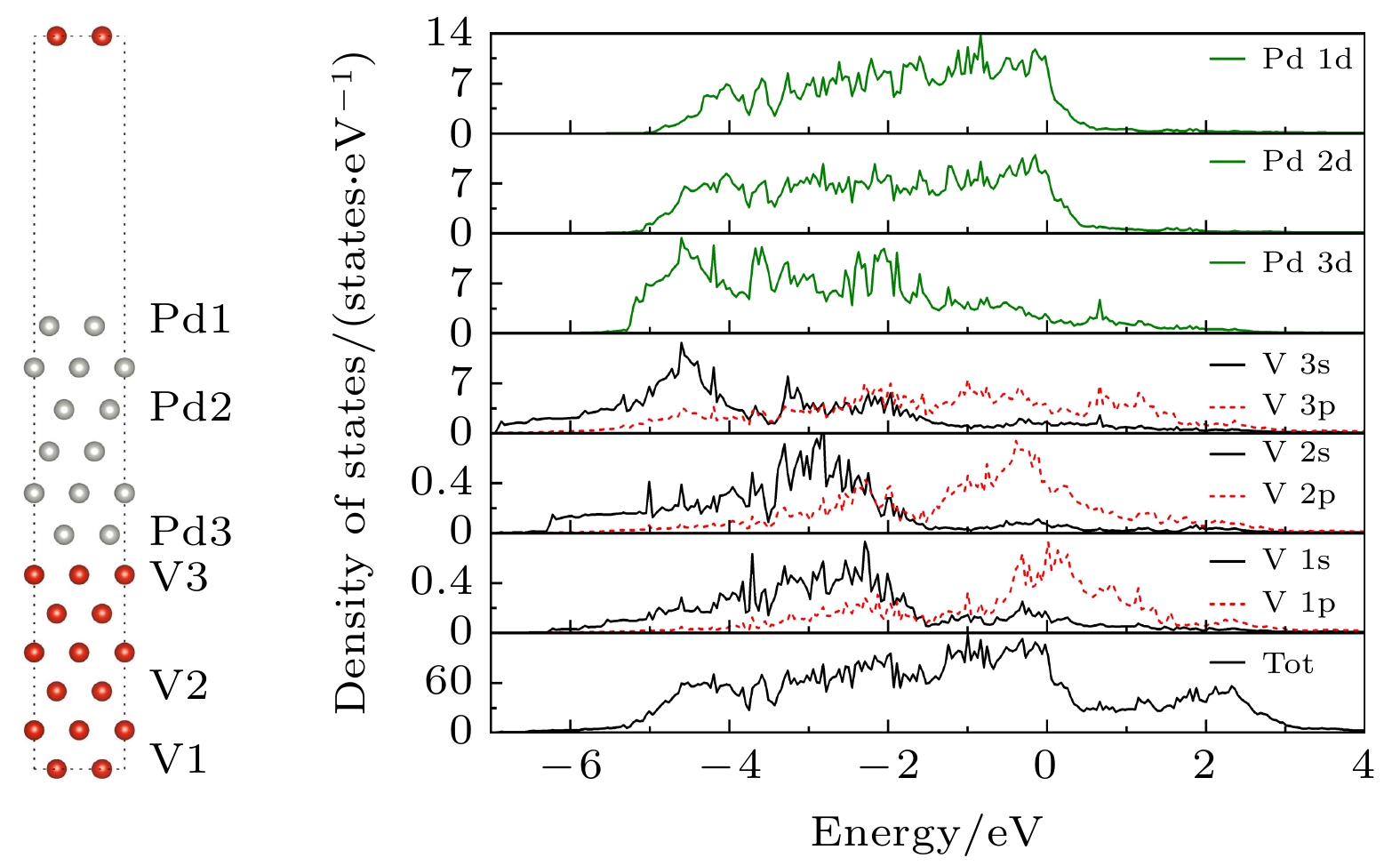
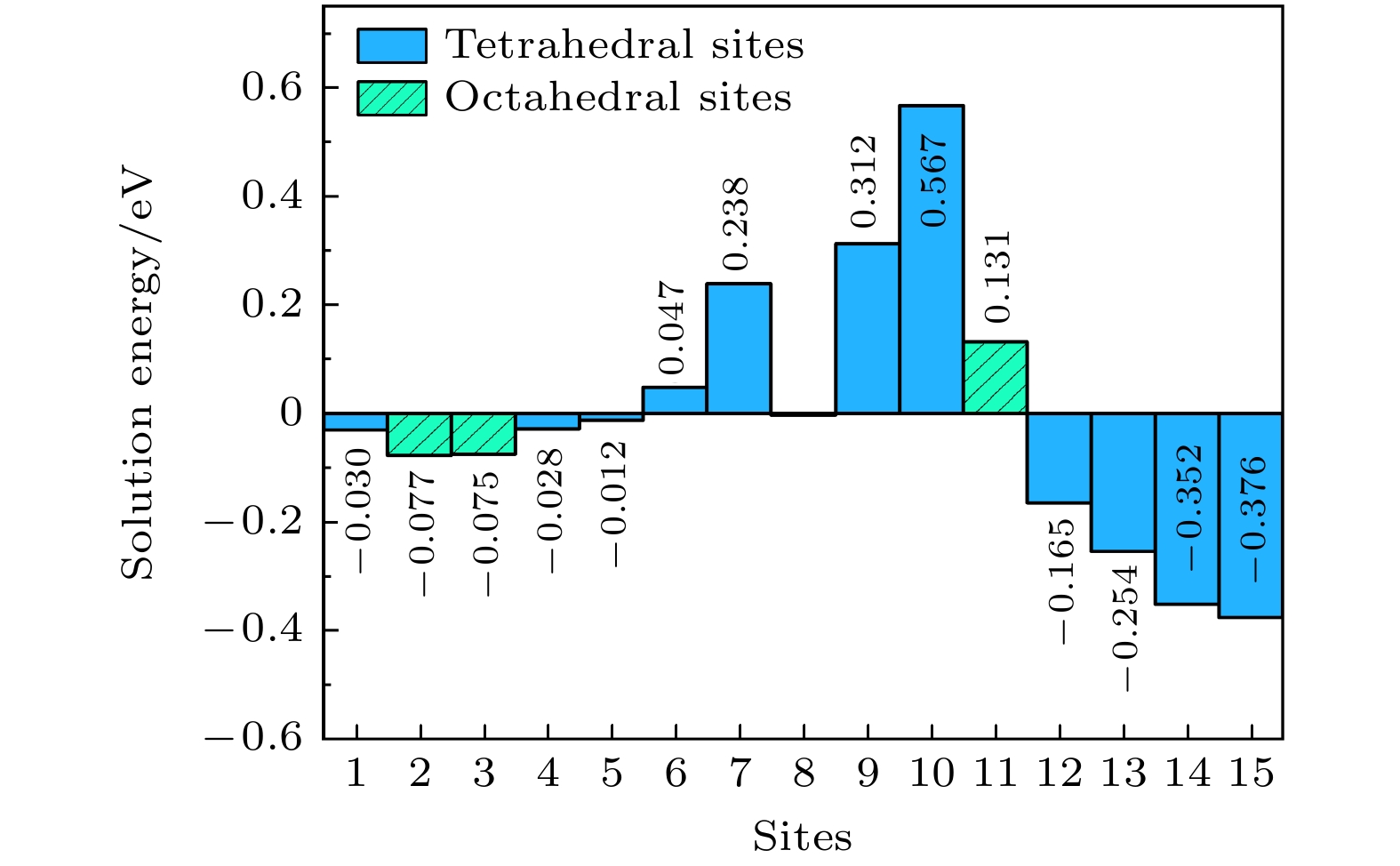
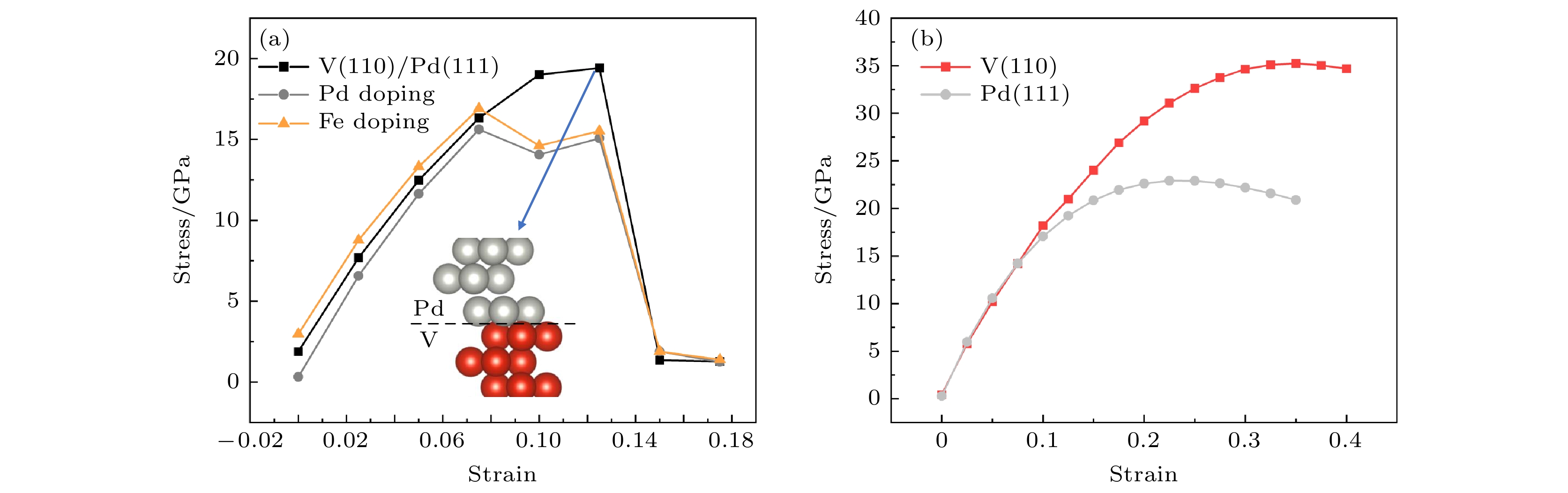
-
采用钒/钯(V/Pd)金属复合膜渗氢是从混合气体中分离氢气的一种有效实用方法. 为深入地了解催化Pd层与金属膜结合处的界面结构与吸氢/渗氢特性的关联性, 进而提升合金膜提纯氢气的能力, 本文采用基于密度泛函理论的第一性原理研究了V/Pd金属复合膜界面的氢吸附/扩散行为. 研究结果表明: 由于V/Pd界面的电荷密度随着V/Pd成键而增加, 导致氢原子(H)溶解能随着接近界面而增大, 在V/Pd界面附近具有最高的溶解能(0.567 eV). 氢迁移能垒计算表明, 与H沿V/Pd界面水平扩散的最大能垒(0.64 eV)相比, H垂直V/Pd界面能垒(0.56 eV)更小, 因而H倾向于垂直V/Pd界面进行迁移, 并由Pd层扩散到V基体一侧, 因V/Pd界面处Pd层的氢溶解能(0.238 eV)高于V膜侧(–0.165 eV), H将在界面的V膜侧积累, 易引起氢脆. V基体掺杂Pd/Fe的计算表明, 与未掺杂的能垒(0.56 eV)相比, 掺杂Pd/Fe可明显地降低界面扩散路径中的最大能垒(0.45 eV/0.54 eV), 利于氢的渗透扩散, 且掺杂界面能一定程度抑制V和催化Pd层的相互扩散, 提高复合膜的结构稳定性.Hydrogen permeation through vanadium/palladium (V/Pd) metal composite membranes is an effective and practical method of separating hydrogen from gas mixtures. In order to gain an insight into the relation between the interfacial structure and hydrogen adsorption/diffusion properties of the catalytic Pd layer bonded to the metal membrane, and then improve the ability of the alloy membrane to purify hydrogen, the first principle based on the density functional theory is used to study the hydrogen adsorption/diffusion behavior at the V/Pd metal composite membrane interface. The results show that because the charge density at the V/Pd interface increases with the V/Pd bonding increasing, the dissolution energy of hydrogen atom (H) increases with it approaching to the interface, and it has the highest dissolution energy near the V/Pd interface (0.567 eV). Hydrogen migration energy barrier calculations show that compared with the maximum energy barrier for horizontal diffusion of H along the V/Pd interface (0.64 eV), the H vertical V/Pd interface energy barrier (0.56 eV) is small, thus H tends to migrate vertically V/Pd interface and diffuse from the Pd layer to the V substrate side. As the hydrogen solvation energy of the Pd layer at the V/Pd interface (0.238 eV) is higher than that on the V membrane side (–0.165 eV), H will gather on the V film side of the interface, which is easy to cause hydrogen to be embrittled. Calculations of Pd/Fe doping of the V matrix show that comparing with the undoped energy barrier (0.56 eV), Pd/Fe doping can significantly reduce the maximum energy barrier (0.45 eV/0.54 eV) in the diffusion path of the interface, which is favorable for hydrogen permeation and diffusion. And the doped interface can inhibit the interdiffusion of V layer and catalytic Pd layer to a certain extent, which improves the structural stability of the composite film.
-
Keywords:
- V/Pd interface /
- first principle /
- hydrogen permeation characteristics /
- mechanical property
[1] Li Q, Garcia-Muelas R, Lopez N 2018 Nat. Commun. 9 526
Google Scholar
[2] Kim T, Song Y, Kang J, Kim S K, Kim S 2022 Int. J. Hydrogen Energy 47 24817
Google Scholar
[3] Agnolin S, Melendez J, Di Felice L, Gallucci F 2022 Int. J. Hydrogen Energy 47 28505
Google Scholar
[4] Park S B, Nam G H, Park Y I 2022 Thin Solid Films 757 139391
Google Scholar
[5] Dolan M D 2010 J. Membr. Sci. 362 12
Google Scholar
[6] Kozhakhmetov S, Sidorov N, Piven V, Sipatov I, Gabis I, Arinov B 2015 J. Alloys Compd. 645 S36
Google Scholar
[7] Dolan M D, Viano D M, Langley M J, Lamb K E 2018 J. Membr. Sci. 549 306
Google Scholar
[8] Zhang S, Zhang Z, Li J, Tu R, Shen Q, Wang C, Luo G, Zhang L 2020 J. Wuhan Univ. Technol. 35 879
Google Scholar
[9] Ko W S, Jeon J B, Shim J H, Lee B J 2012 Int. J. Hydrogen Energy 37 13583
Google Scholar
[10] Fasolin S, Barison S, Agresti F, Battiston S, Fiameni S, Isopi J, Armelao L 2022 Membranes (Basel, Switz.) 12 16
Google Scholar
[11] Alimov V N, Busnyuk A O, Notkin M E, Livshits A I 2014 J. Membr. Sci. 457 103
Google Scholar
[12] Dai J H, Xie R W, Chen Y Y, Song Y 2015 Phys. Chem. Chem. Phys. 17 16594
Google Scholar
[13] Il Jeon S, Park J H, Magnone E, Lee Y T, Fleury E 2012 Curr. Appl. Phys. 12 394
Google Scholar
[14] Ko W S, Oh J Y, Shim J H, Suh J Y, Yoon W Y, Lee B J 2014 Int. J. Hydrogen Energy 39 12031
Google Scholar
[15] Qin J Y, Wang Z M, Wang D H, Wang F, Yan X F, Zhong Y, Hu C H, Zhou H Y 2019 J. Alloys Compd. 805 747
Google Scholar
[16] Qin J Y, Hao C Y, Wang D H, Wang F, Yan X F, Zhong Y, Wang Z M, Hu C H, Wang X T 2020 J. Adv. Res. 21 25
Google Scholar
[17] Mills G, Jonsson H, Schenter G K 1995 Surf. Sci. 324 305
Google Scholar
[18] Henkelman G, Jonsson H 2000 J. Chem. Phys. 113 9978
Google Scholar
[19] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[20] Blochl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[21] Qin J Y, Liu Z G, Zhao W, Wang D A H, Zhang Y L, Zhong Y, Zhang X H, Wang Z M, Hu C H, Liu J W 2021 Materials 14 12
Google Scholar
[22] Mills G, Schenter G K, Makarov D E, Jonsson H 1997 Chem. Phys. Lett. 278 91
Google Scholar
[23] Mills G, Jonsson H 1994 Phys. Rev. Lett. 72 1124
Google Scholar
[24] Chen J F, Hu L, Tang T 2022 J. Phys. Chem. C 126 7468
Google Scholar
[25] Castleton C W M, Hoglund A, Gothelid M, Qian M C, Mirbt S 2013 Phys. Rev. B 88 7
Google Scholar
[26] Zhang H L, Wang J J, Huang W J, Wang L Q, Lu Z B 2022 Surf. Interfaces 30 10
Google Scholar
[27] Rose J H, Ferrante J, Smith J R 1981 Phys. Rev. Lett. 47 675
Google Scholar
[28] Jin N, Yang Y Q, Li J, Luo X, Huang B, Sun Q, Guo P F 2014 J. Appl. Phys. 115 11
Google Scholar
[29] Lu T, Chen Q X 2018 Acta Phys. Chim. Sin. 34 503
Google Scholar
[30] Wang J W, Song M, He Y H, Gong H R 2016 J. Membr. Sci. 503 124
Google Scholar
[31] Kang S G, Coulter K E, Gade S K, Way J D, Sholl D S 2011 J. Phys. Chem. Lett. 2 3040
Google Scholar
[32] Yang L, Wirth B D 2020 J. Appl. Phys. 127 12
Google Scholar
[33] Puska M J, Nieminen R M, Manninen M 1981 Phys. Rev. B 24 3037
Google Scholar
[34] Ko W S, Shim J H, Jung W S, Lee B J 2016 J. Membr. Sci. 497 270
Google Scholar
-
图 1 V(110)面与Pd(111)面的6种组合模型(Pd原子为银白色, V原子为红色) (a) AA'; (b) AB'; (c) AC'; (d) BA'; (e) BB'; (f) BC'
Fig. 1. Six combined models of V(110) plane and Pd(111) plane (Pd atoms are silvery white and V atoms are red): (a) AA'; (b) AB'; (c) AC'; (d) BA'; (e) BB'; (f) BC'.
图 2 V(110)/Pd(111)界面的理想黏附功随着V和Pd原子层之间的分离的变化
Fig. 2. Change of ideal adhesion work of V(110)/Pd(111) interface with the separation between V and Pd layers.
图 3 V/Pd界面模型和相应的局域态密度
Fig. 3. V/Pd interface model and the corresponding local density of states.
图 4 V/Pd界面电子局域化函数图
Fig. 4. Electronic localization function diagram of V/Pd interface.
图 5 (a) V/Pd界面模型, 其中方块表示Ｈ原子溶解的间隙位; (b) 四面体位置; (c)八面体位置; (d) 伪八面体位置
Fig. 5. (a) V/Pd interface model, where the square represents the interstitial position of H atom dissolution; (b) tetrahedron position; (c) octahedron position; (d) pseudo octahedron position.
图 6 界面中不同位置上的氢溶解能
Fig. 6. Hydrogen dissolution energy at different positions in the interface.
图 7 H垂直通过V/Pd界面的迁移能量
Fig. 7. Migration energy of H passing through V/Pd interface vertically.
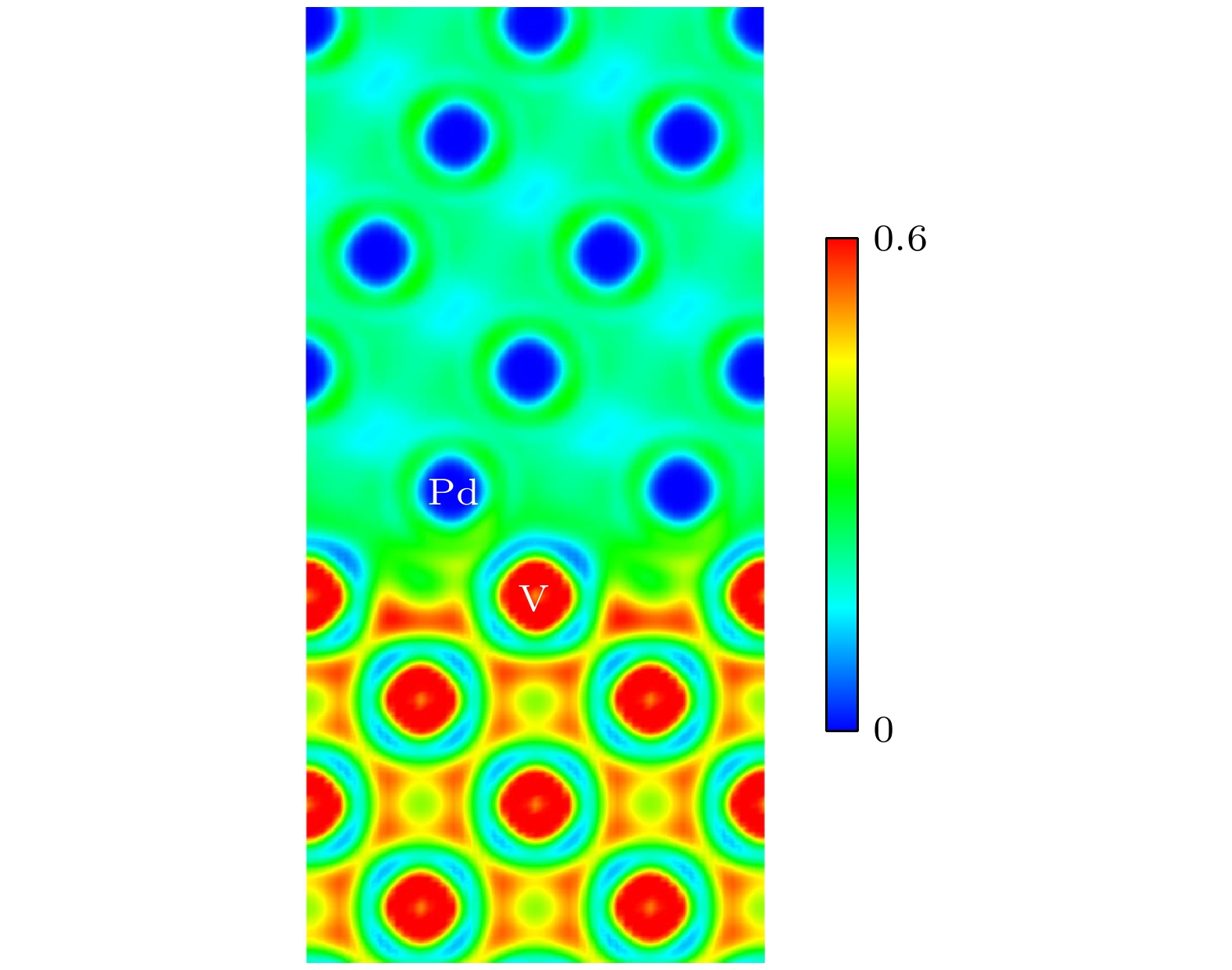
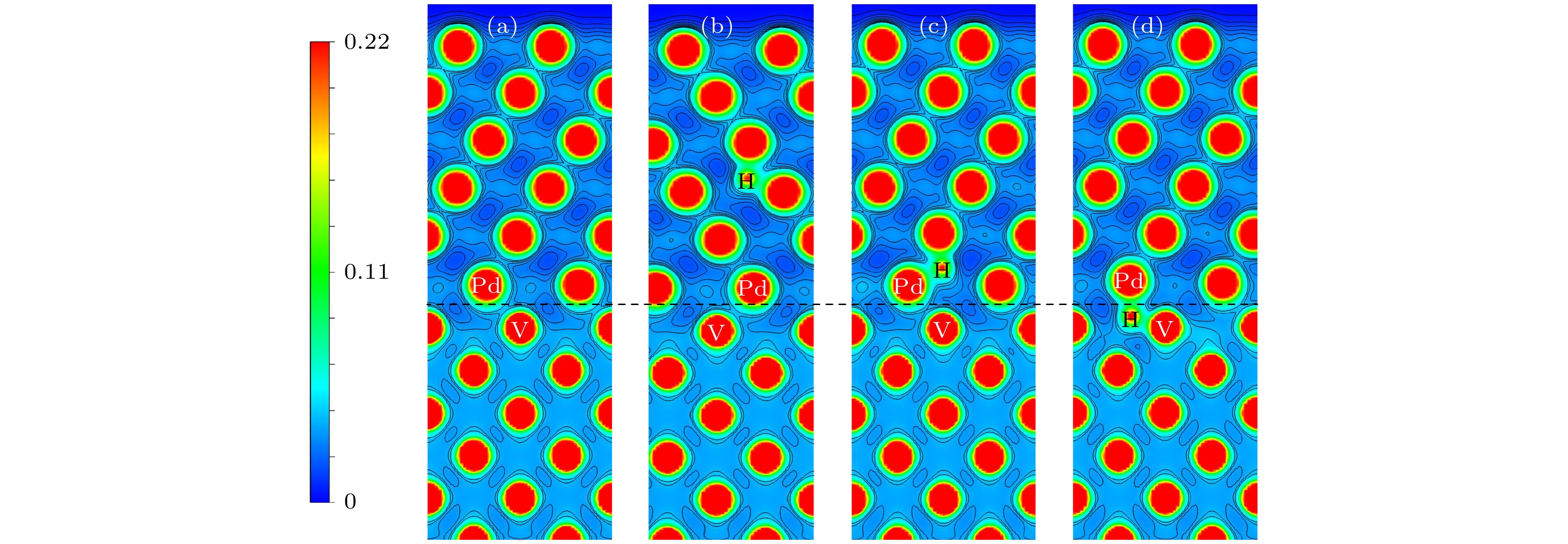
图 9 V/Pd界面的电荷密度 (a) 没有H原子的界面电荷密度图; (b)—(d) 分别为H原子在图5(a)中1, 7, 9位置的电荷密度图
Fig. 9. Charge density at V/Pd interface: (a) Interface charge density map without H atom; (b)–(d) the charge density maps of H atoms at positions 1, 7, and 9 in Fig. 5 (a), respectively.
图 10 (a) H在Pd掺杂界面的垂直扩散; (b) H在Fe掺杂界面的垂直扩散; (c) H垂直通过掺杂界面的迁移能量
Fig. 10. (a) Vertical diffusion of H at the Pd doping interface; (b) vertical diffusion of H at the Fe doping interface; (c) migration energy of H through the doping interface vertically.
图 11 (a) Pd向V空位的扩散; (b) Pd向Pd掺杂界面中V空位的扩散; (c) Pd向Fe掺杂界面中V空位的扩散; (d) Pd向V空位的扩散能垒
Fig. 11. (a) Diffusion of Pd to V vacancy; (b) diffusion of Pd to V vacancy in Pd doped interface; (c) diffusion of Pd to V vacancy in Fe doped interface; (d) diffusion energy barrier of Pd to V vacancy.
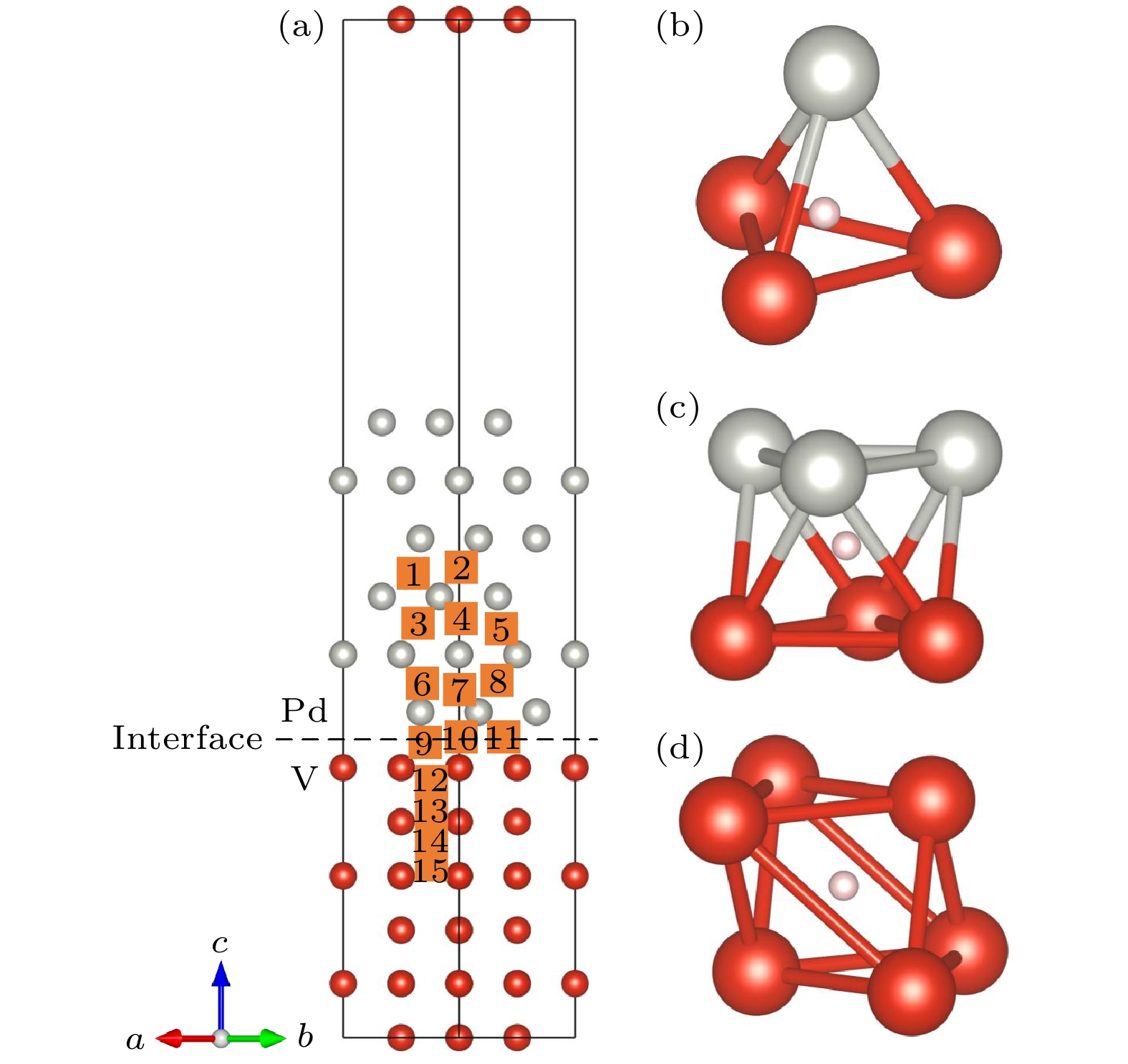
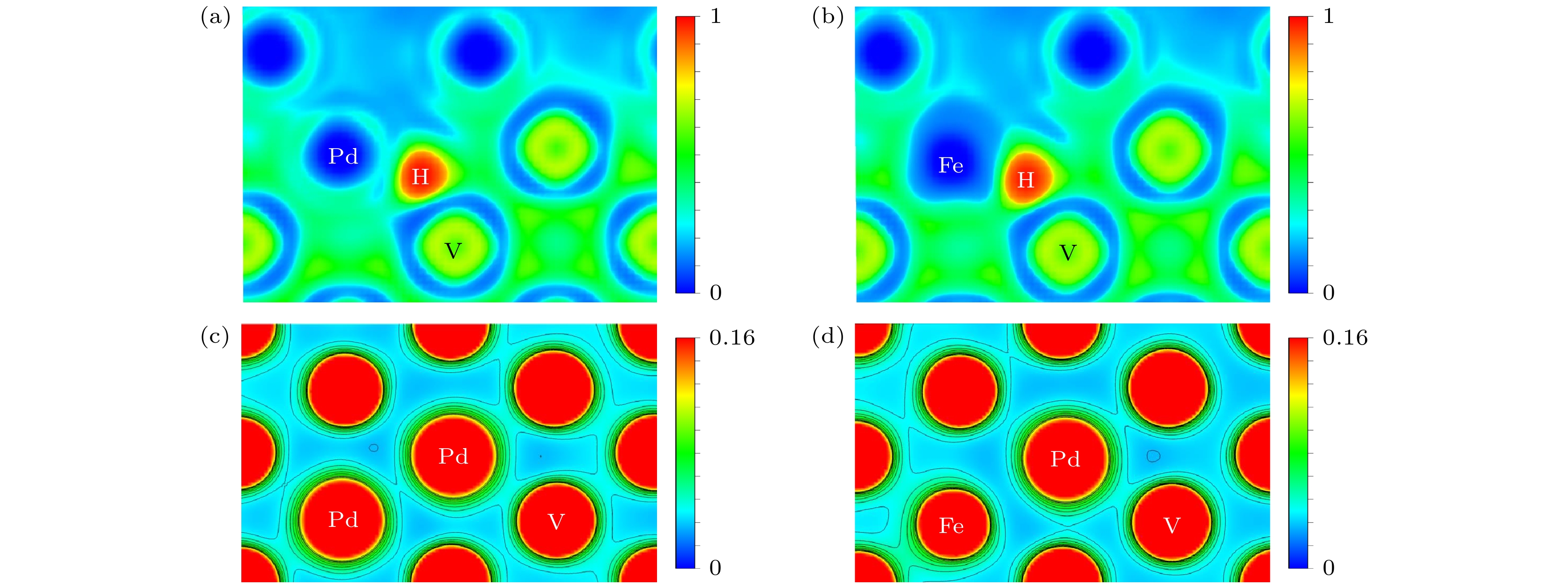
图 12 (a) H在Pd掺杂界面S4位置的电子局域函数图; (b) H在Fe掺杂界面S4位置的电子局域函数图; (c) Pd在Pd掺杂V基体空位的电荷密度图; (d) Pd在Fe掺杂V基体空位的电荷密度图
Fig. 12. (a) Electronic local function diagram of H at S4 position of Pd doping interface; (b) electronic local function diagram of H at S4 position of Fe doping interface; (c) charge density diagram of Pd in Pd-doped V matrix vacancies; (d) charge density diagram of Pd in Fe-doped V matrix vacancies.
图 13 (a) 界面拉应力随应变的变化; (b) V(110)面与Pd(111)面的拉应力随应变的变化
Fig. 13. (a) Change of interface tensile stress with strain; (b) change of tensile stress with strain on V(110) plane and Pd(111) plane.
表 1 不同厚度的V(110)面表面能
Table 1. Surface energy of V(110) surface with different thickness.
Number of atomic
layers of V (Pd)Surface energy/(J·m–2) V(110) Pd(111) 2(3) 2.42 1.434 4(6) 2.36 1.363 6(9) 2.37 1.361 8(12) 2.35 1.362 10(15) 2.34 1.361 
下载: 导出CSV
-
[1] Li Q, Garcia-Muelas R, Lopez N 2018 Nat. Commun. 9 526
Google Scholar
[2] Kim T, Song Y, Kang J, Kim S K, Kim S 2022 Int. J. Hydrogen Energy 47 24817
Google Scholar
[3] Agnolin S, Melendez J, Di Felice L, Gallucci F 2022 Int. J. Hydrogen Energy 47 28505
Google Scholar
[4] Park S B, Nam G H, Park Y I 2022 Thin Solid Films 757 139391
Google Scholar
[5] Dolan M D 2010 J. Membr. Sci. 362 12
Google Scholar
[6] Kozhakhmetov S, Sidorov N, Piven V, Sipatov I, Gabis I, Arinov B 2015 J. Alloys Compd. 645 S36
Google Scholar
[7] Dolan M D, Viano D M, Langley M J, Lamb K E 2018 J. Membr. Sci. 549 306
Google Scholar
[8] Zhang S, Zhang Z, Li J, Tu R, Shen Q, Wang C, Luo G, Zhang L 2020 J. Wuhan Univ. Technol. 35 879
Google Scholar
[9] Ko W S, Jeon J B, Shim J H, Lee B J 2012 Int. J. Hydrogen Energy 37 13583
Google Scholar
[10] Fasolin S, Barison S, Agresti F, Battiston S, Fiameni S, Isopi J, Armelao L 2022 Membranes (Basel, Switz.) 12 16
Google Scholar
[11] Alimov V N, Busnyuk A O, Notkin M E, Livshits A I 2014 J. Membr. Sci. 457 103
Google Scholar
[12] Dai J H, Xie R W, Chen Y Y, Song Y 2015 Phys. Chem. Chem. Phys. 17 16594
Google Scholar
[13] Il Jeon S, Park J H, Magnone E, Lee Y T, Fleury E 2012 Curr. Appl. Phys. 12 394
Google Scholar
[14] Ko W S, Oh J Y, Shim J H, Suh J Y, Yoon W Y, Lee B J 2014 Int. J. Hydrogen Energy 39 12031
Google Scholar
[15] Qin J Y, Wang Z M, Wang D H, Wang F, Yan X F, Zhong Y, Hu C H, Zhou H Y 2019 J. Alloys Compd. 805 747
Google Scholar
[16] Qin J Y, Hao C Y, Wang D H, Wang F, Yan X F, Zhong Y, Wang Z M, Hu C H, Wang X T 2020 J. Adv. Res. 21 25
Google Scholar
[17] Mills G, Jonsson H, Schenter G K 1995 Surf. Sci. 324 305
Google Scholar
[18] Henkelman G, Jonsson H 2000 J. Chem. Phys. 113 9978
Google Scholar
[19] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[20] Blochl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[21] Qin J Y, Liu Z G, Zhao W, Wang D A H, Zhang Y L, Zhong Y, Zhang X H, Wang Z M, Hu C H, Liu J W 2021 Materials 14 12
Google Scholar
[22] Mills G, Schenter G K, Makarov D E, Jonsson H 1997 Chem. Phys. Lett. 278 91
Google Scholar
[23] Mills G, Jonsson H 1994 Phys. Rev. Lett. 72 1124
Google Scholar
[24] Chen J F, Hu L, Tang T 2022 J. Phys. Chem. C 126 7468
Google Scholar
[25] Castleton C W M, Hoglund A, Gothelid M, Qian M C, Mirbt S 2013 Phys. Rev. B 88 7
Google Scholar
[26] Zhang H L, Wang J J, Huang W J, Wang L Q, Lu Z B 2022 Surf. Interfaces 30 10
Google Scholar
[27] Rose J H, Ferrante J, Smith J R 1981 Phys. Rev. Lett. 47 675
Google Scholar
[28] Jin N, Yang Y Q, Li J, Luo X, Huang B, Sun Q, Guo P F 2014 J. Appl. Phys. 115 11
Google Scholar
[29] Lu T, Chen Q X 2018 Acta Phys. Chim. Sin. 34 503
Google Scholar
[30] Wang J W, Song M, He Y H, Gong H R 2016 J. Membr. Sci. 503 124
Google Scholar
[31] Kang S G, Coulter K E, Gade S K, Way J D, Sholl D S 2011 J. Phys. Chem. Lett. 2 3040
Google Scholar
[32] Yang L, Wirth B D 2020 J. Appl. Phys. 127 12
Google Scholar
[33] Puska M J, Nieminen R M, Manninen M 1981 Phys. Rev. B 24 3037
Google Scholar
[34] Ko W S, Shim J H, Jung W S, Lee B J 2016 J. Membr. Sci. 497 270
Google Scholar
下载:
计量
- 文章访问数: 2638
- PDF下载量: 70
- 被引次数: 0
