-
To meet the demands for various applications in optical filtering and microwave signal processing, integrated silicon photonic filters are required to be multifunctional, reconfigurable and tunable. In this work, an integrated multi-functional optical filter is proposed, which is designed based on a tunable Sagnac loop reflector and a microring resonator. The through port and drop port of an add-drop microring resonator are connected with the two ports of a tunable reflector. By controlling the thermal phase shifters in different scenarios, the device can be reconfigured into a reflective-type asymmetric Mach-Zehnder interferometer filter, a reflective-type all-pass microring resonator filter and self-interference microring resonator filters. An analytical model is established based on the transfer matrix. The simulation results show that the device can achieve the following functions: sinusoidal spectral filtering with four different free spectral ranges, Lorentzian spectral filtering toggling between band pass and band stop, and spectral reconfigurations of Fano resonance, electromagnetically induced transparency, and electromagnetically induced absorption. Each spectrum mentioned above can be tuned fast and widely. Reflection provides a new degree of freedom in design, breaks through the inherent footprint limit, and achieves a wide range of free spectral ranges. Our proposed tunable Sagnac loop reflector assisted microring resonator provides a new scheme for realizing flexible, tunable and multi-functional reconfigurable integrated photonic filters, and has broad applications in the integrated photonic analog signal processing and microwave photonics.
-
Keywords:
- integrated optics /
- tunable reflector /
- microring resonator /
- reconfigurable optical filter
[1] Won R 2010 Nat. Photonics 4 498
Google Scholar
[2] Marpaung D, Yao J P, Capmany J 2019 Nat. Photonics 13 80
Google Scholar
[3] Xiang C, Jin W, Bowers J E 2022 Photonics Res. 10 A82
Google Scholar
[4] Bogaerts W, de Heyn P, Van Vaerenbergh T, de Vos K, Kumar Selvaraja S, Claes T, Dumon P, Bienstman P, van Thourhout D, Baets R 2012 Laser Photonics Rev. 6 47
Google Scholar
[5] Ong J R, Kumar R, Mookherjea S 2013 IEEE Photonics Technol. Lett. 25 1543
Google Scholar
[6] Kumar R R, Tsang H K 2021 Opt. Lett. 46 134
Google Scholar
[7] 任光辉, 陈少武, 曹彤彤 2012 61 034215
Google Scholar
Ren G H, Chen S W, Cao T T 2012 Acta Phys. Sin. 61 034215
Google Scholar
[8] Cohen R A, Amrani O, Ruschin S 2018 Nat. Photonics 12 706
Google Scholar
[9] Sun Q K, Zhou L J, Lu L J, Zhou G Q, Chen J P 2018 IEEE Photonics J. 10 1
Google Scholar
[10] Zheng P F, Xu X M, Hu G H, Zhang R H, Yun B F, Cui Y P 2021 J. Light. Technol. 39 1429
Google Scholar
[11] Geng Z H, Xie Y W, Zhuang L M, Burla M, Hoekman M, Roeloffzen C G H, Lowery A J 2017 Opt. Express 25 27635
Google Scholar
[12] Li A, Bogaerts W 2019 Laser Photonics Rev. 13 1800244
Google Scholar
[13] Li A, Bogaerts W 2017 Opt. Express 25 31688
Google Scholar
[14] Pan S L, Tang Z Z, Huang M H, Li S M 2020 IEEE J. Sel. Top. Quantum Electron. 26 1
Google Scholar
[15] Shu H W, Chang L, Tao Y S, Shen B T, Xie W Q, Jin M, Netherton A, Tao Z H, Zhang X G, Chen R X, Bai B W, Qin J, Yu S H, Wang X J, Bowers J E 2022 Nature 605 457
Google Scholar
[16] Jiang X H, Wu J Y, Yang Y X, Pan T, Mao J M, Liu B Y, Liu R L, Zhang Y, Qiu C Y, Tremblay C, Su Y K 2016 Opt. Express. 24 2183
Google Scholar
[17] El Shamy R S, Afifi A E, Badr M M, Swillam M A 2022 Sci. Rep. 12 3598
Google Scholar
[18] 田赫, 掌蕴东, 白岩 2017 光学精密工程 25 59
Google Scholar
Tian H, Zhang Y D, Bai Y 2017 Opt. Precis. Eng. 25 59
Google Scholar
[19] Smith D D, Chang H, Fuller K A, Rosenberger A T, Boyd R W 2004 Phys. Rev. A 69 063804
Google Scholar
[20] Naweed A 2015 Opt. Express 23 12573
Google Scholar
[21] 焦新泉, 陈家斌, 王晓丽, 薛晨阳, 任勇峰 2015 64 144202
Google Scholar
Jiao X Q, Chen J B, Wang X L, Xue C Y, Ren Y F 2015 Acta Phys. Sin. 64 144202
Google Scholar
[22] Feng J B, Li Q Q, Zhou Z P 2011 IEEE Photonics Technol. Lett. 23 79
Google Scholar
[23] 鹿利单, 祝连庆, 曾周末, 崔一平, 张东亮, 袁配 2021 70 034204
Google Scholar
Lu L D, Zhu L Q, Zeng Z M, Cui Y P, Zhang D L, Yuan P 2021 Acta Phys. Sin. 70 034204
Google Scholar
[24] Ma K, Zhang Y D, Wu Y F, Su H Y, Zhang X N, Yuan P 2017 J. Opt. Soc. Am. B 34 2400
Google Scholar
[25] Tian H, Zhang Y D 2018 J. Light. Technol. 36 1792
Google Scholar
[26] Wang C, Zhang M, Yu M J, Zhu R R, Hu H, Loncar M 2019 Nat. Commun. 10 978
Google Scholar
-
图 1 可调反射器辅助的MRR滤波器结构 (SLR, Sagnac环路反射器; PS, 热光移相器; MMI, 多模干涉)
Fig. 1. Diagram of the tunable reflector assisted MRR filter (SLR, Sagnac loop reflector; PS, phase shifter; MMI, multi-mode interference).
图 2 (a) 可调反射器辅助的MRR简化结构; (b) MZI型可调耦合器结构; (c) 光在图(a)中传输的等效路径
Fig. 2. (a) Simplified diagram of a tunable reflector assisted MRR filter; (b) diagram of an optical tunable coupler based on the MZI structure; (c) equivalent paths of optical transmission in panel (a).
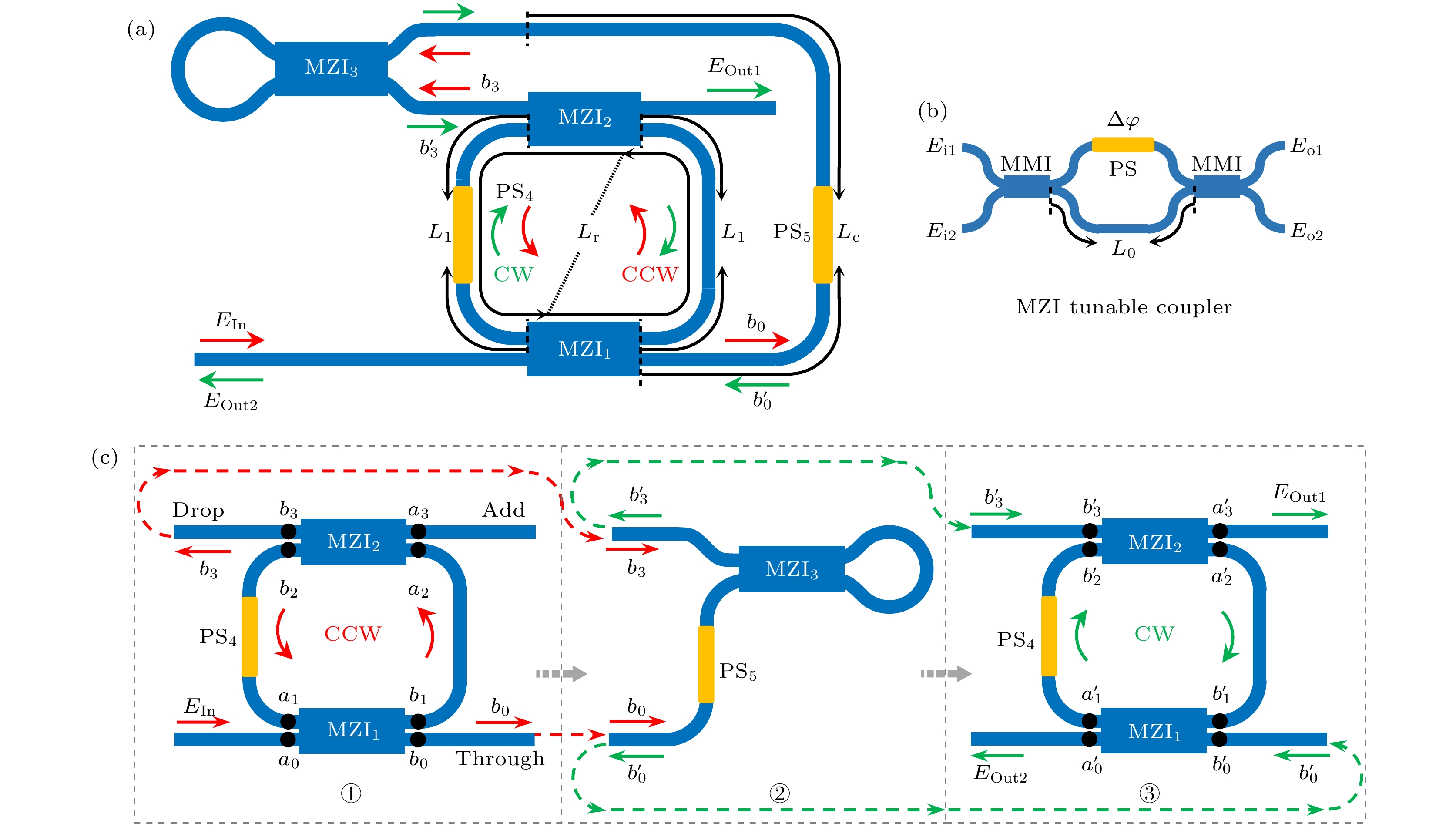
图 3 反射式非对称MZI滤波器结构 (a) MZI1处于可调状态且MZI2处于交叉状态; (b) MZI1处于交叉状态且MZI2处于可调状态; (c) 等效反射式非对称MZI结构
Fig. 3. Diagram of reflective-type asymmetric MZI filters: (a) MZI1 is in tunable state and MZI2 is in cross state; (b) MZI1 is in cross state and MZI2 is in tunable state; (c) schematic of the equivalent reflective-type asymmetric MZI.
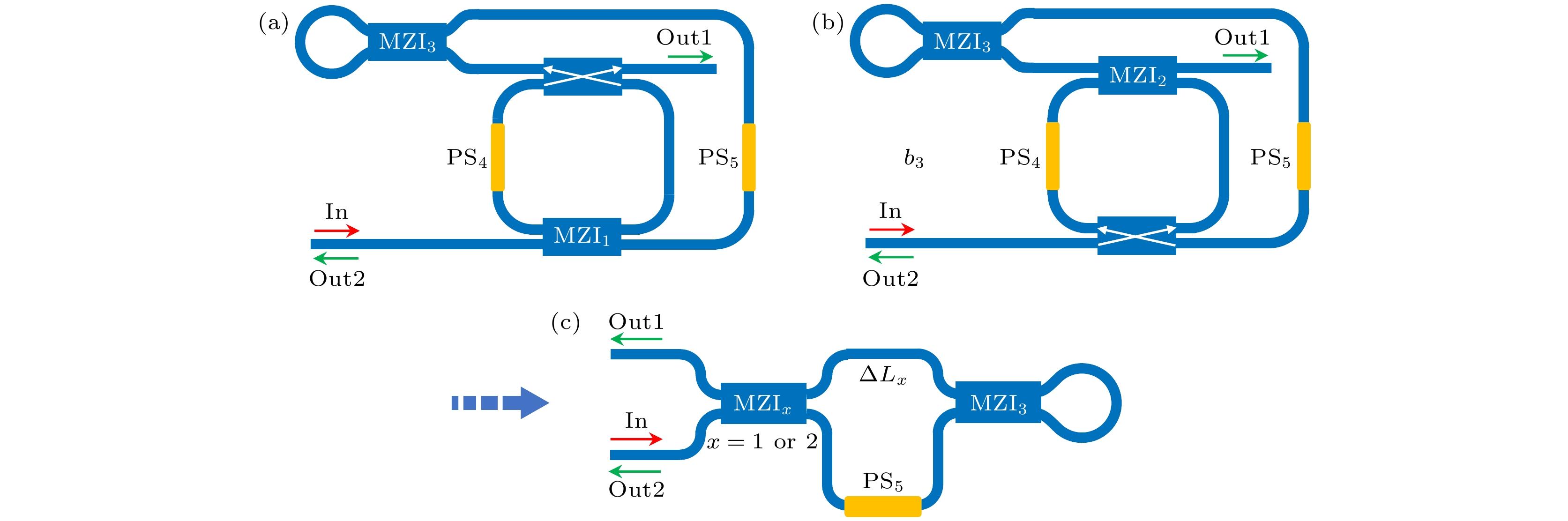
图 4 四种FSR的反射式非对称MZI滤波器频谱 (a) FSR = ∆λ, Φ = [0.50, 0, 0.50, 0, 0]×π; (b) FSR = 2∆λ, Φ = [0.25, 0, 0.25, 0, 0]×π;(c) FSR = ∆λ/3, Φ = [0, 0.50, 0.50, 0, 0]×π; (d) FSR = 2∆λ/3, Φ = [0, 0.25, 0.25, 0, 0]×π; (e) 频谱调谐及对应的相位变化量
Fig. 4. Reflective-type asymmetric MZI filters spectra with four different FSRs: (a) FSR = ∆λ, Φ = [0.50, 0, 0.50, 0, 0]×π; (b) FSR = 2∆λ, Φ = [0.25, 0, 0.25, 0, 0]×π; (c) FSR = ∆λ/3, Φ = [0, 0.50, 0.50, 0, 0]×π; (d) FSR = 2∆λ/3, Φ = [0, 0.25, 0.25, 0, 0]×π; (e) the spectrum tuning and corresponding phase change.
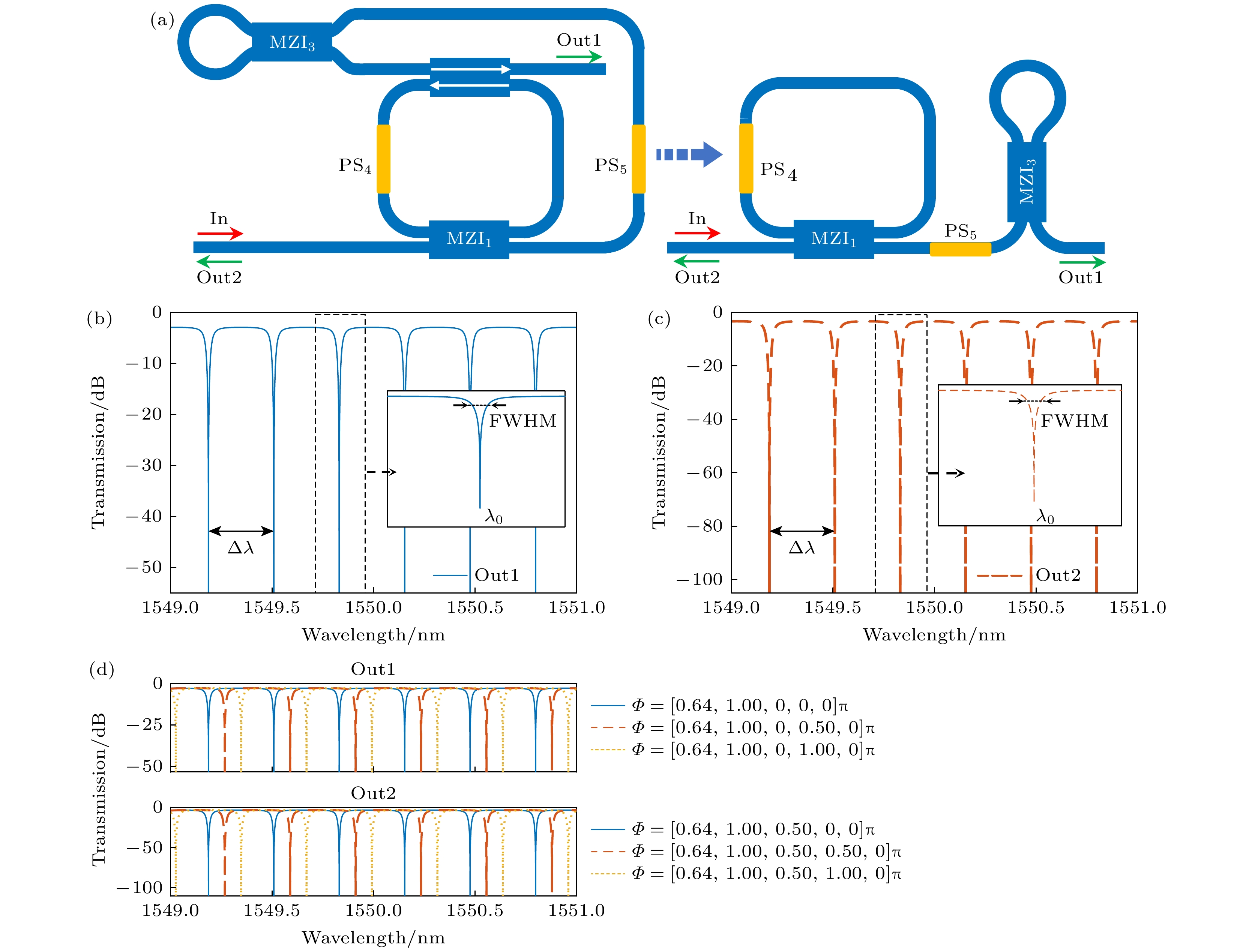
图 5 (a) 反射式全通型微环滤波器及等效结构示意图; (b) 反射器处于透射状态时(Φ = [0.64, 1.00, 0, 0, 0]×π), Out1的陷波响应;(c) 反射器处于反射状态时(Φ = [0.64, 1.00, 0.50, 0, 0]×π), Out2的陷波响应; (d) 频谱调谐及对应的相位变化量
Fig. 5. (a) Diagram of a reflective-type all-pass MMR filter and equivalent structure; (b) notch response of the Out1 port when the reflector is in transmission state (Φ = [0.64, 1.00, 0, 0, 0]×π); (c) notch response of the Out2 port when the reflector is in reflection state (Φ = [0.64, 1.00, 0.50, 0, 0]×π); (d) spectrum tuning and corresponding phase change.
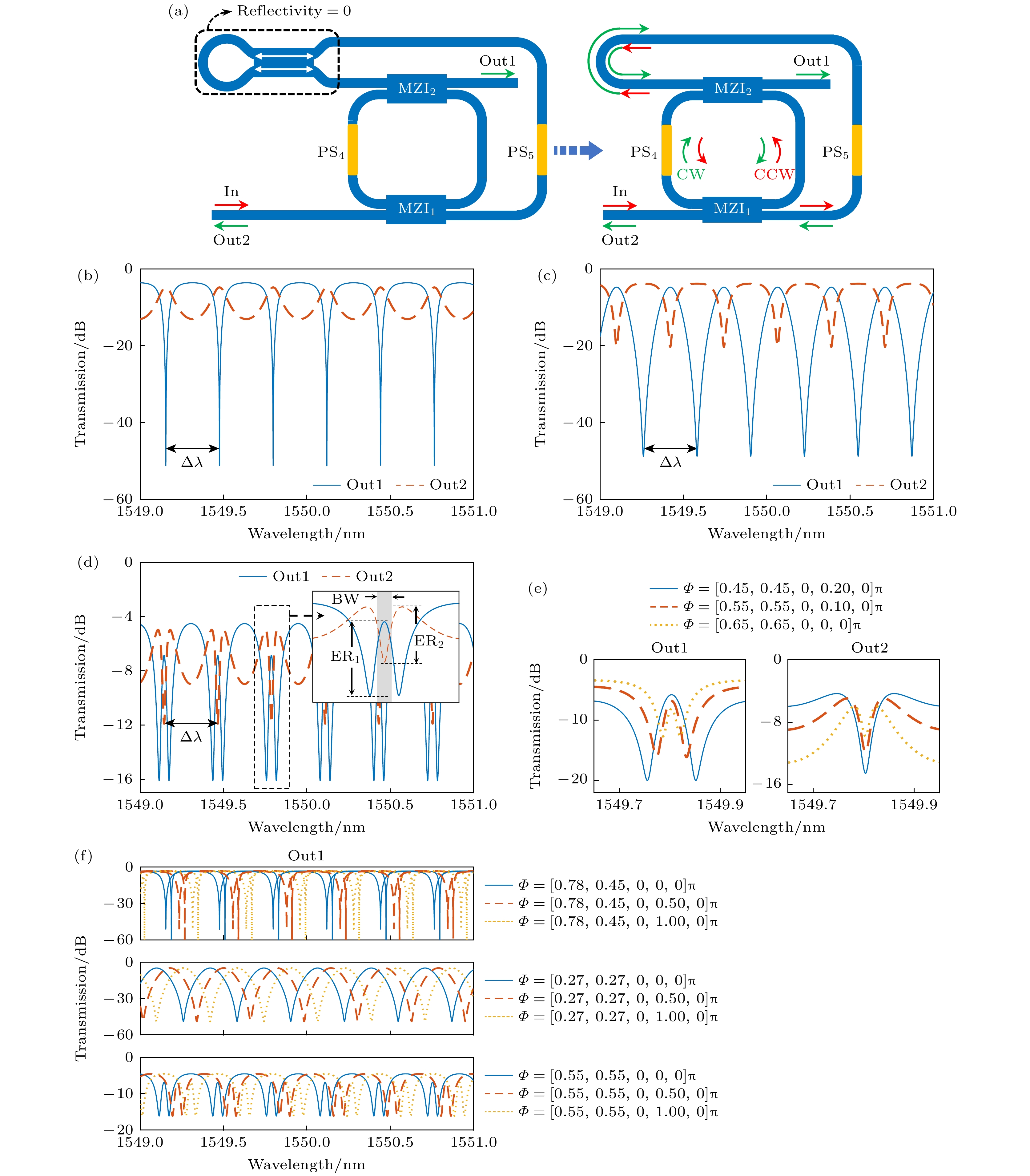
图 6 (a) 直通型自干涉微环滤波器及等效结构示意图; (b) Out1带阻与Out2带通响应(Φ = [0.78, 0.45, 0, 0, 0]×π); (c) Out1带通与Out2带阻响应(Φ = [0.27, 0.27, 0, 0, 0]×π); (d) EIT与EIA频谱(Φ = [0.55, 0.55, 0, 0, 0]×π); (e) EIT与EIA频谱调谐(带宽与ER); (f) 频谱调谐及对应的相位变化量
Fig. 6. (a) Diagram of a self-interference MMR filter and equivalent structure; (b) band-stop response of the Out1 port and band-pass response of the Out2 port (Φ = [0.78, 0.45, 0, 0, 0]×π); (c) band-pass response of the Out1 port and band-stop response of the Out2 port (Φ = [0.27, 0.27, 0, 0, 0]×π); (d) spectrum of EIT and EIA (Φ = [0.55, 0.55, 0, 0, 0]×π); (e) spectrum tuning of EIT and EIA (bandwidth and ER); (f) spectrum tuning and corresponding phase change.
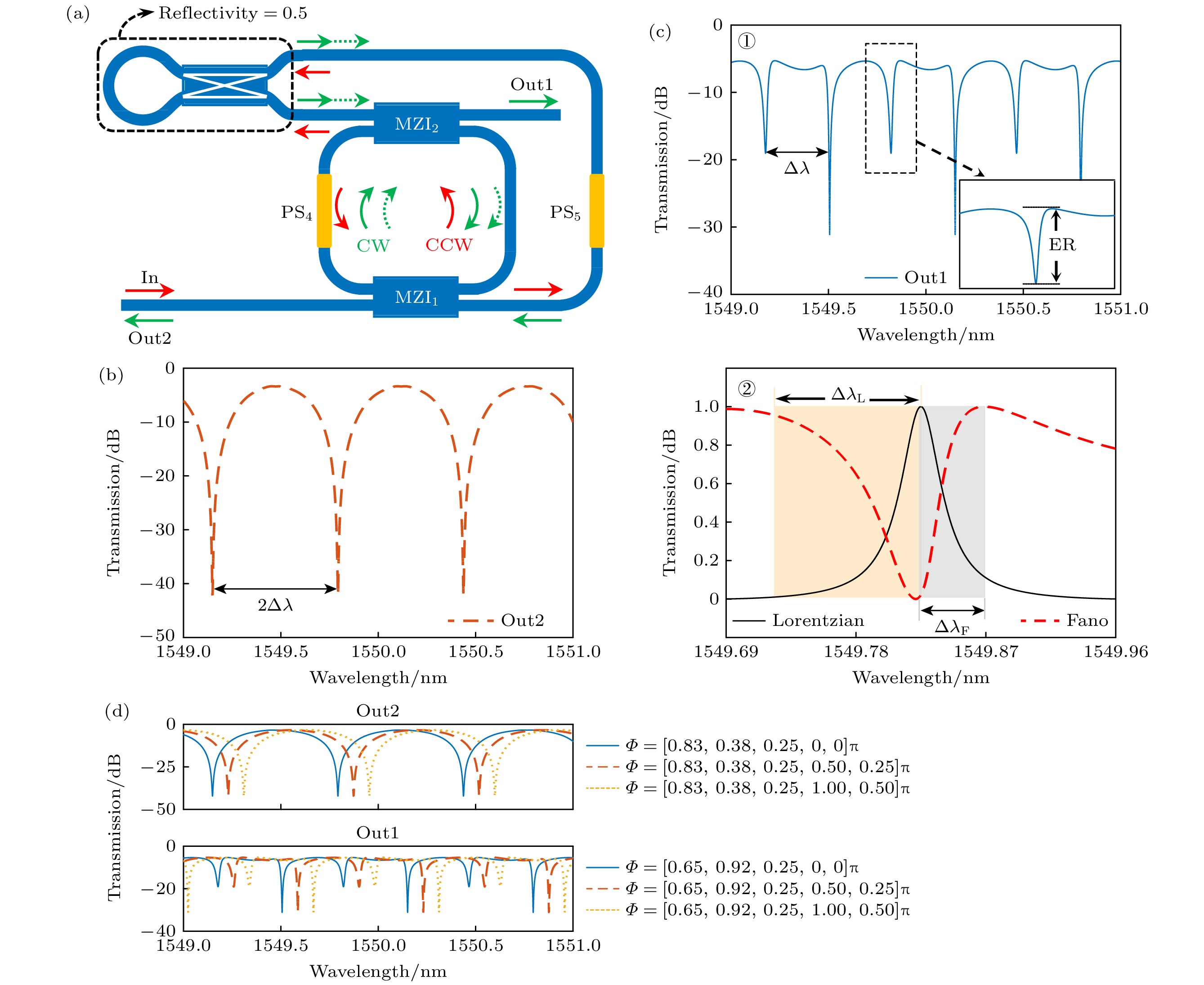
图 7 (a) 半透半反型自干涉微环滤波器结构; (b) 两倍FSR的带阻响应(Φ = [0.83, 0.38, 0.25, 0, 0]×π); (c) Fano共振频谱(Φ = [0.65, 0.92, 0.25, 0, 0]×π); (d) 频谱调谐以及对应的相位变化量
Fig. 7. (a) Structure of a semi-transparent and semi-reflective self-interference MMR filter; (b) band-stop response with doubled FSR (Φ = [0.83, 0.38, 0.25, 0, 0]×π); (c) spectrum of Fano resonance (Φ = [0.65, 0.92, 0.25, 0, 0]×π); (d) spectrum tuning and corresponding phase change.
-
[1] Won R 2010 Nat. Photonics 4 498
Google Scholar
[2] Marpaung D, Yao J P, Capmany J 2019 Nat. Photonics 13 80
Google Scholar
[3] Xiang C, Jin W, Bowers J E 2022 Photonics Res. 10 A82
Google Scholar
[4] Bogaerts W, de Heyn P, Van Vaerenbergh T, de Vos K, Kumar Selvaraja S, Claes T, Dumon P, Bienstman P, van Thourhout D, Baets R 2012 Laser Photonics Rev. 6 47
Google Scholar
[5] Ong J R, Kumar R, Mookherjea S 2013 IEEE Photonics Technol. Lett. 25 1543
Google Scholar
[6] Kumar R R, Tsang H K 2021 Opt. Lett. 46 134
Google Scholar
[7] 任光辉, 陈少武, 曹彤彤 2012 61 034215
Google Scholar
Ren G H, Chen S W, Cao T T 2012 Acta Phys. Sin. 61 034215
Google Scholar
[8] Cohen R A, Amrani O, Ruschin S 2018 Nat. Photonics 12 706
Google Scholar
[9] Sun Q K, Zhou L J, Lu L J, Zhou G Q, Chen J P 2018 IEEE Photonics J. 10 1
Google Scholar
[10] Zheng P F, Xu X M, Hu G H, Zhang R H, Yun B F, Cui Y P 2021 J. Light. Technol. 39 1429
Google Scholar
[11] Geng Z H, Xie Y W, Zhuang L M, Burla M, Hoekman M, Roeloffzen C G H, Lowery A J 2017 Opt. Express 25 27635
Google Scholar
[12] Li A, Bogaerts W 2019 Laser Photonics Rev. 13 1800244
Google Scholar
[13] Li A, Bogaerts W 2017 Opt. Express 25 31688
Google Scholar
[14] Pan S L, Tang Z Z, Huang M H, Li S M 2020 IEEE J. Sel. Top. Quantum Electron. 26 1
Google Scholar
[15] Shu H W, Chang L, Tao Y S, Shen B T, Xie W Q, Jin M, Netherton A, Tao Z H, Zhang X G, Chen R X, Bai B W, Qin J, Yu S H, Wang X J, Bowers J E 2022 Nature 605 457
Google Scholar
[16] Jiang X H, Wu J Y, Yang Y X, Pan T, Mao J M, Liu B Y, Liu R L, Zhang Y, Qiu C Y, Tremblay C, Su Y K 2016 Opt. Express. 24 2183
Google Scholar
[17] El Shamy R S, Afifi A E, Badr M M, Swillam M A 2022 Sci. Rep. 12 3598
Google Scholar
[18] 田赫, 掌蕴东, 白岩 2017 光学精密工程 25 59
Google Scholar
Tian H, Zhang Y D, Bai Y 2017 Opt. Precis. Eng. 25 59
Google Scholar
[19] Smith D D, Chang H, Fuller K A, Rosenberger A T, Boyd R W 2004 Phys. Rev. A 69 063804
Google Scholar
[20] Naweed A 2015 Opt. Express 23 12573
Google Scholar
[21] 焦新泉, 陈家斌, 王晓丽, 薛晨阳, 任勇峰 2015 64 144202
Google Scholar
Jiao X Q, Chen J B, Wang X L, Xue C Y, Ren Y F 2015 Acta Phys. Sin. 64 144202
Google Scholar
[22] Feng J B, Li Q Q, Zhou Z P 2011 IEEE Photonics Technol. Lett. 23 79
Google Scholar
[23] 鹿利单, 祝连庆, 曾周末, 崔一平, 张东亮, 袁配 2021 70 034204
Google Scholar
Lu L D, Zhu L Q, Zeng Z M, Cui Y P, Zhang D L, Yuan P 2021 Acta Phys. Sin. 70 034204
Google Scholar
[24] Ma K, Zhang Y D, Wu Y F, Su H Y, Zhang X N, Yuan P 2017 J. Opt. Soc. Am. B 34 2400
Google Scholar
[25] Tian H, Zhang Y D 2018 J. Light. Technol. 36 1792
Google Scholar
[26] Wang C, Zhang M, Yu M J, Zhu R R, Hu H, Loncar M 2019 Nat. Commun. 10 978
Google Scholar
下载:
计量
- 文章访问数: 5045
- PDF下载量: 135
- 被引次数: 0
