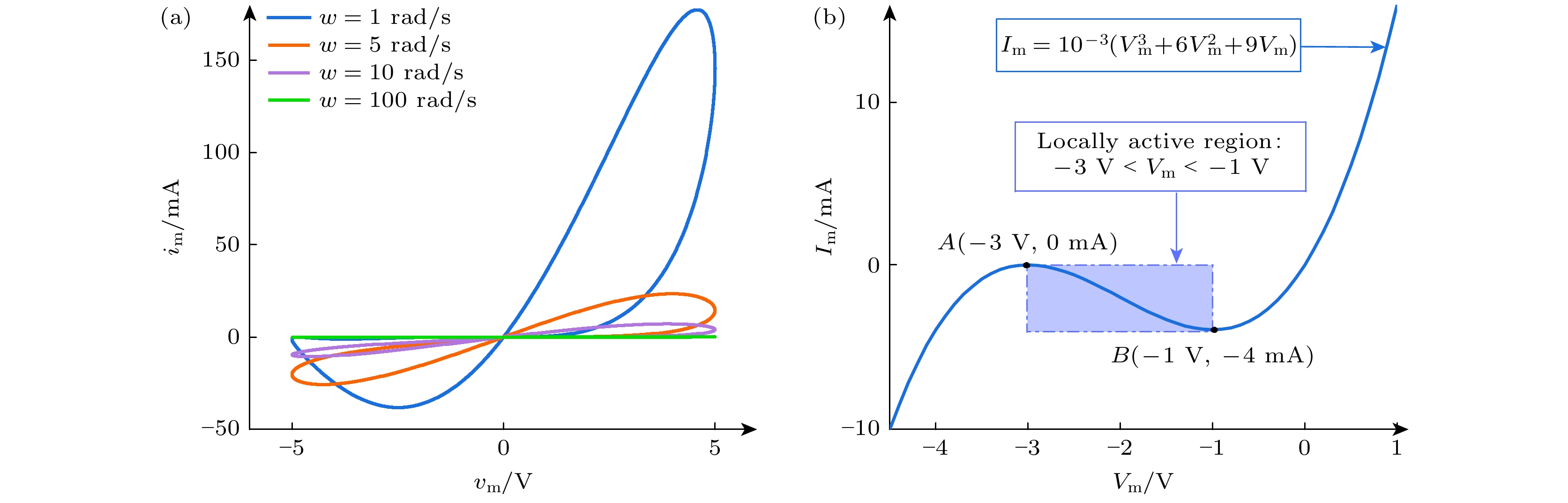
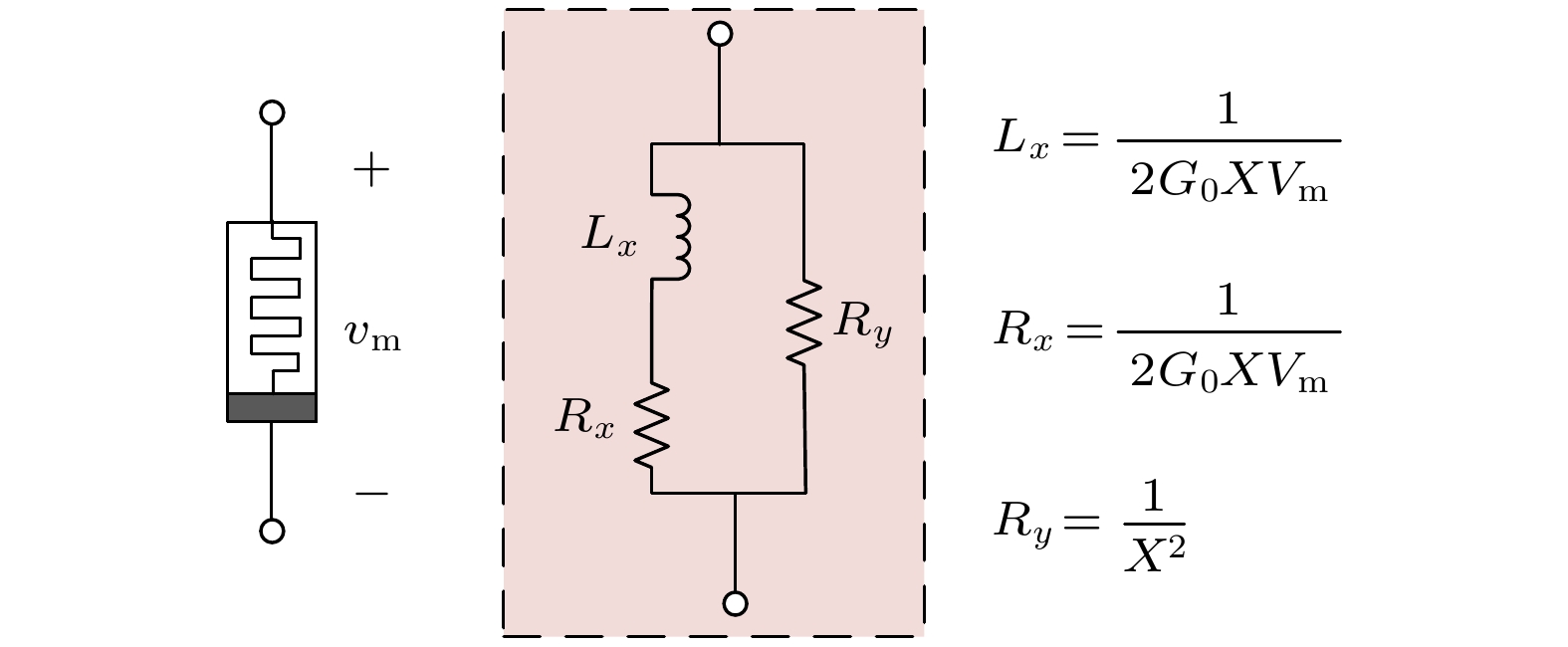
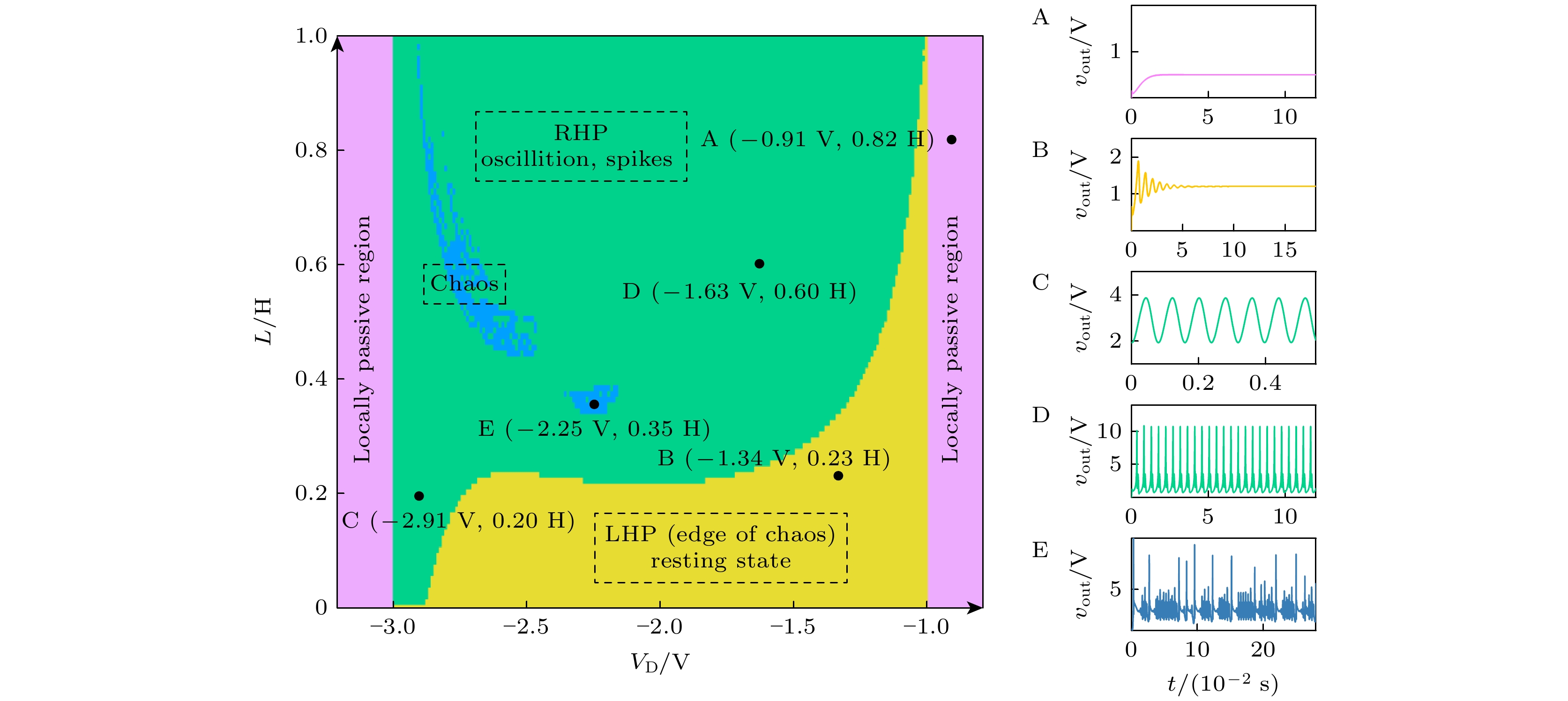
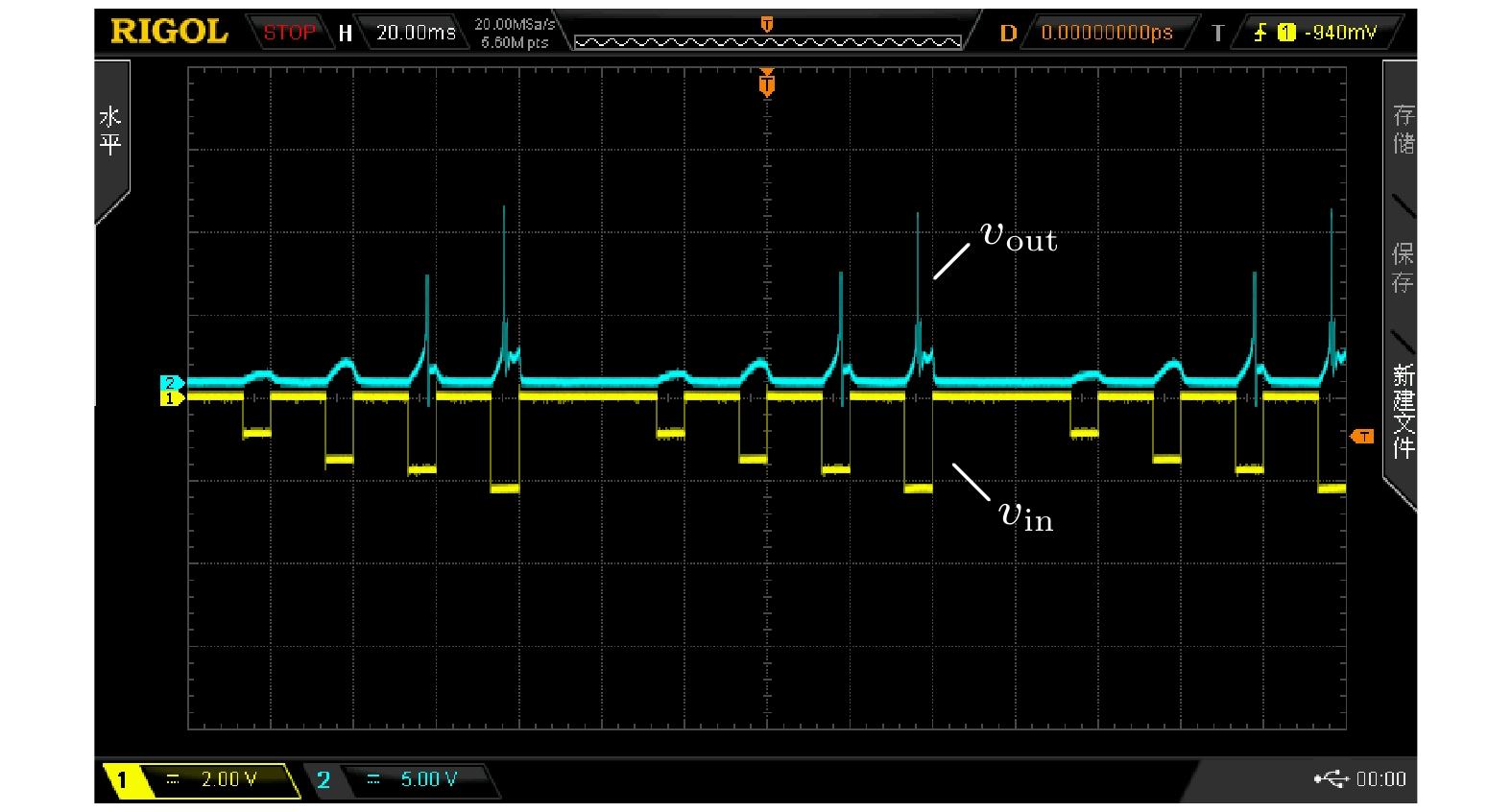
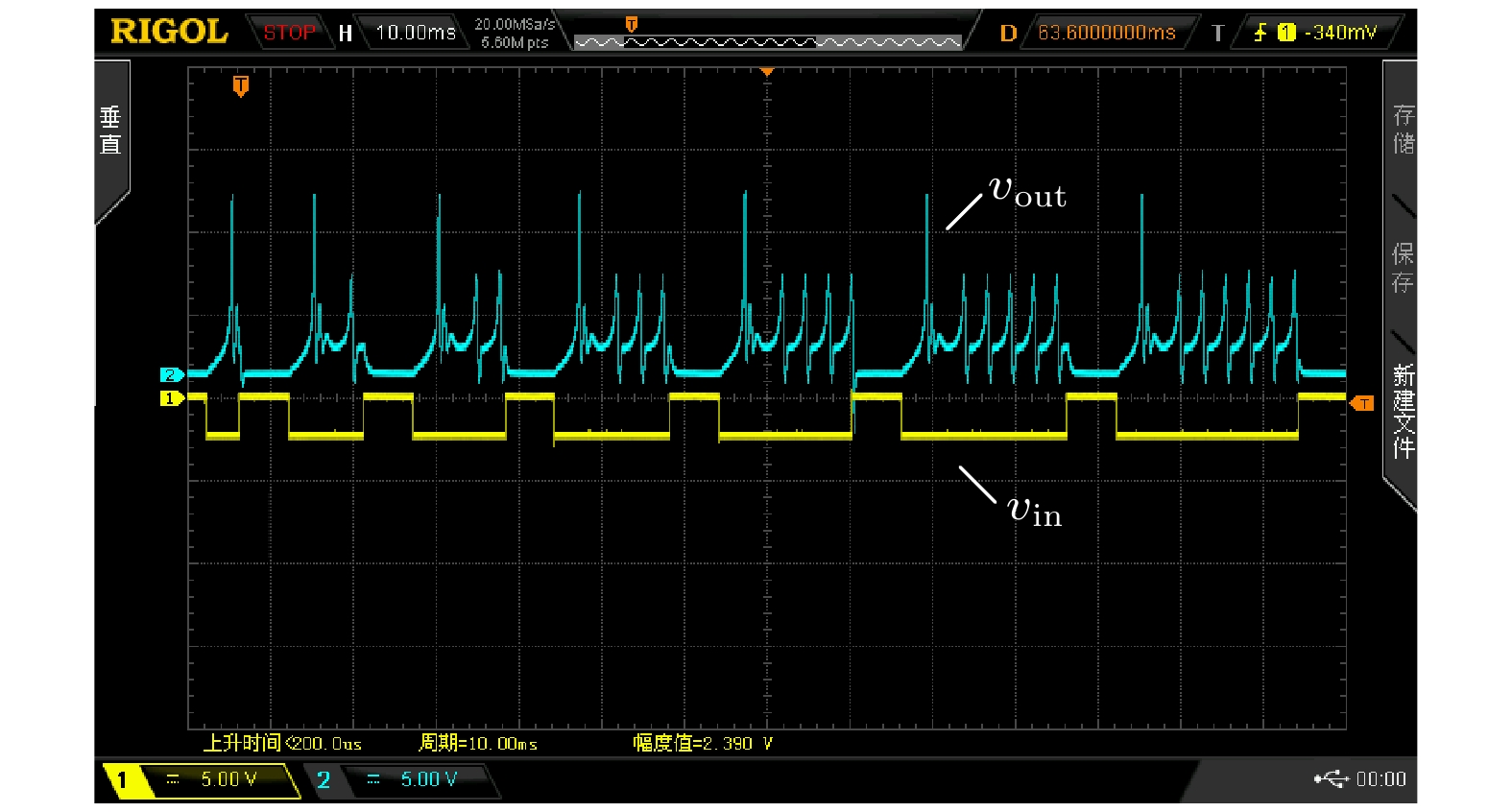
-
蔡氏结型忆阻器(Chua corsage memristor, CCM)属于压控型局部有源忆阻器, 具有复杂的动力学行为, 在神经形态计算领域具有潜在的应用价值. 根据静态电压-电流特性曲线, CCM可分为二翼、四翼和六翼型. 本文基于神经形态行为的产生机制, 将CCM的数学模型进行简化, 简化后的模型表达式中无绝对值符号, 且小信号等效电路的导纳函数与简化前完全相同. 进一步采用简化的CCM模型与电容和电感元件相连, 构建了三阶神经元电路. 利用局部有源、混沌边缘、及李雅普诺夫指数等理论分析方法, 预测了该神经元电路产生神经形态行为的参数域. 根据简化的CCM数学模型, 采用运算放大器、乘法器、电阻和电容等常用电路元件构建了该忆阻器的电路仿真器, 并连接电容和电感进一步给出了神经元电路的硬件实现. 实验结果表明该神经元电路可以产生丰富的神经形态行为, 包括静息状态、周期尖峰、混沌状态、双峰响应、周期振荡现象、全或无现象和尖峰簇发现象.
The Chua corsage memristor (CCM) is a voltage-controlled locally-active memristor, which has complex dynamic behaviors and potential applications in the field of neuromorphic computing. According to the DC V-I plot, the CCM can be classified as two-lobe, four-lobe, and six-lobe type. By analyzing their non-volatility and local activity, it is found that they have the same locally-active region and a common stable equilibrium. The mathematical models of the three CCMs are simplified based on the mechanism of neuromorphic behavior, namely, local activity. After the model simplification, the absolute value operation disappears, but the locally-active domain remains unchanged. For the simplified CCM, its small-signal equivalent circuit at the locally-active operating point is established, which is consistent with CCMs before being simplified. Hence, the model simplification does not change the small-signal characteristics of CCMs. To further investigate the application of voltage-controlled locally-active memristor in modeling the neuromorphic behavior of neurons, the simplified CCM model is used to connect a capacitor and an inductor to construct a third-order neuron circuit. By applying theoretical analysis methods such as local activity, edge of chaos, and Lyapunov exponents, we predict the parameter domains where different neuromorphic behaviors are generated. The distribution of neuromorphic behaviors is described on a dynamic map determined by the parameters of applied voltage VD and external inductance L. When the memristor is biased in the locally-active region, the system response changes among resting state, periodic spiking oscillation, and chaotic behaviors. Finally, according to the simplified CCM mathematical model, the corresponding emulator circuit is designed by using three operational amplifiers, two multipliers, a current conveyor, and several resistors and capacitors. Based on the presented memristor emulator circuit, the hardware implementation of the neuron circuit is given. The experimental results verify the correctness and feasibility of the simplified CCM emulator circuit, and show that the simplified CCM-based neuron circuit can produce a variety of neuromorphic behaviors, including resting state, periodic spiking, chaotic state, bimodal response, periodic oscillation, all-or-nothing phenomenon, and spike clustering phenomenon. We expect that this work is helpful in further studying the mechanism of neuromorphic behaviors of the neuron circuit and its practical applications. -
Keywords:
- memristor /
- local activity /
- neuron circuit /
- chaos /
- hardware implementation
[1] Nawrocki R A, Voyles R M, Shaheen S E 2016 IEEE Trans. Electron Devices 63 3819
Google Scholar
[2] Cassidy A S, Georgiou J, Andreou A G 2013 Neural Netw. 45 4
Google Scholar
[3] Shrestha A, Fang H W, Mei Z D, Rider D P, Wu Q, Qiu Q R 2022 IEEE Circuits Syst. Mag. 22 6
Google Scholar
[4] Shen Z J, Zhao C, Yang L, Zhao C Z 2020 International SoC Design Conference (ISOCC) Yeosu, Korea (South), October 21–24, 2020 p163
[5] Babacan Y, Kaçar F, Gürkan K 2016 Neurocomputing 203 86
Google Scholar
[6] Liu Y, Iu H H C, Qian Y H 2021 IEEE Trans. Circuits Syst. Express Briefs 68 2982
Google Scholar
[7] Chatterjee D, Kottantharayil A 2019 IEEE Electron Device Lett. 40 1301
Google Scholar
[8] Kim S, Du C, Sheridan P, Ma W, Choi S, Lu W D 2015 Nano Lett. 11 2203
Google Scholar
[9] Weiher M, Herzig M, Tetzlaff R, Ascoli A, Mikolajick T, Slesazeck S 2019 IEEE Trans. Circuits Syst. Regul. Pap. 66 2627
Google Scholar
[10] Chua L O 2013 Nanotechnology 24 383001
Google Scholar
[11] Kumar S, Strachan J P, Williams R S 2017 Nature 548 318
Google Scholar
[12] Yi W, Tsang K K, Lam S K, Bai X W, Crowell J A, Flores E A 2018 Nat. Commun. 9 4661
Google Scholar
[13] 李国林 2017 电子电路与系统基础 (北京: 清华大学出版社) 第61页
Li G L 2017 Foundations of Electronic Circuits and Systems (Beijing: Tsinghua University Press) p61 (in Chinese)
[14] 王世场, 卢振洲, 梁燕, 王光义 2022 71 050502
Google Scholar
Wang S C, Lu Z Z, Liang Y, Wang G Y 2022 Acta Phys. Sin. 71 050502
Google Scholar
[15] Lu Z Z, Liang Y, Dong Y J, Wang S C 2022 Electron. Lett. 58 681
Google Scholar
[16] Mannan Z I, Choi H, Kim H 2016 Int. J. Bifurcation Chaos 26 1630009
Google Scholar
[17] Mannan Z I, Yang C, Kim H 2018 IEEE Circuits Syst. Mag. 18 14
Google Scholar
[18] Mannan Z I, Yang C, Adhikari S P, Kim H 2018 Complexity 2018 8405978
Google Scholar
[19] Mannan Z I, Adhikari S P, Kim H, Chua L O 2020 Nonlinear Dyn. 99 3169
Google Scholar
[20] Jin P P, Wang G Y, Liang Y, Iu H H C, Chua L O 2021 IEEE Trans. Circuits Syst. Regul. Pap. 68 4419
Google Scholar
[21] Dong Y J, Wang G Y, Wang Z R, Iu H H C, Chen L 2022 Int. J. Bifurcation Chaos 32 2250058
Google Scholar
[22] Chua L O 2011 Int. J. Bifurcation Chaos 15 3435
Google Scholar
[23] Chua L O, Sbitnev V, Kim H 2012 Int. J. Bifurcation Chaos 22 1250098
Google Scholar
[24] Ahmer M, Kidwai N R, Yusuf Yasin M 2022 Mater. Today Proc. 51 150
Google Scholar
-
图 1 CCMs的POP (a) 二翼CCM; (b) 四翼CCM; (c) 六翼CCM
Fig. 1. POP of CCMs: (a) 2-lobe CCM; (b) 4-lobe CCM; (c) 6-lobe CCM.
图 2 CCM的DC V-I曲线及其局部有源区的放大图 (a) 二翼CCM; (b) 四翼CCM; (c) 六翼CCM
Fig. 2. DC V-I curves of CCM and the magnification of their locally-active region: (a) 2-lobe CCM; (b) 4-lobe CCM; (c) 6-lobe CCM.
图 3 简化后的CCM电学特性 (a) 滞回曲线; (b) DC V-I曲线
Fig. 3. Electrical characteristics of the simplified CCM: (a) Pinched hysteresis loops; (b) DC V-I curve.
图 5 基于简化CCM的三阶神经元电路模型
Fig. 5. Third-order neuron circuit based on the simplified memristor.
图 6 李雅普诺夫指数图 (a) L = 0.5 H, C = 68 nF, 分岔参数为VD∈(–3 V, –1 V); (b) VD = –2.6 V, C = 68 nF, 分岔参数为L∈(0 H, 1 H)
Fig. 6. Lyapunov exponents diagram: (a) L = 0.5 H, C = 68 nF, the bifurcation parameter VD∈[–3 V, –1 V]; (b) VD = –2.6 V, C = 68 nF, the bifurcation parameter L∈[0 H, 1 H].
图 7 C = 68 nF时, 三阶电路随参数L和VD变化的动力学地图
Fig. 7. Dynamic map of the neuron circuit defined by parameters VD and L.
图 8 基于简化CCM的神经元电路结构框图
Fig. 8. Circuit implementation of the neuron circuit based on the simplified CCM.
图 9 简化CCM的电学特性实测曲线 (a) 忆阻器两端施加正弦电压时vm和vI的瞬时波形; (b) 滞回曲线; (c) 忆阻器两端施加三角波信号时vm和vI的瞬时波形; (d) 准静态 V-I曲线
Fig. 9. Experimentally measured the simplified CCM electrical characteristics: (a) Time-domain waveforms of vm and vI under sine excitation; (b) hysteresis curve; (c) time-domain waveforms of vm and vI under quasi-static state; (d) quasi-static V-I curve.
图 11 VD = –1.2 V时, 实验测量静息状态 (a) vout和vx时域图; (b) vout–vx相位图
Fig. 11. Experimentally measured resting state at VD = –1.2 V: (a) Transient waveforms of vout and vx; (b) phase portrait on the vout-vx plane.
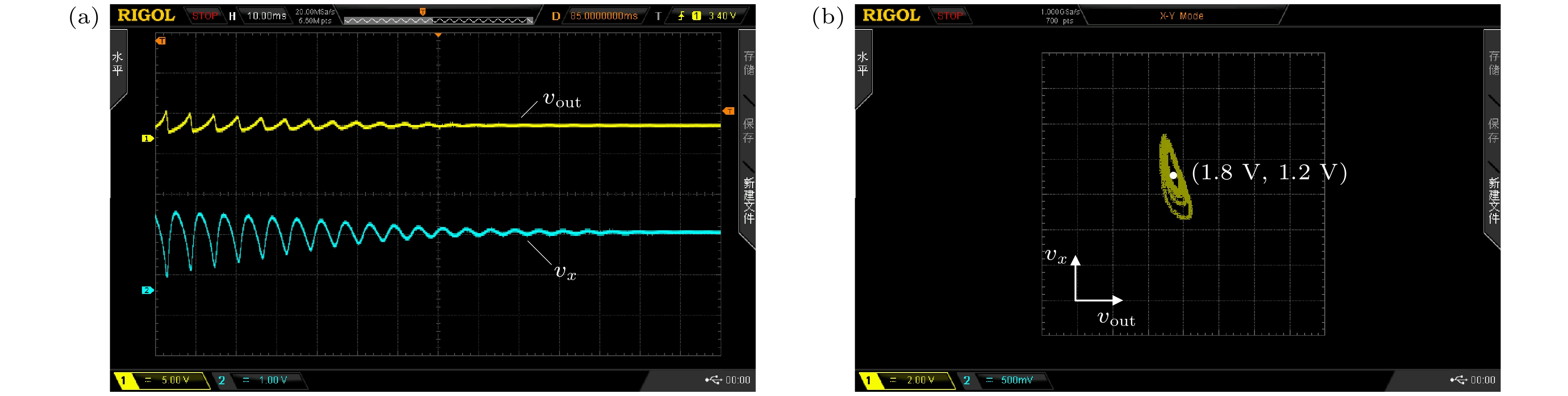
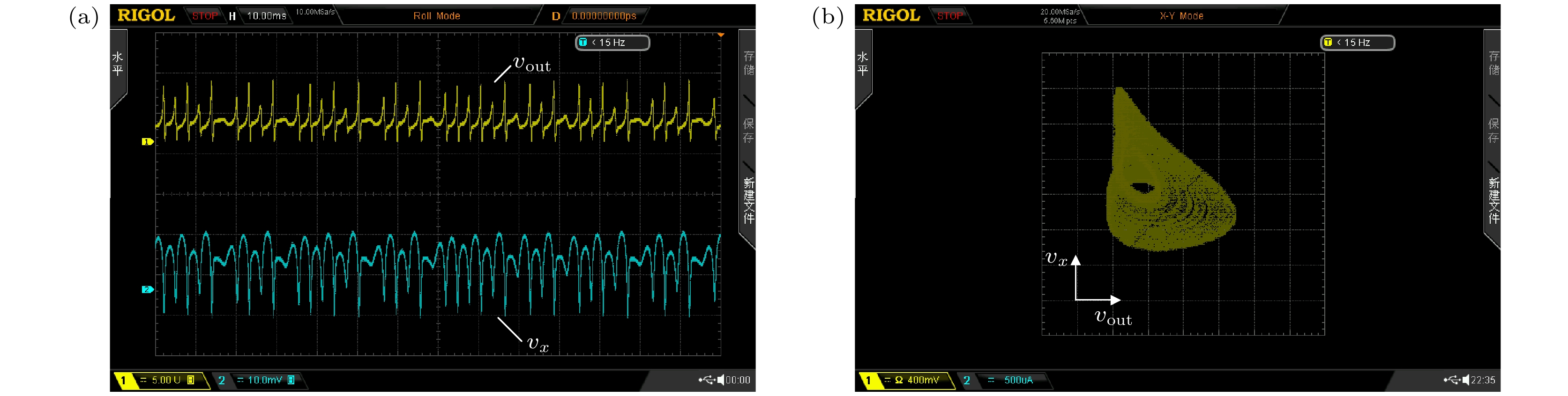
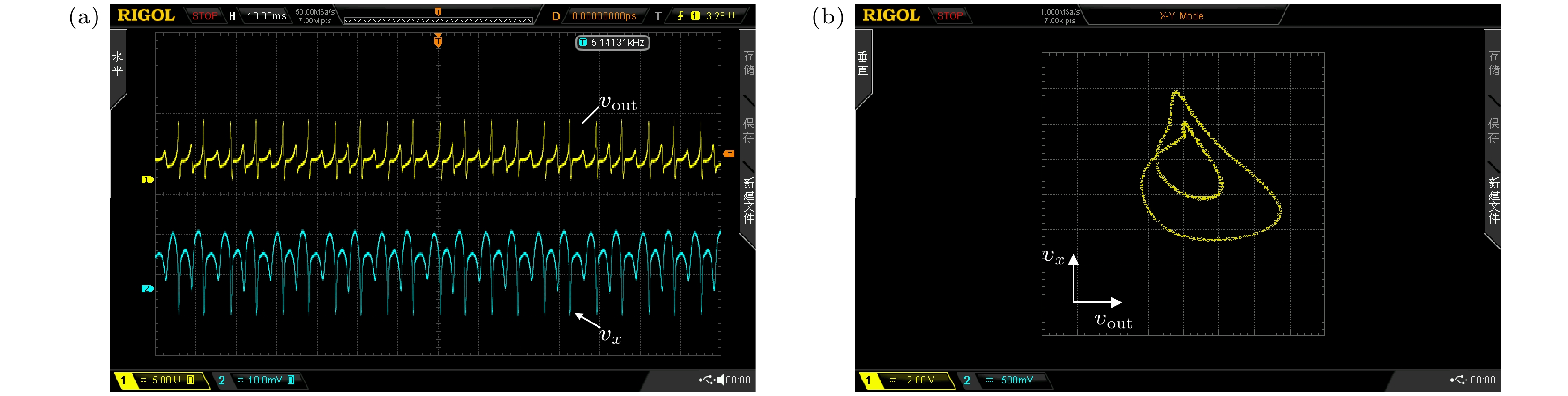
图 12 实验测量周期尖峰状态 (a) VD = –2.1 V时vout和vx时域图; (b) VD = –2.1 V时vout-vx相位图; (c) VD = –2.2 V时vout和vx时域图; (d) VD = –2.2 V时vout-vx相位图
Fig. 12. Experimentally measured periodic spiking phenomenon: (a) Transient waveforms of vout and vx at VD = –2.1 V; (b) phase portrait on the vout-vx plane at VD = –2.1 V; (c) transient waveforms of vout and vx at VD = –2.2 V; (d) phase portrait on the vout-vx plane at VD = –2.2 V.
图 13 当VD = –2.8 V实验测量的混沌状态 (a) vout和vx时域图; (b) vout–vx相位图
Fig. 13. Experimentally measured chaos state at VD = –2.8 V: (a) Transient waveforms of vout and vx; (b) phase portrait on the vout-vx plane.
图 14 当VD = –2.87 V实验测量的双峰响应 (a) vout和vx时域图; (b) vout–vx相位图
Fig. 14. Experimentally measured bimodal response at VD = –2.87 V: (a) Transient waveforms of vout and vx; (b) phase portrait on the vout-vx plane.
图 15 当VD = –2.9 V实验测量周期振荡 (a) vout和vx时域波形; (b) vout-vx相位图
Fig. 15. Experimentally measured periodic oscillation at VD = –2.9 V: (a) Transient waveforms of vout and vx; (b) phase portrait on the vout-vx plane.
表 1 CCM与简化的CCM模型对比
Table 1. Comparisons of CCM and simplified CCM model.
模型种类 DC V-I图段数 局部有源区间 易失/非易失性 绝对值符号数目 简化CCM模型 1 [–3 V, –1 V] 易失型 0 CCM 二翼 3 [–3 V, –1 V] 二值型 2 四翼 5 [–3 V, –1 V] 三值型 4 六翼 7 [–3 V, –1 V] 四值型 6 
下载: 导出CSV
表 2 硬件电路参数配置
Table 2. Parameter configuration of the hardware circuit.
参数 值 参数 值 VCC +15 V VEE –15 V R1, R2, Rin 1 kΩ R3, R5 5 kΩ R6, R8 1 kΩ R4 25 kΩ R7, R9 9 kΩ C1 150 nF C 68 nF L 500 mH
下载: 导出CSV
-
[1] Nawrocki R A, Voyles R M, Shaheen S E 2016 IEEE Trans. Electron Devices 63 3819
Google Scholar
[2] Cassidy A S, Georgiou J, Andreou A G 2013 Neural Netw. 45 4
Google Scholar
[3] Shrestha A, Fang H W, Mei Z D, Rider D P, Wu Q, Qiu Q R 2022 IEEE Circuits Syst. Mag. 22 6
Google Scholar
[4] Shen Z J, Zhao C, Yang L, Zhao C Z 2020 International SoC Design Conference (ISOCC) Yeosu, Korea (South), October 21–24, 2020 p163
[5] Babacan Y, Kaçar F, Gürkan K 2016 Neurocomputing 203 86
Google Scholar
[6] Liu Y, Iu H H C, Qian Y H 2021 IEEE Trans. Circuits Syst. Express Briefs 68 2982
Google Scholar
[7] Chatterjee D, Kottantharayil A 2019 IEEE Electron Device Lett. 40 1301
Google Scholar
[8] Kim S, Du C, Sheridan P, Ma W, Choi S, Lu W D 2015 Nano Lett. 11 2203
Google Scholar
[9] Weiher M, Herzig M, Tetzlaff R, Ascoli A, Mikolajick T, Slesazeck S 2019 IEEE Trans. Circuits Syst. Regul. Pap. 66 2627
Google Scholar
[10] Chua L O 2013 Nanotechnology 24 383001
Google Scholar
[11] Kumar S, Strachan J P, Williams R S 2017 Nature 548 318
Google Scholar
[12] Yi W, Tsang K K, Lam S K, Bai X W, Crowell J A, Flores E A 2018 Nat. Commun. 9 4661
Google Scholar
[13] 李国林 2017 电子电路与系统基础 (北京: 清华大学出版社) 第61页
Li G L 2017 Foundations of Electronic Circuits and Systems (Beijing: Tsinghua University Press) p61 (in Chinese)
[14] 王世场, 卢振洲, 梁燕, 王光义 2022 71 050502
Google Scholar
Wang S C, Lu Z Z, Liang Y, Wang G Y 2022 Acta Phys. Sin. 71 050502
Google Scholar
[15] Lu Z Z, Liang Y, Dong Y J, Wang S C 2022 Electron. Lett. 58 681
Google Scholar
[16] Mannan Z I, Choi H, Kim H 2016 Int. J. Bifurcation Chaos 26 1630009
Google Scholar
[17] Mannan Z I, Yang C, Kim H 2018 IEEE Circuits Syst. Mag. 18 14
Google Scholar
[18] Mannan Z I, Yang C, Adhikari S P, Kim H 2018 Complexity 2018 8405978
Google Scholar
[19] Mannan Z I, Adhikari S P, Kim H, Chua L O 2020 Nonlinear Dyn. 99 3169
Google Scholar
[20] Jin P P, Wang G Y, Liang Y, Iu H H C, Chua L O 2021 IEEE Trans. Circuits Syst. Regul. Pap. 68 4419
Google Scholar
[21] Dong Y J, Wang G Y, Wang Z R, Iu H H C, Chen L 2022 Int. J. Bifurcation Chaos 32 2250058
Google Scholar
[22] Chua L O 2011 Int. J. Bifurcation Chaos 15 3435
Google Scholar
[23] Chua L O, Sbitnev V, Kim H 2012 Int. J. Bifurcation Chaos 22 1250098
Google Scholar
[24] Ahmer M, Kidwai N R, Yusuf Yasin M 2022 Mater. Today Proc. 51 150
Google Scholar
下载:
计量
- 文章访问数: 5922
- PDF下载量: 189
- 被引次数: 0
