-
硅基半导体量子点自旋量子比特因具有相干时间长, 可控性好, 以及与现代先进集成电路制造工艺相兼容等特点, 成为有望实现容错量子计算的潜在候选体系之一, 受到科学界的广泛关注. 近年来, 由于在硅基材料性质, 量子点制造工艺和结构以及量子比特操控技术等方面取得的显著进步, 硅基半导体量子计算在自旋量子比特的高保真度态初始化和读取、单比特逻辑门和两比特逻辑门保真度等方面取得了重要研究进展, 实现了单比特以及两比特逻辑门保真度超过99%的重要突破. 本文将简要介绍硅基半导体量子点的基本概念, 着重讨论在提高单比特以及两比特门操控保真度过程中采用的最新技术手段, 最后简要讨论了需要重点关注的研究方向.
Spin qubits in silicon-based semiconductor quantum dots have become one of the prominent candidates for realizing fault-tolerant quantum computing due to their long coherence time, good controllability, and compatibility with modern advanced integrated circuit manufacturing processes. In recent years, due to the remarkable advances in silicon-based materials, the structure of quantum dot and its fabrication process, and qubit manipulation technology, the great progress of high-fidelity state preparation and readout, single- and two-qubit gates of spin qubits for silicon based semiconductor quantum computation has been achieved. The control fidelities for single- and two-qubit gates all exceed 99%—fault tolerance threshold required by the surface code known for its exceptionally high tolerance to errors. In this paper, we briefly introduce the basic concepts of silicon-based semiconductor quantum dots, discuss the state-of-art technologies used to improve the fidelities of single- and two-qubit gates, and finally highlight the research directions that should be focused on. This paper is organized as follows. Firstly, we introduce three major types of quantum dot (QD) devices fabricated on different silicon-based substrates, including Si/SiGe heterojunction and Si/SiO2. The spin degree of electron or nucleus hosted in QD can be encoded into spin qubits. Electron spin qubits can be thermally initialized to ground state by using an electron reservoirs, and can be read out by spin-charge conversion mechanism: energy-selective readout (Elzerman readout) with reservoirs or Pauli spin blockade (PSB) without reservoirs. Additionally, high fidelity single-shot readout has been demonstrated by using radio-frequency gate reflectometry combined with the PSB, which has unique advantages in large-scale qubit array. To coherently control the spin qubits, electron dipole resonance (ESR) or electron dipole spin resonance (EDSR) for electron and nuclear magnetic resonance (NMR) for nucleus are introduced. With the help of isotope purification greatly improving the dephasing time of qubit and fast single-qubit manipulation based on EDSR, fidelity above 99.9% can be reached. For the two-qubit gates based on exchange interaction between electron spins, the strength of interaction $ J $ combined with Zeeman energy difference$ \Delta {E}_{z} $ determines the energy levels of system, which lead to the different two-qubit gates, such as controlled-Z (CZ), controlled-Rotation (CROT), and the square root of the SWAP gate ($ \sqrt{\rm{S}\rm{W}\rm{A}\rm{P}} $ ) gates. In order to improve the fidelity of two-qubit gates, a series of key technologies is used experimentally, they being isotope purification, symmetry operation, careful Hamiltonian engineering, and gate set tomography. Fidelity of two-qubit gates exceeding 99% has been demonstrated for electron spin qubits in Si/SiGe quantum dots and nuclear spin qubits in donors. These advances have pushed the silicon-based spin qubit platform to become a major stepping stone towards fault-tolerant quantum computation. Finally, we discuss the future study of spin qubits, that is, how to effectively expand the number of qubits, and many other problems to be explored and solved.-
Keywords:
- quantum computing /
- silicon-based semiconductor quantum dot /
- spin qubit /
- logic gate /
- fidelity
[1] Fowler A G, Mariantoni M, Martinis J M, Cleland A N 2012 Phys. Rev. A 86 032324
Google Scholar
[2] Zhang X, Li H O, Cao G, Xiao M, Guo G C, Guo G P 2019 Natl. Sci. Rev. 6 32
Google Scholar
[3] Sun Z, H, Wang B C, Wang N, Liu H, W, Cao G, Li H O, Guo G P 2022 Low. Temp. Phys. Lett. 43 0165
Google Scholar
[4] Hu R Z, Ma R L, Ni M, Zhang X, Zhou Y, Wang K, Luo G, Cao G, Kong Z Z, Wang G L, Li H O, Guo G P 2021 Nanomaterials 11 2486
Google Scholar
[5] Zhang X, Hu R Z, Li H O, Jing F M, Zhou Y, Ma R L, Ni M, Luo G, Cao G, Wang G L, Hu X, Jiang H W, Guo G C, Guo G P 2020 Phys. Rev. Lett. 124 257701
Google Scholar
[6] Pla J J, Tan K Y, Dehollain J P, Lim W H, Morton J J L, Jamieson D N, Dzurak A S, Morello A 2012 Nature 489 541
Google Scholar
[7] Morello A, Pla J J, Zwanenburg F A, Chan Kok W, Tan K Y, Huebl H, Möttönen M, Nugroho C D, Yang C Y, van Donkelaar J A, Alves A D C, Jamieson D N, Escott C C, Hollenberg L C L, Clark R G, Dzurak A S 2010 Nature 467 687
Google Scholar
[8] Yoneda J, Takeda K, Otsuka T, Nakajima T, Delbecq M R, Allison G, Honda T, Kodera T, Oda S, Hoshi Y, Usami N, Itoh K M, Tarucha S 2018 Nat. Nanotechnol. 13 102
Google Scholar
[9] Veldhorst M, Hwang J C C, Yang C H, Leenstra A W, de Ronde B, Dehollain J P, Muhonen J T, Hudson F E, Itoh K M, Morello A, Dzurak A S 2014 Nat. Nanotechnol. 9 981
Google Scholar
[10] Takeda K, Kamioka J, Otsuka T, Yoneda J, Nakajima T, Delbecq M R, Amaha S, Allison G, Kodera T, Oda S, Tarucha S 2016 Sci. Adv. 2 e1600694
Google Scholar
[11] Veldhorst M, Yang C H, Hwang J C C, Huang W, Dehollain J P, Muhonen J T, Simmons S, Laucht A, Hudson F E, Itoh K M, Morello A, Dzurak A S 2015 Nature 526 410
Google Scholar
[12] Watson T F, Philips S G J, Kawakami E, Ward D R, Scarlino P, Veldhorst M, Savage D E, Lagally M G, Friesen M, Coppersmith S N, Eriksson M A, Vandersypen L M K 2018 Nature 555 633
Google Scholar
[13] Zajac D M, Sigillito A J, Russ M, Borjans F, Taylor J M, Burkard G, Petta J R 2018 Science 359 439
Google Scholar
[14] He Y, Gorman S K, Keith D, Kranz L, Keizer J G, Simmons M Y 2019 Nature 571 371
Google Scholar
[15] Huang W, Yang C H, Chan K W, Tanttu T, Hensen B, Leon R C C, Fogarty M A, Hwang J C C, Hudson F E, Itoh K M, Morello A, Laucht A, Dzurak A S 2019 Nature 569 532
Google Scholar
[16] Takeda K, Noiri A, Nakajima T, Yoneda J, Kobayashi T, Tarucha S 2021 Nat. Nanotechnol. 16 695
[17] Mądzik M T, Asaad S, Youssry A, Joecker B, Rudinger K M, Nielsen E, Young K C, Proctor T J, Baczewski A D, Laucht A, Schmitt V, Hudson F E, Itoh K M, Jakob A M, Johnson B C, Jamieson D N, Dzurak A S, Ferrie C, Blume-Kohout R, Morello A 2022 Nature 601 348
Google Scholar
[18] Philips S G J, Mądzik M T, Amitonov S V, de Snoo S L, Russ M, Kalhor N, Volk C, Lawrie W I L, Brousse D, Tryputen L, Wuetz B P, Sammak A, Veldhorst M, Scappucci G, Vandersypen L M K 2022 Nature 609 919
Google Scholar
[19] Petit L, Eenink H G J, Russ M, Lawrie W I L, Hendrickx N W, Philips S G J, Clarke J S, Vandersypen L M K, Veldhorst M 2020 Nature 580 355
Google Scholar
[20] Yang C H, Leon R C C, Hwang J C C, Saraiva A, Tanttu T, Huang W, Camirand Lemyre J, Chan K W, Tan K Y, Hudson F E, Itoh K M, Morello A, Pioro-Ladrière M, Laucht A, Dzurak A S 2020 Nature 580 350
Google Scholar
[21] Mi X, Benito M, Putz S, Zajac D M, Taylor J M, Burkard G, Petta J R 2018 Nature 555 599
Google Scholar
[22] Samkharadze N, Zheng G, Kalhor N, Brousse D, Sammak A, Mendes U C, Blais A, Scappucci G, Vandersypen L M K 2018 Science 359 1123
Google Scholar
[23] Borjans F, Croot X G, Mi X, Gullans M J, Petta J R 2020 Nature 577 195
Google Scholar
[24] Zwerver A M J, Krähenmann T, Watson T F, Lampert L, George H C, Pillarisetty R, Bojarski S A, Amin P, Amitonov S V, Boter J M, Caudillo R, Correas-Serrano D, Dehollain J P, Droulers G, Henry E M, Kotlyar R, Lodari M, Lüthi F, Michalak D J, Mueller B K, Neyens S, Roberts J, Samkharadze N, Zheng G, Clarke J S 2022 Nat. Electron. 5 184
Google Scholar
[25] Xue X, Russ M, Samkharadze N, Undseth B, Sammak A, Scappucci G, Vandersypen L M K 2022 Nature 601 343
Google Scholar
[26] Noiri A, Takeda K, Nakajima T, Kobayashi T, Sammak A, Scappucci G, Tarucha S 2022 Nature 601 338
Google Scholar
[27] Mills A R, Guinn C R, Gullans M J, Sigillito A J, Feldman M M, Nielsen E, Petta J R 2022 Sci Adv. 8 eabn5130
Google Scholar
[28] Pla J J, Tan K Y, Dehollain J P, Lim W H, Morton J J L, Zwanenburg F A, Jamieson D N, Dzurak A S, Morello A 2013 Nature 496 334
Google Scholar
[29] Urdampilleta M, Niegemann D J, Chanrion E, Jadot B, Spence C, Mortemousque P A, Bäuerle C, Hutin L, Bertrand B, Barraud S, Maurand R, Sanquer M, Jehl X, De Franceschi S, Vinet M, Meunier T 2019 Nat. Nanotechnol. 14 737
Google Scholar
[30] West A, Hensen B, Jouan A, Tanttu T, Yang C H, Rossi A, Gonzalez-Zalba M F, Hudson F, Morello A, Reilly D J, Dzurak A S 2019 Nat. Nanotechnol. 14 437
Google Scholar
[31] Zhang X, Zhou Y, Hu R Z, Ma R L, Ni M, Wang K, Luo G, Cao G, Wang G L, Huang P H, Hu X D, Jiang H W, Li H O, Guo G C, Guo G P 2021 Phys. Rev. Appl. 15 044042
Google Scholar
[32] Muhonen J T, Dehollain J P, Laucht A, Hudson F E, Kalra R, Sekiguchi T, Itoh K M, Jamieson D N, McCallum J C, Dzurak A S, Morello A 2014 Nat. Nanotechnol. 9 986
Google Scholar
[33] Fogarty M A, Veldhorst M, Harper R, Yang C H, Bartlett S D, Flammia S T, Dzurak A S 2015 Phys. Rev. A 92 022326
Google Scholar
[34] Yang C H, Chan K W, Harper R, Huang W, Evans T, Hwang J C C, Hensen B, Laucht A, Tanttu T, Hudson F E, Flammia S T, Itoh K M, Morello A, Bartlett S D, Dzurak A S 2019 Nat. Electron. 2 151
Google Scholar
[35] Dehollain J P, Muhonen J T, Blume-Kohout R, Rudinger K M, Gamble J K, Nielsen E, Laucht A, Simmons S, Kalra R, Dzurak A S, Morello A 2016 New J. Phys. 18 103018
Google Scholar
[36] Meunier T, Calado V E, Vandersypen L M K 2021 Phys. Rev. B 83 121403
Google Scholar
[37] Xue X, Patra B, van Dijk J P G, et al. 2021 Nature 593 205
Google Scholar
-
图 1 三种主要的硅基半导体量子点实验装置图 (a) Si/SiGe异质结四量子点装置图[3]; (b) SiMOS双量子点装置图[4]; (c) 硅中掺31P原子量子点装置图[6]
Fig. 1. Three major silicon-based semiconductor quantum dot devices: (a) Quadruple quantum dot with its schematic of the cross-section in Si/SiGe heterojunction[3]; (b) SiMOS double quantum dot and its schematic of cross-section[4]; (c) quantum dot device with 31P atoms in silicon[6].
图 2 自旋量子比特的初始化及读出示意图 (a) 电子自旋初始化; (b) 借助电子库的能量选择读出; (c) 基于耦合双量子点的泡利自旋阻塞读出; (d) 基于量子点栅极的射频读出线路图[29]
Fig. 2. Schematic diagram of initialization and readout of electron spin qubit: (a) Electron spin initialization; (b) energy-selective readout with the aid of reservoirs; (c) Pauli spin blocked readout based on coupled double quantum dots; (d) the circuit diagram of gate-based radiofrequency readout[29].
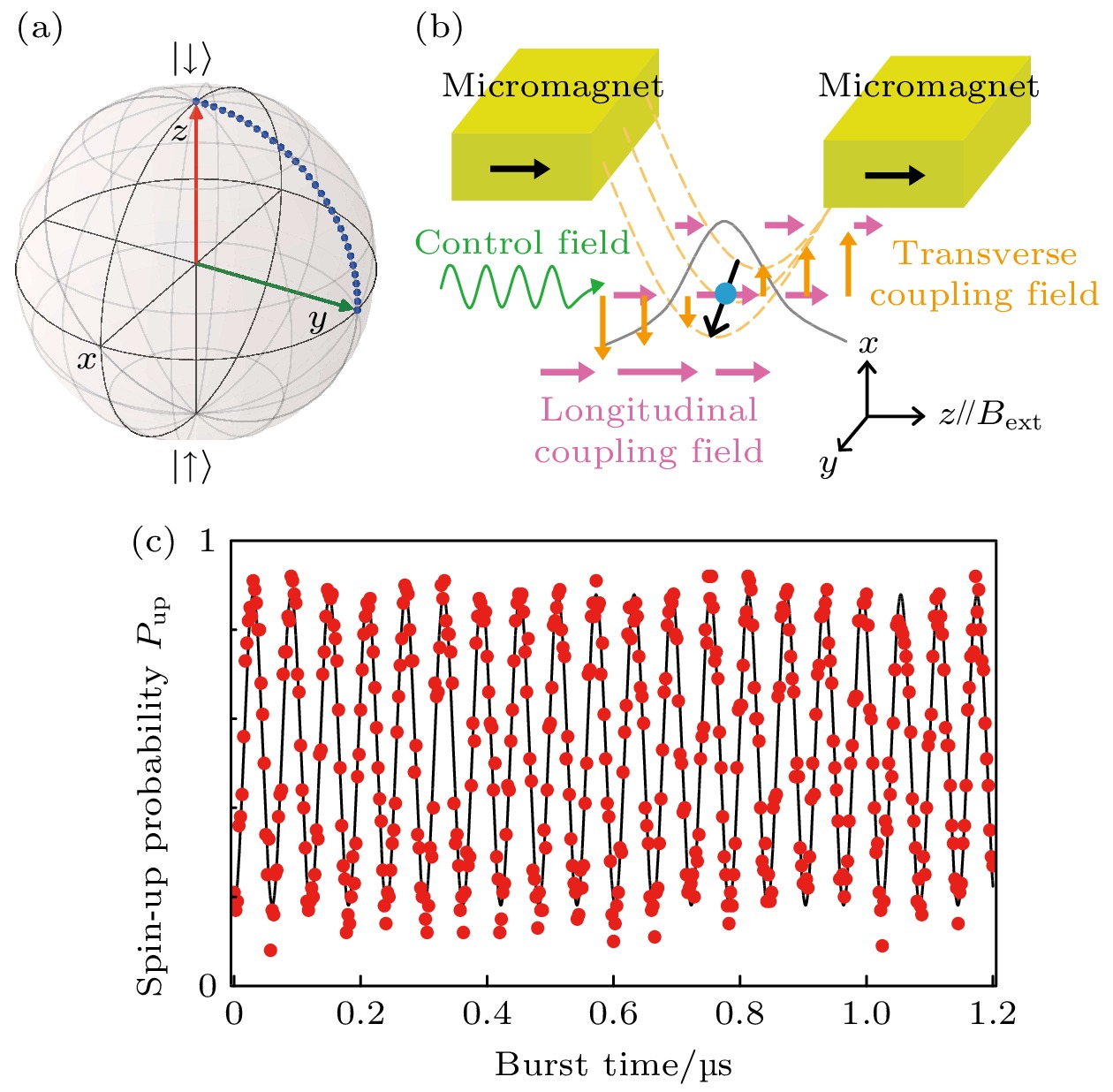
图 3 电子自旋量子比特的相干操控 (a) 电子自旋量子比特的布洛赫球表示; (b) 基于微磁体的EDSR操控原理图[8]; (c) 利用EDSR技术实现的电子自旋量子比特Rabi振荡[8]
Fig. 3. Coherent manipulation of the electron spin qubits: (a) Bloch sphere of an electron spin qubit; (b) micro-magnet-based EDSR manipulation of spin qubit[8]; (c) Rabi oscillation of electron spin qubit using EDSR technology[8].
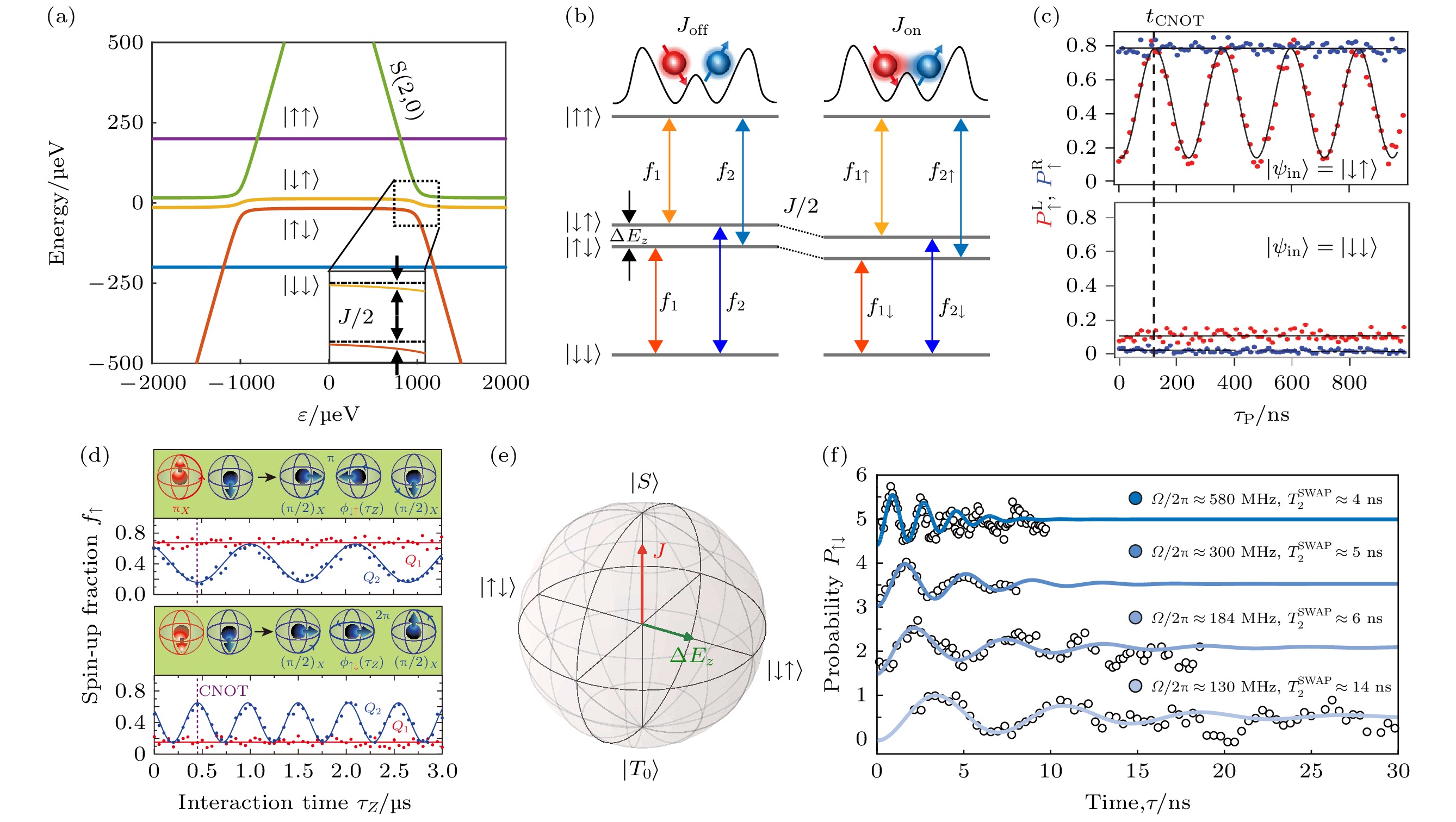
图 4 基于交换相互作用的两比特逻辑门 (a) 两比特系统能级与失谐的关系(
$ J\ll \Delta {E}_{z} $ ); (b) 交换耦合打开和关闭时的能级图; (c) CROT门[13]; (d) CPhase门[11]; (e)$ \left|S\right.\rangle $ 和$ \left|{T}_{0}\right.\rangle $ 态的布洛赫球表示; (f) 交换相互作用驱动下的相干自旋-自旋振荡[14]Fig. 4. Two-qubit gates for electron spin qubits based on exchange interaction. (a) Energy level diagram of two spin qubits as a function of detuning when
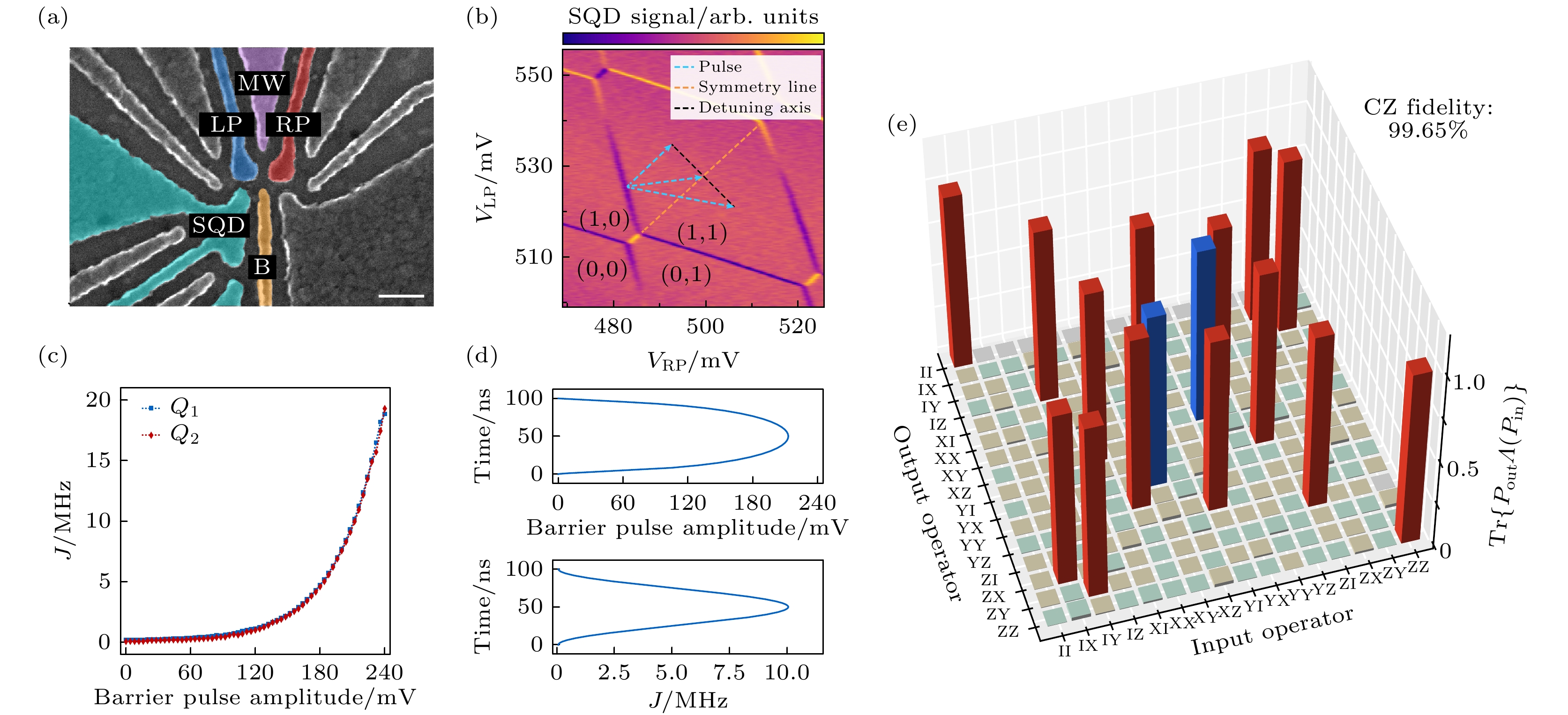
$ J\ll \Delta {E}_{z} $ ; (b) energy level diagram when exchange coupling off (left) and on (right); (c) CROT gate[13]; (d) CPhase gate[11]; (e) Bloch-sphere representation of the$S\text-{T}_{0}$ states; (f) exchange-driven coherent spin-spin oscillations[14].
图 5 高保真度CZ门[25] (a) 28Si/SiGe双量子点实验装置图; (b) 电荷稳定图及对称操作点; (c) 交换相互作用与点间势垒电压的关系; (d) 用于调控交换耦合的脉冲波形; (e) 基于门集断层成像的两比特CZ门保真度表征结果
Fig. 5. High fidelity CZ gate in 28Si/SiGe quantum dots[25]: (a) SEM image of double quantum dot device; (b) symmetry operation point in the charge-stability diagram; (c) exchange strength as a function of barrier pulse amplitude; (d) the exchange pulse for high fidelity CZ gate; (e) gate-set tomography for CZ gate.
图 6 高保真度的CROT门[26] (a) 28Si/SiGe三量子点实验装置图; (b) EDSR能谱; (c) 比特退相干时间以及(d) Rabi振荡幅值与Rabi频率(交换相互作用
$ J $ )的关系; (e) 单比特门以及(f) 两比特逻辑门保真度与Rabi频率(交换相互作用$ J $ )的关系Fig. 6. High fidelity CROT gate in 28Si/SiGe quantum dots[26]: (a) SEM image of the triple quantum dots device; (b) EDSR spectra for Q1 and Q2; (c) Rabi frequency dependance of the dephasing time and (d) Rabi decay during CROT gate; (e) Rabi frequency dependance of single-qubit gate and (f) two-qubit gate fidelity.
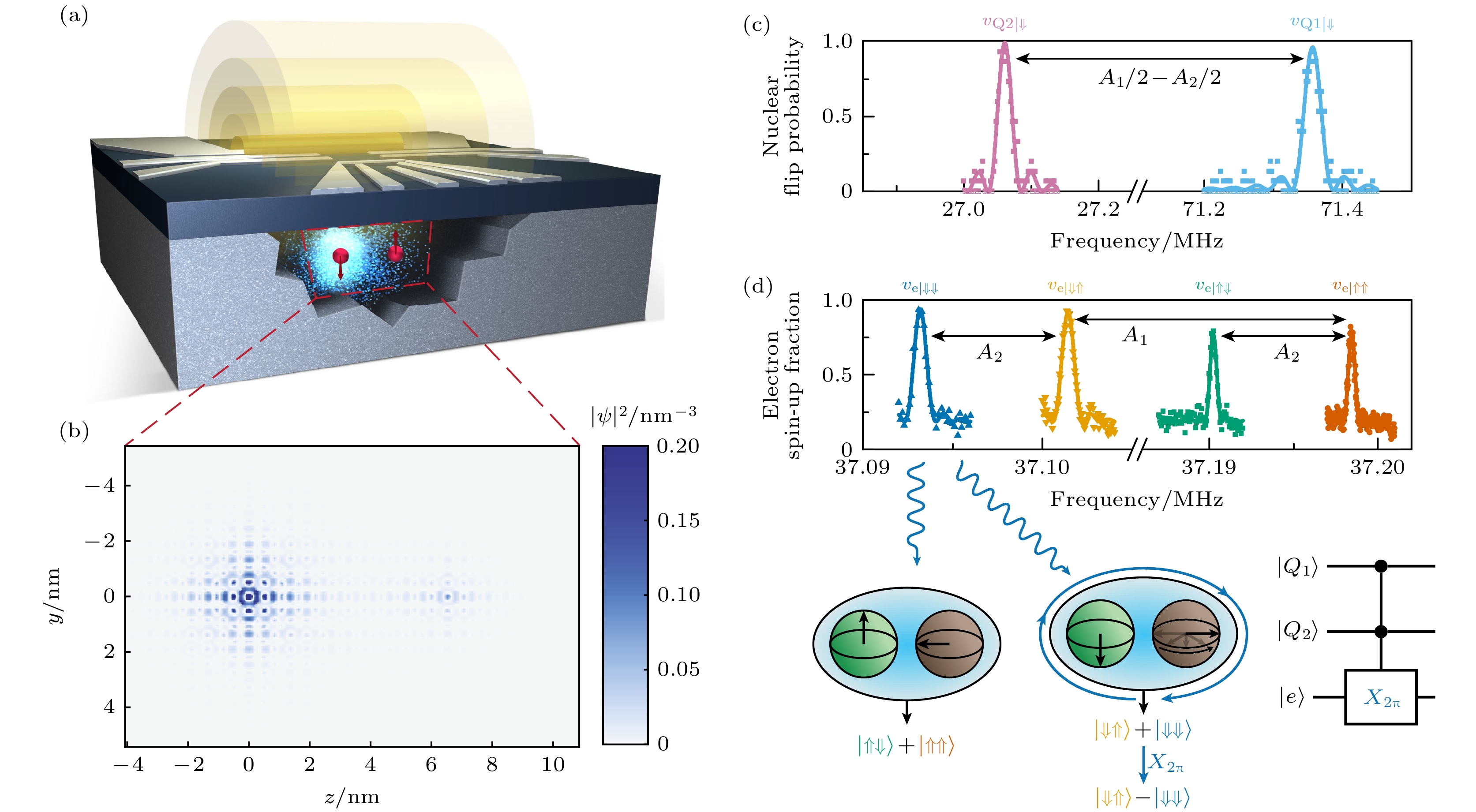
图 7 几何CZ门[17] (a) 两个核自旋耦合到同一个电子的装置示意图; (b) 电子波函数的空间分布; (c) 核自旋的NMR能谱以及电子自旋的ESR能谱; (d) 几何CZ门的实现过程
Fig. 7. Geometric two-qubit CZ gate[17]: (a) Schematic diagram of a pair of 31P nuclei (red) asymmetrically coupled to the same electron (blue); (b) spatial distribution of electron wave function around two nuclear spins; (c) NMR spectra for nuclei spins and ESR spectra for electron spin; (d) implementation of a geometric two-qubit CZ gate.
-
[1] Fowler A G, Mariantoni M, Martinis J M, Cleland A N 2012 Phys. Rev. A 86 032324
Google Scholar
[2] Zhang X, Li H O, Cao G, Xiao M, Guo G C, Guo G P 2019 Natl. Sci. Rev. 6 32
Google Scholar
[3] Sun Z, H, Wang B C, Wang N, Liu H, W, Cao G, Li H O, Guo G P 2022 Low. Temp. Phys. Lett. 43 0165
Google Scholar
[4] Hu R Z, Ma R L, Ni M, Zhang X, Zhou Y, Wang K, Luo G, Cao G, Kong Z Z, Wang G L, Li H O, Guo G P 2021 Nanomaterials 11 2486
Google Scholar
[5] Zhang X, Hu R Z, Li H O, Jing F M, Zhou Y, Ma R L, Ni M, Luo G, Cao G, Wang G L, Hu X, Jiang H W, Guo G C, Guo G P 2020 Phys. Rev. Lett. 124 257701
Google Scholar
[6] Pla J J, Tan K Y, Dehollain J P, Lim W H, Morton J J L, Jamieson D N, Dzurak A S, Morello A 2012 Nature 489 541
Google Scholar
[7] Morello A, Pla J J, Zwanenburg F A, Chan Kok W, Tan K Y, Huebl H, Möttönen M, Nugroho C D, Yang C Y, van Donkelaar J A, Alves A D C, Jamieson D N, Escott C C, Hollenberg L C L, Clark R G, Dzurak A S 2010 Nature 467 687
Google Scholar
[8] Yoneda J, Takeda K, Otsuka T, Nakajima T, Delbecq M R, Allison G, Honda T, Kodera T, Oda S, Hoshi Y, Usami N, Itoh K M, Tarucha S 2018 Nat. Nanotechnol. 13 102
Google Scholar
[9] Veldhorst M, Hwang J C C, Yang C H, Leenstra A W, de Ronde B, Dehollain J P, Muhonen J T, Hudson F E, Itoh K M, Morello A, Dzurak A S 2014 Nat. Nanotechnol. 9 981
Google Scholar
[10] Takeda K, Kamioka J, Otsuka T, Yoneda J, Nakajima T, Delbecq M R, Amaha S, Allison G, Kodera T, Oda S, Tarucha S 2016 Sci. Adv. 2 e1600694
Google Scholar
[11] Veldhorst M, Yang C H, Hwang J C C, Huang W, Dehollain J P, Muhonen J T, Simmons S, Laucht A, Hudson F E, Itoh K M, Morello A, Dzurak A S 2015 Nature 526 410
Google Scholar
[12] Watson T F, Philips S G J, Kawakami E, Ward D R, Scarlino P, Veldhorst M, Savage D E, Lagally M G, Friesen M, Coppersmith S N, Eriksson M A, Vandersypen L M K 2018 Nature 555 633
Google Scholar
[13] Zajac D M, Sigillito A J, Russ M, Borjans F, Taylor J M, Burkard G, Petta J R 2018 Science 359 439
Google Scholar
[14] He Y, Gorman S K, Keith D, Kranz L, Keizer J G, Simmons M Y 2019 Nature 571 371
Google Scholar
[15] Huang W, Yang C H, Chan K W, Tanttu T, Hensen B, Leon R C C, Fogarty M A, Hwang J C C, Hudson F E, Itoh K M, Morello A, Laucht A, Dzurak A S 2019 Nature 569 532
Google Scholar
[16] Takeda K, Noiri A, Nakajima T, Yoneda J, Kobayashi T, Tarucha S 2021 Nat. Nanotechnol. 16 695
[17] Mądzik M T, Asaad S, Youssry A, Joecker B, Rudinger K M, Nielsen E, Young K C, Proctor T J, Baczewski A D, Laucht A, Schmitt V, Hudson F E, Itoh K M, Jakob A M, Johnson B C, Jamieson D N, Dzurak A S, Ferrie C, Blume-Kohout R, Morello A 2022 Nature 601 348
Google Scholar
[18] Philips S G J, Mądzik M T, Amitonov S V, de Snoo S L, Russ M, Kalhor N, Volk C, Lawrie W I L, Brousse D, Tryputen L, Wuetz B P, Sammak A, Veldhorst M, Scappucci G, Vandersypen L M K 2022 Nature 609 919
Google Scholar
[19] Petit L, Eenink H G J, Russ M, Lawrie W I L, Hendrickx N W, Philips S G J, Clarke J S, Vandersypen L M K, Veldhorst M 2020 Nature 580 355
Google Scholar
[20] Yang C H, Leon R C C, Hwang J C C, Saraiva A, Tanttu T, Huang W, Camirand Lemyre J, Chan K W, Tan K Y, Hudson F E, Itoh K M, Morello A, Pioro-Ladrière M, Laucht A, Dzurak A S 2020 Nature 580 350
Google Scholar
[21] Mi X, Benito M, Putz S, Zajac D M, Taylor J M, Burkard G, Petta J R 2018 Nature 555 599
Google Scholar
[22] Samkharadze N, Zheng G, Kalhor N, Brousse D, Sammak A, Mendes U C, Blais A, Scappucci G, Vandersypen L M K 2018 Science 359 1123
Google Scholar
[23] Borjans F, Croot X G, Mi X, Gullans M J, Petta J R 2020 Nature 577 195
Google Scholar
[24] Zwerver A M J, Krähenmann T, Watson T F, Lampert L, George H C, Pillarisetty R, Bojarski S A, Amin P, Amitonov S V, Boter J M, Caudillo R, Correas-Serrano D, Dehollain J P, Droulers G, Henry E M, Kotlyar R, Lodari M, Lüthi F, Michalak D J, Mueller B K, Neyens S, Roberts J, Samkharadze N, Zheng G, Clarke J S 2022 Nat. Electron. 5 184
Google Scholar
[25] Xue X, Russ M, Samkharadze N, Undseth B, Sammak A, Scappucci G, Vandersypen L M K 2022 Nature 601 343
Google Scholar
[26] Noiri A, Takeda K, Nakajima T, Kobayashi T, Sammak A, Scappucci G, Tarucha S 2022 Nature 601 338
Google Scholar
[27] Mills A R, Guinn C R, Gullans M J, Sigillito A J, Feldman M M, Nielsen E, Petta J R 2022 Sci Adv. 8 eabn5130
Google Scholar
[28] Pla J J, Tan K Y, Dehollain J P, Lim W H, Morton J J L, Zwanenburg F A, Jamieson D N, Dzurak A S, Morello A 2013 Nature 496 334
Google Scholar
[29] Urdampilleta M, Niegemann D J, Chanrion E, Jadot B, Spence C, Mortemousque P A, Bäuerle C, Hutin L, Bertrand B, Barraud S, Maurand R, Sanquer M, Jehl X, De Franceschi S, Vinet M, Meunier T 2019 Nat. Nanotechnol. 14 737
Google Scholar
[30] West A, Hensen B, Jouan A, Tanttu T, Yang C H, Rossi A, Gonzalez-Zalba M F, Hudson F, Morello A, Reilly D J, Dzurak A S 2019 Nat. Nanotechnol. 14 437
Google Scholar
[31] Zhang X, Zhou Y, Hu R Z, Ma R L, Ni M, Wang K, Luo G, Cao G, Wang G L, Huang P H, Hu X D, Jiang H W, Li H O, Guo G C, Guo G P 2021 Phys. Rev. Appl. 15 044042
Google Scholar
[32] Muhonen J T, Dehollain J P, Laucht A, Hudson F E, Kalra R, Sekiguchi T, Itoh K M, Jamieson D N, McCallum J C, Dzurak A S, Morello A 2014 Nat. Nanotechnol. 9 986
Google Scholar
[33] Fogarty M A, Veldhorst M, Harper R, Yang C H, Bartlett S D, Flammia S T, Dzurak A S 2015 Phys. Rev. A 92 022326
Google Scholar
[34] Yang C H, Chan K W, Harper R, Huang W, Evans T, Hwang J C C, Hensen B, Laucht A, Tanttu T, Hudson F E, Flammia S T, Itoh K M, Morello A, Bartlett S D, Dzurak A S 2019 Nat. Electron. 2 151
Google Scholar
[35] Dehollain J P, Muhonen J T, Blume-Kohout R, Rudinger K M, Gamble J K, Nielsen E, Laucht A, Simmons S, Kalra R, Dzurak A S, Morello A 2016 New J. Phys. 18 103018
Google Scholar
[36] Meunier T, Calado V E, Vandersypen L M K 2021 Phys. Rev. B 83 121403
Google Scholar
[37] Xue X, Patra B, van Dijk J P G, et al. 2021 Nature 593 205
Google Scholar
下载:
计量
- 文章访问数: 8395
- PDF下载量: 462
- 被引次数: 0
