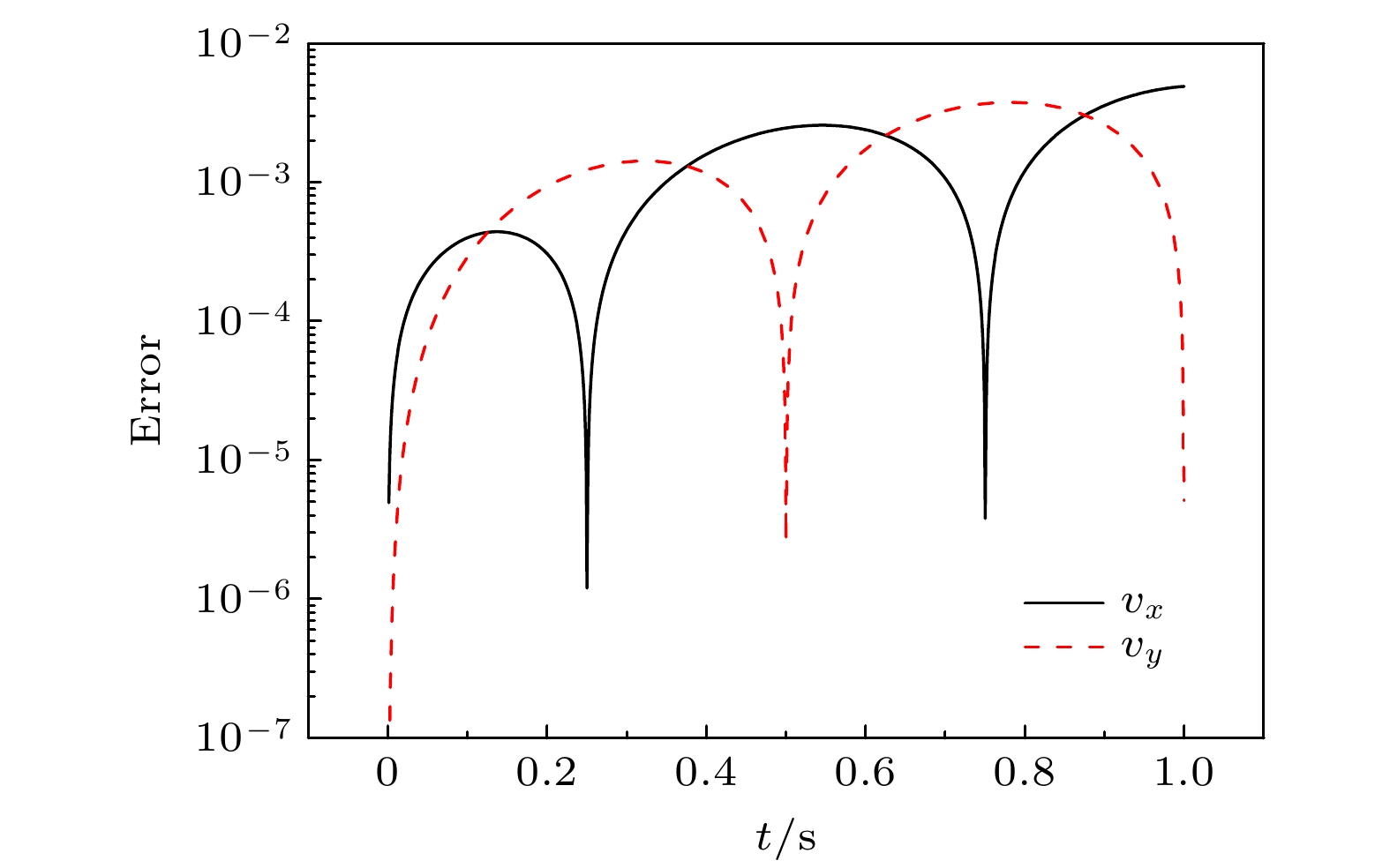
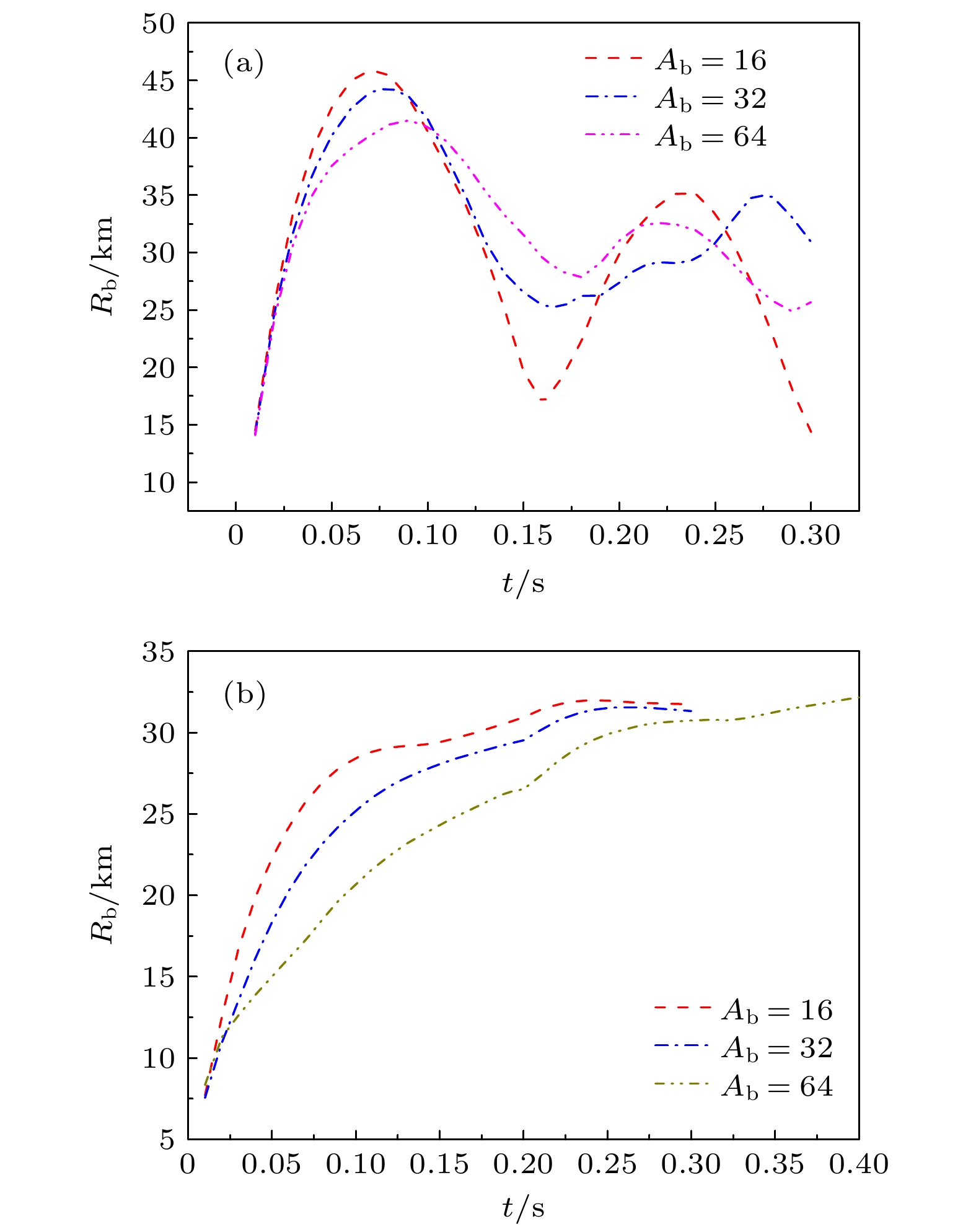
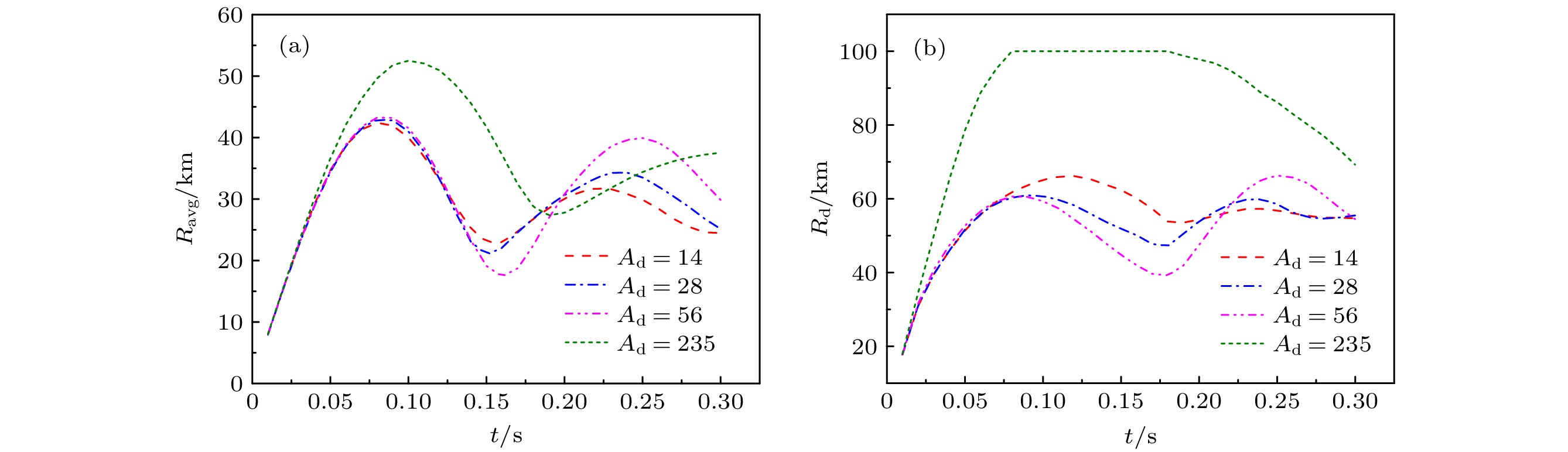
-
利用三维混合模拟程序计算了大量超热碎片离子在低密度背景等离子体中爆炸膨胀的过程. 通过定量计算磁泡的变化过程和磁泡对碎片云运动的约束效果, 分析了背景等离子体电荷密度、背景离子原子量、碎片离子荷质比等参数对磁泡的影响. 计算结果表明, 背景电荷密度对磁泡和碎片云的运动有重要影响. 在碎片云扩张早期, 背景离子原子量对磁泡扩张影响较小, 但对后期碎片云的运动有一定影响. 当碎片离子荷质比较小时, 离子回旋半径大于磁泡半径, 此时磁泡半径较小, 且磁泡无法约束碎片云. 当碎片离子荷质比较大时, 离子回旋半径小于磁泡半径, 如果此时背景电荷密度较低, 磁泡和碎片云的早期扩张几乎不受碎片离子荷质比影响, 但对系统后续演化有一定影响, 如果此时背景电荷密度较大, 碎片离子荷质比对磁泡和碎片云的运动有较大影响.In this work, a three-dimensional hybrid simulation program is used to investigate the process of the explosion and expansion of a large number of thermal debris ions in a low density background plasma. By quantitatively calculating the variation of the magnetic bubble and the bubble constraint in the motion of the debris cloud, studied are the influences of the background plasma charge density, the background ion atomic weight, and the charge-mass ratio of the fragment ion on the magnetic bubble. The results show that the background charge density has an important effect on the motion of the bubble and debris cloud. In the early stage of the debris cloud expansion, the background ion atomic weight has little effect on the bubble expansion, but it affects the movement of debris cloud at a later time. When the charge-mass ratio of the debris ion is small, the radius of the ion gyration is larger than the bubble radius, and the bubble radius is small so that it cannot restrain the debris cloud. When the charge-mass ratio of the debris ion is large, the radius of the ion gyration is smaller than the bubble radius. If the background charge density is low in this condition, the early expansion of the bubble and the debris cloud are slightly affected by the charge-mass ratio of the fragment ions, and only the subsequent evolution of the system is influenced. If the background charge density is large in this condition, the charge-mass ratio of the fragment ion has a prominent influence on the motion of the bubble and the debris cloud.
-
Keywords:
- magnetic bubble /
- debris cloud /
- background charge density /
- background ion atomic weight /
- charge-mass ratio of fragment ion
[1] Gilbert J, Kappenman J, Radasky W, Savage E 2010 The Late-Time (E3) High-Altitude Electromagnetic Pulse (HEMP) and its Impact on the U. S. Power Grid (Oak Ridge National Laboratory: HEMP TAPS/HEMP-PC Audit Report) Meta-R-321
[2] Valenzuela A, Haerendel G, Föppl H, Melzner F, Neuss H, Rieger E, Stöcker J, Bauer O, Höfner H, Loidl J 1986 Nature 320 700
 Google Scholar
Google Scholar
[3] Plechaty C, Presura R, Esaulov A A 2013 Phys. Rev. Lett. 111 185002
 Google Scholar
Google Scholar
[4] Siebert K, Witt E 2019 Nominal Waveforms for Late-Time High-Altitude Electromagnetic Pulse (Applied Research Associates Inc.) DTRA-TR-19-41
[5] 彭国良, 张俊杰 2021 70 180703
 Google Scholar
Google Scholar
Peng G L, Zhang J J 2021 Acta Phys. Sin. 70 180703
 Google Scholar
Google Scholar
[6] Winske D, Huba J D, Niemann C, Le A 2019 Front. Astron. Space Sci. 5 51
 Google Scholar
Google Scholar
[7] Berezin Y A, Dudnikova G I, Fedoruk M P, Vshivkov V A 1998 Int. J. Comp. Fluid D 10 117
 Google Scholar
Google Scholar
[8] Ripin B H, Huba J D, McLean E A, Manka C K, Peyser T, Burris H R 1993 Phys. Fluids 5 3491
 Google Scholar
Google Scholar
[9] Gisler G, Lemons D S 1989 J. Geophys. Res. 94 10145
 Google Scholar
Google Scholar
[10] Winske D 1991 Simulations of HANE/VHANE Dynamics (Defense Nuclear Agency) AD-A243198
[11] Zakharov Y P 2003 IEEE Trans. Plasma Sci. 31 1243
 Google Scholar
Google Scholar
[12] Yamauchi K, Ohsawa Y 2007 Phys. Plasmas 14 053110
 Google Scholar
Google Scholar
[13] Winske D, Gary S P 2007 J. Geophys. Res. 112 A10303
 Google Scholar
Google Scholar
[14] Hewett D W, Brecht S H, Larson D J 2011 J. Geophys. Res. 116 A11310
[15] Peng G L, Zhang J J, Chen J N 2021 Phys. Fluids 33 076602
 Google Scholar
Google Scholar
[16] Sergeivich B A 2015 Ph. D. Dissertation (Berkeley: University of California)
[17] Harned D S 1982 J. Comp. Phys. 47 452
 Google Scholar
Google Scholar
[18] Brecht S H, Thomas V A 1988 Comp. Phys. Commun. 48 135
 Google Scholar
Google Scholar
[19] Lipatov A S 2002 The Hybrid Multiscale Simulation Technology (Berlin Heidelberg: Springer)
[20] 傅竹风, 胡友秋 1994 空间等离子体数值模拟 (合肥: 安徽科学技术出版社) 第6页
Fu Z F, Hu Y Q 1994 Simulation of Space Plasma (Hefei: Anhui Science Press) p6 (in Chinese)
[21] 郑开春 2009 等离子体物理 (北京: 北京大学出版社) 第26页
Zheng K C 2009 Plasma Physics (Beijing: Peking University Press) p26 (in Chinese)
[22] Dedner A, Kemm F, Kroner D, Munz C. D, Schnitzer T, Wesenberg M 2002 J. Comp. Phys. 175 645
 Google Scholar
Google Scholar
[23] Christopher G C Jr 2007 Ph. D. Dissertation (Monterey, California: Naval Postgraduate School)
[24] Mignone A, Bodo G, Vaida B, Mattia G 2018 Astrophys. J. 859 13
 Google Scholar
Google Scholar
[25] Mignone A 2014 J. Comp. Phys. 270 784
 Google Scholar
Google Scholar
[26] Toth G, Odstrcil D 1996 J. Comp. Phys. 128 82
 Google Scholar
Google Scholar
-
-
[1] Gilbert J, Kappenman J, Radasky W, Savage E 2010 The Late-Time (E3) High-Altitude Electromagnetic Pulse (HEMP) and its Impact on the U. S. Power Grid (Oak Ridge National Laboratory: HEMP TAPS/HEMP-PC Audit Report) Meta-R-321
[2] Valenzuela A, Haerendel G, Föppl H, Melzner F, Neuss H, Rieger E, Stöcker J, Bauer O, Höfner H, Loidl J 1986 Nature 320 700
 Google Scholar
Google Scholar
[3] Plechaty C, Presura R, Esaulov A A 2013 Phys. Rev. Lett. 111 185002
 Google Scholar
Google Scholar
[4] Siebert K, Witt E 2019 Nominal Waveforms for Late-Time High-Altitude Electromagnetic Pulse (Applied Research Associates Inc.) DTRA-TR-19-41
[5] 彭国良, 张俊杰 2021 70 180703
 Google Scholar
Google Scholar
Peng G L, Zhang J J 2021 Acta Phys. Sin. 70 180703
 Google Scholar
Google Scholar
[6] Winske D, Huba J D, Niemann C, Le A 2019 Front. Astron. Space Sci. 5 51
 Google Scholar
Google Scholar
[7] Berezin Y A, Dudnikova G I, Fedoruk M P, Vshivkov V A 1998 Int. J. Comp. Fluid D 10 117
 Google Scholar
Google Scholar
[8] Ripin B H, Huba J D, McLean E A, Manka C K, Peyser T, Burris H R 1993 Phys. Fluids 5 3491
 Google Scholar
Google Scholar
[9] Gisler G, Lemons D S 1989 J. Geophys. Res. 94 10145
 Google Scholar
Google Scholar
[10] Winske D 1991 Simulations of HANE/VHANE Dynamics (Defense Nuclear Agency) AD-A243198
[11] Zakharov Y P 2003 IEEE Trans. Plasma Sci. 31 1243
 Google Scholar
Google Scholar
[12] Yamauchi K, Ohsawa Y 2007 Phys. Plasmas 14 053110
 Google Scholar
Google Scholar
[13] Winske D, Gary S P 2007 J. Geophys. Res. 112 A10303
 Google Scholar
Google Scholar
[14] Hewett D W, Brecht S H, Larson D J 2011 J. Geophys. Res. 116 A11310
[15] Peng G L, Zhang J J, Chen J N 2021 Phys. Fluids 33 076602
 Google Scholar
Google Scholar
[16] Sergeivich B A 2015 Ph. D. Dissertation (Berkeley: University of California)
[17] Harned D S 1982 J. Comp. Phys. 47 452
 Google Scholar
Google Scholar
[18] Brecht S H, Thomas V A 1988 Comp. Phys. Commun. 48 135
 Google Scholar
Google Scholar
[19] Lipatov A S 2002 The Hybrid Multiscale Simulation Technology (Berlin Heidelberg: Springer)
[20] 傅竹风, 胡友秋 1994 空间等离子体数值模拟 (合肥: 安徽科学技术出版社) 第6页
Fu Z F, Hu Y Q 1994 Simulation of Space Plasma (Hefei: Anhui Science Press) p6 (in Chinese)
[21] 郑开春 2009 等离子体物理 (北京: 北京大学出版社) 第26页
Zheng K C 2009 Plasma Physics (Beijing: Peking University Press) p26 (in Chinese)
[22] Dedner A, Kemm F, Kroner D, Munz C. D, Schnitzer T, Wesenberg M 2002 J. Comp. Phys. 175 645
 Google Scholar
Google Scholar
[23] Christopher G C Jr 2007 Ph. D. Dissertation (Monterey, California: Naval Postgraduate School)
[24] Mignone A, Bodo G, Vaida B, Mattia G 2018 Astrophys. J. 859 13
 Google Scholar
Google Scholar
[25] Mignone A 2014 J. Comp. Phys. 270 784
 Google Scholar
Google Scholar
[26] Toth G, Odstrcil D 1996 J. Comp. Phys. 128 82
 Google Scholar
Google Scholar
计量
- 文章访问数: 4333
- PDF下载量: 63
- 被引次数: 0













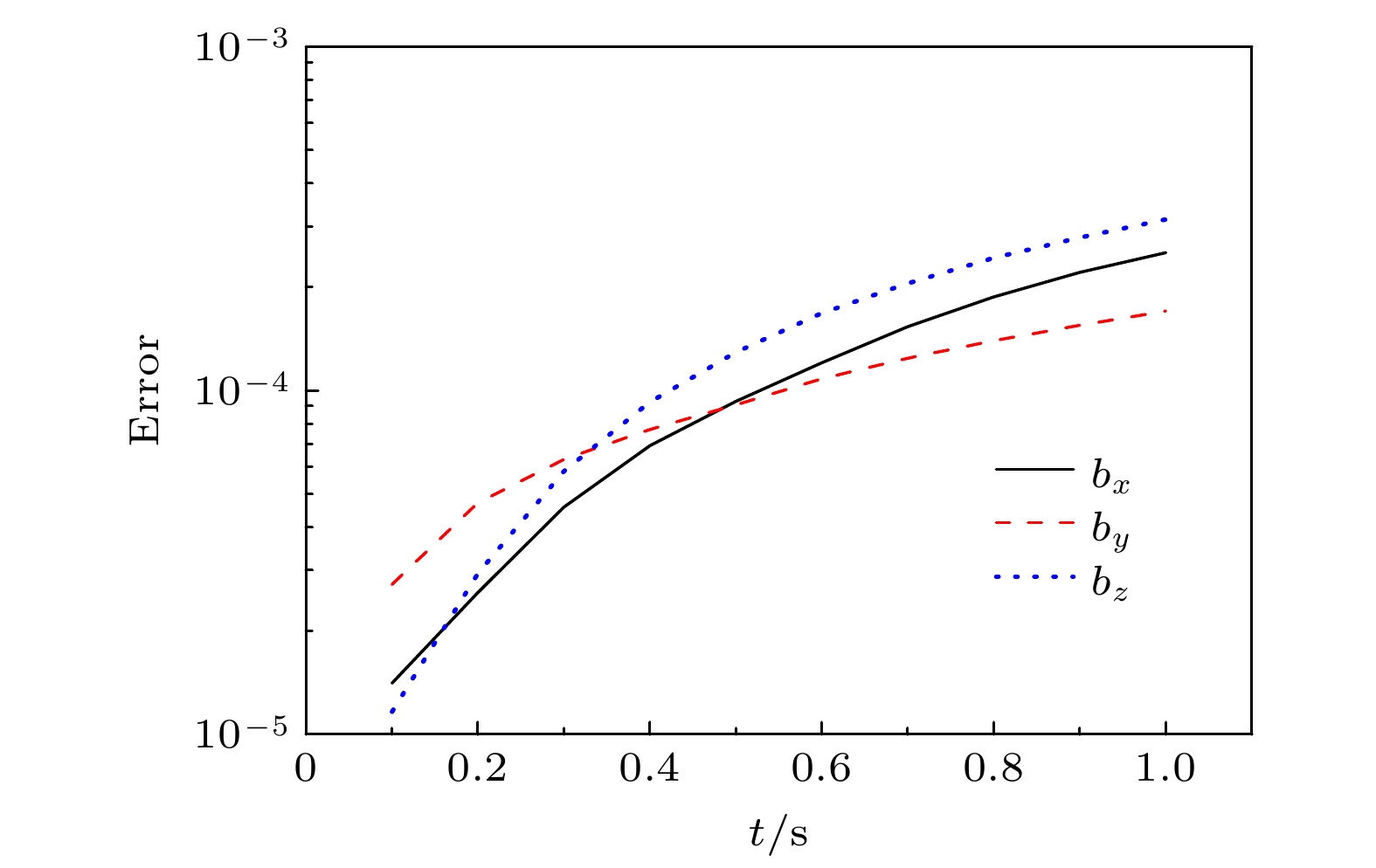
 下载:
下载: