反向旋转双色椭偏场中原子隧穿电离电子的全息干涉

黄雪飞; 苏杰; 廖健颖; 李盈傧; 黄诚

doi:10.7498/aps.71.20212226

摘要

利用数值求解含时薛定谔方程和强场近似理论研究了反向旋转双色椭偏光场中氩原子隧穿电离电子的干涉. 固定两脉冲的椭偏率均为0.3, 当两椭偏场的相对相位为0.25π时, 光电子动量谱中周期内干涉、叉状全息干涉和弧形全息干涉相互重叠. 当两椭偏场的相对相位为0时, 光电子动量谱中弧形全息干涉消除, 并且周期内干涉和叉状全息干涉被彻底分离到动量谱的左右部分, 从而得到一个在空间上独立的叉状全息干涉条纹. 进一步研究表明, 通过改变两椭偏光场的椭偏率还可以增强或抑制该独立叉状全息干涉条纹. 这为干涉条纹的控制和分离提供了一个有效的手段, 同时也有利于从全息干涉条纹中提取靶材结构信息和电子超快动力学信息.

关键词:

Abstract

In this paper, photoelectron interference in tunneling ionization of atoms by counter-rotating two-color elliptically polarized (TCEP) laser fields are investigated by numerically solving the two-dimensional time-dependent Schrödinger equation (TDSE) and strong field approximation (SFA). When the ellipticities of the two pulses are both 0.3, for a relative phase of 0.25π, the intracycle interference, fork-like holographic interference and arc-like holographic interference in the photoelectron momentum distribution overlap with each other. For a relative phase of 0, the arc-like holographic interference disappears and the intracycle interference and fork-like holographic interference are fully separated into the –p_x direction and the +p_x direction. Furthermore, the independent fork-like holographic interference can be enhanced or suppressed by changing the ellipticities of the two pulses. This provides an efficient tool for controlling and separating the interference structures in the photoelectron momentum distribution, which facilitates extracting the information about the target structure and the photoelectron ultrafast dynamics in strong fields.

Keywords:

作者及机构信息

1.
西南大学物理科学与技术学院, 微纳结构光电子学重庆市重点实验室, 重庆　400715

2.
信阳师范学院物理电子工程学院, 信阳　464000

通信作者: 李盈傧, liyingbin2008@163.com ; 黄诚, huangcheng@swu.edu.cn

基金项目: 国家自然科学基金(批准号: 11504302, 12074329, 12004323)和河南省高等学校重点科研项目(批准号: 20A140025)资助的课题.

Authors and contacts

1.
Chongqing City Key Laboratory of Micro & Nano Structure Optoelectronics, School of Physical Science and Technology, Southwest University, Chongqing 400715, China

2.
College of Physics and Electronic Engineering, Xinyang Normal University, Xinyang 464000, China

Corresponding author: Li Ying-Bin, liyingbin2008@163.com ; Huang Cheng, huangcheng@swu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11504302, 12074329, 12004323) and the Key Scientific Research Projects of Higher Education of Henan Province, China (Grant No. 20A140025).

文章全文 : translate this paragraph

参考文献

[1]	Corkum P 1993 Phys. Rev. Lett. 71 1994 Google Scholar
[2]	Agostini P, Fabre F, Mainfray G, Petite G 1979 Phys. Rev. Lett. 42 1127 Google Scholar
[3]	Arbó D G, Persson E, Burgdörfer J 2006 Phys. Rev. A 74 063407 Google Scholar
[4]	Tan J, Xu S, Han X, Zhou Y, Li M, Cao W, Zhang Q, Lu P 2021 Adv. Photonics 3 035001 Google Scholar
[5]	Li M, Zhang P, Luo S Q, Zhou Y M, Zhang Q B, Lan P F, Lu P X 2015 Phys. Rev. A 92 063404 Google Scholar
[6]	Yang W, Zhang H, Lin C, Xu J, Sheng Z, Song X, Hu S, Chen J 2016 Phys. Rev. A 94 043419 Google Scholar
[7]	Wang Y, Yu S, Lai X, Kang H, Xu S, Sun R, Quan W, Liu X 2018 Phys. Rev. A 98 043422 Google Scholar
[8]	Huismans Y, Rouzée A, Gijsbertsen A, Jungmann J H, Smolkowska A S, Logman P S W M, Lépine F, Cauchy C, Zamith S, Marchenko T, Bakker J M, Berden G, Redlich B, van der Meer A F G, Muller H G, Vermin W, Schafer K J, Spanner M, Ivanov M Yu, Smirnova O, Bauer D, Popruzhenko S V, Vrakking M J J 2011 Science 331 6164 Google Scholar
[9]	Xia Q, Tao J, Cai J, Fu L, Liu J 2018 Phys. Rev. Lett. 121 143201 Google Scholar
[10]	Du H, Li J, Wang H, Yue S, Wu H, Hu B 2017 Phys. Lett. A 381 1563 Google Scholar
[11]	Chen F, Yao R, Luo J, Wang C 2018 Chin. Phys. B 27 103202 Google Scholar
[12]	Marchenko T, Huismans Y, Schafer K J, Vrakking M J J 2011 Phys. Rev. A 84 053427 Google Scholar
[13]	Bian X B, Huismans Y, Smirnova O, Yuan K J, Vrakking M J J, Bandrauk A D 2011 Phys. Rev. A 84 043420 Google Scholar
[14]	Bian X B, Bandrauk A D, 2012 Phys. Rev. Lett. 108 263003 Google Scholar
[15]	Song X, Lin C, Sheng Z, Liu P, Chen Z, Yang W, Hu S, Lin C D, Chen J 2016 Sci. Rep. 6 28392 Google Scholar
[16]	林呈, 张华堂, 盛志浩, 余显环, 刘鹏, 徐竟文, 宋晓红, 胡师林, 陈京, 杨玮枫 2016 65 223207 Google Scholar Lin C, Zhang H J, Sheng Z H, Yu X H, Liu P, Xu J W, Song X H, Hu S L, Chen J, Yang W F 2016 Acta Phys. Sin. 65 223207 Google Scholar
[17]	Qin P, Sun X, Liu Y, Chen Z 2021 Phys. Rev. A 104 053111 Google Scholar
[18]	Yu S, Lai X, Wang Y, Xu S, Hua L, Quan W, Liu X 2020 Phys. Rev. A 101 023414 Google Scholar
[19]	Zhou Y, Tolstikhin O I, Morishita T 2016 Phys. Rev. Lett. 116 173001 Google Scholar
[20]	Hickstein D D, Ranitovic P, Witte S, Tong X M, Huismans Y, Arpin P, Zhou X, Keister K E, Hogle C W, Zhang B, Ding C, Johnsson P, Toshima N, Vrakking M J J, Murnane M M, Kapteyn H C 2012 Phys. Rev. Lett. 109 073004 Google Scholar
[21]	Zhou Y, Tan J, Li M, Lu P 2021 Sci. China Phys. Mech. Astron. 64 273011 Google Scholar
[22]	Meckel M, Staudte A, Patchkovskii S, Villeneuve D M, Corkum P B, Drner R, Spanner M 2014 Nat. Phys. 10 594 Google Scholar
[23]	Liu M M, Li M, Wu C, Gong Q, Staudte A, Liu Y 2016 Phys. Rev. Lett. 116 163004 Google Scholar
[24]	Li M, Xie H, Cao W, Luo S, Tan J, Feng Y, Du B, Zhang W, Li Y, Zhang Q, Lan P, Zhou Y, Lu P X 2019 Phys. Rev. Lett. 122 183202 Google Scholar
[25]	Tan J, Zhou Y, He M, Chen Y, Ke Q, Liang J, Zhu X, Li M, Lu P X 2018 Phys. Rev. Lett. 121 253203 Google Scholar
[26]	Tan J, Zhou Y, He M, Ke Q, Liang J T, Li Y, Li M, Lu P X 2019 Phys. Rev. A 99 033402 Google Scholar
[27]	Haertelt M, Bian X B, Spanner M, Staudte A, Corkum P B 2016 Phys. Rev. Lett. 116 133001 Google Scholar
[28]	Porat G, Alon G, Rozen S, Pedatzur O, Krüger M, Azoury D, Natan A, Orenstein G, Bruner B D, Vrakking M J J, Dudovich N 2018 Nat. Commun 9 2805 Google Scholar
[29]	He M, Li Y, Zhou Y, Li M, Cao W, Lu P 2018 Phys. Rev. Lett. 120 133204 Google Scholar
[30]	Walt S G, Bhargava Ram N, Atala M, Shvetsov Shilovski N I, von Conta A, Baykusheva D, Lein M, Worner H J 2017 Nat. Commun. 8 15651 Google Scholar
[31]	Hasovic E, Becker W, Milosević D B 2016 Opt. Express 24 6413 Google Scholar
[32]	Huang C, Pang H, Huang X, Zhong M, Wu Z 2020 Opt. Express 28 10505 Google Scholar
[33]	Li B, Yang X, Ren X, Zhang J 2019 Opt. Express 27 32700 Google Scholar
[34]	Xu T, Zhu Q, Chen J, Ben S, Zhang J, Liu X 2018 Opt. Express 26 1645 Google Scholar
[35]	曾雪, 苏杰, 黄雪飞, 庞惠玲, 黄诚 2021 70 243201 Google Scholar Zeng X, Su J, Huang X F, Pang H L, Huang C 2021 Acta Phys. Sin. 70 243201 Google Scholar
[36]	Li M, Jiang W C, Xie H, Luo S, Zhou Y M, Lu P X 2018 Phys. Rev. A 97 023415 Google Scholar
[37]	Ke Q H, Zhou Y M, Tan J, He M R, Liang J T, Zhao Y, Li M, Lu P X 2019 Opt. Express 27 32193 Google Scholar
[38]	Feit M D, Fleck J A, Steiger A 1982 J. Comput. Phys. 47 412 Google Scholar
[39]	Tong X M, Hino K, Toshima N 2006 Phys. Rev. A 74 031405 Google Scholar
[40]	Liao Q, Winney A H, Lee S K, Lin Y, Adhikari P, Li W 2017 Phys. Rev. A 96 023401 Google Scholar
[41]	Lehtovaara L, Toivanen J, Eloranta J 2007 J. Comput. Phys. 221 148 Google Scholar
[42]	Lewenstein M, Balcou P, Ivanov M Yu, Anne L'Huillier, Corkum P B 1994 Phys. Rev. A 49 2117 Google Scholar
[43]	Milošević D B, Becker W 2002 Phys. Rev. A 66 063417 Google Scholar

施引文献

图 1 (a), (b)反向旋转TCEP复合电场(虚线)及其负矢势(实线); (c), (d)反向旋转TCEP场中Ar电离电子的末态动量分布; 其中(a), (c)相对相位为φ = 0.25π; (b), (d)相对相位φ = 0; 两椭偏场的椭偏率均为0.3

Fig. 1. (a), (b) Combined laser electric field E(t) (dashed curve) and the corresponding negative vector potential－A(t) (solid curve) for counter-rotating TCEP fields; (c), (d) photoelectron momentum distributions of Ar ionized by counter-rotating TCEP fields. (a), (c) The relative phase is φ = 0.25π; (b), (d) The relative phase is φ = 0. Both ellipticities of the two pulses are 0.3.

下载: 全尺寸图片幻灯片

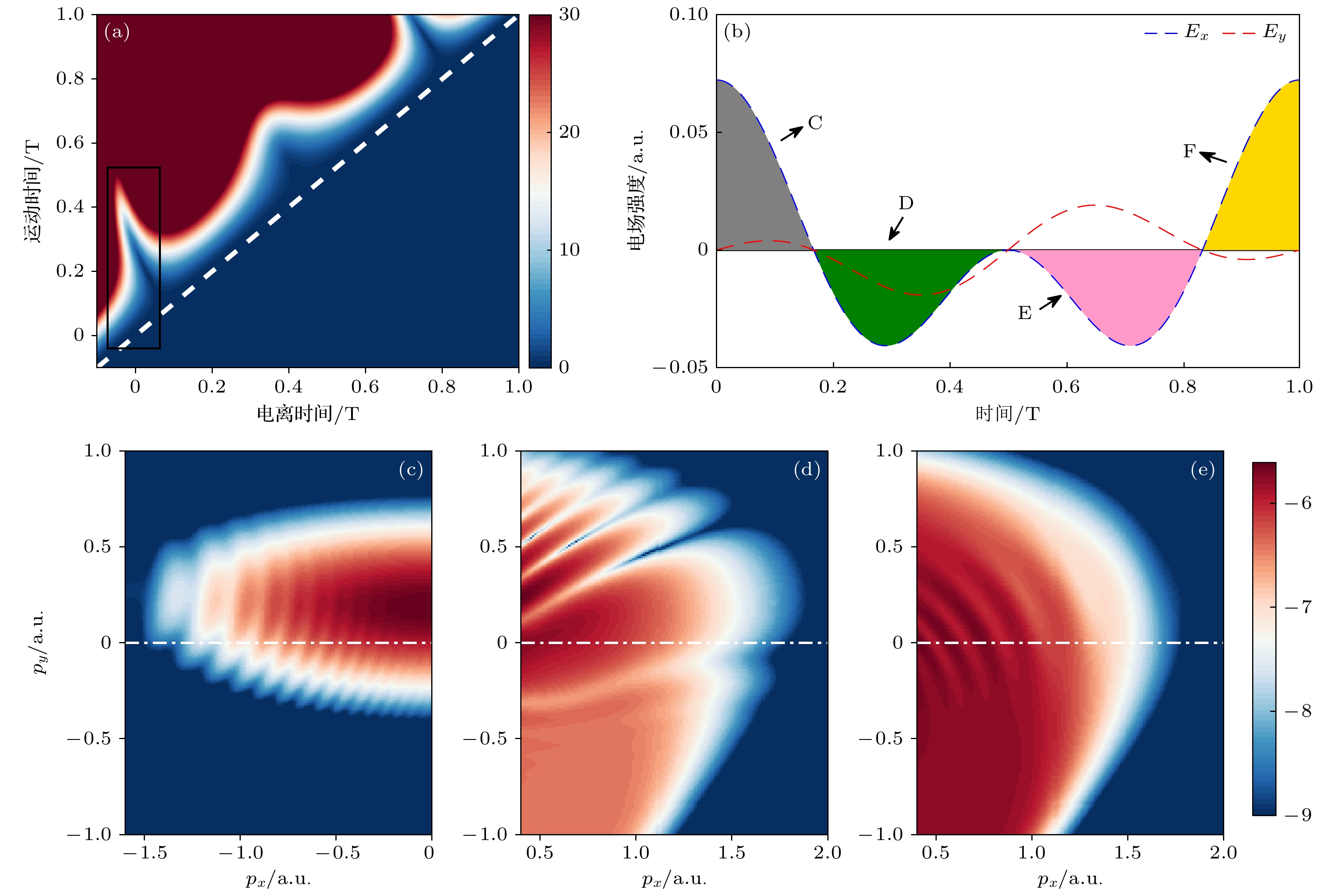
图 2 (a) 不同时刻电离电子经典轨迹中电子离母离子距离的时间演化; (b) 反向旋转TCEP场的x分量(蓝虚线)和y分量(红虚线); (c) SFA计算所得直接电子波包A和直接电子波包B形成的干涉图样; (d) SFA计算所得前向散射电子波包A和直接电子波包A形成的干涉图样; (e) SFA计算所得前向散射电子波包A和直接电子波包B形成的干涉图样. 两椭偏场的椭偏率均为0.3. 相对相位φ = 0.25π

Fig. 2. (a) Time evolutions of the distances between the electron and the parent ion for different ionization times; (b) the x and y components of counter-rotating TCEP fields; (c) the simulated interference pattern between the direct electrons ionized during A and the direct electrons ionized during B with SFA; (d) the simulated interference pattern between the rescattering electrons and the direct electrons ionized during A with SFA; (e) the simulated interference pattern between the rescattering electrons ionized during A and the direct electrons ionized during B with SFA. Both ellipticities of the two pulses are 0.3. The relative phase is 0.25π.

下载: 全尺寸图片幻灯片

图 3 (a) 不同时刻电离电子经典轨迹中电子离母离子距离的时间演化; (b) 反向旋转TCEP场的x分量(蓝虚线)和y分量(红虚线); (c) SFA计算所得直接电子波包E和直接电子波包F形成的干涉图样; (d) SFA计算所得前向散射电子波包C和直接电子波包C形成的干涉图样; (e) SFA计算所得前向散射电子波包C和直接电子波包D形成的干涉图样. 两椭偏场的椭偏率均为0.3. 相对相位φ = 0

Fig. 3. (a) Time evolutions of the distances between the electron and the parent ion for different ionization times; (b) the x and y components of counter-rotating TCEP fields; (c) the simulated interference pattern between the direct electrons ionized during C and the direct electrons ionized during D with SFA; (d) the simulated interference pattern between the rescattering electrons and the direct electrons ionized during C with SFA; (e) the simulated interference pattern between the rescattering electrons during C and the direct electrons ionized during D with SFA. Both ellipticities of the two pulses are 0.3. The relative phase is 0.

下载: 全尺寸图片幻灯片

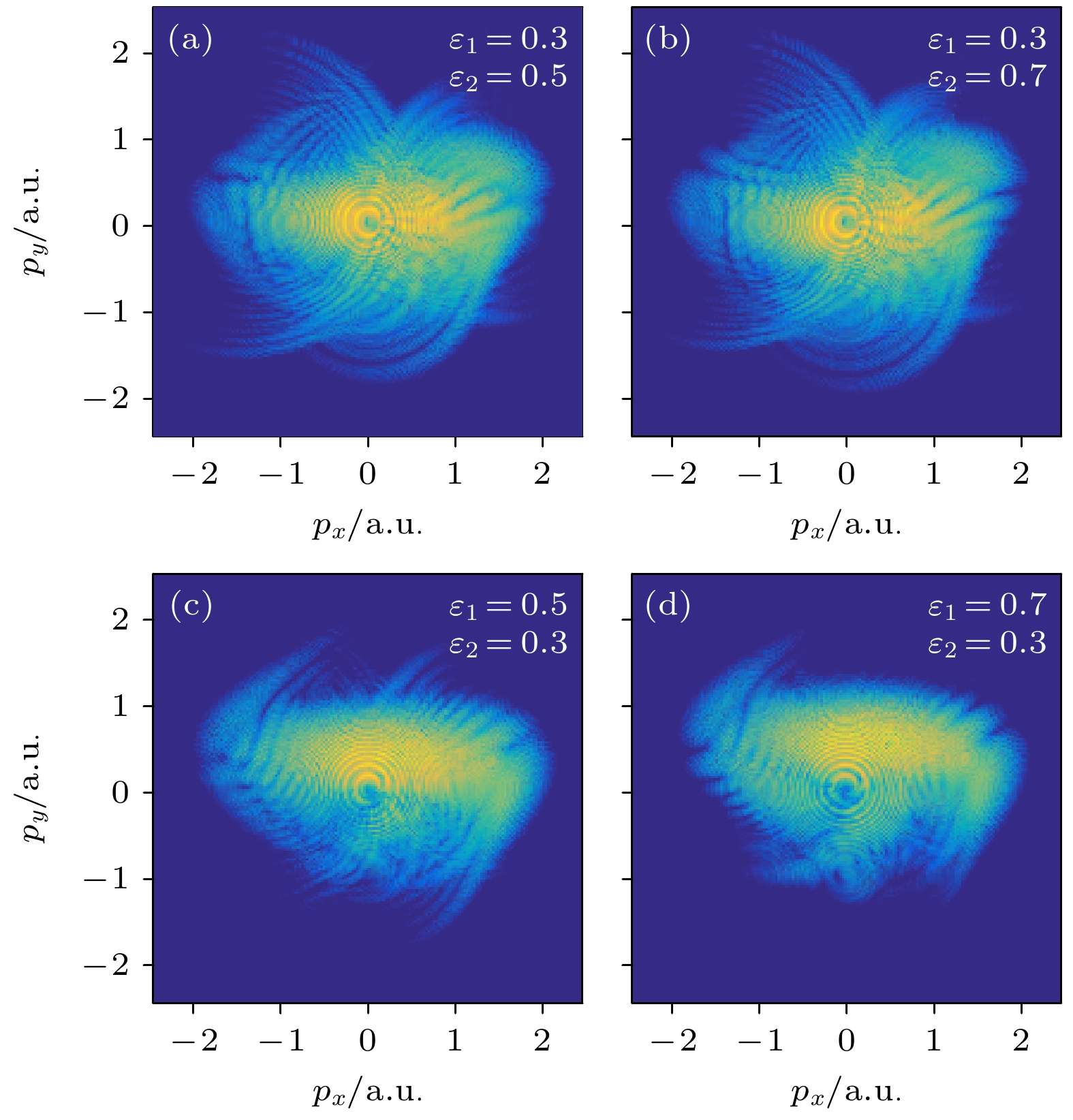
图 4 反向旋转TCEP场驱动Ar原子隧穿电离电子末态动量分布, 其中两椭偏场的相对相位φ = 0; 两脉冲椭偏率分别为(a) $\varepsilon_1 $ = 0.3, $\varepsilon_2 $ = 0.5; (b) $\varepsilon_1 $ = 0.3, $\varepsilon_2 $ = 0.7; (c) $\varepsilon_1 $ = 0.5, $\varepsilon_2 $ = 0.3; (d) $\varepsilon_1 $ = 0.7, $\varepsilon_2 $ = 0.3

Fig. 4. Photoelectron momentum distributions of Ar ionized by counter-rotating TCEP fields. The relative phase is 0. The ellipticities of the two pulses: (a) $\varepsilon_1 $ = 0.3, $\varepsilon_2 $ = 0.5; (b) $\varepsilon_1 $ = 0.3, $\varepsilon_2 $ = 0.7; (c) $\varepsilon_1 $ = 0.5, $\varepsilon_2 $ = 0.3; (d) $\varepsilon_1 $ = 0.7, $\varepsilon_2 $ = 0.3.

下载: 全尺寸图片幻灯片

map

[1]	Corkum P 1993 Phys. Rev. Lett. 71 1994 Google Scholar
[2]	Agostini P, Fabre F, Mainfray G, Petite G 1979 Phys. Rev. Lett. 42 1127 Google Scholar
[3]	Arbó D G, Persson E, Burgdörfer J 2006 Phys. Rev. A 74 063407 Google Scholar
[4]	Tan J, Xu S, Han X, Zhou Y, Li M, Cao W, Zhang Q, Lu P 2021 Adv. Photonics 3 035001 Google Scholar
[5]	Li M, Zhang P, Luo S Q, Zhou Y M, Zhang Q B, Lan P F, Lu P X 2015 Phys. Rev. A 92 063404 Google Scholar
[6]	Yang W, Zhang H, Lin C, Xu J, Sheng Z, Song X, Hu S, Chen J 2016 Phys. Rev. A 94 043419 Google Scholar
[7]	Wang Y, Yu S, Lai X, Kang H, Xu S, Sun R, Quan W, Liu X 2018 Phys. Rev. A 98 043422 Google Scholar
[8]	Huismans Y, Rouzée A, Gijsbertsen A, Jungmann J H, Smolkowska A S, Logman P S W M, Lépine F, Cauchy C, Zamith S, Marchenko T, Bakker J M, Berden G, Redlich B, van der Meer A F G, Muller H G, Vermin W, Schafer K J, Spanner M, Ivanov M Yu, Smirnova O, Bauer D, Popruzhenko S V, Vrakking M J J 2011 Science 331 6164 Google Scholar
[9]	Xia Q, Tao J, Cai J, Fu L, Liu J 2018 Phys. Rev. Lett. 121 143201 Google Scholar
[10]	Du H, Li J, Wang H, Yue S, Wu H, Hu B 2017 Phys. Lett. A 381 1563 Google Scholar
[11]	Chen F, Yao R, Luo J, Wang C 2018 Chin. Phys. B 27 103202 Google Scholar
[12]	Marchenko T, Huismans Y, Schafer K J, Vrakking M J J 2011 Phys. Rev. A 84 053427 Google Scholar
[13]	Bian X B, Huismans Y, Smirnova O, Yuan K J, Vrakking M J J, Bandrauk A D 2011 Phys. Rev. A 84 043420 Google Scholar
[14]	Bian X B, Bandrauk A D, 2012 Phys. Rev. Lett. 108 263003 Google Scholar
[15]	Song X, Lin C, Sheng Z, Liu P, Chen Z, Yang W, Hu S, Lin C D, Chen J 2016 Sci. Rep. 6 28392 Google Scholar
[16]	林呈, 张华堂, 盛志浩, 余显环, 刘鹏, 徐竟文, 宋晓红, 胡师林, 陈京, 杨玮枫 2016 65 223207 Google Scholar Lin C, Zhang H J, Sheng Z H, Yu X H, Liu P, Xu J W, Song X H, Hu S L, Chen J, Yang W F 2016 Acta Phys. Sin. 65 223207 Google Scholar
[17]	Qin P, Sun X, Liu Y, Chen Z 2021 Phys. Rev. A 104 053111 Google Scholar
[18]	Yu S, Lai X, Wang Y, Xu S, Hua L, Quan W, Liu X 2020 Phys. Rev. A 101 023414 Google Scholar
[19]	Zhou Y, Tolstikhin O I, Morishita T 2016 Phys. Rev. Lett. 116 173001 Google Scholar
[20]	Hickstein D D, Ranitovic P, Witte S, Tong X M, Huismans Y, Arpin P, Zhou X, Keister K E, Hogle C W, Zhang B, Ding C, Johnsson P, Toshima N, Vrakking M J J, Murnane M M, Kapteyn H C 2012 Phys. Rev. Lett. 109 073004 Google Scholar
[21]	Zhou Y, Tan J, Li M, Lu P 2021 Sci. China Phys. Mech. Astron. 64 273011 Google Scholar
[22]	Meckel M, Staudte A, Patchkovskii S, Villeneuve D M, Corkum P B, Drner R, Spanner M 2014 Nat. Phys. 10 594 Google Scholar
[23]	Liu M M, Li M, Wu C, Gong Q, Staudte A, Liu Y 2016 Phys. Rev. Lett. 116 163004 Google Scholar
[24]	Li M, Xie H, Cao W, Luo S, Tan J, Feng Y, Du B, Zhang W, Li Y, Zhang Q, Lan P, Zhou Y, Lu P X 2019 Phys. Rev. Lett. 122 183202 Google Scholar
[25]	Tan J, Zhou Y, He M, Chen Y, Ke Q, Liang J, Zhu X, Li M, Lu P X 2018 Phys. Rev. Lett. 121 253203 Google Scholar
[26]	Tan J, Zhou Y, He M, Ke Q, Liang J T, Li Y, Li M, Lu P X 2019 Phys. Rev. A 99 033402 Google Scholar
[27]	Haertelt M, Bian X B, Spanner M, Staudte A, Corkum P B 2016 Phys. Rev. Lett. 116 133001 Google Scholar
[28]	Porat G, Alon G, Rozen S, Pedatzur O, Krüger M, Azoury D, Natan A, Orenstein G, Bruner B D, Vrakking M J J, Dudovich N 2018 Nat. Commun 9 2805 Google Scholar
[29]	He M, Li Y, Zhou Y, Li M, Cao W, Lu P 2018 Phys. Rev. Lett. 120 133204 Google Scholar
[30]	Walt S G, Bhargava Ram N, Atala M, Shvetsov Shilovski N I, von Conta A, Baykusheva D, Lein M, Worner H J 2017 Nat. Commun. 8 15651 Google Scholar
[31]	Hasovic E, Becker W, Milosević D B 2016 Opt. Express 24 6413 Google Scholar
[32]	Huang C, Pang H, Huang X, Zhong M, Wu Z 2020 Opt. Express 28 10505 Google Scholar
[33]	Li B, Yang X, Ren X, Zhang J 2019 Opt. Express 27 32700 Google Scholar
[34]	Xu T, Zhu Q, Chen J, Ben S, Zhang J, Liu X 2018 Opt. Express 26 1645 Google Scholar
[35]	曾雪, 苏杰, 黄雪飞, 庞惠玲, 黄诚 2021 70 243201 Google Scholar Zeng X, Su J, Huang X F, Pang H L, Huang C 2021 Acta Phys. Sin. 70 243201 Google Scholar
[36]	Li M, Jiang W C, Xie H, Luo S, Zhou Y M, Lu P X 2018 Phys. Rev. A 97 023415 Google Scholar
[37]	Ke Q H, Zhou Y M, Tan J, He M R, Liang J T, Zhao Y, Li M, Lu P X 2019 Opt. Express 27 32193 Google Scholar
[38]	Feit M D, Fleck J A, Steiger A 1982 J. Comput. Phys. 47 412 Google Scholar
[39]	Tong X M, Hino K, Toshima N 2006 Phys. Rev. A 74 031405 Google Scholar
[40]	Liao Q, Winney A H, Lee S K, Lin Y, Adhikari P, Li W 2017 Phys. Rev. A 96 023401 Google Scholar
[41]	Lehtovaara L, Toivanen J, Eloranta J 2007 J. Comput. Phys. 221 148 Google Scholar
[42]	Lewenstein M, Balcou P, Ivanov M Yu, Anne L'Huillier, Corkum P B 1994 Phys. Rev. A 49 2117 Google Scholar
[43]	Milošević D B, Becker W 2002 Phys. Rev. A 66 063417 Google Scholar

[1]	李观荣, 郑怡婷, 徐琼怡, 裴笑山, 耿玥, 严冬, 杨红. 闭合回路相干增益原子系统中完美非互易反射光放大. , 2024, 73(12): 126401. doi: 10.7498/aps.73.20240347
[2]	徐耀坤, 孙仕海, 曾瑶源, 杨俊刚, 盛卫东, 刘伟涛. 基于双光子干涉的量子全息理论框架. , 2023, 72(21): 214207. doi: 10.7498/aps.72.20231242
[3]	车佳殷, 陈超, 李卫艳, 李维, 陈彦军. 强场原子电离响应时间的研究进展. , 2023, 72(19): 193301. doi: 10.7498/aps.72.20230983
[4]	赵猛, 全威, 肖智磊, 许松坡, 王志强, 王明辉, 成思进, 吴文卓, 王艳兰, 赖炫扬, 柳晓军. 强激光场驱动Ar原子电离中的隧穿延时. , 2022, 71(23): 233203. doi: 10.7498/aps.71.20221295
[5]	苏杰, 刘子超, 廖健颖, 李盈傧, 黄诚. 反旋双色椭偏场中Ar非次序双电离电子关联的强度依赖. , 2022, 71(19): 193201. doi: 10.7498/aps.71.20221044
[6]	沈星晨, 刘洋, 陈淇, 吕航, 徐海峰. 超快强激光场中原子分子的里德伯态激发. , 2022, 71(23): 233202. doi: 10.7498/aps.71.20221258
[7]	陶建飞, 夏勤智, 廖临谷, 刘杰, 刘小井. 强激光场原子电离光电子轨迹干涉全息理论及应用. , 2022, 71(23): 233206. doi: 10.7498/aps.71.20221296
[8]	曾雪, 苏杰, 黄雪飞, 庞惠玲, 黄诚. 同向旋转双色圆偏场中非次序双电离的频率比依赖. , 2021, 70(24): 243201. doi: 10.7498/aps.70.20211112
[9]	吴广智, 王强, 周沧涛, 傅立斌. 双势阱产生正负电子对过程中的正电子波干涉与克莱因隧穿现象. , 2017, 66(7): 070301. doi: 10.7498/aps.66.070301
[10]	林呈, 张华堂, 盛志浩, 余显环, 刘鹏, 徐竟文, 宋晓红, 胡师林, 陈京, 杨玮枫. 用推广的量子轨迹蒙特卡罗方法研究强场光电子全息. , 2016, 65(22): 223207. doi: 10.7498/aps.65.223207
[11]	郭丽, 韩申生, 陈京. 利用类维格纳分布函数方法研究阈上电离. , 2016, 65(22): 223203. doi: 10.7498/aps.65.223203
[12]	赵磊, 张琦, 董敬伟, 吕航, 徐海峰. 不同原子在飞秒强激光场中的里德堡态激发和双电离. , 2016, 65(22): 223201. doi: 10.7498/aps.65.223201
[13]	刘灿东, 贾正茂, 郑颖辉, 葛晓春, 曾志男, 李儒新. 双色场控制与测量原子分子超快电子动力学过程的研究进展. , 2016, 65(22): 223206. doi: 10.7498/aps.65.223206
[14]	王艳海. 强场隧穿电离模式下的氦原子电离时间问题研究. , 2016, 65(15): 153201. doi: 10.7498/aps.65.153201
[15]	吕志忠, 张天祺, 钟功祥. 双色场诱导气体产生相干可控的四次谐波. , 2015, 64(17): 174204. doi: 10.7498/aps.64.174204
[16]	李春雷, 徐燕, 张燕翔, 叶宝生. 双量子阱中光子辅助电子自旋隧穿. , 2013, 62(10): 107301. doi: 10.7498/aps.62.107301
[17]	周青春, 狄尊燕. 声子对隧穿量子点分子辐射场系统量子相位的影响. , 2013, 62(13): 134206. doi: 10.7498/aps.62.134206
[18]	黄芳, 李海彬. 双势阱中玻色-爱因斯坦凝聚的绝热隧穿. , 2011, 60(2): 020303. doi: 10.7498/aps.60.020303
[19]	周远明, 俞国林, 高矿红, 林铁, 郭少令, 褚君浩, 戴宁. 弱耦合GaAs/AlGaAs/InGaAs双势阱隧穿结构的磁隧穿特性研究. , 2010, 59(6): 4221-4225. doi: 10.7498/aps.59.4221
[20]	王训春, 邱锡钧, 郑丽萍. 相对相位对双色激光场中线性多原子分子离子增强电离行为的影响. , 2001, 50(11): 2155-2158. doi: 10.7498/aps.50.2155

计量

文章访问数: 4246
PDF下载量: 87
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

反向旋转双色椭偏场中原子隧穿电离电子的全息干涉