-
阿秒钟实验方案是当前研究原子分子体系的价电子在强激光场中隧穿延时问题的有效手段. 基于阿秒钟方案, 本文实验研究了Ar原子在800 nm椭圆偏振激光场中的光电子动量分布随激光光强的演化规律. 理论上采用包含库仑场效应、非绝热效应、Stark效应、多电子屏蔽和极化效应的半经典模型对Ar原子的强场电离动力学进行了模拟. 通过对比实验测量和数值模拟结果发现, 在本文所研究的光强范围内, Ar原子的价电子在800 nm椭圆偏振激光场中隧穿延时上限为10 as. 进一步分析表明, 阿秒钟方案中, 考虑多电子屏蔽效应对得到的隧穿延时影响最小, 而考虑非绝热效应的影响最大.“Attoclock” provides a promising experimental scheme to explore the timing of tunnel ionization of atoms and molecules in intense laser fields. In this work, we perform a systematical investigation of tunneling delay time in strong field ionization of atomic Ar, based on the “attoclock” experimental scheme. Experimentally, the laser intensity dependence of the photoelectron momentum distributions of Ar subject to strong elliptically polarized laser fields at 800 nm has been measured. Theoretically, a dedicated semiclassical model, in which the Coulomb potential effect, the nonadiabatic effect, the Stark effect, the multielectron screening and polarization effect have been well considered, is employed to simulate the ionization dynamics of Ar. By comparing the experimental and simulated results, an upper limit of 10 attoseconds for the tunneling delay time of Ar has been derived for the laser intensity ranges explored in this work. In addition, the influence of various physical effects on the extracted tunneling delay time, in the context of semiclassical model, has been analyzed. It is demonstrated that, under otherwise identical conditions, consideration of multielectron screening effect will give rise to the least change of the extracted tunneling delay time. In contrast, consideration of nonadiabatic effect will lead to the most significant change of the extracted tunneling delay time.
-
Keywords:
- attoclock /
- tunneling delay time /
- ultrafast ionization
[1] MacColl L A 1932 Phys. Rev. 40 621
Google Scholar
[2] Schultze M, Fieß M, Karpowicz N, et al. 2010 Science 328 1658
Google Scholar
[3] Shafir D, Soifer H, Bruner B D, Dagan M, Mairesse Y, Patchkovskii S, Ivanov M Y, Smirnova O, Dudovich N 2012 Nature 485 343
Google Scholar
[4] Goulielmakis E, Loh Z, Wirth A, Santra R, Rohringer N, Yakovlev V S, Zherebtsov S, Pfeifer T, Azzeer A M, Kling M F, Leone S R, Krausz F 2010 Nature 466 739
Google Scholar
[5] Eckle P, Pfeiffer A N, Cirelli C, Staudte A, Dörner R, Muller H G, Büttiker M, Keller U 2008 Science 322 1525
Google Scholar
[6] Eckle P, Smolarski M, Schlup P, Biegert J, Staudte A, Schöffler M, Muller H G, Dörner R, Keller U 2008 Nat. Phys. 4 565
Google Scholar
[7] Pfeiffer A N, Cirelli C, Smolarski M, Wang X, Eberly J H, Dörner R, Keller U 2011 New J. Phys. 13 093008
Google Scholar
[8] Pfeiffer A N, Cirelli C, Smolarski M, Dimitrovski D, Abu-samha M, Madsen L B, Keller U 2012 Nat. Phys. 8 76
Google Scholar
[9] Sainadh U S, Xu H, Wang X, Atia-Tul-Noor A, Wallace W C, Douguet N, Bray A, Ivanov I, Bartschat K, Kheifets A, Sang R T, Litvinyuk I V 2019 Nature 568 75
Google Scholar
[10] Han M, Ge P, Wang J, Guo Z, Fang Y, Ma X, Yu X, Deng Y, Wörner H J, Gong Q, Liu Y 2021 Nat. Photonics 15 765
Google Scholar
[11] Torlina L, Morales F, Kaushal J, Ivanov I, Kheifets A, Zielinski A, Scrinzi A, Muller H G, Sukiasyan S, Ivanov M, Smirnova O 2015 Nat. Phys. 11 503
Google Scholar
[12] Quan W, Serov V V, Wei M, Zhao M, Zhou Y, Wang Y, Lai X, Kheifets A S, Liu X 2019 Phys. Rev. Lett. 123 223204
Google Scholar
[13] Landsman A S, Weger M, Maurer J, Boge R, Ludwig A, Heuser S, Cirelli C, Gallmann L, Keller U 2014 Optica 1 343
Google Scholar
[14] Landsman A S, Keller U 2015 Phys. Rep. 547 1
Google Scholar
[15] Camus N, Yakaboylu E, Fechner L, Klaiber M, Laux M, Mi Y, Hatsagortsyan K Z, Pfeifer T, Keitel C H, Moshammer R 2017 Phys. Rev. Lett. 119 023201
Google Scholar
[16] Quan W, Yuan M, Yu S, Xu S, Chen Y, Wang Y, Sun R, Xiao Z, Gong C, Hua L, Lai X, Liu X, Chen J 2016 Opt. Express 24 23248
Google Scholar
[17] Zhi-Lei Xiao, Wei Quan, Song-Po Xu, Shao-Gang Yu, Xuan-Yang Lai, Jing Chen, Liu X-J 2020 Chin. Phys. Lett. 37 043201
Google Scholar
[18] Xiao Z, Quan W, Xu S, Yu S, Wang Y, Zhao M, Wei M, Zhou Y, Lai X, Chen J, Liu X 2020 Chin. Opt. Lett. 18 010201
Google Scholar
[19] Xiao Z, Quan W, Yu S, Lai X, Liu X, Wei Z, Chen J 2022 Opt. Express 30 14873
Google Scholar
[20] Quan W, Hao X, Chen Y, Yu S, Xu S, Wang Y, Sun R, Lai X, Wu C, Gong Q, He X, Liu X, Chen J 2016 Sci. Rep. 6 27108
Google Scholar
[21] Chen J, Liu J, Chen S G 2000 Phys. Rev. A 61 033402
Google Scholar
[22] Hao X, Wang G, Jia X, Li W, Liu J, Chen J 2009 Phys. Rev. A 80 023408
Google Scholar
[23] Quan W, Lin Z, Wu M, Kang H, Liu H, Liu X, Chen J, Liu J, He X T, Chen S G, Xiong H, Guo L, Xu H, Fu Y, Cheng Y, Xu Z Z 2009 Phys. Rev. Lett. 103 093001
Google Scholar
[24] Wang Y, Xu S, Chen Y, et al. 2017 Phys. Rev. A 95 063415
Google Scholar
[25] Ammosov M V, Delone N B, Krainov V P 1986 Sov. Phys. JETP 664 138
[26] Delone N B, Krainov V P 1991 J. Opt. Soc. Am. B 8 1207
Google Scholar
[27] Ye D F, Liu X, Liu J 2008 Phys. Rev. Lett. 101 233003
Google Scholar
[28] Chen J, Liu J, Zheng W M 2002 Phys. Rev. A 66 043410
Google Scholar
[29] Fu L B, Liu J, Chen J, Chen S G 2001 Phys. Rev. A 63 043416
Google Scholar
[30] Chen J, Liu J, Fu L B, Zheng W M 2000 Phys. Rev. A 63 011404
Google Scholar
[31] Brabec T, Ivanov M Y, Corkum P B 1996 Phys. Rev. A 54 R2551
Google Scholar
[32] Hu B, Liu J, Chen S G 1997 Phys. Lett. A 236 533
Google Scholar
[33] Han M, Li M, Liu M-M, Liu Y 2017 Phys. Rev. A 95 023406
Google Scholar
[34] Li M, Liu M M, Geng J W, Han M, Sun X, Shao Y, Deng Y, Wu C, Peng L Y, Gong Q, Liu Y 2017 Phys. Rev. A 95 053425
Google Scholar
[35] Becker W, Grasbon F, Kopold R, Milošević D B, Paulus G G, Walther H 2002 Adv. At. Mol. Opt. Phys. 48 35
Google Scholar
[36] Salières P, Carré B, Le Déroff L, Grasbon F, Paulus G G, Walther H, Kopold R, Becker W, Milošević D B, Sanpera A, Lewenstein M 2001 Science 292 902
Google Scholar
[37] Cloux F, Fabre B, Pons B 2015 Phys. Rev. A 91 023415
Google Scholar
[38] Muller H G 1999 Phys. Rev. A 60 1341
Google Scholar
[39] Dimitrovski D, Martiny C P J, Madsen L B 2010 Phys. Rev. A 82 053404
Google Scholar
[40] Dimitrovski D, Abu-samha M, Madsen L B, Filsinger F, Meijer G, Küpper J, Holmegaard L, Kalhøj L, Nielsen J H, Stapelfeldt H 2011 Phys. Rev. A 83 023405
Google Scholar
[41] Xu S, Liu M, Hu S, Shu Z, Quan W, Xiao Z, Zhou Y, Wei M, Zhao M, Sun R, Wang Y, Hua L, Gong C, Lai X, Chen J, Liu X 2020 Phys. Rev. A 102 043104
Google Scholar
[42] Corkum P B, Burnett N H, Brunel F 1989 Phys. Rev. Lett. 62 1259
Google Scholar
[43] Corkum P B 1993 Phys. Rev. Lett. 71 1994
Google Scholar
[44] Hofmann C, Landsman A S, Keller U 2019 J. Mod. Opt. 66 1052
Google Scholar
-
图 1 (a) 实验测量的Ar原子光电子动量分布, 激光波长800 nm, 光强1.5×1014 W/cm2, 椭偏率0.7, 插图标识出激光场偏振面的长轴和短轴方向; (b) 黑色方框表示从(a)中提取的Ar原子光电子角度分布; (c) 半经典理论计算的光电子动量分布, 激光参数与(a)相同; (d) 黑色圆圈表示从(c)中提取的Ar原子光电子角度分布. (b)(d)中的红色曲线代表采用最小二乘法对实验测量和数值模拟数据的拟合结果
Fig. 1. (a) The measured photoelectron momentum distributions of Ar subject to the laser electric field with a wavelength of 800 nm, an intensity of 1.5×1014 W/cm2, and an ellipticity of 0.7, the inset shows the directions of the major and minor axes of the polarization ellipse of the laser field; (b) the black boxes indicate the photoelectron angular distribution extracted from the data in (a); (c) the photoelectron momentum distributions calculated by the semiclassical model with the laser parameters identical to those of (a); (d) the black circles represent the photoelectron angular distribution extracted from the data in (c). The red curves in (c) and (d) show the results of the least square fittings of experimental data and numerical calculations, respectively.
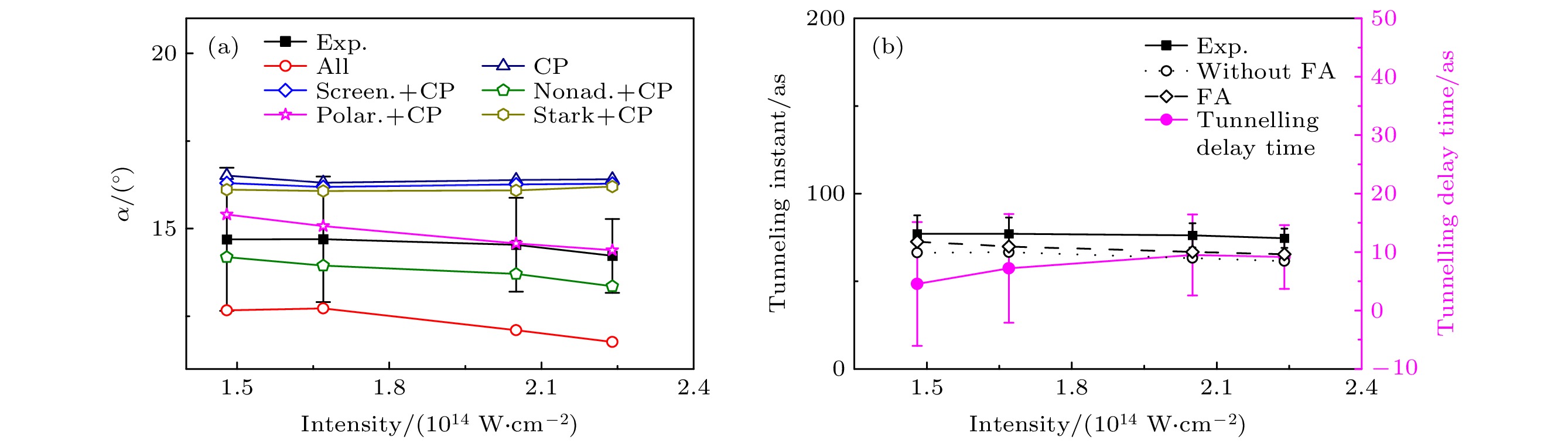
图 2 (a)实验测量与半经典理论计算得到的α随激光光强的依赖曲线; 黑色实心方块(Exp.)为实验测量结果. 海军蓝空心三角形(CP)为仅包含库仑场效应的计算结果、蓝色空心四边形(Screen.+CP)为包含库仑场效应和多电子屏蔽效应的计算结果、橄榄绿空心五边形(Nonad.+CP)为包含库仑场效应和非绝热效应的计算结果、洋红色空心五角星(Polar.+CP)为包含库仑场效应和多电子极化效应的计算结果、深黄色空心六边形(Stark+CP)为包含库仑场效应和Stark效应的计算结果, 红色空心圆形(All)为以上所有效应同时考虑的情况. (b) 由(a)中α得到的电子隧穿时刻随光强的依赖曲线. 黑色实心方块(Exp.)为实验结果, 黑色空心圆(Without FA)为单一光强计算结果, 黑色空心四边形(FA)为考虑聚焦平均效应的结果. 采用阿秒钟方案得到的隧穿延时随激光光强的变化由洋红色实心圆(Tunneling delay time)表示(右侧纵轴)
Fig. 2. (a) The measured and calculated intensity dependence of α. The filled black boxes (Exp.) show the experimental results. The open navy triangles (CP), open blue diamonds (Screen.+CP), open olive pentagons (Nonad.+CP), open magenta pentacles (Polar.+CP), open dark yellow hexagons (Stark+CP), and open red circles (All) indicate the semiclassical calculations where only the influence of Coulomb potential, the influence of both Coulomb potential and the multi-electron screening effect, the influence of both Coulomb potential and the non-adiabatic effect, the influence of both Coulomb potential and multi-electron polarization effect, the influence of both Coulomb potential and the Stark effect, and all the physical effects have been considred, respectively. (b) The laser intensity dependence of tunneling instant obtained from the measured and calculated α in (a). The filled black boxes (Exp.) represent the experimental data. The open black circles (Without FA) indicate the calculation without focusing average. The open black diamonds (FA) represent the calculation where the focusing average has been considered. The filled magenta circles (Tunneling delay time) indicate the intensity dependence of the tunneling delay time which is obtained based on the attoclock scheme.
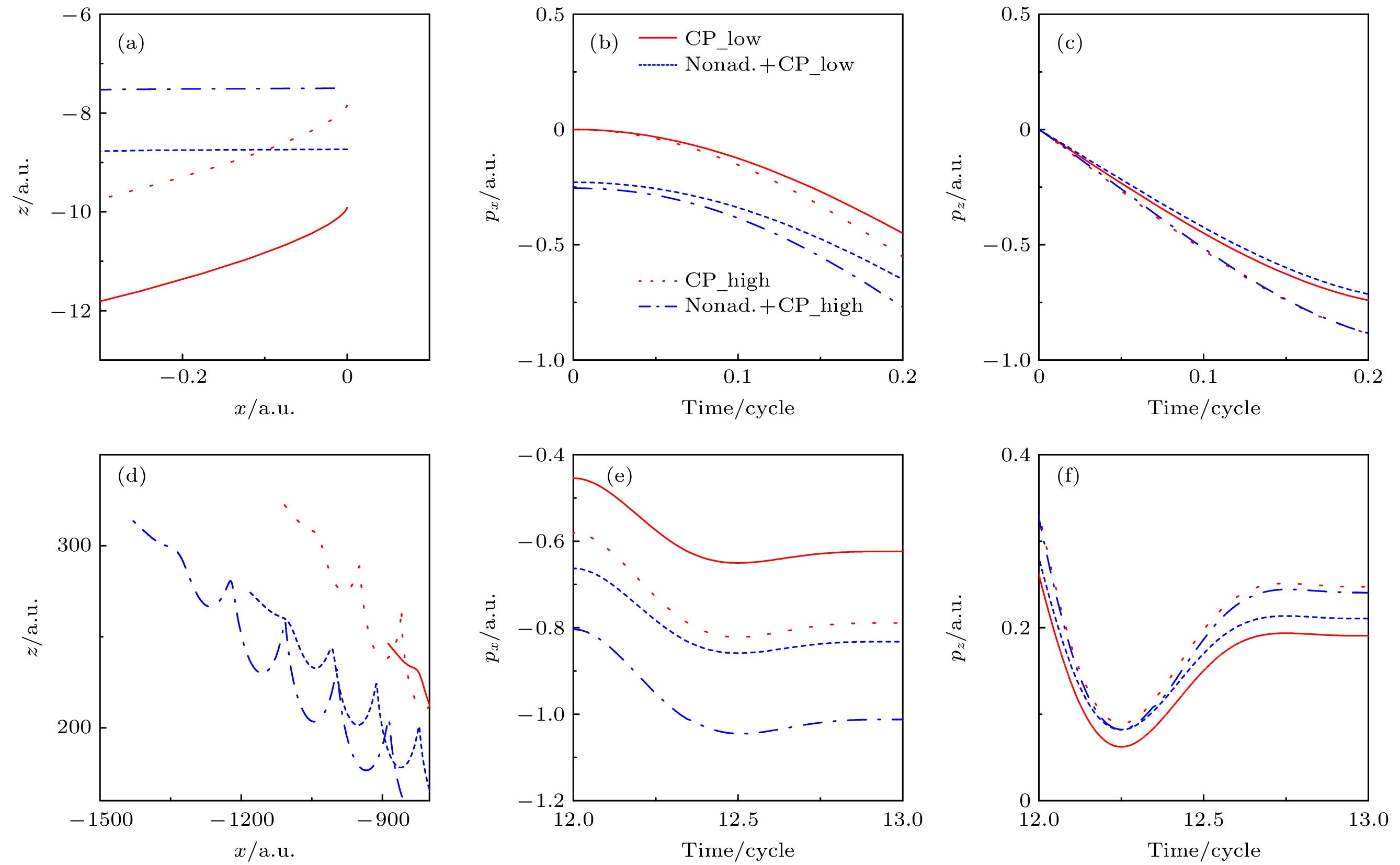
图 3 半经典理论计算的在激光峰值电场发生隧穿的电子轨道 (a), (d)隧穿刚发生时和激光脉冲快结束时电子轨道的空间演化; (b), (e)隧穿刚发生时和激光脉冲快结束时, 沿x方向电子动量(px)随时间的演化; (c), (f)隧穿刚发生时和激光脉冲快结束时, 沿z方向电子动量(pz)随时间的演化, 其中红色细实线和红色粗点线表示仅考虑库仑场效应的轨道, 蓝色细划线和蓝色粗点划线表示同时考虑库仑场和非绝热效应的轨道, 红色细实线和蓝色细划线表示激光光强为1.5 × 1014 W/cm2的计算结果, 红色粗点线和蓝色粗点划线表示激光光强为2.3 × 1014 W/cm2的计算结果
Fig. 3. The typical trajectories calculated by the semiclassical model for the photoelectrons tunneling from the peak of laser field: (a), (d) The spatial evolution of electron trajectories around the tunneling instant and the end of the laser pulse; (b), (e) the temporal evolution of photoelectron momenta along the x direction (px) around the tunneling instant and the end of the laser pulse; (c), (f) the temporal evolution of photoelectron momenta along z direction (pz) around the tunneling instant and the end of the laser pulse. The thin red solid and thick red dotted lines represent the trajectories calculated by the semiclassical model where only the influence of Coulomb potential is considered. The thin blue dashed and thick blue dot-dashed lines represent the trajectories calculated by the semiclassical model where the influence of both Coulomb potential and the nonadiabatic effect are considered. The thin red solid and thin blue dashed lines indicate the trajectories which are calculated at 1.5 × 1014 W/cm2. The thick red dotted and thick blue dot-dashed lines indicate the trajectories which are calculated at 2.3 × 1014 W/cm2.
图 4 (a), (c)半经典理论计算得到的激光峰值电场对应电子轨道在隧穿时刻(initial)电子的位置和动量; (b), (d)半经典理论计算得到的激光峰值电场对应电子轨道在脉冲结束时(final)电子的位置和动量; (d)中连接原点和对应符号的实线标示出电子的最终出射方向, 海军蓝三角形(CP)为仅包含库仑场效应的计算结果、蓝色四边形(Screen.+CP)为包含库仑场效应和多电子屏蔽效应的计算结果、橄榄绿五边形(Nonad.+CP)为包含库仑场效应和非绝热效应的计算结果、洋红色五角星(Polar.+CP)为包含库仑场效应和多电子极化效应的计算结果、深黄色六边形(Stark+CP)为包含库仑场效应和Stark效应的计算结果, 实心(Low)和空心(High)分别表示激光光强为1.5 × 1014W/cm2和2.3 × 1014 W/cm2的相应计算结果
Fig. 4. (a), (c) The calculated positions and the momenta of the photoelectrons tunneling from the peak of laser field at the tunneling instant (initial); (b), (d) the calculated positions and the momenta of the photoelectrons tunneling from the peak of laser field at the end of the laser pulse (final); in (d), the solid lines connecting the origin and the symbols indicate the corresponding emission angles of the photoelectrons at the end of the laser pulse. The navy triangles (CP), blue diamonds (Screen.+CP), olive pentagons (Nonad.+CP), magenta pentacles (Polar.+CP), and dark yellow hexagons (Stark+CP) indicate the semiclassical calculations where only the influence of Coulomb potential, the influence of both Coulomb potential and the multi-electron screening effect, the influence of both Coulomb potential and the non-adiabatic effect, the influence of both Coulomb potential and multi-electron polarization effect, and the influence of both Coulomb potential and the Stark effect have been considred, respectively. The filled (Low) and open (High) Symbols indicate the results calculated at 1.5 × 1014 W/cm2 and 2.3 × 1014 W/cm2, respectively.
-
[1] MacColl L A 1932 Phys. Rev. 40 621
Google Scholar
[2] Schultze M, Fieß M, Karpowicz N, et al. 2010 Science 328 1658
Google Scholar
[3] Shafir D, Soifer H, Bruner B D, Dagan M, Mairesse Y, Patchkovskii S, Ivanov M Y, Smirnova O, Dudovich N 2012 Nature 485 343
Google Scholar
[4] Goulielmakis E, Loh Z, Wirth A, Santra R, Rohringer N, Yakovlev V S, Zherebtsov S, Pfeifer T, Azzeer A M, Kling M F, Leone S R, Krausz F 2010 Nature 466 739
Google Scholar
[5] Eckle P, Pfeiffer A N, Cirelli C, Staudte A, Dörner R, Muller H G, Büttiker M, Keller U 2008 Science 322 1525
Google Scholar
[6] Eckle P, Smolarski M, Schlup P, Biegert J, Staudte A, Schöffler M, Muller H G, Dörner R, Keller U 2008 Nat. Phys. 4 565
Google Scholar
[7] Pfeiffer A N, Cirelli C, Smolarski M, Wang X, Eberly J H, Dörner R, Keller U 2011 New J. Phys. 13 093008
Google Scholar
[8] Pfeiffer A N, Cirelli C, Smolarski M, Dimitrovski D, Abu-samha M, Madsen L B, Keller U 2012 Nat. Phys. 8 76
Google Scholar
[9] Sainadh U S, Xu H, Wang X, Atia-Tul-Noor A, Wallace W C, Douguet N, Bray A, Ivanov I, Bartschat K, Kheifets A, Sang R T, Litvinyuk I V 2019 Nature 568 75
Google Scholar
[10] Han M, Ge P, Wang J, Guo Z, Fang Y, Ma X, Yu X, Deng Y, Wörner H J, Gong Q, Liu Y 2021 Nat. Photonics 15 765
Google Scholar
[11] Torlina L, Morales F, Kaushal J, Ivanov I, Kheifets A, Zielinski A, Scrinzi A, Muller H G, Sukiasyan S, Ivanov M, Smirnova O 2015 Nat. Phys. 11 503
Google Scholar
[12] Quan W, Serov V V, Wei M, Zhao M, Zhou Y, Wang Y, Lai X, Kheifets A S, Liu X 2019 Phys. Rev. Lett. 123 223204
Google Scholar
[13] Landsman A S, Weger M, Maurer J, Boge R, Ludwig A, Heuser S, Cirelli C, Gallmann L, Keller U 2014 Optica 1 343
Google Scholar
[14] Landsman A S, Keller U 2015 Phys. Rep. 547 1
Google Scholar
[15] Camus N, Yakaboylu E, Fechner L, Klaiber M, Laux M, Mi Y, Hatsagortsyan K Z, Pfeifer T, Keitel C H, Moshammer R 2017 Phys. Rev. Lett. 119 023201
Google Scholar
[16] Quan W, Yuan M, Yu S, Xu S, Chen Y, Wang Y, Sun R, Xiao Z, Gong C, Hua L, Lai X, Liu X, Chen J 2016 Opt. Express 24 23248
Google Scholar
[17] Zhi-Lei Xiao, Wei Quan, Song-Po Xu, Shao-Gang Yu, Xuan-Yang Lai, Jing Chen, Liu X-J 2020 Chin. Phys. Lett. 37 043201
Google Scholar
[18] Xiao Z, Quan W, Xu S, Yu S, Wang Y, Zhao M, Wei M, Zhou Y, Lai X, Chen J, Liu X 2020 Chin. Opt. Lett. 18 010201
Google Scholar
[19] Xiao Z, Quan W, Yu S, Lai X, Liu X, Wei Z, Chen J 2022 Opt. Express 30 14873
Google Scholar
[20] Quan W, Hao X, Chen Y, Yu S, Xu S, Wang Y, Sun R, Lai X, Wu C, Gong Q, He X, Liu X, Chen J 2016 Sci. Rep. 6 27108
Google Scholar
[21] Chen J, Liu J, Chen S G 2000 Phys. Rev. A 61 033402
Google Scholar
[22] Hao X, Wang G, Jia X, Li W, Liu J, Chen J 2009 Phys. Rev. A 80 023408
Google Scholar
[23] Quan W, Lin Z, Wu M, Kang H, Liu H, Liu X, Chen J, Liu J, He X T, Chen S G, Xiong H, Guo L, Xu H, Fu Y, Cheng Y, Xu Z Z 2009 Phys. Rev. Lett. 103 093001
Google Scholar
[24] Wang Y, Xu S, Chen Y, et al. 2017 Phys. Rev. A 95 063415
Google Scholar
[25] Ammosov M V, Delone N B, Krainov V P 1986 Sov. Phys. JETP 664 138
[26] Delone N B, Krainov V P 1991 J. Opt. Soc. Am. B 8 1207
Google Scholar
[27] Ye D F, Liu X, Liu J 2008 Phys. Rev. Lett. 101 233003
Google Scholar
[28] Chen J, Liu J, Zheng W M 2002 Phys. Rev. A 66 043410
Google Scholar
[29] Fu L B, Liu J, Chen J, Chen S G 2001 Phys. Rev. A 63 043416
Google Scholar
[30] Chen J, Liu J, Fu L B, Zheng W M 2000 Phys. Rev. A 63 011404
Google Scholar
[31] Brabec T, Ivanov M Y, Corkum P B 1996 Phys. Rev. A 54 R2551
Google Scholar
[32] Hu B, Liu J, Chen S G 1997 Phys. Lett. A 236 533
Google Scholar
[33] Han M, Li M, Liu M-M, Liu Y 2017 Phys. Rev. A 95 023406
Google Scholar
[34] Li M, Liu M M, Geng J W, Han M, Sun X, Shao Y, Deng Y, Wu C, Peng L Y, Gong Q, Liu Y 2017 Phys. Rev. A 95 053425
Google Scholar
[35] Becker W, Grasbon F, Kopold R, Milošević D B, Paulus G G, Walther H 2002 Adv. At. Mol. Opt. Phys. 48 35
Google Scholar
[36] Salières P, Carré B, Le Déroff L, Grasbon F, Paulus G G, Walther H, Kopold R, Becker W, Milošević D B, Sanpera A, Lewenstein M 2001 Science 292 902
Google Scholar
[37] Cloux F, Fabre B, Pons B 2015 Phys. Rev. A 91 023415
Google Scholar
[38] Muller H G 1999 Phys. Rev. A 60 1341
Google Scholar
[39] Dimitrovski D, Martiny C P J, Madsen L B 2010 Phys. Rev. A 82 053404
Google Scholar
[40] Dimitrovski D, Abu-samha M, Madsen L B, Filsinger F, Meijer G, Küpper J, Holmegaard L, Kalhøj L, Nielsen J H, Stapelfeldt H 2011 Phys. Rev. A 83 023405
Google Scholar
[41] Xu S, Liu M, Hu S, Shu Z, Quan W, Xiao Z, Zhou Y, Wei M, Zhao M, Sun R, Wang Y, Hua L, Gong C, Lai X, Chen J, Liu X 2020 Phys. Rev. A 102 043104
Google Scholar
[42] Corkum P B, Burnett N H, Brunel F 1989 Phys. Rev. Lett. 62 1259
Google Scholar
[43] Corkum P B 1993 Phys. Rev. Lett. 71 1994
Google Scholar
[44] Hofmann C, Landsman A S, Keller U 2019 J. Mod. Opt. 66 1052
Google Scholar
下载:
计量
- 文章访问数: 4832
- PDF下载量: 249
- 被引次数: 0
