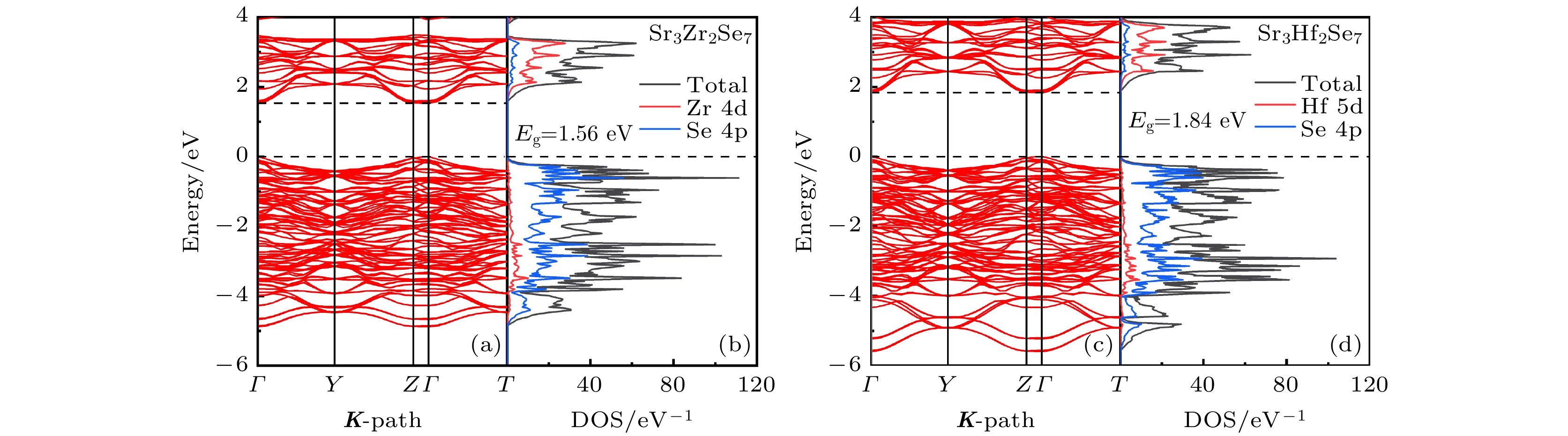
-
近年来, 层状钙钛矿材料中存在的非常规铁电性为新型铁电体设计提供了新的途径. 基于第一性原理, 本文系统研究了具有Ruddlesden-Popper (RP) (n = 2) 结构的Sr3B2Se7 (B = Zr, Hf)化合物的基态结构、电子结构和非常规铁电性. 研究表明, Sr3B2Se7 (B = Zr, Hf) 基态均为具有A21am极性相的直接带隙半导体; 其非常规铁电性来源于BSe6八面体的两种旋转模式的耦合. 而且, 因具有较强的铁电极化值与可见光吸收带隙, Sr3B2Se7 (B = Zr, Hf)有望成为新一代铁电光伏材料.
-
关键词:
- 第一性原理 /
- Ruddlesden-Popper /
- 非常规铁电 /
- 铁电光伏
Recently, perovskite ferroelectric photovoltaic materials have been studied extensively. Traditional photovoltaic device usually uses the internal electric field formed by PN junction to realize the separation of photogenerated carriers to form the photovoltaic effect, while ferroelectric material, due to the existence of spontaneous polarization, can spontaneously realize the separation of photogenerated electrons and holes without the formation of PN junction, presenting the ferroelectric photovoltaic effect. Chalcogenide perovskite with suitable band gap and visible light absorption is expected to be a new generation of ferroelectric photovoltaic materials. However, its application is limited due to the lack of ferroelectric properties. Hybrid improper ferroelectricity (HIF) in layered perovskites has opened a new way for developing the new ferroelectrics. In contrast to the proper ferroelectricity in which the polarization is the main order parameter as the driving force, the improper ferroelectricity possesses the ferroelectric polarization that becomes a secondary order parameter induced by other orders. In this work, we study the ground state, electronic structure and hybrid improper ferroelectricity of n = 2 Ruddlesden-Popper (RP) Sr3B2Se7 (B = Zr, Hf ) based on the first principles. The total energy calculations and phonon spectrum analysis show that the ground state of Sr3B2Se7 (B = Zr, Hf ) is of A21am polar phase. The hybrid improper ferroelectricity originates from the coupling between two rotation modes of BSe6 octahedron. Electronic structure calculations show that Sr3Zr2Se7 and Sr3Hf2Se7 are semiconductors with direct band-gaps, which are around 1.56 eV and 1.84 eV, respectively. The ferroelectric polarization values calculated by the Berry phase method are around 12.75 μC/cm2 and 9.69 μC/cm2, respectively. The contribution of each atomic layer to the ferroelectric polarization is investigated when the Born effective charge method is used. The results show that the polarization of Sr3B2Se7 (B = Zr, Hf ) mainly comes from the Sr-Se atomic layers. To sum up, Sr3B2Se7 (B = Zr, Hf ) show strong ferroelectric polarization and good visible light absorption characteristics and they are expected to be candidates of a new generation of ferroelectric photovoltaic materials.-
Keywords:
- first-principle /
- Ruddlesden-Popper /
- hybrid improper ferroelectricity /
- ferroelectric photovoltaic
[1] Zhou H, Chen Q, Li G, Luo S, Song T B, Duan H S, Hong Z, You J, Liu Y, Yang Y 2014 Science 345 542
Google Scholar
[2] Grinberg I, West D V, Torres M, Gou G, Stein D M, Wu L, Chen G, Gallo E M, Akbashev A R, Davies P K, Spanier J E, Rappe A M 2013 Nature 503 509
Google Scholar
[3] Zheng F, Takenaka H, Wang F, Koocher N Z, Rappe A M 2015 J. Phys. Chem. Lett 6 31
Google Scholar
[4] Yang S Y, Seidel J, Byrnes S J, Shafer P, Yang C H, Rossell M D, Yu P, Chu Y H, Scott J F, Ager J W, Martin L W, Ramesh R 2010 Nat. Nanotechnol. 5 143
Google Scholar
[5] Shockley W, Queisser H J 1961 J. Appl. Phys. 32 510
Google Scholar
[6] Xu X S, Ihlefeld J F, Lee J H, Ezekoye O K, Vlahos E, Ramesh R, Gopalan V, Pan X Q, Schlom D G, Musfeldt J L 2010 Appl. Phys. Lett. 96 192901
Google Scholar
[7] Nechache R, Harnagea C, Li S, Cardenas L, Huang W, Chakrabartty J, Rosei F 2015 Nat. Photonics 9 61
Google Scholar
[8] Choi K, Jang E S 2012 Electron. Lett. 48 689
Google Scholar
[9] Nechache R, Harnagea C, Licoccia S, Traversa E, Ruediger A, Pignolet A, Rosei F 2011 Appl. Phys. Lett. 98 202902
Google Scholar
[10] Zhou W, Deng H, Yang P, Chu J 2014 Appl. Phys. Lett. 105 111904
Google Scholar
[11] Bennett J W, Grinberg I, Rappe A M 2008 J. Am. Chem. Soc. 130 17409
Google Scholar
[12] Guo R, You L, Zhou Y, Shiuh L Z, Zou X, Chen L, Ramesh R, Wang J 2013 Nat. Commun. 4 1990
Google Scholar
[13] Zhang J, Su X, Shen M, Dai Z, Zhang L, He X, Cheng W, Cao M, Zou G 2013 Sci. Rep. 3 2109
Google Scholar
[14] Huang H 2010 Nat. Photonics 4 134
Google Scholar
[15] Arima T H 2007 J. Phys. Soc. Jpn. 76 073702
Google Scholar
[16] Wilkins S B, Forrest T R, Beale T A W, Bland S R, Walker H C, Mannix D, Yakhou F, Prabhakaran D, Boothroyd A T, Hill J P, Hatton P D, McMorrow D F 2009 Phys. Rev. Lett. 103 207602
Google Scholar
[17] Naito Y, Sato K, Yasui Y, Kobayashi Y, Kobayashi Y, Sato M 2007 J. Phys. Soc. Jpn. 76 023708
Google Scholar
[18] Xiang H J, Kan E J, Zhang Y, Whangbo M H, Gong X G 2011 Phys. Rev. Lett. 107 157202
Google Scholar
[19] Dong S, Liu J M, Cheong S W, Ren Z 2015 Adv. Phys 64 519
Google Scholar
[20] Bousquet E, Dawber M, Stucki N, Lichtensteiger C, Hermet P, Gariglio S, Triscone J M, Ghosez P 2008 Nature 452 732
Google Scholar
[21] Benedek N A, Fennie C J 2011 Phys. Rev. Lett. 106 107204
Google Scholar
[22] Zhao H J, Íñiguez J, Ren W, Chen X M, Bellaiche L 2014 Phys. Rev. B 89 174101
Google Scholar
[23] Pitcher M J, Mandal P, Dyer M S, Alaria J, Borisov P, Niu H, Claridge J B, Rosseinsky M J 2015 Science 347 420
Google Scholar
[24] Liu X Q, Wu J W, Shi X X, Zhao H J, Zhou H Y, Qiu R H, Zhang W Q, Chen X M 2015 Appl. Phys. Lett. 106 202903
Google Scholar
[25] Li G J, Liu X Q, Lu J J, Zhu H Y, Chen X M 2018 J. Appl. Phys. 123 014101
Google Scholar
[26] Yoshida S, Fujita K, Akamatsu H, Hernandez O, Sen Gupta A, Brown F G, Padmanabhan H, Gibbs A S, Kuge T, Tsuji R, Murai S, Rondinelli J M, Gopalan V, Tanaka K 2018 Adv. Funct. Mater. 28 1801856
Google Scholar
[27] Xu X, Wang Y, Huang F T, Du K, Nowadnick E A, Cheong S W 2020 Adv. Funct. Mater. 30 2003623
Google Scholar
[28] Liu X Q, Chen B H, Lu J J, Hu Z Z, Chen X M 2018 Appl. Phys. Lett. 113 242904
Google Scholar
[29] Yoshida S, Akamatsu H, Tsuji R, Hernandez O, Padmanabhan H, Sen Gupta A, Gibbs A S, Mibu K, Murai S, Rondinelli J M, Gopalan V, Tanaka K, Fujita K 2018 J. Am. Chem. Soc. 140 15690
Google Scholar
[30] Ishikawa A, Takata T, Kondo J N, Hara M, Kobayashi H, Domen K 2002 J. Am. Chem. Soc. 124 13547
Google Scholar
[31] Meng W, Saparov B, Hong F, Wang J, Mitzi D B, Yan Y 2016 Chem. Mater. 28 821
Google Scholar
[32] Bennett J W, Grinberg I, Rappe A M 2009 Phys. Rev. B 79 235115
Google Scholar
[33] Rondinelli J M, Fennie C J 2012 Adv. Mater. 24 1961
Google Scholar
[34] Wang H, Gou G, Li J 2016 Nano Energy 22 507
Google Scholar
[35] Zhang Y, Shimada T, Kitamura T, Wang J 2017 J. Phys. Chem. Lett 8 5834
Google Scholar
[36] Zhang Y, Sahoo M P K, Shimada T, Kitamura T, Wang J 2017 Phys. Rev. B 96 144110
Google Scholar
[37] Li W, Niu S, Zhao B, Haiges R, Zhang Z, Ravichandran J, Janotti A 2019 Phys. Rev. Mater. 3 101601
Google Scholar
[38] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[39] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[40] Perdew J P, Ruzsinszky A, Csonka G I, Vydrov O A, Scuseria G E, Constantin L A, Zhou X, Burke K 2008 Phys. Rev. Lett. 100 136406
Google Scholar
[41] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[42] Baroni S, de Gironcoli S, Dal Corso A, Giannozzi P 2001 Rev. Mod. Phys. 73 515
Google Scholar
[43] Gonze X 1995 Phys. Rev. A 52 1096
Google Scholar
[44] Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207
Google Scholar
[45] Spaldin N A 2012 J. Solid State Chem. 195 2
Google Scholar
[46] Gonze X, Lee C 1997 Phys. Rev. B 55 10355
Google Scholar
[47] Ruddlesden S N, Popper P 1958 Acta Crystallogr. 11 54
Google Scholar
[48] Glazer A 1972 Acta Crystallogr. B 28 3384
Google Scholar
-
图 1 Sr3B2Se7结构示意图 (a) I4/mmm (No. 139)相结构; (b) A21am (No. 36)相结构. P表示钙钛矿结构单元, R表示岩盐层结构单元, 绿球和红球分别表示Sr原子和Se原子
Fig. 1. Schematic of the crystal structures of Ruddlesden-Popper Sr3B2Se7: (a) I4/mmm (No. 139); (b) A21am (No. 36) phases. Perovskite (P) and rocksalt (R) blocks are marked, and the green and red balls represents Sr and Se atoms in Sr3B2Se7 respectively.
图 2 (a), (b) Sr3Zr2Se7和Sr3Hf2Se7 I4/mmm (No. 139)的相声子谱; (c), (d) Sr3Zr2Se7和Sr3Hf2Se7 A21am (No. 36)的铁电相声子谱
Fig. 2. Calculated phonon dispersion curves of (a) Sr3Zr2Se7 and (b) Sr3Hf2Se7 with I4/mmm (No. 139) phase respectively; calculated phonon dispersion curves of (c) Sr3Zr2Se7 and (d) Sr3Hf2Se7 with ferroelectric A21am (No. 36) phase.
图 3 (a), (b)结构总能随八面体两种旋转模式振幅(
$ {Q}_{{{X}}_{2}^{+}} $ ,$ {Q}_{{{X}}_{3}^{-}} $ )的变化曲线; (c) 总能在不同极性位移模式振幅($ {Q}_{{\varGamma }_{5}^{-}} $ )下, 随不同($ {Q}_{{{X}}_{2}^{+}} $ ,$ {Q}_{{{X}}_{3}^{-}} $ )振幅组合的变化曲线Fig. 3. (a), (b) Energy variations as functions of rotation mode amplitude (
$ {Q}_{{{X}}_{2}^{+}} $ ) and rotation mode amplitude ($ {Q}_{{{X}}_{3}^{-}} $ ) of octahedral; (c) the amplitude of polar$ {Q}_{{\varGamma }_{5}^{-}} $ mode for various sets of$ {Q}_{{{X}}_{2}^{+}} $ and$ {Q}_{{{X}}_{3}^{-}} $ .
图 4 (a), (c) Sr3Zr2Se7和Sr3Hf2Se7铁电相的能带结构; (b), (d) Sr3Zr2Se7和Sr3Hf2Se7铁电相的态密度
Fig. 4. (a), (c) The band structures of Sr3Zr2Se7 and Sr3Hf2Se7 ferroelectric phases respectively; (b), (d) the density of states of Sr3Zr2Se7 and Sr3Hf2Se7 ferroelectric phases respectively.
图 5 (a) Sr3Hf2Se7的A21am铁电相结构的原子位移图; (b)使用BEC方法计算的Sr3Hf2Se7的A21am铁电相结构中每层原子位移对铁电极化的贡献, Sr-Se1表示钙钛矿之间的SrSe原子层, Sr-Se2表示岩盐矿层
Fig. 5. (a) Cation displacement of the ferroelectric A21am phase of Sr3Hf2Se7; (b) contribution of atomic displacement of each layer to ferroelectric polarization in the A21am ferroelectric phase of Sr3Hf2Se7 calculated by BEC method. Sr-Se1 represents the SrSe atomic layer between perovskites, and SrSe2 represents the rock salt layer
表 1 Sr3B2Se7无畸变顺电相I4/mmm结构经受原子极性位移或不同模式的八面体旋转而形成的所有可能结构, 及其与I4/mmm结构的能量差
Table 1. All possible structures derived from the distortionless paraelectric prototype I4/mmm structure of Sr3B2Se7 under atomic polar displacement or different octahedral rotations, and the energy differences between the abovementioned structures and I4/mmm structure.
空间群 相对能量ΔE/(meV·f.u.–1) 声子软模 Sr3Zr2Se7 Sr3Hf2Se7 I4/mmm (No. 139) 1349.1 1236.30 — Fmm2 (No. 42) 1217.5 1144.50 $ {\varGamma }_{5}^{-} $(a, a) Acaa (No. 68) 529.9 483.15 $ {X}_{1}^{-} $(a, 0) Acam (No. 64) 561.2 518.70 $ {X}_{2}^{+} $(a, 0) P4/mbm (No. 127) 1113.4 1006.00 $ {X}_{2}^{+} $(a, a) Pbam (No. 55) 561.4 518.70 $ {X}_{2}^{+} $(a, b) Amam (No. 63) 522.1 474.60 $ {X}_{3}^{-} $(a, 0) P42/mnm (No. 136) 385.7 363.10 $ {X}_{3}^{-} $(a, a) Pnnm (No. 58) 381.0 359.20 $ {X}_{3}^{-} $(a, b) P42/mcn (No. 132) 557.2 524.10 $ {X}_{4}^{-} $(a, a) B2/b (No. 15) 342.6 320.80 $ {X}_{1}^{-}\oplus {X}_{3}^{-} $(a, 0; b, 0) Pnab (No. 60) 315.9 299.20 $ {\rm{X}}_{1}^{-}\oplus {X}_{3}^{-} $(0, a; b, 0) P2/c (No. 13) 438.6 409.20 $ {X}_{1}^{-}\oplus {X}_{4}^{-} $(a, 0; b, 0) Pbaa (No. 54) 442.8 408.70 $ {X}_{1}^{-}\oplus {X}_{4}^{-} $(0, a; b, 0) A21am (No. 36) 0 0 $ {X}_{2}^{+}\oplus {X}_{3}^{-} $(a, 0; b, 0) Pbnm (No. 62) 35.2 30.70 $ {X}_{2}^{+}\oplus {X}_{3}^{-} $(0, a; b, 0) B2cm (No. 39) 173.6 156.5 $ {X}_{2}^{+}\oplus {X}_{4}^{-} $(a, 0; b, 0) A2/m (No. 12) 383.9 366.1 $ {X}_{3}^{-}\oplus {X}_{4}^{-} $(a, 0; b, 0) 
下载: 导出CSV
表 2 分别利用Berry相与BEC法计算的Sr3B2Se7 (B = Zr, Hf ) 材料铁电极化值
Table 2. Sr3B2Se7 (B = Zr, Hf ) ferroelectric polarization calculated by Berry phase and BEC methods.
Berry相 /(μC·cm–2) BEC /(μC·cm–2) Sr3Zr2Se7 12.75 11.12 Sr3Hf2Se7 9.69 8.14
下载: 导出CSV
-
[1] Zhou H, Chen Q, Li G, Luo S, Song T B, Duan H S, Hong Z, You J, Liu Y, Yang Y 2014 Science 345 542
Google Scholar
[2] Grinberg I, West D V, Torres M, Gou G, Stein D M, Wu L, Chen G, Gallo E M, Akbashev A R, Davies P K, Spanier J E, Rappe A M 2013 Nature 503 509
Google Scholar
[3] Zheng F, Takenaka H, Wang F, Koocher N Z, Rappe A M 2015 J. Phys. Chem. Lett 6 31
Google Scholar
[4] Yang S Y, Seidel J, Byrnes S J, Shafer P, Yang C H, Rossell M D, Yu P, Chu Y H, Scott J F, Ager J W, Martin L W, Ramesh R 2010 Nat. Nanotechnol. 5 143
Google Scholar
[5] Shockley W, Queisser H J 1961 J. Appl. Phys. 32 510
Google Scholar
[6] Xu X S, Ihlefeld J F, Lee J H, Ezekoye O K, Vlahos E, Ramesh R, Gopalan V, Pan X Q, Schlom D G, Musfeldt J L 2010 Appl. Phys. Lett. 96 192901
Google Scholar
[7] Nechache R, Harnagea C, Li S, Cardenas L, Huang W, Chakrabartty J, Rosei F 2015 Nat. Photonics 9 61
Google Scholar
[8] Choi K, Jang E S 2012 Electron. Lett. 48 689
Google Scholar
[9] Nechache R, Harnagea C, Licoccia S, Traversa E, Ruediger A, Pignolet A, Rosei F 2011 Appl. Phys. Lett. 98 202902
Google Scholar
[10] Zhou W, Deng H, Yang P, Chu J 2014 Appl. Phys. Lett. 105 111904
Google Scholar
[11] Bennett J W, Grinberg I, Rappe A M 2008 J. Am. Chem. Soc. 130 17409
Google Scholar
[12] Guo R, You L, Zhou Y, Shiuh L Z, Zou X, Chen L, Ramesh R, Wang J 2013 Nat. Commun. 4 1990
Google Scholar
[13] Zhang J, Su X, Shen M, Dai Z, Zhang L, He X, Cheng W, Cao M, Zou G 2013 Sci. Rep. 3 2109
Google Scholar
[14] Huang H 2010 Nat. Photonics 4 134
Google Scholar
[15] Arima T H 2007 J. Phys. Soc. Jpn. 76 073702
Google Scholar
[16] Wilkins S B, Forrest T R, Beale T A W, Bland S R, Walker H C, Mannix D, Yakhou F, Prabhakaran D, Boothroyd A T, Hill J P, Hatton P D, McMorrow D F 2009 Phys. Rev. Lett. 103 207602
Google Scholar
[17] Naito Y, Sato K, Yasui Y, Kobayashi Y, Kobayashi Y, Sato M 2007 J. Phys. Soc. Jpn. 76 023708
Google Scholar
[18] Xiang H J, Kan E J, Zhang Y, Whangbo M H, Gong X G 2011 Phys. Rev. Lett. 107 157202
Google Scholar
[19] Dong S, Liu J M, Cheong S W, Ren Z 2015 Adv. Phys 64 519
Google Scholar
[20] Bousquet E, Dawber M, Stucki N, Lichtensteiger C, Hermet P, Gariglio S, Triscone J M, Ghosez P 2008 Nature 452 732
Google Scholar
[21] Benedek N A, Fennie C J 2011 Phys. Rev. Lett. 106 107204
Google Scholar
[22] Zhao H J, Íñiguez J, Ren W, Chen X M, Bellaiche L 2014 Phys. Rev. B 89 174101
Google Scholar
[23] Pitcher M J, Mandal P, Dyer M S, Alaria J, Borisov P, Niu H, Claridge J B, Rosseinsky M J 2015 Science 347 420
Google Scholar
[24] Liu X Q, Wu J W, Shi X X, Zhao H J, Zhou H Y, Qiu R H, Zhang W Q, Chen X M 2015 Appl. Phys. Lett. 106 202903
Google Scholar
[25] Li G J, Liu X Q, Lu J J, Zhu H Y, Chen X M 2018 J. Appl. Phys. 123 014101
Google Scholar
[26] Yoshida S, Fujita K, Akamatsu H, Hernandez O, Sen Gupta A, Brown F G, Padmanabhan H, Gibbs A S, Kuge T, Tsuji R, Murai S, Rondinelli J M, Gopalan V, Tanaka K 2018 Adv. Funct. Mater. 28 1801856
Google Scholar
[27] Xu X, Wang Y, Huang F T, Du K, Nowadnick E A, Cheong S W 2020 Adv. Funct. Mater. 30 2003623
Google Scholar
[28] Liu X Q, Chen B H, Lu J J, Hu Z Z, Chen X M 2018 Appl. Phys. Lett. 113 242904
Google Scholar
[29] Yoshida S, Akamatsu H, Tsuji R, Hernandez O, Padmanabhan H, Sen Gupta A, Gibbs A S, Mibu K, Murai S, Rondinelli J M, Gopalan V, Tanaka K, Fujita K 2018 J. Am. Chem. Soc. 140 15690
Google Scholar
[30] Ishikawa A, Takata T, Kondo J N, Hara M, Kobayashi H, Domen K 2002 J. Am. Chem. Soc. 124 13547
Google Scholar
[31] Meng W, Saparov B, Hong F, Wang J, Mitzi D B, Yan Y 2016 Chem. Mater. 28 821
Google Scholar
[32] Bennett J W, Grinberg I, Rappe A M 2009 Phys. Rev. B 79 235115
Google Scholar
[33] Rondinelli J M, Fennie C J 2012 Adv. Mater. 24 1961
Google Scholar
[34] Wang H, Gou G, Li J 2016 Nano Energy 22 507
Google Scholar
[35] Zhang Y, Shimada T, Kitamura T, Wang J 2017 J. Phys. Chem. Lett 8 5834
Google Scholar
[36] Zhang Y, Sahoo M P K, Shimada T, Kitamura T, Wang J 2017 Phys. Rev. B 96 144110
Google Scholar
[37] Li W, Niu S, Zhao B, Haiges R, Zhang Z, Ravichandran J, Janotti A 2019 Phys. Rev. Mater. 3 101601
Google Scholar
[38] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[39] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[40] Perdew J P, Ruzsinszky A, Csonka G I, Vydrov O A, Scuseria G E, Constantin L A, Zhou X, Burke K 2008 Phys. Rev. Lett. 100 136406
Google Scholar
[41] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[42] Baroni S, de Gironcoli S, Dal Corso A, Giannozzi P 2001 Rev. Mod. Phys. 73 515
Google Scholar
[43] Gonze X 1995 Phys. Rev. A 52 1096
Google Scholar
[44] Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207
Google Scholar
[45] Spaldin N A 2012 J. Solid State Chem. 195 2
Google Scholar
[46] Gonze X, Lee C 1997 Phys. Rev. B 55 10355
Google Scholar
[47] Ruddlesden S N, Popper P 1958 Acta Crystallogr. 11 54
Google Scholar
[48] Glazer A 1972 Acta Crystallogr. B 28 3384
Google Scholar
下载:
计量
- 文章访问数: 4901
- PDF下载量: 109
- 被引次数: 0
