-
相比于传统基于毛细管或针孔的 X 射线成像系统, 编码孔径成像系统具有结构简单、灵敏度高、扩展性强等优势,使其在 X 射线荧光成像中极具潜力. 本工作应用新型编码孔径成像计算模型, 设计了一种基于可分离编码的X射线成像系统. 利用Geant4蒙特卡罗仿真对系统的性能进行了研究, 并根据快速迭代收缩阈值算法进行了图像重建. 模拟及分析结果显示, 近场成像时, 与传统基于卷积模型的成像系统不同, 该系统的性能不受准直效应的影响. 成像系统的空间分辨率约为65 μm, 并能够准确地重建出不同能量的线源和形状复杂物体的图像. 重建图像的质量受校准时所用X射线能量和物体发射X射线能量的影响, 两者差异越小, 重建图像的质量越高. 三维重建结果显示, 系统能够从单次获取的二维投影图像, 正确地重建出物体与系统的距离, 轴向空间分辨率约为1.1 mm.Compared with traditional X-ray imaging systems based on polycapillary X-ray optics or a pinhole, coded aperture imaging system has the advantages in simple structure, high sensitivity, and strong expandability, which make it possess the potential applications in X-ray fluorescence imaging. In this work, a new coded aperture X-ray imaging system based on a novel imaging model which decomposes the mask projections into a superposition of two separable functions is designed and proposed for high-resolution X-ray imaging. The performance of the system is demonstrated by using the Geant4 package. To reduce the computational complexity of calibration and image reconstruction, a separable mask with 90 × 90 pixels is used. The mask is designed by selecting the central part of the original rank 463 modified uniformly redundant arrays. The mask is made of platinum foil with a pixel pitch of 25 microns. To study the effect of mask thickness on system performance, the mask thickness is varied from 25 to 200 microns. The active area of the Si detector employed in the system is 2 mm × 2 mm, divided into 80 × 80 pixels, each with a size of 25 μm × 25 μm. The field of view of the system is equal to the area of the detector, which is 2 mm × 2 mm. The detector is parallel to and center-aligned with the mask with a fixed distance of 2.0 mm. The images are reconstructed by using the fast iterative shrinkage-thresholding algorithm. The high-quality reconstructed images of different energy line sources and complex shaped objects are obtained. The simulation and analysis results indicate that for the near-field imaging, unlike imaging systems based on the conventional convolution model, the system has the performance that is not affected by the aperture collimation effect. The spatial resolution of the imaging system is about 65 microns. The calibrated matrices used have an important influence on the image quality. The quality of the reconstructed image is affected by the energy of X-rays used during calibration and the energy of X-rays emitted from the object; the smaller the difference between these two energy values, the higher the quality of the reconstructed images will be. The three-dimensional reconstruction results show that the system can correctly estimate the distance between the object and the system from a single two-dimensional projection. The axial spatial resolution of the system is about 1.1 mm.
-
Keywords:
- coded aperture /
- X-ray fluorescence imaging /
- Monte Carlo simulation /
- image reconstruction
[1] Terzano R, Denecke M A, Falkenberg G, Miller B, Paterson D, Janssens K 2019 Pure Appl. Chem. 91 1029
 Google Scholar
Google Scholar
[2] Romano F P, Caliri C, Cosentino L, Gammino S, Giuntini L, Mascali D, Neri L, Pappalardo L, Rizzo F, Taccetti F 2014 Anal. Chem. 86 10892
 Google Scholar
Google Scholar
[3] Tsuji K, Matsuno T, Takimoto Y, Yamanashi M, Kometani N, Sasaki Y C, Hasegawa T, Kato S, Yamada T, Shoji T, Kawahara N 2015 Spectrom. Acta B 113 43
 Google Scholar
Google Scholar
[4] Buzanich G, Radtke M, Reinholz U, Riesemeier H, Thünemann A F, Streli C 2012 J. Anal. At. Spectrom. 27 1875
 Google Scholar
Google Scholar
[5] Rauwolf M, Turyanskaya A, Roschger A, Prost J, Simon R, Scharf O, Radtke M, Schoonjans T, Buzanich A G, Klaushofer K, Wobrauschek P, Hofstaetter J G, Roschger P, Streli C 2017 J. Synchrotron Radiat. 24 307
 Google Scholar
Google Scholar
[6] Zhang H, Jiang S W, Liao J, Deng J J, Liu J, Zhang Y B, Zheng G A 2019 Opt. Express 27 7498
 Google Scholar
Google Scholar
[7] 强鹏飞, 盛立志, 李林森, 闫永清, 刘哲, 周晓红 2019 68 160702
 Google Scholar
Google Scholar
Qiang P F, Sheng L Z, Li L S, Yan Y Q, Liu Z, Zhou X H 2019 Acta Phys. Sin. 68 160702
 Google Scholar
Google Scholar
[8] Dicke R H 1968 Astrophys. J. 153 L101
 Google Scholar
Google Scholar
[9] Ables J G 1968 Astron. Soc. Aust. 1 172
 Google Scholar
Google Scholar
[10] Cieślak M J, Gamage K A A, Glover R 2016 Radiat. Meas. 92 59
 Google Scholar
Google Scholar
[11] Liu Y T, Xiao X, Zhang Z M, Wei L 2020 Nucl. Instrum. Methods Phys. Res. A 957 163385
 Google Scholar
Google Scholar
[12] Sun S F, Liu Y, Ouyang X P 2020 Radiat. Phys. Chem. 174 108891
 Google Scholar
Google Scholar
[13] Haboub A, MacDowell A A, Marchesini S, Parkinson D Y 2014 Rev. Sci. Instrum. 85 063704
 Google Scholar
Google Scholar
[14] Kulow A, Buzanich A G, Reinholz U, Streli C, Radtke M 2020 J. Anal. At. Spectrom. 35 347
 Google Scholar
Google Scholar
[15] DeWeert M J, Farm B P 2015 Opt. Eng. 54 023102
 Google Scholar
Google Scholar
[16] Asif M S, Ayremlou A, Sankaranarayanan A, Veeraraghavan A, Baraniuk R G 2017 IEEE Trans. Comput. Imaging 3 384
 Google Scholar
Google Scholar
[17] Adams J K, Boominathan V, Avants B W, Vercosa D G, Ye F, Baraniuk R G, Robinson J T, Veeraraghavan A 2017 Sci. Adv. 3 e1701548
 Google Scholar
Google Scholar
[18] Sun S F, Liu Y, Ouyang X P 2020 Nucl. Instrum. Methods Phys. Res. A 951 163001
 Google Scholar
Google Scholar
[19] Beck A, Teboulle M 2009 SIAM J. Imaging Sci. 2 183
 Google Scholar
Google Scholar
[20] Allison J, Amako K, Apostolakis J, Arce P, Asai M, Aso T, Bagli E, Bagulya A, Banerjee S, Barrand G 2016 Nucl. Instrum. Methods Phys. Res. A 835 186
 Google Scholar
Google Scholar
[21] Wang Z, Bovik A C 2002 IEEE Signal Process. Lett. 9 81
 Google Scholar
Google Scholar
-
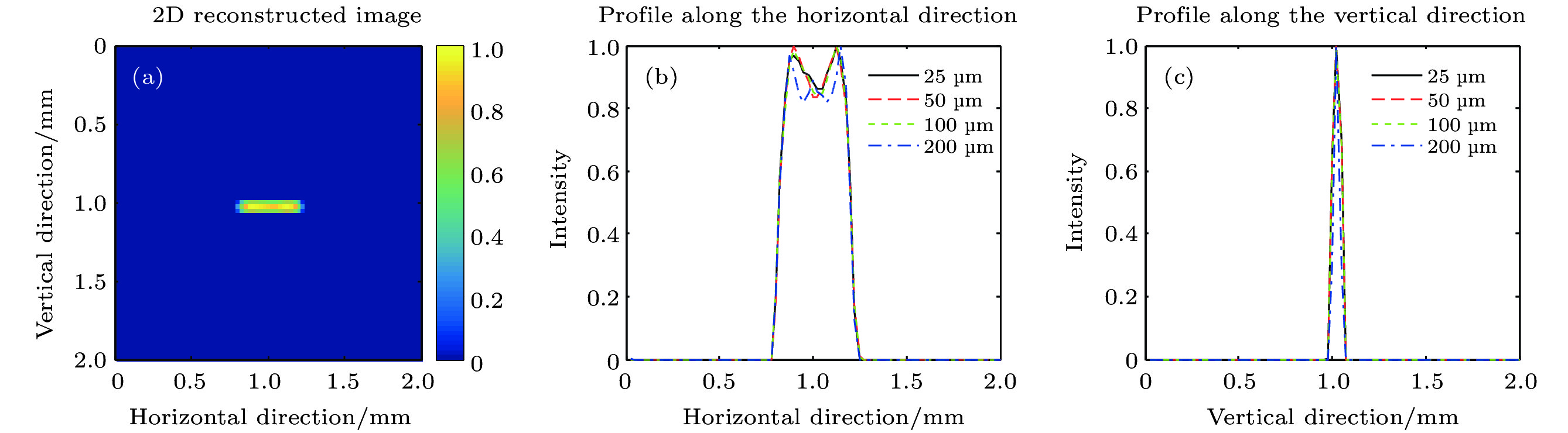
图 3 线源二维重建图像及其沿横纵向分布 (a)准直器厚度25微米时的线源重建图像; (b)不同准直器厚度时的横向分布; (c)不同准直器厚度时的纵向分布
Fig. 3. 2 d reconstructed image of the line source and its horizontal and vertical distribution: (a) Reconstructed image of the line source when the mask thickness was 25 micron; (b) horizontal distribution for different mask thickness; (c) vertical distribution for different mask thickness.
-
[1] Terzano R, Denecke M A, Falkenberg G, Miller B, Paterson D, Janssens K 2019 Pure Appl. Chem. 91 1029
 Google Scholar
Google Scholar
[2] Romano F P, Caliri C, Cosentino L, Gammino S, Giuntini L, Mascali D, Neri L, Pappalardo L, Rizzo F, Taccetti F 2014 Anal. Chem. 86 10892
 Google Scholar
Google Scholar
[3] Tsuji K, Matsuno T, Takimoto Y, Yamanashi M, Kometani N, Sasaki Y C, Hasegawa T, Kato S, Yamada T, Shoji T, Kawahara N 2015 Spectrom. Acta B 113 43
 Google Scholar
Google Scholar
[4] Buzanich G, Radtke M, Reinholz U, Riesemeier H, Thünemann A F, Streli C 2012 J. Anal. At. Spectrom. 27 1875
 Google Scholar
Google Scholar
[5] Rauwolf M, Turyanskaya A, Roschger A, Prost J, Simon R, Scharf O, Radtke M, Schoonjans T, Buzanich A G, Klaushofer K, Wobrauschek P, Hofstaetter J G, Roschger P, Streli C 2017 J. Synchrotron Radiat. 24 307
 Google Scholar
Google Scholar
[6] Zhang H, Jiang S W, Liao J, Deng J J, Liu J, Zhang Y B, Zheng G A 2019 Opt. Express 27 7498
 Google Scholar
Google Scholar
[7] 强鹏飞, 盛立志, 李林森, 闫永清, 刘哲, 周晓红 2019 68 160702
 Google Scholar
Google Scholar
Qiang P F, Sheng L Z, Li L S, Yan Y Q, Liu Z, Zhou X H 2019 Acta Phys. Sin. 68 160702
 Google Scholar
Google Scholar
[8] Dicke R H 1968 Astrophys. J. 153 L101
 Google Scholar
Google Scholar
[9] Ables J G 1968 Astron. Soc. Aust. 1 172
 Google Scholar
Google Scholar
[10] Cieślak M J, Gamage K A A, Glover R 2016 Radiat. Meas. 92 59
 Google Scholar
Google Scholar
[11] Liu Y T, Xiao X, Zhang Z M, Wei L 2020 Nucl. Instrum. Methods Phys. Res. A 957 163385
 Google Scholar
Google Scholar
[12] Sun S F, Liu Y, Ouyang X P 2020 Radiat. Phys. Chem. 174 108891
 Google Scholar
Google Scholar
[13] Haboub A, MacDowell A A, Marchesini S, Parkinson D Y 2014 Rev. Sci. Instrum. 85 063704
 Google Scholar
Google Scholar
[14] Kulow A, Buzanich A G, Reinholz U, Streli C, Radtke M 2020 J. Anal. At. Spectrom. 35 347
 Google Scholar
Google Scholar
[15] DeWeert M J, Farm B P 2015 Opt. Eng. 54 023102
 Google Scholar
Google Scholar
[16] Asif M S, Ayremlou A, Sankaranarayanan A, Veeraraghavan A, Baraniuk R G 2017 IEEE Trans. Comput. Imaging 3 384
 Google Scholar
Google Scholar
[17] Adams J K, Boominathan V, Avants B W, Vercosa D G, Ye F, Baraniuk R G, Robinson J T, Veeraraghavan A 2017 Sci. Adv. 3 e1701548
 Google Scholar
Google Scholar
[18] Sun S F, Liu Y, Ouyang X P 2020 Nucl. Instrum. Methods Phys. Res. A 951 163001
 Google Scholar
Google Scholar
[19] Beck A, Teboulle M 2009 SIAM J. Imaging Sci. 2 183
 Google Scholar
Google Scholar
[20] Allison J, Amako K, Apostolakis J, Arce P, Asai M, Aso T, Bagli E, Bagulya A, Banerjee S, Barrand G 2016 Nucl. Instrum. Methods Phys. Res. A 835 186
 Google Scholar
Google Scholar
[21] Wang Z, Bovik A C 2002 IEEE Signal Process. Lett. 9 81
 Google Scholar
Google Scholar
计量
- 文章访问数: 9109
- PDF下载量: 148
- 被引次数: 0














 下载:
下载: